WBCHSE Class 11 Physics Notes For Newton’s Laws Of Gravitation

Newton’s Laws Of Gravitation

Sir Isaac Newton analysed these laws of planetary motion and formulated the nature of the force of attraction between the sun and the planets and at the same time established a rule for the measurement of this force. This force of attraction is called gravitation, which acts not only between the sun and the planets but also between any two particles in the universe.

Newton’s Law of Gravitation Definition: The force of attraction between any two particles in the universe is called gravitation.

The rule for the measurement of this force of attraction is known as Newton’s law of gravitation or the law of universal gravitation.

Newton’s Law of Gravitation Statement: Any two particles in the universe attract each other along a straight line joining them. The magnitude of this force of attraction is directly proportional to the product of the masses of the particles and inversely proportional to the square of the linear distance between them.

Let two particles of masses m₁ and m₂ be kept at a distance r from each other. If the the force of attraction between them is F, then as per Newton’s law of gravitation F ∝ m₁m₂, when r remains constant and \(\), when m₁ and m₂ remain unchanged.

Read and Learn More: Class 11 Physics Notes

Hence, \(F \propto \frac{m_1 m_2}{r^2} \text { or, } F=G \frac{m_1 m_2}{r^2}\)….(1)

G is called the universal gravitational constant or the gravitational constant. Equation (1) is the mathematical representation of the law of gravitation.

The masses m₁ and m₂ used in Newton’s law of gravitation are called gravitational masses. On the other hand, the mass referred to in Newton’s second law of motion is called inertial mass. Though the concept of these two different masses is derived from two different laws, experiments and observations show that the two masses are identical physical quantities.

Hence, it is not necessary to mention these two masses separately.

Vector Form Of The Law Of Gravitation: Let \(\vec{r_1}\) and \(\vec{r_2}\) be the position vectors of two particles of mass m₁ and m₂ respectively. So the position vector of m₂ with respect to m₁ and that of m₁ with respect to m₂ are respectively,

⇒ \(\vec{r}_{21}=\vec{r}_2-\vec{r}_1\)….(1)

and \(\vec{r}_{12}=\vec{r}_1-\vec{r}_2\)….(2)

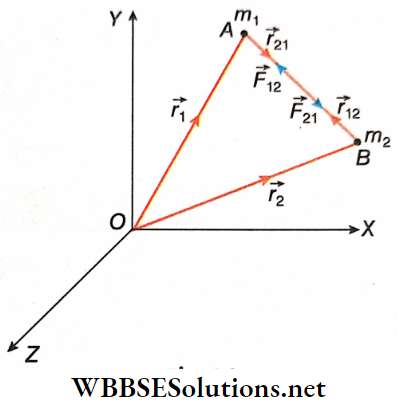
Let the unit vector along \(m_2\) from \(m_1\) is \(\hat{r}_{21}\)

and \(\left|\vec{r}_{21}\right|=r=\left|\vec{r}_{12}\right|\).

Therefore, \(\hat{r}_{21}=\frac{\vec{r}_{21}}{r}\) and similarly, \(\hat{r}_{12}=\frac{\vec{r}_{12}}{r}\)

Hence, according to Newton’s law of gravitation, the force on \(m_2\) due to \(m_1\) is,

⇒ \(\vec{F}_{12}=-G \frac{m_1 m_2}{\left|\overrightarrow{r_{21}}\right|^2} \hat{r}_{21}=-G \frac{m_1 m_2}{r^3} \vec{r}_{21}\)….(4)

Similarly, the force on \(m_1\) due to \(m_2\) is, \(\vec{F}_{21}=-G \frac{m_1 m_2}{\left|\vec{r}_{12}\right|^2} \hat{r}_{12}=-G \frac{m_1 m_2}{r^3} \vec{r}_{12}\)

Equations (4) and (5) are the vector form of the law of gravitation.

Gravitational Force Between Two Parties Is Equal And Opposite: From equations (2) and (4) we get,

⇒ \(\vec{F}_{12}=-G \frac{m_1 m_2}{r^3}\left(\vec{r}_2-\vec{r}_1\right)=G \frac{m_1 m_2}{r^3}\left(\vec{r}_1-\vec{r}_2\right)\)

= \(G \frac{m_1 m_2}{r^3} \vec{r}_{12}\) [according to equation (3)]

= \(-\vec{F}_{21}\) [according to equaiton (5)]

Gravitational Constant: On substitution of m₁ = m₂ = 1 and r = 1, equation (1) is reduced to G = F. This defines the universal gravitational constant.

Gravitational Constant Definition: The mutually operative force of attraction between two particles of unit mass kept a unit distance apart is called the gravitational constant.

Unit: From equation (1),

unit of G = \(=\frac{\text { unit of } F \times(\text { unit of } r)^2}{(\text { unit of } m)^2}\)

Hence, the units of G in different systems are as follows:

CGS System: dyn · cm² · g^-2

SI: N · m² · kg^-2

Dimension And Ahemative Units: From equation (1), the dimension of G = \(\frac{\text { dimension of } F \times(\text { dimension of } r)^2}{(\text { dimension of } m)^2}\)

= \(\frac{M L T^{-2} \times L^2}{\mathrm{M}^2}=\mathrm{M}^{-1} \mathrm{~L}^3 \mathrm{~T}^{-2}\)
Following this analysis, we can write the alternative units of G in different systems.

CGS System: cm³ ·  g^-1 · s^-2

SI: m³ · kg^-1 · s^-2

The units stated earlier and those mentioned above are obviously identical.

Magnitude: The magnitude of the universal gravitational constant obtained from the results of different experiments is:

G = 6.67 x 10^-8 dyn · cm² · g^-2 = 6.67 x 10^-8 CGS unit

= 6.67 x 10^-11 N · m² · kg^-2 =6.67 x 10^-11 in SI

As per the definition of G, when two objects of mass 1 kg each are kept lm away from each other, they attract each other with a force of 6.67 x 10^-11 N.

Clearly, this is a force of very low magnitude. Hence, we cannot feel the gravitational force acting between objects used in our daily lives.

But, on the other hand, the force of attraction of the earth is considerable as the mass of the earth is quite immense.

Derivation Of Newton’s Law Of Gravitation From Kepler’s Law: Let a planet of mass m move around the sun in a circular orbit of radius r. Let M be the mass of the sun, and F is the centripetal force exerted by the sun on the planet.

We know that F = mrω²; but ω = \(\frac{2 \pi}{T}\) [where cω = angular velocity and T is the period of revolution of the planet around the sun]

Then, \(F=m r\left(\frac{2 \pi}{T}\right)^2=\frac{4 \pi^2 m r}{T^2}\)

According to Kepler’s third law, T² = Kr³, where K is constant for all planets.

∴ F = \(\frac{4 \pi^2 m r}{K r^3}=\frac{4 \pi^2 m}{K r^2}\)

Hence, F ∝ \(\frac{m}{r^2}\)

Therefore, the force F, exerted by the sun on the planet is directly proportional to the mass of the planet and inversely proportional to the square of the distance of the planet from the sun.

Since the force is mutual, so, the planet will also exert the same force (in magnitude) on the sun in the opposite direction. Thus, the force exerted by the planet on the sun will also be directly proportional to the mass M of the sun.

Therefore, F ∝ \(\frac{m M}{r^2}\)

This is Newton’s law of gravitation.

Universality Of The Law Of Gravitation: As per Newton’s law of gravitation, the magnitude of the force of attraction between two bodies depends on their masses and the distance between them. This force of attraction does not depend on

1. The state of the objects (solid, liquid, gas),
2. Their chemical composition,
3. Temperature,

The intermediate medium, or any other factor. The law of gravitation is equally applicable to earthly objects separated by small distances and celestial bodies in space, widely separated from one another.

It has been possible to explain the motion of planets around the sun satisfactorily using this law. For these reasons, the law of gravitation is taken as a universal law and the gravitational constant is called the universal gravitational constant.

But as per the theory of relativity propounded by Einstein,

1. The mass of a body depends on its velocity,
2. The distance measured between two objects depends on the velocity of the observer, i.e., The distance between two objects measured by an observer at rest will not be the same as that measured by an observer in motion,
3. Newton’s law of gravitation is not applicable in the case of interatomic distances (10^-9 m).

Because of the reasons stated above, Newton’s law of gravitation cannot be called a universal law any more. However, the universality of G has not been denied even in Einstein’s theory.

 Newtonian Gravitation And Planetary Motion

Newton’s Laws Of Gravitation Numerical Examples

Example 1. If the distance between the centre of a gold sphere of radius 5 nun and of a lead sphere of radius 11.5 cm is 15cm and the force of gravitational attraction between them is 2.16 x 10^-4 dyn, find the value of G, the universal gravitational constant. Densities of gold and lead are 19.3 g · cm^-3 and 11.3 g · cm^-3 respectively.
Solution:

Mass of gold sphere, \(m_1=\frac{4}{3} \times \pi \times(0.5)^3 \times 19.3=10.1 \mathrm{~g}\)

Mass of lead sphere, \(m_2=\frac{4}{3} \times \pi \times(11.5)^3 \times 11.3=7.2 \times 10^4 \mathrm{~g}\)

Given, \(r=15 \mathrm{~cm}\)

∴ G = \(\frac{F r^2}{m_1 m_2}=\frac{2.16 \times 10^{-4} \times(15)^2}{10.1 \times 7.2 \times 10^4}\)

= \(6.68 \times 10^{-8} \mathrm{CGS} \text { unit }\)

Example 2. Three particles of the same mass are kept at the if vertices of an equilateral triangle. The mass of each particle is m and the length of an arm of the triangle is l. Due to the mutual gravitational force of attraction, the particles revolve along the circumcircle of the triangle. Find the velocity of each particle.
Solution:

ABC is an equilateral triangle. At its vertices, three particles A, B and C, each of mass m, are kept. The point of intersection of the E medians of ABC is O, which is also the centre of the circumcircle of the triangle.

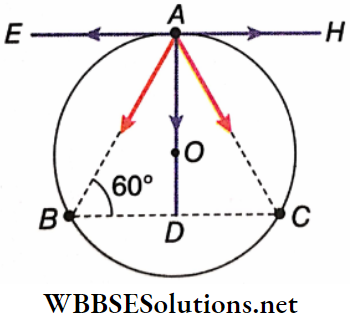
Hence, radius of the circumcircle, r = OA = \(\frac{2}{3}\)AD = \(\frac{2}{3}\)AB sin60° = \(\frac{2}{3} \cdot l \cdot \frac{\sqrt{3}}{2}=\frac{l}{\sqrt{3}}\)

Hence, the centripetal force needed by each particle to revolve along the circumcircle with velocity v is

∴ \(F_1=\frac{m v^2}{r}=\frac{m v^2}{\frac{l}{\sqrt{3}}}=\frac{\sqrt{3} m v^2}{l}\)

Now the gravitational force acting on the particle at point A can be calculated. The force of gravitation on the particle at A due to the particle at B along AB,

F = \(\frac{G \cdot m \cdot m}{(A B)^2}=\frac{G m^2}{R}\)

Component of this force along AE = F cos 60° and that along AD = F sin60°

Again, gravitational attraction on the particle at A due to the particle kept at C is F along AC.

The component of this force along AH = F cos 60° and that along AD = F sin60°.

Clearly, components along AH and AE cancel each other. Hence, the resultant force F₂ on the particle kept at A = sum of the components along AD.

∴ F₂ = Fsin60° + F sin60° = 2 F sin60°

= \(2 F \cdot \frac{\sqrt{3}}{2}=\sqrt{3} F=\frac{\sqrt{3} G m^2}{R}\)

As per the question, this force of attraction due to gravitation supplies the necessary centripetal force.

Hence, \(F_1=F_2 \text { or, } \frac{\sqrt{3} m v^2}{l}=\frac{\sqrt{3} G m^2}{l^2}\)

or, \(\nu^2=\frac{G m}{l}\)

or, \(\nu=\sqrt{\frac{G m}{l}} \)

Example 3. A mass M is broken into two parts of masses m₁ and m₂. How are m₁ and m₂ related so that the force of gravitational attraction between the two parts is maximum?
Solution:

Let m₁ = m, then m₂ = M – m.

The gravitational force between the two parts, when they are placed at a distance r apart, is,

F = \(G \frac{m(M-m)}{r^2}=\frac{G m M}{r^2}-\frac{G m^2}{r^2}\)

Differentiating with respect to m, we have, \(\frac{d F}{d m}=\frac{G M}{r^2}-\frac{2 G m}{r^2}\)

For F to be maximum, \(\frac{d F}{d m}=0\)

∴ \(\frac{G M}{r^2}=\frac{2 G M}{r^2} \text { or, } M=2 m \text { or, } m=\frac{M}{2}\)

∴ \(m_1=m_2=\frac{M}{2}\)

Example 4. Assuming the earth’s orbit around the sun to be circular, show that the area swept by its radius vector in unit time (areal velocity of the earth) is a constant.
Solution:

Suppose the earth moves in a circular orbit of radius r with the sun at the centre (O) of the orbit.

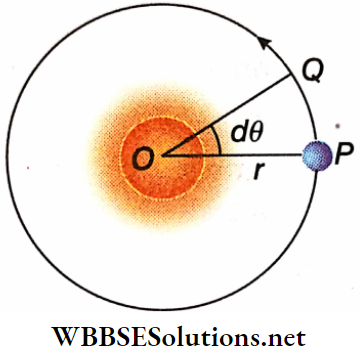
Let the radius vector OP, in an infinitesimal interval of time dt, describe an angle dθ at the centre. Hence, arc PQ = rθ. As the value of PQ is very small, the arc PQ can be taken to be a straight line (chord PQ).

∴ Area swept in time dt = area of triangle OPQ = \(\frac{1}{2} O P \times P Q=\frac{1}{2} r \cdot r d \theta=\frac{1}{2} r^2 d \theta\)

∴ Area swept per unit time = \(\frac{1}{2} r^2 \cdot \frac{d \theta}{d t}=\frac{1}{2} r^2 \omega\) = constant [as the orbit is circular, r and co of the earth should be constants]

∴ The area swept in unit time by the radius vector of the earth is a constant.

Example 5. How fast (in m²/s) is the area swept out by

1. The radius from the sun to Earth?
2. The radius from Earth to the Moon? Given the distance of the sun to earth = 1.496 x 10¹¹ m, the distance of the earth to the moon = 3.845 x 10⁸ m and the period of revolution of the moon = 27\(\frac{1}{3}\) days.

Solution:

1. The rate, at which the area is swept out by the radius from the sun to the earth, \(\frac{d A}{d t}=\frac{\pi r^2}{T}\)

Here, r = 1.496 x 10¹¹ m , T = 365 days = 365 x 24 x 60 x 60 s

∴ \(\frac{d A}{d t}=\frac{\pi \times\left(1.496 \times 10^{11}\right)^2}{365 \times 24 \times 60 \times 60}=2.23 \times 10^{15} \mathrm{~m}^2 \cdot \mathrm{s}^{-1}\)

2. Here, r = 3.845 x 10⁸ m, \(T=27 \frac{1}{3} \text { days }=\frac{82}{3} \times 60 \times 60 \times 24 \mathrm{~s}\)

∴ The rate, at which the area is swept out by radius from the earth to the moon,

⇒ \(\frac{d A}{d t}=\frac{\pi \times\left(3.845 \times 10^8\right)^2}{\frac{82}{3} \times 24 \times 60 \times 60}\)]

= \(1.97 \times 10^{11} \mathrm{~m}^2 \cdot \mathrm{s}^{-1}\)