WBBSE Solutions For Class 9 Maths Solid Geometry Chapter 4 Theorems Of Concurrence

Solid Geometry Chapter 4 Theorems Of Concurrence

Chapter 4 Theorems Of Concurrence Concurrent straight lines and point of concurrence:

Concurrent straight lines :

1. A significant property of two straight lines is
   1. they are either parallel
   2. will meet at a point in infinity.
2. As per this property of straight lines, we can say that if three or more three straight lines are not parallel, then they will meet at one point or more than one point.
3. When the straight lines intersect at one point, then we call the straight lines concurrent straight lines.

Definition:

1. If three or more three straight lines meet or intersect at a point, then they are called concurrent straight lines.
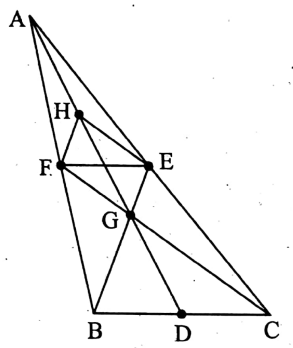
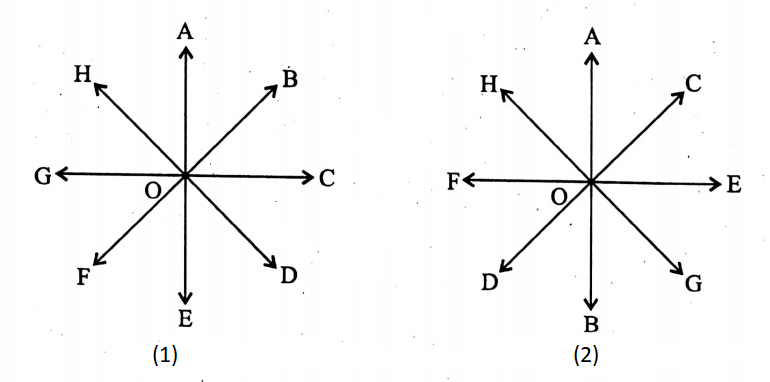
2. Such as, in the following(1), the line segments OA, OB, OC, OD, OE, OF, OG, and OH have met at point O. T
3. Therefore, they are concurrent straight lines.
4. Again, in the following(2), the straight lines AB, CD, EF, and GH intersect each other at point O.
5. Therefore, they are also concurrent straight lines.

Read and Learn More WBBSE Solutions For Class 9 Maths

 

 

Point of concurrence:

Definition:

1. The point at which the unparallel straight lines intersect or meet is called the point of concurrence.
2. In the above 1 and 2, O is the point of concurrence.

For Example:

 

 

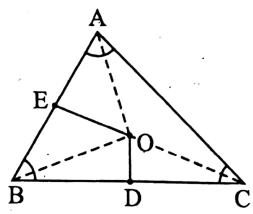
Chapter 4 Theorems Of Concurrence Circum-Circle Circum Centre And Cerium Radius Of A Triangle

 

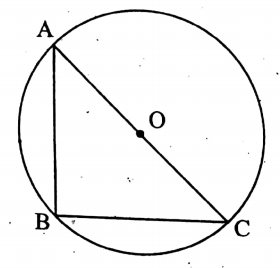
Circum-circle of a triangle :

Definition: The circle through the three vertices of a triangle, is called the circum-circle of the triangle.

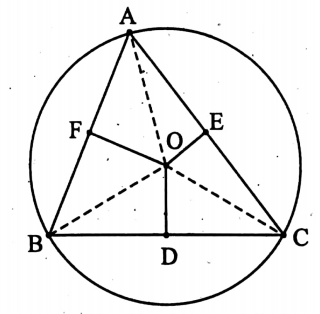
In the following, the circle having O as the centre and passing through the vertices A, B and C is the circum-circle of the triangle Δ ABC.

 

 

Circum-centre of a triangle:

Definition: The centre of the circum-circle of a triangle is called the circum-centre of the triangle.

In the above figure, O is the circum-centre of the Δ ABC. 

Again, O is the curcumin centre of Δ ABC,

∴ and the distances of all the points on the circum-circle, from O are equal.

∴ OA = OB = OC

i.e., Δ BOC, Δ COA and Δ AOB are all isosceles triangles. 

We know that the perpendicular drawn from any vertex of an isosceles triangle to its base bisects the base.

in the above, the perpendiculars OD, OE and OF from O to the sides BC, CA and AB respectively of the Δ ABC have bisected the corresponding bases and these perpendicular bisectors intersect each other at a point O.

Therefore, we can say that the perpendicular bisectors of the sides of a triangle are concurrent and the point at which the perpendicular bisectors intersect each other, is the circum-centre of the triangle.

Thus, we take the point at which any two perpendicular bisectors meet one another as the circum- centre of the triangle.

If the triangle be

1. an acute-angled triangle, then the circum-centre of the triangle lies inside the triangle.

 

 

2. a right-angled triangle, then the circum-centre of the triangle lie on the hypotenuse of the triangle and it bisects the hypotenuse, i.e., the mid-point of the hypotenuse of a right-angled triangle is its circum-centre and circum-radius = \(\frac{1}{2}\) x length of the hypotenuse.

 

 

3. an obtuse-angled triangle, then the circum-centre of its circum- circle lies on the outside of the triangle.

 

 

Circum-radius of a triangle :

Definition:

The radius of the circumcircle of a triangle is called the circum-radius of the triangle.

i.e. the distance of any vertex of a triangle from the point at which the perpendicular bisectors of the sides of a triangle meet each other is called the circum-radius of the triangle.

In the above figure, OA or OB or OC is called the circum-radius of the ΔABC.

We have learned from the above that the three perpendicular bisectors of the sides of a triangle are concurrent.

We shall now logically prove this theorem.

 

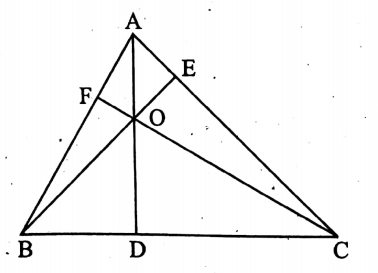
Theorem 1. The perpendicular bisectors of the sides of a triangle are concurrent. 

Given: Let D, E and F are the mid-points of the sides AB, BC and CA of theΔABC. Let us construct two perpendiculars OD and OE to AB and BC respectively. Let OD and OE intersect each other at O. 

To prove The perpendiculars drawn at D, E and F of AB, BC and CA are concurrent, i.e., if we can prove that OF is perpendicular to AC, then the theorem will be proved sufficiently.

Construction: Let us join O, A; O, B and O, C.

Proof: InΔ AOD andΔ BOD, AD = BD

(∵ D is the mid-point of AB).

∠ADO = BDO (∵ OD ⊥ AB) and OD are common to both.

∴ΔAOD = Δ BOD (by the condition of S-A-S congruence)

∴  OA=OB………………..(1) (∵ they are similar sides of congruent triangles)

 Similarly it can be proved that OB = OC……………..(2)

Then, from (1) and (2) we get, OA = OB = OC…………..(3)

Now, in ΔAOF and A COF, AF = CF (∵ F is the mid-point of AC ] 

OA = OC [ by (3)] and OF is common to both.

∴ ΔAOF = ΔCOF (by the condition of S-S-S congruence)

∠AFO = ∠ CFO (∵ these are similar angles of congruent triangles)

i.e., the adjacent angles formed by OF by standing on the side AC.

∴ OF ⊥ AC 

or, OF is perpendicular to AC.

Hence the theorem. (Proved)

 

 

Orthocentre, Foot of the perpendicular and Pedal triangle:

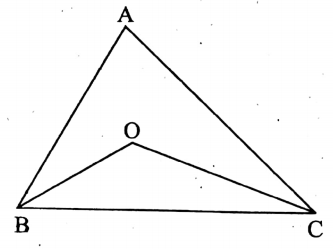
Orthocentre of a triangle :

Definition: The point of intersections of the perpendiculars drawn from the vertices of a triangle to the corresponding opposite sides, is called the orthocentre of the triangle.

In the following figures, O is the orthocentre of the ΔABC.

The orthocentre of a triangle may lie inside, on or outside of the triangle. 

If the triangle be- 

1. An acute-angled triangle, then the orthocentre will lie inside the triangle.
2. A right-angled triangle, then the orthocentre will lie on the rectangular vertex of the triangle. 
3. An obtuse-angled triangle, then the orthocentre will lie outside the triangle.

The following are shown:

 

 

 

 

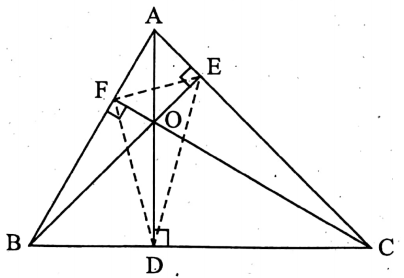
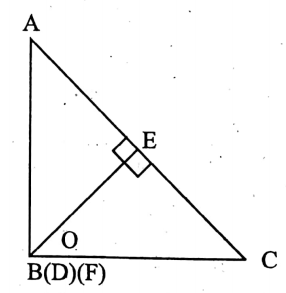
The foot of the perpendicular :

Definition:

1. The point at which the perpendicular is drawn from the vertex to the opposite sides intersects the sides is called the foot of the perpendicular.
2. In the case of an acute-angled triangle, the number of feet of perpendiculars is three.
3. In the figure, the feet of the perpendiculars are D, E and F.
4. In the case of a right-angled triangle, the number is two. In the figure, the feet of the perpendiculars are B (D) (F) and E.
5. Here, D and F coincide with B.
6. In the case of an obtuse-angled triangle, the number is three.
7. In the above, the three feet of perpendiculars are D, E and F.

 

Pedal triangle :

Definition:

1. The triangle formed by joining the feet of the perpendiculars, drawn from the vertices of the triangle to the corresponding sides of the triangle is called the pedal triangle of the original triangle.
2. In the above (In the case of acute-angled and obtuse-angled triangles), Δ DEF is the pedal triangle of ΔABC.
3. We have seen in the above discussion that the perpendiculars drawn from the vertices of the triangle to its opposite sides are concurrent.
4. We shall now prove this theorem logically.

 

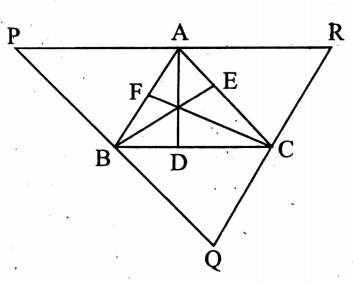
Theorem 2. The perpendiculars drawn from the vertices of any triangle to its corresponding opposite sides are concurrent.

Given: Let AD, BE and CF are the perpendiculars drawn from the vertices A, B and C of the ΔABC to its corresponding opposite sides BC, CA and AB respectively.

To prove: AD, BE and CF are concurrent.

Construction: Let us draw straight lines through A, B and C parallel to the sides BC, CA and AB, which intersect each other at P, Q and R.

∴ a triangle PQR is produced.

Proof: According to the construction, PBCA, ABCR and ABQC are each a parallelogram.

Then from PBCA and ABCR, we get AP=BC and AR = BC,

PBCA and ABCR we get AP = BC and AR = BC,

∴ AP = AR.

A is the mid-point of PR.

Similarly, it can be proved that B is the mid-point of PQ and C

is the mid-point of QR.

Again, PR || BC (by construction) and AD ⊥ BC, AD ⊥ PR.

Similarly, it can be proved that BE ⊥ PQ and CF ⊥ QR.

∴ AD, BE and CF are the perpendicular bisectors of the sides PR, PQ and QR of the ΔPQR respectively. 

We know that the perpendicular bisectors of any triangle are concurrent.

∴ AD, BE and CF are concurrent.

the perpendiculars drawn from the vertices of any triangle to its corresponding opposite sides are concurrent. (Proved)

 

 

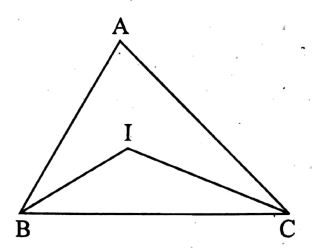
Chapter 4 Theorems Of Concurrence Incircle Incentre and Inradius

 

Incircle :

Definition:

The circle which completely lies inside any triangle and its circumference just touches all three sides of the triangle are called the incircle of the triangle.

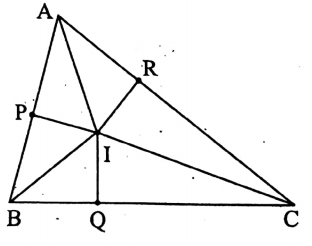
The incentre of the incircle of any triangle is usually denoted by I.

In the given figure beside, the circle with the centre I 

1. lie completely inside the ΔABC 

2. its circumference has just touched the sides BC, CA and AB at points D, E and F.

∴ the circle DEFD with the centre at I is an incircle of the Δ ABC.

 

Incentre :

Definition: The centre of the incircle of any triangle is called the incentre of the triangle.

In the above figure, I am the incentre of the ΔABC.

Now, the circle have touched BC at D, 

∴ ID ⊥ BC.

Similarly, IE ⊥ CA and IF ⊥ AB.

Since the distances of any point on the circumference of a circle from its centre are all equal, we have, ID = IE = IF…………….(1)

Then, in the triangles ΔAEI and ΔAFI, IE = IF [ by (1)]

< AEI = ∠AFI [each is a right angle] and AI is common to both.

ΔAEI ≅ ΔAFI. [ by the condition of R-H-S congruence ]

∴ < IAE ≅ < IAF [they are the similar angles of congruent triangles ]

∴ AI is the bisector of ∠A.

Similarly, it can be proved that BI and CI are the bisectors of B and C respectively.

Therefore, the incentre of the incircle of any ΔABC lie on the bisectors of its angles and they are

concurrent.

Hence, the point at which the bisectors of the angles of a triangle is called the incentre of the triangle, The incentres of any type of triangle lie inside it. 

Since the incentre of any triangle lie on the bisectors of its angles, 

∴ to find the incentre of any triangle we take the point as the incentre at which any two bisectors of its angles intersect and to draw the incircle we take the perpendicular distance of any of its sides from this incentre as its radius.

Inradius:

Definition:

The radius of the incircle of any triangle is called its inradius.

i.e., the perpendicular distance of any of the three sides of a triangle from the point at which the bisectors of its angles intersect is called the incentre of the triangle.

In the above, ID, IE and IF are all the inradii of ΔABC.

Clearly, ID = IE = IF and ID ⊥ BC, IE ⊥ CA and IF ⊥ AB.

In the above discussion, we have seen that the three bisectors of the angles of any triangle are concurrent.

We shall now prove this theorem logically.

 

Theorem 3. The three bisectors of the three angles of any triangle are concurrent.

Given: Let ABC be a triangle. Its bisectors of B and C intersect at I. 

Let us join A, I.

To prove The bisectors of A, B and C are concurrent, i.e., to prove the theorem it is sufficient to prove that AI is the bisector of <BAC.

Construction: Let us draw IP ⊥ AB, IQ ⊥ BC and IR ⊥ CA.

Proof: In A BIQ and A BIP, IBQ = < IBP

[∵ BI is the bisector of B ]

< IQB = IPB [each is right angle] and BI is common to both.

∴ ΔBIQ ≅ Δ BIP [ by the condition A-A-S congruence ]

IQ = IP they are the similar sides of two congruent triangles ]…….. (1)

Similarly, it can be proved that IQ = IR………… (2) and IP = IR . . . . . (3)

Now, in Δ AIP and ΔAIR,

Al is common to both and IP = IR [ by (3)]

∠IPA = ∠IRA [each is right-angled ]

∴ ΔAIP = ΔAIR [ by the condition R-H-S of congruence ] 

∠IAP = ∠IAR [ they are angles of two congruent]

∴ AI is the bisector of the angle ∠A.

∴ Hence the theorem. (Proved)

 

 

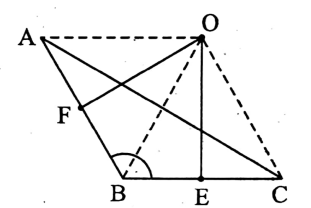
Ex-Circle, Ex-centre and Ex-radius of a triangle:

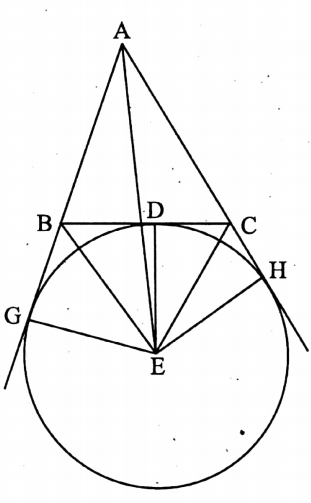
1. Ex-Circle:

Taking the point of intersection of the bisectors of any two exterior angles of any triangle and the bisector of the third interior angle, as a centre and the perpendicular distance of any of its sides from that point as the radius we can draw a circle, which is called the ex-circle of the triangle.

In the given beside, the circle DHGD with centre E is an excircle of the ΔABC.

2. Ex-Centre:

The centre of the ex-circle of any triangle is called its ex-centre.

In the given beside, E is the ex-centre of the ΔABC.

 

 

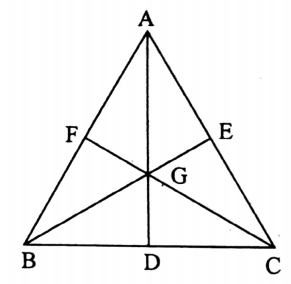
Chapter 4 Theorems Of Concurrence Centroid Of A Triangle

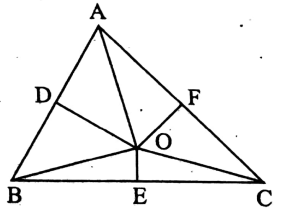
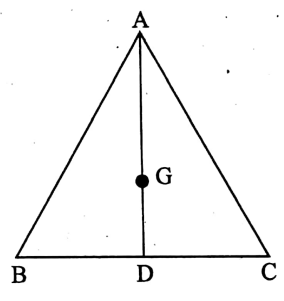
Centroid Of A Triangle:-

Definition:

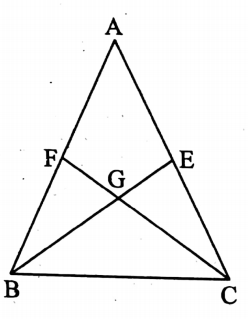
1. The point at which the three medians of any triangle intersect or meet with each other is called the centroid of the triangle.
2. In the figure given beside, the three medians AD, BE and CF of the triangle ABC intersect each other at point G. 
3. G is the centroid of theΔABC.
4. From the definition above, we see that the three medians intersect at a point, i.e., are concurrent. 
5. We shall prove this significant theorem logically.

 

 

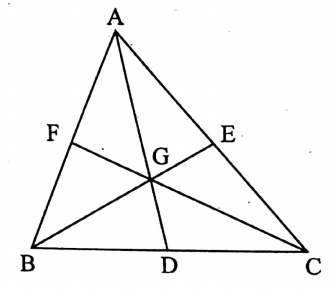
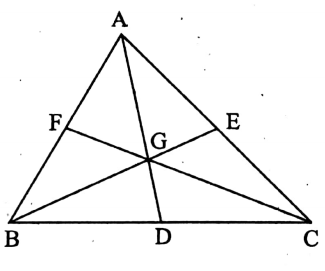
Theorem 4. The three medians of any triangle are concurrent.

Given: Let the medians BE and CF of the ΔABC intersects each other at G.

Let us extend AG by joining A and G, which intersect BC at D.

To prove: The three medians of the Δ ABC are concurrent, i.e., if it can be proved that D is the mid-point of BC, then the theorem will be proved.

Construction: The line segment AD is extended to H in such a way that AG = GH.

Let us join B, H and C, H.

Proof: In ΔABH, the mid-points of AB and AH are F and G respectively,

∴ FG || BH or, GC || BH……… (1)

[∵ the line segment joining two mid-points of any two sides of a triangle is parallel to its third side. ]

Again, in ΔACH, the mid-points of AC and AH are E and G respectively.

∴ EG || CH or, GB || CH . . . . (2) [ for same reason] 

From (1) and (2) we get, in BGCH, GC || BH and BG || HC.

∴ BGCH is a parallelogram.

We know that the diagonals of any parallelogram bisect each other.. the two diagonals BC and GH of the parallelogram BGCH, bisect each other.

∴ D is the mid-point of BC, i.e., AD is the median of the ΔABC.

Hence the theorem. (Proved)

 

 

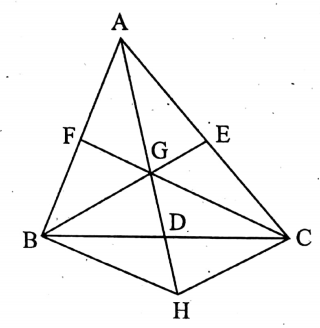
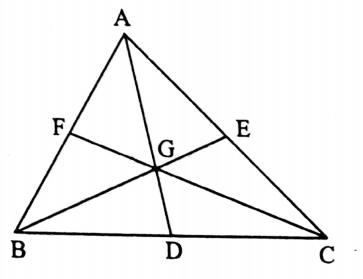
Corollary: Each of the medians of a triangle is divided by the centroid in the ratio 2 : 1 from the side of vertices.

Given: Let G is the centroid of the ΔABC, i.e., the three medians

AD, BE and CF of the ΔABC meet at the point G.

To prove: AG GD = BG: GE = CG: GF = 2: 1.

Construction: Let us extend AG to H such that AG = GH.

Join B, H and C, H.

Proof: In Δ ABH, F is the mid-point of AB and G is the mid-point of AH (by construction).

∴ FG || BH

or, GC || BH………. (1)

Again, inΔACH, E is the mid-point of AC and G is the mid-point

of AH (by construction)

∴ GE || HC or, BG || HC……(2)

Then from (1) and (2) we get, in BGCH, GC || BH and BG || HC.

∴ BGCH is a parallelogram.

∴ the diagonals BC and GH of the quadrilateral BGCH bisect each other, i.e., GD = HD.

GD = \(\frac{1}{2}\) GH

or, GD = \(\frac{1}{2}\)AG [by construction ]

or, \(\frac{\mathrm{AG}}{\mathrm{GD}}=\frac{2}{1}\)

or, AG: GD = 2:1

Similarly, it can be proved that BG: GE= 2:1 and CG: GF = 2: 1.

Hence the theorem. (Proved)

 

 

Chapter 4 Theorems Of Concurrence Select The Correct Answer (MCQ)

 

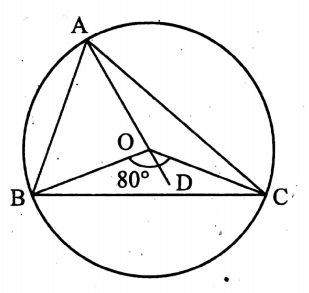
Question 1. The circumcentre of the triangle ABC is O; If ∠ BOC = 80°, then ∠BAC =

1. 40°
2. 160°
3. 130°
4. 110°

Solution: 

Given 

The circumcentre of the triangle ABC is O; If ∠ BOC = 80°.

Let us join A, O. AO is produced to D.

Now, in ΔAOB, ∠BOD is an external angle.

∴ ∠BOD = internally opposite (∠OAB+ ∠OBA)

= ∠OAB + ∠OAB [∵ OA = OB, ∴ ∠OBA = ∠OAB ]

= 2 ∠OAB …….…. (1)

Similarly, in ΔAOC, ∠ COD is an external angle.

∴ < COD= internally opposite (∠OAC + ∠ OCA)

=∠OAC+∠OAC [ OA = OC, ∴ ∠OCA = ∠OAC]

=2 ∠OAC………….(2)

Now, adding (1) and (2) we get,

∠ BOD + ∠COD = 2 OAB + 2 ∠OAC

or, BOC = 2 (< OAB + ∠OAC) or,

∠BOC = 2 ∠BAC 

∴ 2 BAC = < BOC

or, \(\angle \mathrm{BAC}=\frac{\angle \mathrm{BOC}}{2}=\frac{80^{\circ}}{2}=40^{\circ}\)    [∵ ∠ BOC= 80° ]

∠ BOC = 40°

 

 

Question 2. Orthocentre of the triangle ABC is O; If ∠BAC 

1. 80°
2. 140°
3. 110°
4. 40°

Solution:

Given

Orthocentre of the triangle ABC is O.

O is the orthocentre of the ΔABC

∴ AD ⊥ BC, BE ⊥ CA and CF ⊥ AB.

∵ BE ⊥ CA,

∴ ∠AEO = 90°

Again, ∵ CF ⊥ AB, ∠AFO = 90°

Now, ∠EAF + ∠AEO+∠EOF+∠AFO = 360° [∵ sum of the angles of a quadrilateral is 360° ] 

or, 40° 90° + EOF + 90° 360°

[∵ \(\angle \mathrm{EAF}=\angle \mathrm{BAC}=40^{\circ}, \angle \mathrm{AEO}=90^{\circ}, \angle \mathrm{AFO}=90^{\circ}\) ]

or, \(\angle \mathrm{EOF}=360^{\circ}-220^{\circ} \text { or, } \angle \mathrm{EOF}=140^{\circ}\)

or, ∠BOC = 140°   [∵ EOF= opposite BOC]. 

∴ ∠BOC = 140°

[ Short-cut: ∠ BOC = 180° – ∠ BAC = 180°-  40° = 140° ]

 

 

Question 3. Incentre of the triangle ABC is O; If ∠BAC = 40″, then ∠BOC =

1. 80°
2. 110°
3. 140°
4. 40°

Solution:

Given 

Incentre of the triangle ABC is O; If ∠BAC = 40°.

Let the bisectors of B and C of ΔABC intersect each other at O; O is the incentre of the ΔABC.

Now, In ΔABC,

∠A + ∠B + ∠C = 180° [ sum of the three angles of a triangle is 180° ]

or, \(\frac{1}{2}^{\circ} \angle \mathrm{A}+\frac{1}{2} \angle \mathrm{B}+\frac{1}{2} \angle \mathrm{C}=90^{\circ}\)

or, \(\frac{1}{2} \angle \mathrm{BAC}+\angle \mathrm{OBC}+\angle \mathrm{OCB}=90^{\circ}\)

or, \(\frac{1}{2} \times 40^{\circ}+\angle \mathrm{OBC}+\angle \mathrm{OCB}=90^{\circ}\) [∵ <BAC = 40°]

or, ∠OBC+∠OCB = 70°……………(1)

Again, in ΔBOC, ∠BOC+ ∠OBC+∠OCB = 180°

or, ∠ BOC + 70° 180° [ by (1)] 

or, ∠BOC= 110°

∴ ∠ BOC = 110°.

 

 

Question 4. The centroid of the ΔABC is G; If the area of the ΔGBC is 12 Sq. cm, then the area of the ΔABC is

1. 24 Sq. cm
2. 6 Sq. cm
3. 36 Sq. cm
4. None of these

Solution.

Given 

The centroid of the ΔABC is G; If the area of the ΔGBC is 12 Sq. cm.

Let the medians AD, BE and CF of the ΔABC have intersected at point G.

∴ G is the centroid of the Δ ABC.

We know that

\(\Delta \mathrm{GBC}=\frac{1}{3} \Delta \mathrm{ABC} \quad \text { or, } 12 \text { Sq. } \mathrm{cm}=\frac{1}{3} \Delta \mathrm{ABC}\)

or, Δ ABC = 36 Sq. cm. 

∴ ΔABC = 36 Sq. cm

 

 

Question 5. If the circum-radius of the right-angled triangle ABC be 5 cm, then the length of its hypotenuse will be-

1. 2.5 cm
2. 10 cm
3. 5 cm
4. None of these

Solution:

Given 

The circum-radius of the right-angled triangle ABC be 5 cm.

We know that the circum-radius of a right-angled triangle

= \(\frac{1}{2}\)length of hypotenuse.

∴ Length of hypotenuse = 2 x length of the circum-radius 

= 2 x 5 cm = 10 cm.

∴ the required length = 10 cm.

 

 

Chapter 4 Theorems Of Concurrence Short Answer Type Questions

 

Question 1. The sides of a triangle are 6 cm, 8 cm and 10 cm, find the position of the circum-centre of the triangle.

Solution:

Given

The sides of a triangle are 6 cm, 8 cm and 10 cm.

6²+ 8² + 10², 

∴ the given triangle is a right-angled triangle, the length of whose hypotenuse is 10 cm.

We know that the circum-centre of any right-angled triangle lie on the mid-point of its hypotenuse. 

∴ The circum-centre of the given right-angled triangle lie on the mid-point of its hypotenuse of length 10 cm.

 

Question 2. AD is the median of the equilateral ΔABC and G is its centroid. The sides of the triangle are 3√3 cm, then find the length of AG.

Solution:

Given 

AD is the median of the equilateral ΔABC and G is its centroid.

The sides of the triangle are 3√3 cm.

In ΔABC, AB = BC = CA = 3√3 cm (given)

AD is a median of the Δ ABC.

∴ BD = CD

Now, in ΔABD and ΔACD, AB = AC, BD = CD and AD are common to both.

∴ ΔABD ≅ ΔACD [by the condition of S-S-S congruence],

∴ ∠ADB = ∠ADC [ ∵ similar angles ]

But these angles are two adjacent angles formed by standing AD on BC and are equal,

∴ these are right angles,

∴ AD ⊥ BC.

Now, by the Pythagoras theorem, AD² + BD² = AB²

or, \(\mathrm{AD}^2+\left(\frac{1}{2} \mathrm{BC}\right)^2=\mathrm{AB}^2 \text { or, } \mathrm{AD}^2+\left(\frac{1}{2} \times 3 \sqrt{3}\right)^2=(3 \sqrt{3})^2\)

or, \(\mathrm{AD}^2=27-\frac{27}{4} \text { or, } \mathrm{AD}=\sqrt{\frac{81}{4}}=\frac{9}{2}\)

Again,  \(\mathrm{AG}=\frac{2}{3} \mathrm{AD} \quad \text { or, } \mathrm{AG}=\frac{2}{3} \times \frac{9}{2} \mathrm{~cm}=3 \mathrm{~cm}\)

 

 

Question 3. DEF is the Pedal triangle of the equilateral triangle ABC; Find ∠FDA.

Solution:

Given 

DEF is the Pedal triangle of the equilateral triangle ABC.

ΔDEF, is the pedal triangle of the ΔABC, ΔDEF is also an equilateral triangle.

∴ FDE = 60°……….. (1)

Again, ∵ FE || BC [∵ F and E are the mid-points of AB and AC respectively ] 

and AD ⊥ BC, 

∴ AP I FE where P is the point of intersection of AD and FE.

FPD = 2 EPD [ ∵ each is 90°]………..(2)

Now, in A FPD and A EPD, ∠FPD = < EPD [by (2)]

FD = ED [ ΔDEF is equilateral] and PD is common to both.]

∴ AFPD AEPD [ by the condition of RHS of congruence]

∴ FDP = EDP [ they are similar angles of congruent triangles] 

∴ <FDP = ∠FDA= \(\frac{1}{2}\) ∠FDE 

or, ∠FDA= \(\frac{1}{2}\) x 60°

= 30°. [by (1)]

 

∠FDA = 30°. [by (1)]

 

 

Question 4. In the isosceles triangle ABC, ∠ABC = ∠ACB and median AD = \(\frac{1}{2}\) BC. If AB = √2 then find the length of the circum-radius of the Δ ABC.

Solution:

Given

In the isosceles triangle ABC, ∠ABC = ∠ACB and median AD = \(\frac{1}{2}\) BC.

AB = √2.

∵ in Δ ABC, ∠ABC = < ACB, 

∴ AC = AB = √2 cm………(1)

Again AD is a median of ΔABC and AC = AB, 

∴ AD ⊥ BC

Now, AD=BC (Given)

or, AD = BD [ ∵  D is the mid-point of BC]

∴ from the right-angled triangle ABD, we get, AD² + BD² = AB²

BD² = BD+BD-(√2)  [by (1) and ∵  AD = BD ] and [by (1) and (2)

or, 2BD² = 2 or, BD = 1 cm

∴ BD = 1cm ……………..(1)

∴ BC = 2 x 1 cm = 2 cm

Again AB²+AC² = AB² – AB² – 2AB² = 2 x (√2)² Sq.cm

= 4 Sq. cm

= 23 Sq. cm – BC

i.e., AB² + AC² = BC²,

∴ ΔABC is an isosceles right-angled triangle of which ∠A is right-angle. 

∴ the circum-centre of the ΔABC lies on the mid-point D of its hypotenuse BC. 

∴ The required circum-radius = BD = 1 cm [ by (2)]

 

The length of the circum-radius of the Δ ABC = 1 cm 

 

Chapter 4 Theorems Of Concurrence Long Answer Type Questions

 

Question 1. Prove that if any two medians of a triangle be equal, then it is an isosceles triangle.

Given: Let the medians BE and CF of the ΔABC are equal, i.e., BE = CF.

To prove: ΔABC is an isosceles triangle.

Proof: Let BE and CF intersects each other at G. We know that the three medians of a triangle intersect at a tri-sector point within it, 

∴ EG = \(\frac{1}{2}\) BE and FG = \(\frac{1}{2}\) CF

But, BE CF, 

∴ EG FG……. (1) and BG = CG…….(2)

Now, in Δ BGF and ΔCGE, FG = EG [by (1)], 

BG = CG [by (2)] 

and ∠BGF = ∠CGE [∵ these are opposite angles]

∴ ΔBGF ≅ ΔCGE [ by the condition of S-A-S congruence]

∴ BF = CE [∵ these are similar sides of congruent triangles]

or, \(\frac{1}{2}\) AB = \(\frac{1}{2}\)AC [∵ F and E are the mid-points of AB and AC respectively]

or, AB = AC

∴ ΔABC is an isosceles triangle. (Proved)

 

 

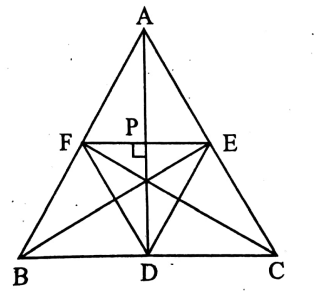
Question 2. The three medians AD, BE and CF of the ΔABC intersect each other at G. Prove

1. ΔGBC = ΔGCA = ΔGAB = \(\frac{1}{3}\) ΔΑΒΟ

Solution:

Given

The three medians AD, BE and CF of the ΔABC intersect each other at G.

AD is a median of ΔABC,

∴ ΔABD = ΔACD …..(1)

Similarly, ΔGBD = ΔGCD……….(2)

Subtracting (2) from (1) we get, ΔABD – ΔGBD = ΔACD – ΔGCD

or, ΔGAB = ΔGCA ……………(3)

Similarly, it can be proved that ΔGAB = ΔGBC ………………………(4)

From (3) and (4) we get, ΔGBC = ΔGCA = ΔGAB ……………………… .(5)

Again, ΔABC = ΔGBC + ΔGCA + ΔGAB

= ΔGBC + ΔGBC + ΔGBC [ by (5)] = 3 ΔGBC.

∴ 3 ΔGBC = ΔABC

or, ΔGBC = ΔABC …………………….(6)

∴ From (5) and (6), we get, B D C

ΔGBC = ΔGCA = ΔGAB = \(\frac{1}{3}\) ΔABC. (Proved)

 

 

2. ΔGBD = ΔGCD = ΔGCE = ΔGAE = ΔGAF = ΔGBF = \(\frac{1}{6}\) ΔABC

Solution:

GD is a median of ΔGBC,

∴ Δ GBD = Δ GCD …………………………….(1)

GE is a median of Δ GCA,

∴ Δ GCE = ΔGAE …………………………………..  (2)

GF is a median of Δ GAB,

‍∴ ΔGAF = ΔGBF ……………………………………(3)

Also, from (1) we have,ΔGBC = ΔGCA = ΔGAB = \(\frac{1}{3}\) ΔABC.

or, \(\frac{1}{2}\) ΔGBC = \(\frac{1}{2}\) ΔGCA = \(\frac{1}{2}\) ΔGAB = \(\frac{1}{2}\) x \(\frac{1}{3}\) ΔABC

or, ΔGBD = Δ GCE = ΔGAF = \(\frac{1}{6}\) ΔABC ……………. .(4)

∴ From (1), (2), (3) and (4) we get,

ΔGBD = ΔGCD = ΔGCE = ΔGAE = ΔGAF = ΔGBF = \(\frac{1}{6}\) ΔABC. (Proved)

 

Question 2. The bisectors of the interior angles B and C of the ΔABC, meet at I. Prove that 1 <BIC=90º+ \(\frac{1}{2}\)<BAC.

Given: The bisectors BI and CI of the interior angles of B and C respectively of the Δ ABC, meet at I.

To prove: \(\angle \mathrm{BIC}=90^{\circ}+\frac{1}{2} \angle \mathrm{BAC} .\)

Proof: In \(\Delta \mathrm{ABC}, \angle \mathrm{A}+\angle \mathrm{B}+\angle \mathrm{C}=180^{\circ} \quad \text { or, } \frac{1}{2} \angle \mathrm{A}+\frac{1}{2} \angle \mathrm{B}+\frac{1}{2} \angle \mathrm{C}=90^{\circ}\)

or, \(\frac{1}{2} \angle \mathrm{BAC}+\angle \mathrm{IBC}+\angle \mathrm{ICB}=90^{\circ}\)

or, \(\angle \mathrm{IBC}+\angle \mathrm{ICB}=90^{\circ}-\frac{1}{2} \angle \mathrm{BAC}\)………..(1)

Again, in \(\angle \mathrm{BIC}=180^{\circ}-90^{\circ}+\frac{1}{2} \angle \mathrm{BAC} \quad \text { or, } \angle \mathrm{BIC}=90^{\circ}+\frac{1}{2} \angle \mathrm{BAC}\)

∴ \(\angle \mathrm{BIC}=90^{\circ}+\frac{1}{2} \angle \mathrm{BAC} .\)    (proved)

 

 

Question 4. Prove that if the three medians of the triangle be equal, then it is an equilateral

Given: Let the three medians AD, BE and CF of the ΔABC are equal, i.e., let AD = BE = CF.

To prove ΔABC is an equilateral triangle, i.e., AB = BC = CA.

Proof: Let AD, BE and CF intersect at G. G is the centroid of the ΔABC.

GF = \(\frac{1}{3}\) CF and GD = \(\frac{1}{3}\) AD

But, CF = AD,

∴ GF = GD…….... (1)

Again, AG = \(\frac{2}{3}\) AD and CG = \(\frac{2}{3}\) CF

But, AD CF, 

∴ AG = CG …………(2)

Now, in ΔGAF and ΔGCD, GF = GD [ by (1)], 

AG = CG [by (2)] 

and, ∠FGA = ∠DGC [∵ opposite angles]

AGAF ≅ AGCD [ by the S-A-S condition of congruence]

AF = CD [∵ they are similar sides of two congruent triangles ]

or, AB \(\frac{1}{2}\) = \(\frac{1}{2}\) BC [∵ F and D are the mid-points of AB and BC respectively.]

AB = BC……. (3)

Similarly, proving ΔGBD = ΔGAE, it can be proved that BC = CA……….(4)

from (3) and (4) we get, AB = BC = CA.

∴ ΔABC is an equilateral triangle. (Proved)

 

 

Question 5. AD, BE and CF are the medians of the ΔABC, proving that

1. 4 (AD+ BE + CF) > 3 (AB+ BC + CA)

2. 3 (AB+ BC + CA)> 2 (AD + BE + CF)

Given AD, BE and CF are three medians of the ΔABC.

To prove

1. 4 (AD + BE + CF) > 3 (AB+ BC + CA)

2. 3 (AB+ BC + CA) > 2 (AD + BE + CF)

Proof:

1. Let AD, BE and CF intersect at G.

∴ G is the centroid of the ΔABC.

\(\mathrm{AG}=\frac{2}{3} \mathrm{AD}, \mathrm{BG}=\frac{2}{3} \mathrm{BE} \text { and } \mathrm{CG}=\frac{2}{3} \mathrm{CF}\) ……….(1)

We know that sum of any two sides of a triangle is greater than the third side.

∴ in \(\Delta \mathrm{GAB}, \mathrm{AG}+\mathrm{BG}>\mathrm{AB}, \text { or, } \frac{2}{3} \mathrm{AD}+\frac{2}{3} \mathrm{BE}>\mathrm{AB}[\text { by }(1)]\)

or, 2(AD + BE) > 3 AB………….(2)

In \(\Delta \mathrm{GBC}, \mathrm{BG}+\mathrm{CG}>\mathrm{BC}, \quad \text { or, } \frac{2}{3} \mathrm{BE}+\frac{2}{3} \mathrm{CF}>\mathrm{BC}[\text { by }(1)]\)

or, 2 (BE+CF) > 3 BC………….(3)

In \(\Delta \mathrm{GCA}, \mathrm{CG}+\mathrm{AG}>\mathrm{CA}, \quad \text { or, } \frac{2}{3} \mathrm{CF}+\frac{2}{3} \mathrm{AD}>\mathrm{CA} \quad[\text { by }(1)]\)

or, 2 (CF+ AD) > 3 CA………….(4)

Then adding (2), (3) and (4) we get,

2 (AD + BE) + 2 (BE+ CF) + 2 (CF+ AD) > 3 AB+3 BC + 3 CA

or, 2 (AD + BE + BE + CF + CF + AD) > 3 (AB+ BC + CA)

or, 2 (2AD + 2BE+2CF) > 3 (AB+ BC + CA) 

or, 2. 2 (AD + BE + CF) >3 (AB+ BC+ CA).

or, 4 (AD + BE + CF) > 3 (AB + BC + CA)

∴ 4 (AD+ BE+ CF) > 3 (AB + BC + CA). (Proved)

 

 

2. We know that sum of any two sides of a triangle is greater than the third side.

∴ In ΔABD, AB + BD > AD 

or, \(A B+\frac{1}{2} B C>A D\)     [ ∵ D is the mid-point of BC]

or, 2 AB+ BC > 2 AD…………(1)

In A BCE, BC + CE > BE, 

or, \(\mathrm{BC}+\frac{1}{2} \mathrm{CA}>\mathrm{BE}\)         [∵ E is the mid-point of CA]

or, 2BC+ CA> 2 BE………(2)

In A CAF, CA + AF > CF, 

Or, \(\mathrm{CA}+\frac{1}{2} \mathrm{AB}>\mathrm{CF}\)    [ ∵ F is the mid-point of AB]

or, 2 CA + AB > 2 CF………………(3)

Now, adding, (1), (2) and (3) we get, 2AB+ BC + 2BC + CA + 2CA + AB > 2AD + 2BE + 2CF. 

or, 3AB+ 3BC + 3CA > 2 (AD + BE+ CF) 

or, 3 (AB+ BC + CA) > 2 (AD + BE + CF). 

∴ 3 (AB+ BCCA) > 2 (AD + BE+CF). (Proved)

 

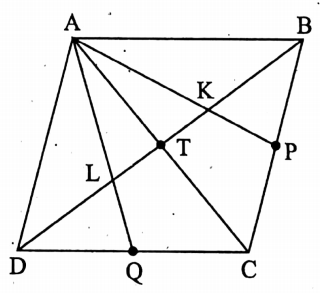
Question 6. In the parallelogram ABCD, P and Q are the mid-points of BC and CD. AP and AQ intersect the diagonal BD at K and L respectively. Prove that BK = KL = LD.

Given: In the parallelogram ABCD, P and Q are the mid-points of BC and CD. AP and AQ intersect the diagonal BD at K and L respectively.

To prove BK = KL = LD.

Construction: Let us draw the diagonal AC, which intersects BD at T.

Proof: Since, the diagonals AC and BD of the parallelogram

ABCD bisect each other.

∴ BT = DT and T is the mid-point of AC.

two medians AP and BT of ΔABC intersect each other at K.

∴ K is the centroid of the ΔABC

∴ \(\mathrm{BK}=\frac{2}{3} \mathrm{BT}=\frac{2}{3} \mathrm{DT}\)……………….(1) [∵ BT DT ]

and KT =\(\frac{1}{3}\) BT………...(2)

Again, two medians of AQ and DT of ΔACD intersect each other at L. 

∴ L is the centroid of the AACD.

∴ \(\mathrm{LD}=\frac{2}{3} \mathrm{DT}=\frac{2}{3} \mathrm{BT}\)……………..(3)  [∵ DT = BT ]

∴ \(\mathrm{LT}=\frac{1}{3} \mathrm{DT}=\frac{1}{3} \mathrm{BT}\)……………..(4)  [∵ DT = BT ]

Now, from (1) and (3) we get, BK = LD………………(5)

Again, (2) + (4) gives \(\mathrm{KT}+\mathrm{LT}=\frac{1}{3} \mathrm{BT}+\frac{1}{3} \mathrm{BT} \text { or, } \mathrm{KL}=\frac{2}{3} \mathrm{BT}\)

or, KL = LD [ by (3)] 

or, LD = KL…………..(6)

Then from (5) and (6) we get, BK = LD = KL.

∴ BK = KL = LD. (Proved)

 

 

 

Question 7. AD, BE and CF are the medians of the ΔABC. If the angle between two other medians is 90°.

Given: AD, BE and CF are the medians of the Δ ABC. \(\frac{2}{3}\) AD = BC 

or, 2AD = 3 BC. 

The two AD=BC other medians are BE and CF.

Let the medians meet at G.

To prove: The angle between BE and CF is 90°, i.e., ∠EGF = 90°.

Construction: Let us cut GH from AG equal to GD.

Let us join E, H; E, F and F, H.

Proof: G is the centroid ofΔ ABC.

\(\mathrm{GD}=\frac{1}{3} \mathrm{AD} \text { or, } \mathrm{GH}=\frac{1}{3} \mathrm{AD}\) …………..(1)    [ by construction GD = GH ]

Given that \(\frac{2}{3} \mathrm{AD}=\mathrm{BC} \text { or, } \frac{1}{3} \mathrm{AD}=\frac{1}{2} \mathrm{BC}\) ………..(2)

Now, F and E are the mid-points of AB and AC.

∴ FE || BC and FE = \(\frac{1}{2}\) BC

or, FE = \(\frac{1}{3}\) AD [by (2)].………….. (3)

 Then from (1) and (3) we get, FE GH,

But FE and GH are the two diagonals of the quadrilateral EGFH, which are equal.

We know that the diagonals of a rectangle or of a square are equal.

∴ EGFH is either a rectangle or a square.

∴ each of its angles is 90°

∴ ∠EGF = 90° Hence the theorem. (Proved)