Ameerun

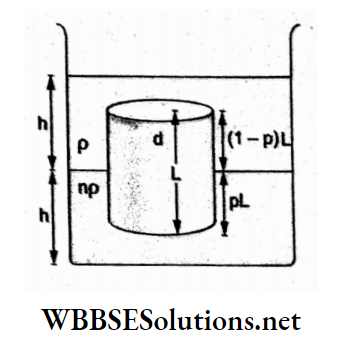
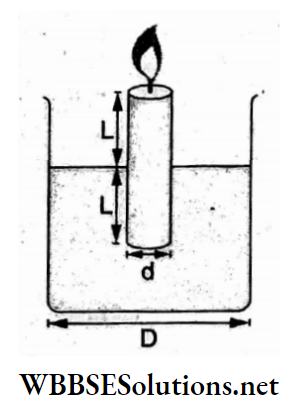
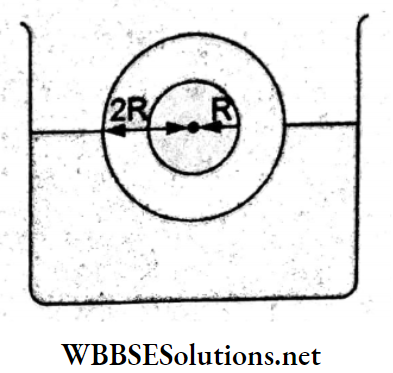
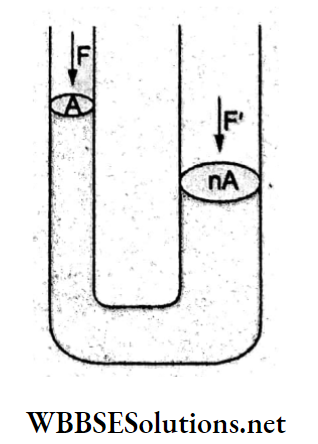
Mechanical Properties of Matter Elasticity Surface Tension and Viscosity

Question 1. Which of the following has no dimensions?

1. Young modulus
2. Stress
3. Poisson ratio
4. Compressibility

Answer: 3. Poisson ratio

Poisson ratio = \(\sigma=\frac{\text { lateral strain }}{\text { longitudinal strain }}\)

As strain is dimensionless, the Poisson ratio also has no dimensions.

Question 2. The dimensional formula for the modulus of rigidity is

1. ML^-2r^-2
2. ML^-3T^-2
3. ML²T^-2
4. ML^-1T^-2

Answer: 4. ML^-1T^-2

Modulus of rigidity = \(\eta=\frac{\text { tangential stress }}{\text { shear strain }}\)

Shear strain is dimensionless, so

⇒ \([\eta]=\frac{[\text { force }]}{[\text { area }]}=\mathrm{ML}^{-1} \mathrm{~T}^{-2}\)

Question 3. Which of the following symbols does not denote a unit of the Young modulus?

1. MPa
2. dyn cm^-2
3. Nm^-1
4. Nm^-2

Answer: 3. Nm^-1

Y is \(\frac{fprce}{area}\) ,so the newton per metre (N m^-1) is not a unit of Y

Question 4. A 10-m-long steel wire of a cross-sectional area 10^-5 m² subjected to an extensional force of 2500 N elongates by 1.0 cm. The Young modulus for steel is

1. 2.5 x 10⁷ N m^-2
2. 2.5 x 10⁹ N m^-2
3. 2.5 x 10¹¹ N m^-2
4. 3.0 x 10¹⁰ N m^-2

Answer: 3. 2.5 x 10¹¹ N m^-2

⇒ \(Y=\frac{\frac{F}{A}}{\frac{\Delta L}{L}}=\frac{\frac{2500 \mathrm{~N}}{10^{-5} \mathrm{~m}^2}}{\frac{1 \times 10^{-2} \mathrm{~m}}{10 \mathrm{~m}}}\)

= \(2.5 \times 10^{11} \mathrm{~N} \mathrm{~m}^{-2}\)

Question 5. The theoretical value of the Poisson ratio lies between

1. -∞ and +∞
2. -1 and +1
3. 0 and +1
4. -1 and +\(\frac{1}{2}\)

Answer: 4. -1 and +\(\frac{1}{2}\)

Theoretically, the value of the Poisson ratio lies between-1 and + \(\frac{1}{2}\)

Question 6. If the longitudinal strain for a wire is 0.03 and its Poisson ratio is 0.5, its lateral strain is

1. 0.003
2. 0.0075
3. 0.015
4. 0.4

Answer: 3. 0.015

Poisson ratio = \(\sigma=\frac{\text { lateral strain }}{\text { longitudinal strain }}\)

∴ 0.5 = \(\frac{\text { lateral strain }}{0.03}\)

lateral strain = 0.015

Question 7. The relation between the Young modulus (Y), bulk modulus (B), and modulus of rigidity (n) is

1. \(\frac{1}{Y}=\frac{1}{B}+\frac{1}{\eta}\)
2. \(\frac{3}{Y}=\frac{1}{\eta}+\frac{1}{3 B}\)
3. \(\frac{1}{Y}=\frac{3}{\eta}+\frac{1}{3 B}\)
4. \(\frac{1}{\eta}=\frac{3}{Y}+\frac{1}{3 B}\)

Answer: 2. \(\frac{3}{Y}=\frac{1}{\eta}+\frac{1}{3 B}\)

The relation connecting Y, n, and B is

⇒ \(\frac{3}{Y}=\frac{1}{\eta}+\frac{1}{3 B}\)

Question 8. A uniform steel wire of a cross-sectional area of 4 mm² is stretched by 0.1 mm by some stretching force. How much will another steel wire of the same length but of a cross-sectional area of 8 mm² get stretched by the same stretching force?

1. 0.5 mm
2. 1.0 mm
3. 0.05 mm
4. 0.08 mm

Answer: 3. 0.05 mm

⇒ \(Y=\frac{F}{A} \cdot \frac{L}{\Delta L}\)

Given that F and L are constant.

∴ Δ₁ . ΔL₁ = Δ₂.ΔL₂

(4mm²)(0.1mm) = (8mm²)ΔL₂

ΔL₂ = 0.05mm.

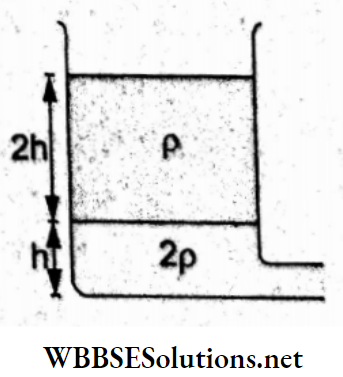
Question 9. The breaking force for a wire of diameter D of a material is F. The breaking force for a wire of the same material of radius D is

1. \(\frac{F}{4}\)
2. F
3. 2F
4. 4F

Answer: 4. 4F

The breaking stress for a given material is constant. Thus, the breaking force (F) is proportional to the area (A).

Here, \(F=k \pi\left(\frac{D}{2}\right)^2\)

and F’ = knD² = 4F.

Question 10. A cube is subjected to a uniform volume compression. If the length of each side of the cube is reduced by 2%, the bulk strain is

1. 0.02
2. 0.03
3. 0.04
4. 0.06

Answer: 4. 0.06

Volume strain = \(\frac{\Delta V}{V}\)

∵ V = L³,

∴ \(\frac{\Delta V}{V}=3\left(\frac{\Delta L}{L}\right)\)

= 3(2%)

= 6%

= 0.06′

Question 11. The energy density in a stretched string is equal to

1. stress x strain
2. \(\frac{stress}{strain}\)
3. \(\frac{1}{2}\) (stress x strain)
4. \(\frac{strain}{stress}\)

Answer: 3. \(\frac{1}{2}\) (stress x strain)

The elastic energy stored is

U = \(\frac{1}{}2\) (stress)(strain)(volume).

∴ energy density = \(\frac{U}{\text { volume }}=\frac{1}{2}\) (stress)(strain).

Question 12. A wire fixed at the upper end stretches by a length l by applying a stretching force F. The work done in this stretching is stress

1. \(\frac{E}{2l}\)
2. Fl
3. 2Fl
4. \(\frac{Fl}{2}\)

Answer: 4. \(\frac{Fl}{2}\)

Work done = \(\frac{1}{2}\)(stretching force)(stretch)

= \(\frac{1}{2}\)Fl.

Question 13. A uniform wire suspended vertically from one of its ends is stretched by a 20-kg load suspended from the lower end. The load stretched the wire by 1 mm. The elastic energy stored in the wire is

1. 0.1 J
2. 0.21 J
3. 10 J
4. 20 J

Answer: 1. 0.1 J

Given that F = 20 kg F

= 200 N

and stretch = 1 mm

= 1 x 10^-3 m.

∴ energy stored = U = \(\frac{1}{2}\)Fl

= 0.1 J.

Question 14. If S is the stress and Y is the Young modulus of the material of a wire, the energy stored per unit volume in the wire is

1. \(\frac{2Y}{S}\)
2. \(\frac{S^2}{2 Y}\)
3. \(\frac{S}{2Y}\)
4. 2S²Y

Answer: 2. \(\frac{S^2}{2 Y}\)

Energy density = \(\frac{1}{2}\) (stress)(strain)

= \(\frac{1}{2} \text { (stress) }\left(\frac{\text { stress }}{Y}\right)\)

= \(\frac{S^2}{2 Y}\)

Question 15. A pendulum made of a uniform wire of cross-sectional area A has a time period of T. When an additional mass M is added to its bob, the time period changes to TM. If Y is the Young modulus of the wire then is equal to

1. \(\left[\left(\frac{T_M}{T}\right)^2-1\right] \frac{A}{M g}\)
2. \(\left[\left(\frac{T_M}{T}\right)^2-1\right] \frac{M g}{A}\)
3. \(\left[1-\left(\frac{T_M}{T}\right)^2\right] \frac{A}{M g}\)
4. \(\left[1-\left(\frac{T}{T_M}\right)^2\right] \frac{A}{M g}\)

Answer: 1. \(\left[\left(\frac{T_M}{T}\right)^2-1\right] \frac{A}{M g}\)

⇒ \(T=2 \pi \sqrt{\frac{L}{g}} \text { and } T_M=2 \pi \sqrt{\frac{L+\Delta L}{g}}\)

∴ \(\frac{T_M}{T}=\sqrt{\frac{L+\Delta L}{L}} \Rightarrow\left(\frac{T_M}{T}\right)^2=1+\frac{\Delta L}{L}\)

But \(Y=\frac{F L}{A \cdot \Delta L}=\frac{M g L}{A \cdot \Delta L}\)

∴ \(\frac{1}{Y}=\frac{A \cdot \Delta L}{M g L}=\left[\left(\frac{T_M}{T}\right)^2-1\right] \frac{A}{M g}\)

Question 16. The potential-energy function for the force between two atoms in a diatomic molecule is approximately given by  \(U(x)=\frac{a}{x^{12}}-\frac{b}{x^6}\) where a and b are two constants, and x is the distance between the atoms. If the dissociation energy is \(D=U(x=\infty)-U_{\mathrm{eq}}\) then D is equal to

1. \(\frac{b^2}{6 a}\)
2. \(\frac{b^2}{2 a}\)
3. \(\frac{b^2}{12 a}\)
4. \(\frac{b^2}{4 a}\)

Answer: 4. \(\frac{b^2}{4 a}\)

Potential energy = U = \(\frac{a}{x^{12}}-\frac{b}{x^6}\)

Force = \(F=-\frac{d U}{d x}=\frac{12 a}{x^{13}}-\frac{6 b}{x^7}\)

For equilibrium, F = 0

x⁶ = \(\frac{2a}{b}\)

U at x = ∞ is zero, and U at equilibrium, when x = \(x=\left(\frac{2 a}{b}\right)^{1 / 6}\), will be equal to

⇒ \(\frac{a}{\left(\frac{2 a}{b}\right)^2}-\frac{b}{\frac{2 a}{b}}=-\frac{b^2}{4 a}\)

∴ dissociation energy = \(=\frac{b^2}{4 a}\)

Question 17. If in a wire of Young modulus Y, a longitudinal strain of x is produced, the value of the potential energy stored in its unit volume will be

1. Yx²
2. 2Yx²
3. 0.5Yx²
4. 0.5Y²x

Answer: 4. 0.5Y²x

Elastic potential energy density = \(\frac{U}{\text { volume }}=\frac{1}{2}\) (stress)(strain)

= \(\frac{1}{2}\) [Y(strain)(strain)]

= \(\frac{1}{2}\) Y(strain)²

= 0.5Yx²

Question 18. A metal ring of initial radius r and cross-sectional area A is fitted onto a wooden disc of radius R > r. If the Young modulus of the metal is Y, the tension in the ring is

1. \(\frac{A Y R}{r}\)
2. \(\frac{Y r}{AR}\)
3. \(\frac{A Y(R-r)}{r}\)
4. \(\frac{Y(R-r)}{A r}\)

Answer: 3. \(\frac{A Y(R-r)}{r}\)

Initial length = L = 2πr and final length = 2πR.

∴ extension = AL = final length- initial length = (R- r).

∴ tension = \(F=\frac{Y A \cdot \Delta L}{L}\)

= \(\frac{Y A \cdot 2 \pi(R-r)}{2 \pi r}\)

= \(\frac{A Y(R-r)}{r}\)

Question 19. The compressibility of water is 4 x 10^-5 per unit atmospheric pressure. The decrease in the volume of 100 cm3 water under a pressure of 100 atm will be

1. 0.4 cm³
2. 4 x 10^–5 cm³
3. 0.025 cm³
4. 0.004 cm³

Answer: 1. 0.4 cm³

Compressibility = \(\frac{1}{B}=\frac{\Delta V}{p V}\)

∴ \(4 \times 10^{-5} \mathrm{~atm}=\frac{\Delta V}{\left(100 \times 10^{-6} \mathrm{~m}^3\right)(100 \mathrm{~atm})}\)

∴ the decrease in volume = AV = 4 x 10^-7 m³

= 0.4 cm³.

Question 20. The breaking stress of a wire depends upon the

1. Length of the wire
2. The radius of the wire
3. Material of the wire
4. The shape of its cross-section

Answer: 3. Material of the wire

The breaking stress does not depend on the shape and size of the wire. It depends on the material of the wire.

Question 21. The length of a metal wire is l₂under a tension of T₁and l₁ under a tension of T₂. The natural length of the wire is

1. \(\frac{l_1+l_2}{2}\)
2. \(\sqrt{h_1 l_2}\)
3. \(\frac{l_1 T_2-l_2 T_1}{T_2-T_1}\)
4. \(\frac{h_1 T_2+l_2 T_1}{T_1+T_2}\)

Answer: 3. \(\frac{l_1 T_2-l_2 T_1}{T_2-T_1}\)

Let U be the original length of the wire,

∴ under the tension T₁ stretch = l1-l

and under the tendon Tystretch = l2-l

∵ stretching force = extension

∴ \(\frac{T_1}{T_2}=\frac{l_1-l}{l_2-l}\)

Hence, \(l=\frac{l_1 T_2-l_2 T_1}{T_2-T_1}\)

Question 22. A steel cable with a cross-sectional area of 3 cm² has an elastic limit of 2.4 x 10⁸ N m^-2. The maximum upward acceleration that can be given to a 1200-kg elevator supported by this cable, if the stress is not to exceed one-third of its elastic limit, is (assuming g = 10 m s^-2)

1. 12 ms^-2
2. 10ms^-2
3. 8ms^-2
4. 7ms^-2

Answer: 2. 10ms^-2

Maximum tension = \(\frac{1}{3}\) (stress)(area)

= \(\frac{1}{3}\left(2.4 \times 10^8 \mathrm{Nm}^{-2}\right)\left(3 \times 10^{-4} \mathrm{~m}^2\right)\)

= 2.4 x 10⁴N

Ifais the maximum upward acceleration of die elevator,
tension = T= m(g +a)

2.4 X10⁴N = (1200 kg)(10 ms^-2 + a)

a = 10 ms^-2

Question 23. The Young modulus for a steel wire is 2 x 10¹¹ Pa and- its elastic limit is 2.5 x 10⁸ Pa. By how much can a steel wire of 3 m length and 2 mm diameter be stretched before its elastic limit is reached?

1. 3.75 mm
2. 7.50 mm
3. 4.75 mm
4. 4.00 mm

Answer: 1. 3.75 mm

The maximum stretching force is

F = (elastic limit)(area of cross-section)

= (2.5 x 10⁸ N m^-2)(rc)(10^-3 m)²

= 2.5n x 10²N.

∴ the length by which the wire can be stretched is

⇒ \(\Delta L=\frac{F}{A} \cdot \frac{L}{Y}=\frac{250 \times \pi \times 3}{\pi \times 10^{-6} \times 2 \times 10^{11}}\)

= 3.75 x 10^-3m

= 3.75 mm.

Question 24. For a constant hydraulic stress P on an object of bulk modulus B, the fractional change in the volume of the object will be

1. \(\frac{P}{B}\)
2. \(\frac{B}{P}\)
3. \(\sqrt{\frac{P}{B}}\)
4. \(\left(\frac{B}{P}\right)^2\)

Answer: 3. \(\sqrt{\frac{P}{B}}\)

∵ bulk modulus = B = \(B=\frac{P}{\frac{\Delta V}{V}}\),

∴ fractionalchange in volume = \(\frac{\Delta V}{V}=\frac{P}{B}\)

Question 25. Four wires having the following lengths and diameters are made of the same material. Which of these will have the largest extension when the same tension is applied?

1. Length = 50 cm and diameter = 0.5 mm
2. Length = 100 cm and diameter =1 mm
3. Length = 200 cm and diameter = 2 mm
4. Length = 300 cm and diameter = 3 mm

Answer: 1. Length = 50 cm and diameter = 0.5 mm

Youngmodulus = Y = \(Y=\frac{\frac{F}{A}}{\frac{\Delta L}{L}}-\frac{4 F L}{\pi D^2 \cdot \Delta L}\)

For all the four wires, Y and F are the same.

Hence, \(\Delta L \propto \frac{L}{D^2}\)

In (a), \(\frac{L}{D^2}=\frac{50 \mathrm{~cm}}{(0.05 \mathrm{~cm})^2}=20 \times 10^3 \mathrm{~cm}^{-1}\)

In (b), \(\frac{L}{D^2}=\frac{100 \mathrm{~cm}}{(0.1 \mathrm{~cm})^2}=10 \times 10^3 \mathrm{~cm}^{-1}\)

In (c), \(\frac{L}{D^2}=\frac{200 \mathrm{~cm}}{(0.2 \mathrm{~cm})^2}=5 \times 10^3 \mathrm{~cm}^{-1}\)

In (d), \(\frac{L}{D^2}=\frac{300 \mathrm{~cm}}{(0.3 \mathrm{~cm})^2}=3.3 \times 10^3 \mathrm{~cm}^{-}\)

Hence, AL is maximum in option (1)

Question 26. The approximate depth of an ocean is 2700 m. The compressibility of water is 45.4 x 10¹¹ Pa^-1 and the density of water is 10³ kg m. What fractional compression of water will be obtained at the bottom of the ocean?

1. 1.0 x 10^-2
2. 12 x 10^-2
3. 1.4 x 10^-2
4. 0.8 x 10^-2

Answer: 2. 12 x 10^-2

The excess pressure at the bottom is

Ap = hpg = (2700 m)(10³ kg m)(10 m s^-2) = 27 x 106 Pa.

∵ bulk modulus = B = V \(V\left(\frac{\Delta p}{\Delta V}\right)\)

∴ compressibility = \(\kappa=\frac{1}{B}=\frac{1}{V} \cdot \frac{\Delta V}{\Delta p}\)

∴ the fractional compression in volume is

⇒ \(\frac{\Delta V}{V}\) = k . Ap

= (45.4 x10^-11Pa^-1)(27 x10⁶ Pa)

= 1.2 x 10^-2.

Question 27. Copper of a fixed volume (V) is drawn into a wire of length l. When the wire is subjected to a constant force F, the extension produced in the wire is Al. Which of the following graphs is a straight line?

1. Al versus \(\frac{1}{l}\)
2. Al versus l²
3. AZ versus \(\frac{1}{l^2}\)
4. Al versus l

Answer: 2. Al versus l²

Volume = V= Al.

Young modulus = Y = \(=Y=\frac{\frac{F}{A}}{\frac{\Delta l}{l}}=\frac{F l}{A \cdot \Delta l}\)

∴ extension = \(\Delta l=\frac{F l}{Y A}=\frac{F l^2}{Y V}\)

∴ Δl ∝ l², so the Δl-versus-l² graph is a straight line

Question 28. The Young modulus of steel is twice that of brass. Two wires of the same length and the same area of cross-section, one of steel and another of brass, are suspended from the same roof. If we want the lower ends of the wires to be at the same level, the weights added to the steel and brass wires must be in the ratio

1. 1:1
2. 1:2
3. 2:1
4. 4:1

Answer: 3. 2:1

Let and be the required weights to produce the same extension

⇒ \(\Delta L=\frac{w L}{Y A}\)

Thus, \(\frac{w_{\mathrm{gt}} L}{Y_{\mathrm{st}} A}=\frac{w_{\mathrm{br}} L}{Y_{\mathrm{br}} A}\)

⇒ \(\frac{w_{\mathrm{st}}}{\omega_{\mathrm{br}}}\)

= \(\frac{Y_{\mathrm{st}}}{Y_{\mathrm{br}}}\)

= \(\frac{2}{1} \Rightarrow w_{\mathrm{st}}: w_{\mathrm{br}}=2: 1\)

Question 29. Which among the following materials is the most elastic?

1. Iron
2. Copper
3. Quartz
4. Rubber

Answer: 3. Quartz

Quartz is the closest approach to a perfectly elastic material.

Question 30. A substance breaks down by a stress of 10⁶ N m^-2. If the density of the material of a wire is 3 x 10³ kg m^-3, the length of the wire which will break under its own weight when suspended vertically will be

1. 66.6 m
2. 60.0 m
3. 30.3 m
4. 33.3 m

Answer: 3. 30.3 m

Breaking stress = \(\frac{m g}{A}=\frac{(A L) \rho g}{A}=L \rho g\)

∴ L = \(\frac{\text { breaking stress }}{\rho g}\)

= \(\frac{10^6 \mathrm{~N} \mathrm{~m}^{-2}}{\left(3 \times 10^3 \mathrm{~kg} \mathrm{~m}^{-3}\right)\left(10 \mathrm{~m} \mathrm{~s}^{-2}\right)}\)

= 33.3 m.

Question 31. A body of mass m = 10 kg is attached to a wire of length 0.3 m. Calculate the maximum angular velocity with which it can be rotated in a horizontal circle. (Given that the breaking stress of the wire = 4.8 x 10⁷ N m^-2 and the area of cross-section of the wire = 10^-6 m².)

1. 4 rad s^-1
2. 8 rad s^-1
3. 1 rad s^-1
4. 2 rad s^-1

Answer: 1. 4 rad s^-1

The breaking force is the tension produced in the wire while the body undergoes a circular motion.

Thus, tension = F = (breaking stress)(area of cross-section)

∴ mω²l = (4.8 x 10⁷Nm^-2)(10^-6 m²) = 48 N

∴ angular frequency =ω = \(\sqrt{\frac{48^{\prime} \mathrm{N}}{(10 \mathrm{~kg})(0.3 \mathrm{~m})}}\)

= \(4 \mathrm{rad} \mathrm{s}^{-1}\)

Question 32. A sphere of radius 3 cm is subjected to a pressure of 100 atm. Its volume decreases by 0.3 cm³. What is the bulk modulus of its material?

1. 4π x 10⁵ atm
2. 4π x 10⁶ atm
3. 4π x 3 x 10³ atm
4. 4π x 10⁸ atm

Answer: 3. 4π x 3 x 10³ atm

Bulk modulus = \(B=\frac{\text { stress }}{\text { volume strain }}\)

⇒ \(V\left(\frac{\Delta p}{\Delta V}\right)\)

= \(\frac{\frac{4}{3} \pi(3 {~cm})^3(100 {~atm})}{0.3 {~cm}^3}\)

= 4π x 3 x 10³ atm.

Question 33. A solid sphere of radius R made of a material of bulk modulus B is surrounded by a liquid contained in a cylindrical container. A massless piston of area A floats on the surface of the liquid. When a block of mass m is placed on the piston to compress the liquid, the fractional change in the radius of the sphere is

1. \(\frac{m g}{3 A R}\)
2. \(\frac{m g}{A}\)
3. \(\frac{m g}{3 A B}\)
4. \(\frac{m g}{A B}\)

Answer: 3. \(\frac{m g}{3 A B}\)

Volume of the sphere = V = \(\frac{4}{3} \pi R^3\)

∴ \(\frac{\Delta V}{V}=3\left(\frac{\Delta R}{R}\right)\)

bulk modulus = \(B=V\left(\frac{\Delta p}{\Delta V}\right)\)

∴ \(\frac{\Delta V}{V}=\frac{\Delta p}{B}=\frac{\frac{m g}{A}}{B}=\frac{m g}{A B}\)

⇒ \(3\left(\frac{\Delta R}{R}\right)=\frac{m g}{A B}\)

⇒ \(\frac{\Delta R}{R}=\frac{m g}{3 A B}\)

Question 34. A given quantity of an ideal gas is at a pressure p and an absolute temperature T. The isothermal bulk modulus of the gas is

1. \(\frac{2p}{3}\)
2. p
3. \(\frac{3}{2}\)
4. 2p

Answer: 2. p

For an isothermal process, pV = constant.

∴ P.ΔV + V.Δp = 0

⇒ \(p=-V\left(\frac{\Delta p}{\Delta V}\right)=\frac{\Delta p}{-\frac{\Delta V}{V}}\)

=\(\frac{\text { stress }}{\text { volume strain }}\)

= B

Thus, bulk modulus = B = p.

Question 35. One end of a thick horizontal copper wire of length 2L and radius 2R is welded to one end of a thin horizontal copper wire of length L and radius R. When this arrangement is stretched by applying equal forces at the two ends, the ratio of the elongation in the thin wire to that in the thick wire is equal to

1. 0.25
2. 0.50
3. 2
4. 4

Answer: 3. 2

The elongation in the thin wire is

⇒ \(h=\frac{F L}{\pi R^2 Y}\)

The elongation in the thick wire is

⇒ \(h_2=\frac{F(2 L)}{\pi(2 R)^2 Y}\)

∴ \(\frac{h_1}{h_2}=\frac{L}{2 L} \cdot \frac{4 R^2}{R^2}=2\)

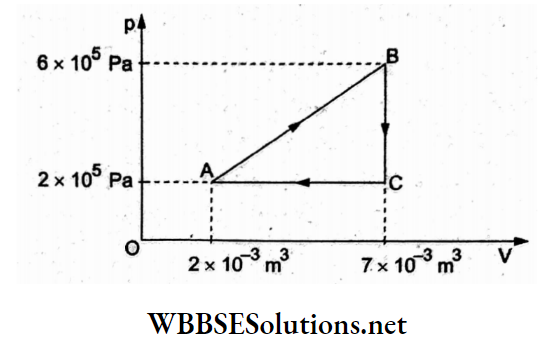
Question 36. The adjoining graph shows the extension (Al) of a wire of length 1 m suspended 4xio-3m from a rigid support at one end with a load w connected to the other end. If the cross-sectional area of the wire is 10^-6 m², calculate 1 x 10 m the Young modulus of the material of the wire.

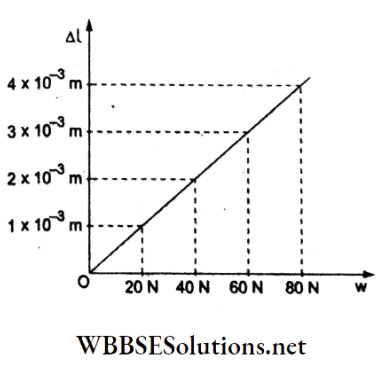
1. 2 x10¹¹ N m^-2
2. 2 x 10^-11 N m^-2
3. 2 x 10¹² Nm^-2
4. 2 x 10¹³ N m^-2

Answer: 1. 2 x10¹¹ N m^-2

From the given load-extension graph, when the load is w = 20 N, the extension is Al = 1 x 10^-4 m.

Young modulus = Y = \(\frac{w / A}{\Delta l / l}\)

= \(\frac{(20 \mathrm{~N})(1 \mathrm{~m})}{\left(10^{-6} \mathrm{~m}^2\right)\left(1 \times 10^{-4} \mathrm{~m}\right)}\)

= 2 x 10¹¹ Nm^-2.

Question 37. A longitudinal strain is possible in

1. Solids
2. Liquids
3. Gases
4. All of these

Answer: 1. Solids

The longitudinal strain is possible only in solids.

Question 38. Which of the following affects the elasticity of a substance? Choose the best option,

1. Impurity of the substance
2. Hammering and annealing
3. A change in temperature
4. All of these

Answer: 4. All of these

Impurity, hammering, and temperature variation affect the elastic property of a substance.

Question 39. For a constant hydraulic stress on an object, the fractional change \(\left(\frac{\Delta V}{V}\right)\) in the object’s volume and its bulk modulus are related as

1. \(\frac{\Delta V}{V} \propto B^2\)
2. \(\frac{\Delta V}{V} \propto \frac{1}{B}\)
3. \(\frac{\Delta V}{V} \propto B\)
4. \(\frac{\Delta V}{V} \propto \frac{1}{B^2}\)

Answer: 2. \(\frac{\Delta V}{V} \propto \frac{1}{B}\)

Bulk modulus = B = \(\frac{\text { strese }}{\frac{\Delta V}{V}}\)

∴ \(B \propto \frac{1}{\frac{\Delta V}{V}} \Rightarrow \frac{\Delta V}{V} \propto \frac{1}{B}\)

Question 40. The shear modulus is zero for

1. Solids only
2. Liquids only
3. Gases only
4. Both liquids and gases

Answer: 3. Gases only

In solids and liquids, a shearing strain may exist, so they have a shear modulus. But in gases, there is no shearing and hence no shear modulus.

Question 41. A uniform wire of length l is extended to a new length l’. The work done is

1. \(\frac{Y A}{l}\left(l^{\prime}-l\right)\)
2. \(\frac{Y A}{l}\left(l^{\prime}-l\right)^2\)
3. \(\frac{Y A}{2 l}\left(l^{\prime}-l\right)^2\)
4. \(\frac{2 Y A}{l}\left(l^{\prime}-l\right)^2\)

Answer: 3. \(\frac{Y A}{2 l}\left(l^{\prime}-l\right)^2\)

Work done = W = strain energy stored = \(\frac{1}{2}\)(stress)(strain)(volume),

stress = Y(strain) = \(Y\left(\frac{l-l}{I}\right)\) and volume = Al.

∴ \(W=\frac{1}{2} Y\left(\frac{l^{\prime}-l}{l}\right)\left(\frac{l^{\prime}-l}{l}\right)(A l)\)

= \(\frac{Y A}{2 l}\left(l^{\prime}-l\right)^2\)

Question 42. The bulk modulus of the material of a sphere is B. If it is subjected to a uniform pressure p, the fractional decrease in its radius is

1. \(\frac{p}{B}\)
2. \(\frac{3p}{B}\)
3. \(\frac{B}{3p}\)
4. \(\frac{p}{3B}\)

Answer: 4. \(\frac{p}{3B}\)

Volume = V = \(\frac{4}{3} \pi R^3\)

⇒ \(\frac{\Delta V}{V}=3\left(\frac{\Delta R}{R}\right)\)

⇒ \(\frac{\Delta R}{R}=\frac{1}{3}\left(\frac{\Delta V}{V}\right)\) ….(1)

Bulk modulus = B = \(\left(\frac{V}{\Delta V}\right) p=\frac{R p}{3 \Delta R}\) [from(1)]

⇒ \(\frac{\Delta R}{R}=\frac{p}{3 B}\)

Question 43. A uniform rod of weight w is supported by two parallel knife edges A and B, and it is in equilibrium in a horizontal position. The two knives are at a distance d from each other. The center of mass of the rod is at a distance x from A. The normal reaction on A is

1. \(\frac{wx}{d}\)
2. \(\frac{wd}{x}\)
3. \(\frac{w(d-x)}{d}\)
4. \(\frac{w(d-x)}{x}\)

Answer: 3. \(\frac{w(d-x)}{d}\)

Let NA and NB be the normal reactions at the knife edges A and B respectively, and w be the weight, as shown. To find NA, we take the moments of the forces about B, which gives

N_Ad – w(d – x) = 0 [ ∵ the system is in equilibrium]

⇒ \(N_{\mathrm{A}}=\frac{w(d-x)}{d}\)

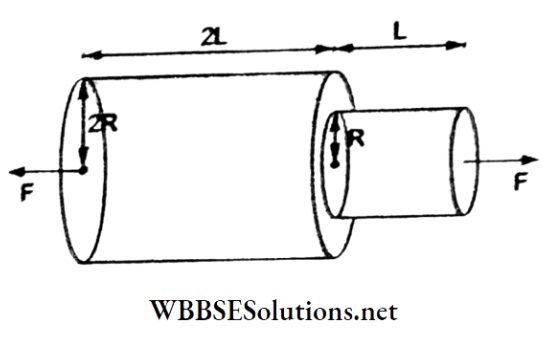
Question 44. If the ratios of the diameters, length,s and Young moduli of the steel and copper wires shown in the given figure are p, q and s respectively, the corresponding ratio of the increases in their lengths would be

1. \(\frac{5 q}{2 s p^2}\)
2. \(\frac{7 q}{5 s p^2}\)
3. \(\frac{2 q}{5 s p}\)
4. \(\frac{7 q}{5 s p}\)

Answer: 2. \(\frac{7 q}{5 s p^2}\)

Young6 modulus = Y = \(\frac{\frac{F}{A}}{\frac{\Delta L}{L}}=\frac{4 F L}{\pi D^2 \cdot \Delta L}\)

∴ extension = \(\Delta L=\frac{4 F L}{\pi D^2 \gamma}\)

For steel, \(\Delta L_{\mathrm{st}}=\frac{4 F_{\mathrm{st}} L_{\mathrm{st}}}{\pi D_{\mathrm{st}}^2 Y_{\mathrm{st}}}\)

Similarly, for copper, \(\Delta L_{C u}=\frac{4 F_{C u} L_{C u}}{\pi D_{C u}^2 Y_{C u}}\)

∴ \(\frac{\Delta L_{\mathrm{st}}}{\Delta L_{\mathrm{Cu}}}=\frac{F_{\mathrm{st}}}{F_{\mathrm{Cu}}} \frac{L_{\mathrm{st}}}{L_{\mathrm{Cu}}}\left(\frac{D_{\mathrm{cu}}}{D_{\mathrm{st}}}\right)^2\left(\frac{Y_{\mathrm{cu}}}{Y_{\mathrm{st}}}\right)\)

⇒ \(\left(\frac{7 m g}{5 m g}\right)(q)\left(\frac{1}{p}\right)^2\left(\frac{1}{s}\right)\)

= \(\frac{7 q}{5 s p^2}\)

Question 45. A uniform cylindrical rod of length L and radius r is made of a material whose Young modulus of elasticity is Y. When this rod is heated by a temperature AT and simultaneously subjected to a net longitudinal compressional force F, its length remains unchanged. The coefficient of volume expansion of the material of the rod is nearly equal to

1. \(\frac{F}{3 \pi r^2 Y \cdot \Delta T}\)
2. \(\frac{6 F}{\pi r^2 \gamma \cdot \Delta T}\)
3. \(\frac{9 F}{\pi r^2 Y \cdot \Delta T}\)
4. \(\frac{3 F}{\pi r^2 Y \cdot \Delta T}\)

Answer: 4. \(\frac{3 F}{\pi r^2 Y \cdot \Delta T}\)

The coefficient of linear expansion is given by

⇒ \(\alpha=\frac{1}{L} \cdot \frac{\Delta L}{\Delta T}=\frac{\text { longitudinal strain }}{\Delta T}\)

∴ Young modulus = Y = \(\frac{\text { stress }}{\text { strain }}=\frac{\frac{F}{A}}{\alpha \cdot \Delta T}\)

⇒ \(\alpha=\frac{F}{Y A \cdot \Delta T}\)

Hence, the coefficient of volume expansion is

⇒ \(\gamma=3 \alpha=\frac{3 F}{A Y \cdot \Delta T}=\frac{3 F}{\pi r^2 Y \cdot \Delta T}\)

Question 46. When a block of mass M is suspended by a long wire of length L, the extension produced is l. The elastic potential energy stored in the stretched wire is

1. Mgl
2. MgL
3. \(\frac{1}{2}\) MgL
4. \(\frac{1}{2}\) Mgl

Answer: 4. \(\frac{1}{2}\) Mgl

The elastic potential energy in a stretched wire is given by

⇒ \(U=\frac{1}{2} k x^2=\frac{1}{2}(k x) x=\frac{1}{2} F_{\max }\) (extension)

= \(\frac{1}{2}\)(stretching force)(stretch)

= \(\frac{1}{2}\) Mgl

Question 47. A boy’s catapult is made of a rubber cord, which is 42 cm long with 6 mm diameter of cross section and of a negligible mass. The boy keeps a stone weighing 0.02 kg on it and stretches the cord by 20 cm by applying a constant force. When released, the stone flies off with a velocity of 20 m s^-1. Neglect the change in the area of the cross-section of the cord while stretched. The Young modulus of rubber is close to

1. 5 x 10⁴ N m^-2
2. 4 x 10⁸ N m^-2
3. 3 x 10⁶ N m^-2
4. 2 x 10³ N m^-2

Answer: 3. 3 x 10⁶ N m^-2

The elastic potential energy stored in the rubber cord on stretching is

⇒ \(U=\frac{1}{2} \text { (stress) (strain)(volume) }\)

⇒ \(\frac{1}{2} Y(\text { strain })^2 \text { (volume) }\)

⇒ \(\frac{1}{2} Y\left(\frac{\Delta L}{L}\right)^2 A L\)

= \(\frac{Y(\Delta L)^2 A}{2 L}\)

This energy appears as the KE of the stone.

Thus, \(\frac{1}{2} m v^2=\frac{Y(\Delta L)^2 A}{2 L}\)

∴ Young modulus = Y = \(\frac{m v^2 L}{(\Delta L)^2 A}\)

Substituting the appropriate values, we have

⇒ \(Y=\frac{\left(2 \times 10^{-2} \mathrm{~kg}\right)\left(20 \mathrm{~m} \mathrm{~s}^{-1}\right)^2(0.42 \mathrm{~m})}{\left(2 \times 10^{-1} \mathrm{~m}\right)^2(3.14)\left(3 \times 10^{-3} \mathrm{~m}\right)^2} \approx 3 \times 10^6 \mathrm{~N} \mathrm{~m}^{-2}\)

Question 48, The elastic limit of brass is 379 MPa. What should be the minimum diameter of a brass rod if it is to support a 400-N load without exceeding the elastic limit?

1. 1.00 mm
2. 1.16 mm
3. 1.86 mm
4. 0.90 mm

Answer: 2. 1.16 mm

The elastic limit is the maximum permissible stress for a given material.

Thus,

⇒ \(379 \times 10^6 \mathrm{~Pa}=\frac{400 \mathrm{~N}}{\pi\left(\frac{d}{2}\right)^2}=\frac{1600 \mathrm{~N}}{\pi d^2}\)

∴ minimum diameter = d = \(\sqrt{\frac{1600 \mathrm{~N}}{(3.14)\left(379 \times 10^6 \mathrm{~N} \mathrm{~m}^{-2}\right)}}\)

= 1.159 mm ≈ 1.16 mm.

Question 49. A wire of length L and an area of cross-section A is hanging from a fixed support. The length of the wire changes to L₁ when a block of mass M is suspended from its free end. The expression for its Young modulus is

1. \(\frac{M g\left(L_1-L\right)}{A L}\)
2. \(\frac{M g L}{A L_1}\)
3. \(\frac{M g L}{A\left(L_1-L\right)}\)
4. \(\frac{M g L_1}{A L}\)

Answer: 3. \(\frac{M g L}{A\left(L_1-L\right)}\)

Young modulus = Y = \(\frac{\text { stress }}{\text { longitudinal strain }}\)

⇒ \(\frac{\frac{F}{A}}{\frac{\Delta L}{L}}\)

= \(\frac{\frac{M g}{A}}{\frac{\left(L_1-L\right)}{L}}\)

= \(\frac{M g L}{A\left(L_1-L\right)}\)

Question 50. A 2-m-long rope of uniform cross-sectional area 2 cm² is being pulled by two persons towards themselves, each exerting a force of 100 N on the rope. If the rope extends in length by 1 cm, the Young modulus of the material of the rope is

1. 2 x 10⁶ N m^-2
2. 1 x 10⁸ Nm^-2
3. 1 x 10¹⁰ N m^-2
4. 1 x 10⁷ N m^-2

Answer: 2. 1 x 10⁸ Nm^-2

Given that tension = F = 100 N, L = 2 m,

ΔL = 1 cm =1 x 10² m and A = 2 x 10^-4 m².

∴ \(Y=\frac{\frac{F}{A}}{\frac{\Delta L}{L}}=\frac{F}{A} \cdot \frac{L}{\Delta L}\)

= \(\frac{(100 \mathrm{~N})(2 \mathrm{~m})}{\left(2 \times 10^{-4} \mathrm{~m}^2\right)\left(1 \times 10^{-2} \mathrm{~m}\right)}\)

= 100 x 10⁶Nm^-2

= 1 x 10⁸ N m^-2.

Question 51. If the liquid in a capillary tube neither rises nor falls inside it, the angle of contact is

1. 0°
2. 180°
3. 90°
4. 45°

Answer: 3. 90°

When the angle of contact is 0 = 90°, the surface tension acts perpendicular to the wall of the capillary tube and its vertically upward component will be zero. Hence, there will be no rise of the liquid column.

In the other words, h = \(\frac{2 S \cos \theta}{r \rho g}\)

= 0 for θ

= 90°.

Question 52. The pressures inside two soap bubbles are 1.01 atm and 1.03 atm. The ratio of their volumes is

1. 27:1
2. 3:1
3. 127:101
4. None of these

Answer: 1. 27:1

The excess pressure inside a soap bubble is given by p = \(\frac{\Delta S}{R}\)

∴ \(\frac{p_1}{p_2}=\frac{R_2}{R_1}\)

Hence, the ratio of die volumes is

⇒ \(\frac{V_1}{V_2}=\left(\frac{R_1}{R_2}\right)^3\)

= \(\left(\frac{p_2}{p_1}\right)^3\)

= \(\left(\frac{0.03}{0.01}\right)^3\)

= 27:1.

Question 53. The wettability of a surface by a liquid depends primarily on the

1. Viscosity of the liquid
2. Surface tension of the liquid
3. Density of lhe liquid
4. The angle of contact between the surface and the liquid

Answer: 4. Angle of contact between the surface and the liquid

The wettability of a solid surface by a liquid depends on the angle of contact 0. Liquids with 0 < 90° wet, and liquids with 0 > 90° do not wet. For no wetting (glass-mercury pair), the adhesive force (F_gl-Hg) is less than the cohesive force (F_Hg-Hg)- For wetting (glass-water pair), the adhesive force (F_gl-W) is greater than the cohesive force (Fw_w).

Question 54. A certain number of spherical drops, each of radius r, of a liquid coalesce into a single drop of radius R and volume V. If T is the surface tension of the liquid then

1. Energy released = \(4 V T\left(\frac{1}{r}-\frac{1}{R}\right)\)
2. Energy absorbed = \(3 V T\left(\frac{1}{r}+\frac{1}{R}\right)\)
3. Energy released = \(=3 V T\left(\frac{1}{r}-\frac{1}{R}\right)\)
4. Energy is neither released nor absorbed

Answer: 3. Energy’released = \(=3 V T\left(\frac{1}{r}-\frac{1}{R}\right)\)

Since the volume remains constant, V = \(\frac{4}{3} \pi R^3=N\left(\frac{4}{3} \pi r^3\right)\)

Initial surface area = A_i = N(4πr²) and final surface area = A_f = 4πR². Since liquids have the property to contract, the final surface area will be less than the initial surface area, and thus energy is released.

The energy released = ΔU = surface tension x ΔA

= T(N.4πr² – 4πR²)

= \(\left(N \cdot \frac{4}{3} \cdot \frac{\pi r^3}{r}-\frac{4 \pi}{3} \cdot \frac{R^3}{R}\right)\)

= \(3 V T\left(\frac{1}{r}-\frac{1}{R}\right)\)

Question 55. A square wireframe of side L is dipped in a liquid. On taking it out, a membrane is formed. If the surface tension of the liquid is T, the force acting on the wireframe will be

1. 2TL
2. 4TL
3. 8TL
4. 10TL

Answer: 3. 8TL

The membrane in the air has two surfaces.

The total force acting on the frame is

F = (surface tension)(perimeter) x 2

= (T)(4L)(2)

= 8TL

Question 56. At its critical temperature, the surface tension of a liquid

1. Is zero
2. Is infinity
3. Is the same as that at any other temperature
4. Cannot be determined

Answer: 1. Is zero

The surface tension decreases with an increase in temperature and its value is zero at the critical temperature.

Question 57. Two spherical soap bubbles of radii a and b in a vacuum coalesce under isothermal conditions. The resulting bubble has a radius equal to

1. \(\frac{a+b}{2}\)
2. \(\frac{a b}{a+b}\)
3. \(\sqrt{a^2+b^2}\)
4. a + b

Answer: 3. \(\sqrt{a^2+b^2}\)

In a vacuum under isothermal conditions, the surface energy remains unchanged. Thus,

8πa²S + 8πb²S = 8πR²S.

⇒ \(R=\sqrt{a^2+b^2}\)

Question 58. The surface tension of a soap solution is 25 x 10^-3 Nml The excess pressure in a soap bubble of diameter 1 cm is

1. 10 Pa
2. 20 Pa
3. 5 Pa
4. None of these

Answer: 2. 20 Pa

The excess pressure inside each soap bubble is

⇒ \(\Delta p=\frac{4 S}{r}\)

= \(\frac{4\left(25 \times 10^{-3} \mathrm{~N} \mathrm{~m}^{-1}\right)}{0.5 \times 10^{-2} \mathrm{~m}}\)

=20 Pa

Question 59. The force required to separate two glass plates of area 10^-2 m², with a 0.05-mm-thick film of water between them, is (given that surface tension of water = 70 x 10^-3 N m^-1)

1. 25 N
2. 20 N
3. 14 N
4. 28 N

Answer: 4. 28 N

The pressure difference across the glass plates due to a thin layer of water of thickness d is given by

⇒ \(\Delta p=\frac{S}{R}=\frac{S}{\frac{d}{2}}=\frac{2 S}{d}\)

Hence, the required force is

⇒ \(F=A \cdot \Delta p\)

= \(\frac{\left(10^{-2} \mathrm{~m}^2\right)\left(2 \times 70 \times 10^{-3} \mathrm{~N} \mathrm{~m}^{-1}\right)}{0.05 \times 10^{-3} \mathrm{~m}}\)

= 28 N.

Question 60. For different capillaries of radius r, the condition for liquid rise (h) above the free surface of the liquid is

1. \(\frac{h}{r}\) = constant
2. hr = constant
3. h + r = constant
4. h-r = constant

Answer: 2. hr = constant

The capillary rise due to the surface tension (S) is given by

⇒ \(h=\frac{2 S \cos \theta}{r \rho g}\)

For a given solid-liquid pair; S, 0, p, and are constant.

Hence, the products also constant.

Question 61. The excess pressure inside a soap bubble of radius r is proportional to

1. r
2. \(\frac{1}{r}\)
3. r2
4. \(\frac{1}{r^2}\)

Answer: 2. \(\frac{1}{r}\)

The excess pressure inside a soap bubble is Δp = 4S/r, which is proportional to 1/r.

Question 62. When the temperature is increased, the angle of contact of a liquid

1. Increases
2. Decreases
3. Remains the same
4. First increases and then decreases

Answer: 1. Increases

When the temperature rises, the surface tension decreases, and the angle of contact increases.

Question 63. The radius of a soap bubble is r and the surface tension of the soap solution is T. Keeping the temperature constant, the extra energy needed to double the radius of the bubble by blowing is

1. 8πr²T
2. 16πr²T
3. 24πr²T
4. 32πr²T

Answer: 3. 24πr²T

The extra energy required = work done

= (surface tension)(increase in surface area)

= \(T\left[2.4 \pi(2 r)^2-2.4 \pi(r)^2\right]=24 \pi r^2 T\)

Question 64. A work of 3.0 x 10^-4J is required to stretch a soap film from 10 cm x 6 cm to 10 cm x 11 cm. The surface tension of the soap solution is

1. 5 x 10^-2 N m^-1
2. 3 x 10^-2 N m^-1
3. 1.5 x 10^-2 N m^-1
4. 1.2 x 10^-2Nm^-1

Answer: 2. 3 x 10^-2 N m^-1

Work done = (surface tension) (increase in surface area)

=> 3.0 x 10^-4J =T[2(110 x 10^-4cm²-60 x 10⁴m²)]

= T(10^-2m²)

⇒ \(T=\frac{3.0 \times 10^{-4} \mathrm{~J}}{10^{-2} \mathrm{~m}^2}\)

= \(3.0 \times 10^{-2} \mathrm{Nm}^{-1}\)

Question 65. One large soap bubble of diameter D breaks into 27 bubbles, each having a surface tension of T. The change in the surface energy is

1. 2πTD²
2. 4πTD²
3. πTD²
4. 8πTD²

Answer: 2. 4πTD²

Assuming the volume of air enclosed to be unchanged,

⇒ \(\frac{4}{3} \pi\left(\frac{D}{2}\right)^3=27 \times \frac{4}{3} \pi r^3\)

∴ the radius of the smaller bubble is r = D/6.

Now, the increase in surface area is

⇒ \(\Delta S=27 \times 2\left(4 \pi r^2\right)-2 \times\left[4 \pi\left(\frac{D}{2}\right)^2\right]\)

⇒ \(27 \times 8 \pi\left(\frac{D}{6}\right)^2-2 \times 4 \pi\left(\frac{D}{2}\right)^2\)

= \(4 \pi D^2\)

Hence, the increase in surface energy is

⇒ \(\Delta U=T(\Delta A)=4 \pi T D^2\)

Question 66. A mercury drop of radius 1 cm is broken into 106 droplets of equal sizes. The work done is (the surface tension of mercury being 35 x 10^-2 N m^-1)

1. 4.35 x 10^-2 J
2. 4.35 x 10^-3 J
3. 4.35 x l0^-6J
4. 4.35 x 10^-8 J

Answer: 1. 4.35 x 10^-2 J

Since the volume remains unchanged,

⇒ \(\frac{4}{3} \pi R^3=10^6 \cdot \frac{4}{3} \pi r^3\)

⇒ \(r=\frac{R}{100}\)

∴ work done = T.ΔA

⇒ \(T \cdot 4 \pi\left(10^6 \cdot r^2-R^2\right)=T \cdot 4 \pi\left(10^6 \cdot \frac{R^2}{10^4}-R^2\right)\)

= 4πT(99R²)

== 4(3.14)(35 x 10^-2 N m^-1) x 99(1 x 10^-2m)²

= 4.35 x 10^-2 J.

Question 67. Eight identical mercury droplets coalesce into one mercury drop. The energy changes by a factor of

1. 1
2. 2
3. 4
4. 6

Answer: 2. 2

⇒ \(V=\frac{4}{3} \pi R^3\)

= \(8 \times \frac{4}{3} \pi r^3\)

⇒ \(r=\frac{R}{2}\)

Initial surface energy = U_i = 4πR²T

and final surface energy = U_f = 8(4πr²)T.

⇒ \(8 \times 4 \pi\left(\frac{R}{2}\right)^2 T\)

= \(2\left(4 \pi R^2 T\right)\)

= \(2 U_i\)

Thus, increases by a factor of 2.

Question 68. There is a small bubble at one end and a bigger bubble at the other end of the tube, as shown in the figure. Which of the following statements is true?

1. The smaller bubbles will grow until they collapse.
2. The bigger bubbles will grow until they collapse.
3. They remain in equilibrium.
4. None of these.

Answer: 2. The bigger bubbles will grow until they collapse.

If p₀ be the pressure outside then

inside the smaller bubble, \(p_1=p_0+\frac{4 T}{r}\)

and inside the bigger bubble, \(p_2=p_0+\frac{4 T}{R}\)

∵ r < R;

∴ p₁ > p₂

Hence, air will flow from the smaller bubble to the bigger bubble, which will further grow until both the bubbles collapse.

Question 69. A 15-cm-long capillary tube is vertically dipped in water. The water rises up to 7 cm. If the entire setup is put in a freely falling elevator, the length of the water column in the capillary tube will be

1. 3.5 cm
2. 15 cm
3. 6 cm
4. 8 cm

Answer: 2. 15 cm

During free fall (under gravity), the system is in a state of artificial weightlessness.

So, effectivelyg = 0.

Hence, gravity does not work but the force of surface tension still acts and the maximum ascent of the water column occurs in the full length of the tube (up to 15 cm).

Question 70. The surface tension of a liquid decreases with a rise in the

1. Viscosity of the liquid
2. Temperature of the liquid
3. Diameter of the container
4. Thickness of the container

Answer: 2. Temperature of the liquid

The surface tension decreases with rise in temperature as

S_θ = S₀ (l – αθ),

where a is the temperature coefficient of surface tension.

Question 71. The potential energy of a soap bubble having a surface tension equal to 0.04 N m^-1 and of diameter 1 cm is

1. 6π x 10^-6 J
2. 4π x 10^-6 J
3. 2π x 10^-6 J
4. 8π x 10^-6 J

Answer: 4. 8n x 10-6J

Potential energy = U = T x surface area

= T x 2(4πR²)

= 8πTR²

= Sπ (0.04 N m^-1) (0.5 x 10^-2 m)²

= 8π X 10^-6 J.

Question 72. When there are no external forces, the shape of a small liquid drop is determined by the

1. Surface tension of the liquid
2. Viscosity of the liquid
3. Temperature of air
4. Density of the liquid

Answer: 1. Surface tension of the liquid

The shape of a free liquid drop is determined by its molecular forces, which gives rise to its surface tension

Question 73. Raindrops are spherical due to their

1. Viscosity
2. Surface tension
3. Thrust on dropping
4. Residual pressure

Answer: 2. Surface tension

A liquid surface tends to contract due to its surface tension and assumes a spherical shape, which has the minimum surface area for a given volume.

Question 74. For a liquid to rise in a capillary tube, the angle of contact should be

1. An acute angle
2. An obtuse angle
3. A right angle
4. None of these

Answer: 1. An acute angle

A liquid rises in a capillary tube if the angle of contact (0) between the solid-liquid pair is acute. The vertically upward component of the surface tension (T cos θ) makes the liquid rise.

Question 75. Two small drops of mercury, each of radius R, coalesce into a single large drop. The ratio of the total surface energies before and after the change is

1. 1:2^1/3
2. 2^1/3:1
3. 2:1
4. 1:2

Answer: 2. 2^1/3:1

Volume, \(V=2\left(\frac{4}{3} \pi R^3\right)\)

= \(\frac{4}{3} \pi R^{\prime 3}\)

R’ = 2^1/3R.

Now, U_i = T(2 x 4πR²)

and U_f = T (4πR²)

= T(4π)2^2/3 R²

∴ \(\frac{U_i}{U_f}=\frac{2 R^2}{2^{2 / 3} R^2}=2^{1 / 3}: 1\)

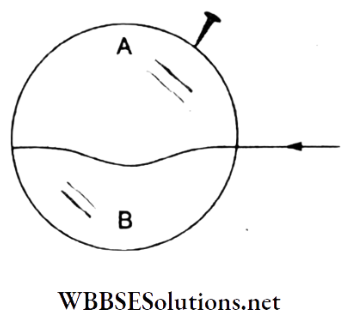
Question 76. A thread is tied slightly loose to a wireframe. The frame is dipped in a soap solution and then taken out. The frame is completely covered with a soap film, as shown in the figure. When the portion A is punctured with a pin, the thread

1. Becomes concave towards A
2. Becomes convex towards A
3. Remains in its initial position
4. Becomes either concave or convex towards A depending on
   the size of A relative to B.

Answer: 1. Becomes concave towards A

When portion A is punctured, the soap film will start contracting and the circular hole created will gradually increase in size. This will lead the thread to become concave towards A.

Question 77. A capillary tube of radius r is immersed in water and the water rises to a height of h. The mass of the water in the capillary tube is 5 g. Another capillary tube of radius 2r is immersed in water. The mass of water that will rise in this tube is

1. 2.5 g
2. 5.0 g
3. 10 g
4. 20 g

Answer: 3. 10 g

In a capillary rise, surface tension = T = \(\frac{{rhpg}}{2 \cos \theta}\)

So, \(r h=\frac{2 T \cos \theta}{\rho g}=\text { constant }\)

⇒ \(r h=2 r h^{\prime}\)

⇒ \(h^{\prime}=\frac{h}{2}\)

Now, \(m=\pi r^2 h\)

and \(m^{\prime}=\pi(2 r)^2\left(\frac{h}{2}\right)\)

∴ \(\frac{m^{\prime}}{m}=\frac{2 \pi r^2 h}{\pi r^2 h}=2\)

m = 2m

= 10g.

Question 78. A rectangular film of a liquid is extended from an area of 4 cm x 2 cm to 5 cm x 4 cm. If the work done is 3 x 10^-4 J, the value of the surface tension of the liquid is

1. 0.25 N m^-1
2. 8.0 Nm^-1
3. 0.125 Nm^-1
4. 0.2 Nm^-1

Answer: 3. 0.125 Nm^-1

Work done = (surface tension)(increase in surface area)

3 x 10^-4 J = T[2(20 x 10^-4 m²-8 x 10^-4m²)]

∴ suface tension = T = \(\frac{3 \times 10^{-4} \mathrm{~J}}{2 \times 12 \times 10^{-4} \mathrm{~m}^2}\)

= \(0.125 \mathrm{Nm}^{-1}\)

Question 79. Three liquids of densities p₁, p_2, and p₃ (where p₁ > p₂ > p₃), and having the same value of surface tension rise to equal heights in three identical capillaries. The angles of contact θ₁, θ₂ and θ₃ obey the relation

1. \(\frac{\pi}{2}>\theta_1>\theta_2>\theta_3>0\)
2. \(0 \leq \theta_1<\theta_2<\theta_3<\frac{\pi}{2}\)
3. \(\frac{\pi}{2}<\theta_1<\theta_2<\theta_3<\pi\)
4. \(\pi>\theta_1>\theta_2>\theta_3>\frac{\pi}{2}\)

Answer: 2. \(0 \leq \theta_1<\theta_2<\theta_3<\frac{\pi}{2}\)

The rise of a liquid in a capillary tube is given by

⇒ \(h=\frac{2 T \cos \theta}{r \rho g}\)

So, \(\frac{\cos \theta}{\rho}=\frac{h r g}{2 T}\)

∴ for the three given capillaries,

⇒ \(\frac{\cos \theta_1}{\rho_1}=\frac{\cos \theta_2}{\rho_2}=\frac{\cos \theta_3}{\rho_3}\)

Given that p₁ > p₂ > p₃

∴ cos θ₁ > cos θ₂ > cos θ₃.

Hence, \(0 \leq \theta_1<\theta_2<\theta_3<\frac{\pi}{2}\)

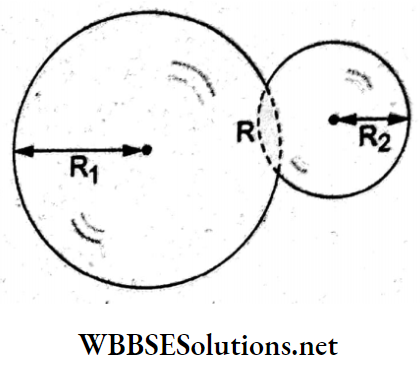
Question 80. Two soap bubbles of radii R₁ and R₂ (where R₁ > R₂) come into contact, as shown in the adjoining figure. The radius of curvature of the common surface is given by

1. \(R=\frac{R_1+R_2}{2}\)
2. \(R=\frac{R_1-R_2}{2}\)
3. \(R=\frac{R_1 R_2}{R_1-R_2}\)
4. \(R=\frac{R_1 R_2}{R_1+R_2}\)

Answer: 3. \(R=\frac{R_1 R_2}{R_1-R_2}\)

If p₀ise the pressure outside the bubbles, the pressure inside the larger bubble is \(p_1=p_0+\frac{4 T}{R_1}\) and that inside the smaller bubble is \(p_2=p_0+\frac{4 T}{R_2}\)

Since p₂ > P₁, the pressure difference across the common surface is

⇒ \(\Delta p=p_2-p_1=\frac{4 T}{R}\)

⇒ \(4 T\left(\frac{1}{R_2}-\frac{1}{R_1}\right)=\frac{4 T}{R}\)

⇒ \(R=\frac{R_1 R_2}{R_1-R_2}\)

Question 81. A liquid drop at a temperature of 0, isolated from its surroundings breaks into a number of droplets. The temperature of the droplets will be

1. Equal to θ
2. Greater than θ
3. Less than θ
4. Either of the three, depending on the surface tension of the liquid

Answer: 3. Less than θ

When the drop breaks into droplets, the total surface area increases, leading to a greater surface energy. This extra energy is drawn from the internal energy of the drop and it cools down. The temperature of the droplets will be less than θ.

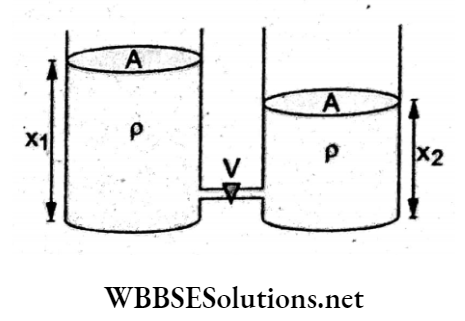
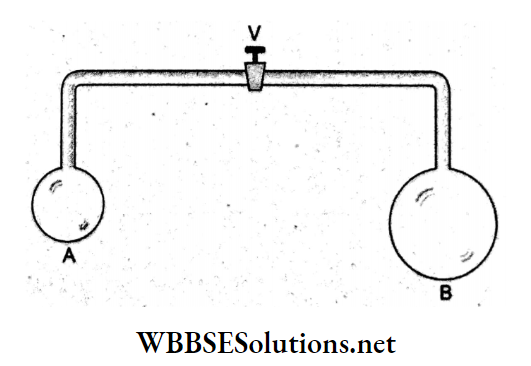
Question 82. The adjoining figure shows two soap bubbles A (smaller) and B (larger) formed at the two open ends of a tube, with its valve V closed. When the valve is opened, air can flow freely between the bubbles. Which of the following options is true?

1. There will be no change in the size of the bubbles.
2. The bubbles will become of equal size.
3. The sizes of A and B will get interchanged.
4. A will become smaller and B will become larger.

Answer: 4. A will become smaller and B will become larger.

The air pressure is greater inside the smaller bubble. Hence, air flows from the smaller bubble to the larger bubble. So, A will become smaller and B will become larger.

Question 83. The arms of a manometer consist of tubes B of radii and r₂ (where r₁ > r₂) filled with a liquid of surface tension S, as shown in the figure. Find the expression for h (the level difference of the menisci at A and B) in terms of r₁,r₂ and S.

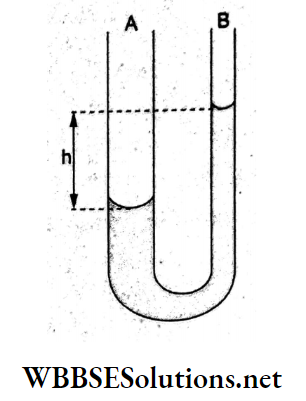
1. \(\frac{S}{\rho g}\left(\frac{r_1-r_2}{r_1 r_2}\right)\)
2. \(\frac{2 S}{\rho g}\left(\frac{r_1-r_2}{r_1 r_2}\right)\)
3. \(\frac{S}{2 \rho g}\left(\frac{r_1+r_2}{r_1 r_2}\right)\)
4. \(\frac{S}{\rho g}\left(\frac{r_1+r_2}{r_1 r_2}\right)\)

Answer: 2. \(\frac{2 S}{\rho g}\left(\frac{r_1-r_2}{r_1 r_2}\right)\)

Let p₀ be the atmospheric pressure.

∴ the pressure below the meniscus at A is \(p_1=p_0-\frac{2 S}{r_1}\)

and that below the meniscus B is \(p_2=p_0-\frac{2 S}{r_2}\)

Equalizing the pressure in the same level at A, we have,

p₁ = p₂ + hpg

⇒ \(p_0-\frac{2 S}{r_1}=p_0-\frac{2 S}{r_2}+h \rho g\)

⇒ \(h=\frac{2 S}{\rho g}\left(\frac{r_1-r_2}{r_1 r_2}\right)\)

Question 84. A soap bubble is blown slowly at the end of a tube by a pump supplying air at a constant rate. Which of the following graphs correctly represents the variation of the excess pressure inside the bubble with time?

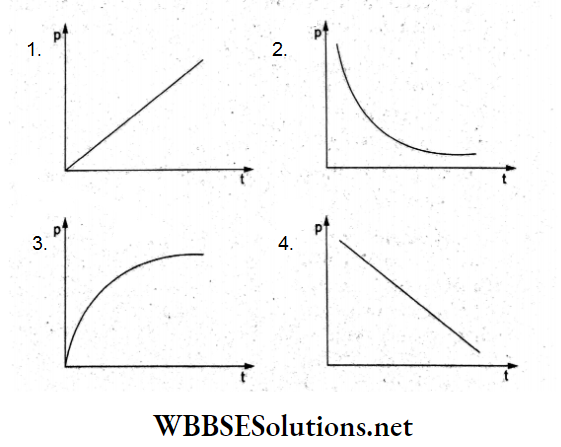
Answer: 2.

The excess pressure inside a soap bubble of radius r is

p = \(\frac{4S}{r}\)

So, \(p \propto \frac{1}{r}\)

Since air is supplied at a constant rate, the radius r will be proportional
to time.

Thus, pt = constant, which is represented by the curve given in option (2)

Question 85. The pressure inside two soap bubbles is 1.01 atm and 1.02 atm respectively. The ratio of their respective volumes is equal to

1. 8
2. 4
3. 16
4. 2

Answer: 1. 8

The excess pressure inside the first bubble is

P_i = \(\frac{4 S}{r_1}\)

= 1.01 atm – 1.0 atm

= 0.01 atm,

and that for the second bubble is

⇒ \(p_2=\frac{4 S}{r_2}=1.02 \mathrm{~atm}-1 \mathrm{~atm}=0.02 \mathrm{~atm}\)

∴ \(\frac{p_2}{p_1}=\frac{r_1}{r_2}=\frac{0.02 \mathrm{~atm}}{0.01 \mathrm{~atm}}\)

= 2.

Hence, \(\frac{V_1}{V_2}=\frac{r_1^3}{r_2^3}\)

= 8.

Question 86. An ice cube floating in a gravity-free space melts and converts into water. The shape of this water is

1. A cylinder
2. An ellipsoid
3. A sphere
4. Unpredictable

Answer: 3. A sphere

In a gravity-free space, any volume of liquid (when free) assumes a spherical shape. Hence, the ice cube will assume the shape of a large spherical drop.

Question 87. Consider an ice cube of edge L kept in a gravity-free space. Assuming the densities of ice and water to be equal, the surface area of the water formed when the ice melts will be

1. (36π)½L²
2. (6π)^1/3L²
3. (36π)^1/3L²
4. None of these

Answer: 3. (36π)^1/3L²

The ice cube of edge L will assume the shape of a sphere of radius R when it melts. Since its volume is unchanged,

⇒ \(L^3=\frac{4}{3} \pi R^3 \Rightarrow R=\left(\frac{3}{4 \pi}\right)^{1 / 3}\)

∴ the final surface area of the spherical drop is

⇒ \(4 \pi R^2=4 \pi\left(\frac{3}{4 \pi}\right)^{2 / 3} L^2=(36 \pi)^{1 / 3} L^2\)

Question 88. A soap bubble having a radius of 1 mm is blown from a detergent solution having a surface tension of 2.5 x 10^-2Nm^-2. The pressure inside the bubble equals a depth z0 below the free surface of water in a container. Taking = 10 ms^-2 and density of water = 10³ kgm^-3, the value of z₀ is

1. 100 cm
2. 10 cm
3. 1 cm
4. 0.5 cm

Answer: 3. 1 cm

The excess pressure inside a soap bubble is given by \(\Delta p=\frac{4 T}{R}\), which is equal to the pressure due to a column (of height z₀) of water (p = Z₀pg).

∴ \(\frac{4 T}{R}=z_0 \rho g\)

⇒ \(z_0=\frac{4 T}{\rho g R}\)

Hence, \(z_0=\frac{4\left(2.5 \times 10^{-2} \mathrm{~N} \mathrm{~m}^{-1}\right)}{\left(10^3 \mathrm{~kg} \mathrm{~m}^{-3}\right)\left(10 \mathrm{~m} \mathrm{~s}^{-2}\right)\left(1 \times 10^{-3} \mathrm{~m}\right)}=10^{-2} \mathrm{n}\)

= 1 cm.

Question 89. The ratio of the surface tensions of mercury and water is given to be 7.5, while the ratio of their densities is 13.6. Their contact angles with their glass containers are close to 135° and 0° respectively. It is observed that mercury gets depressed by an amount of h in a capillary tube of radius rv while water rises by the same amount of h in a capillary tube of radius r₂. The ratio \(\frac{r_1}{r_2}\) is then close to

1. \(\frac{4}{5}\)
2. \(\frac{2}{5}\)
3. \(\frac{2}{3}\)
4. \(\frac{3}{5}\)

Answer: 2. \(\frac{2}{5}\)

The depression of mercury in the capillary tube is

⇒ \(h_{\mathrm{Hg}}=\frac{r_1 h_1 \rho_{\mathrm{Hg}} g}{2 S_{\mathrm{Hg}} \cos \theta_{\mathrm{Hg}}}\)

and the rise of the water level is

⇒ \(h_w=\frac{r_2 h_2 \rho_w g}{2 S_w \cos \theta_w}\)

Given that hHg= hw.

∴ \(\frac{r_1 h_1 \rho_{\mathrm{Hg}} g}{2 S_{\mathrm{Hg}} \cos \theta_{\mathrm{Hg}}}=\frac{r_2 h_2 \rho_{\mathrm{w}} g}{2 S_{\mathrm{w}} \cos \theta_{\mathrm{w}}}\)

∴ \(\frac{r_1}{r_2}=\frac{h_2}{h_1} \cdot \frac{\rho_{\mathrm{w}}}{\rho_{\mathrm{Hg}}} \cdot \frac{S_{\mathrm{Hg}}}{S_{\mathrm{w}}} \cdot \frac{\cos \theta_{\mathrm{Hg}}}{\cos \theta_{\mathrm{w}}}\)

Substituting the appropriate values,

⇒ \(\frac{r_1}{r_2}=\left(\frac{h}{h}\right)\left(\frac{1}{13.6}\right)\left(\frac{7.5}{1}\right)\left(\frac{1}{\sqrt{2}}\right)\)

= 0.4

= \(\frac{2}{5}\)

Question 90. The work done to blow a soap bubble of radius 5 cm is (given that surface tension of the soap solution = 0.1 N m^-1)

1. 6.28 x 10^-3 J
2. 3.14 x 10^-2 J
3. 2.5 x 10^-2 J
4. 5.8 x 10^-3 J

Answer: 1. 6.28 x 10^-3 J

The work done in blowing a soap bubble is

W = (surface tension)(increase in surface area)

= S.ΔA = S(8πR²).

Substituting the values,

W= (0.1 N m^-1) [8 x 3.14 x(5x 10^-2 m)²]

= 62.8 x 10^-4 J

= 6.28 x 10^-3 J.

Question 91. In an isothermal process, two water drops each of a radius 1 mm are combined to form a bigger drop. The amount of energy released during the process is (given that surface tension = 0.1 N m^-1)

1. 1 μJ
2. 0.75 μJ
3. 0.5 μJ
4. 0.25 μJ

Answer: 3. 0.5 μJ

Let R be the radius of the bigger drop.

∴ \(\left(\frac{4}{3} \pi r^3\right) \times 2=\frac{4}{3} \pi R^3\)

=> R = 2^1/3r.

The change in the surface area of the drops is

ΔA = 4πr².2-4πR²

= 4π(2r² -2^2/3r²)

= 4πr²(2-2^2/3).

Since the surface area decreases, energy is released. This energy is
given by

All = S.ΔA = (0.1 Nm^-1)[4 x 3.14 x (10^-3 m)²(2-1.58)]

= 0.52 x 10^-6 J ≈ 0.5 mmmJ

Question 92. A soap bubble, blown by a mechanical pump at the mouth of a tube, increases in volume with time at a constant rate. The graph that correctly depicts, the time dependence of the pressure inside the die bubble is

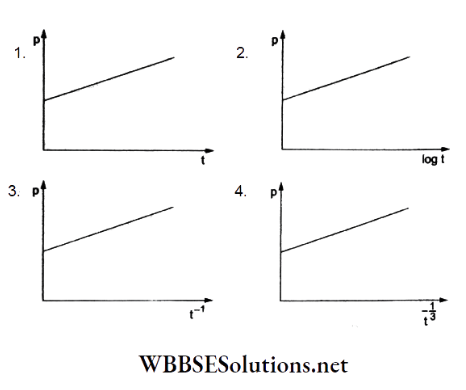
Answer: 4.

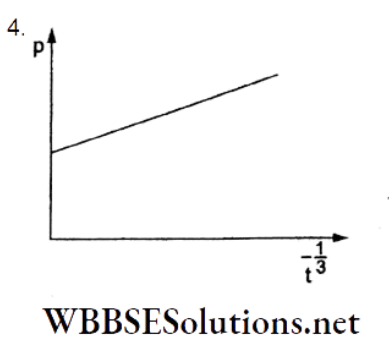
The volume of a soap bubble is \(V=\frac{4}{3} \pi R^3\).

∴ \(\frac{d V}{d t}=4 \pi R^2 \frac{d R}{d t}=\text { constant (given) }\)

∴ R2dR = (constant)dt.

Integrating,

⇒ \(\frac{R^3}{3}=(\text { constant }) t\)

⇒ \(R \propto t^{1 / 3}\)

For a soap bubble, a pressure difference is

⇒ \(p-p_0=\frac{4 S}{R}=\frac{k}{t^{1 / 3}}\)

⇒ \(p=p_0+\frac{k}{t^{1 / 3}}\)

This shows that the graph in option (4) is correct.

Question 93. A capillary tube of radius 0.15 mm is dipped in a liquid of A density 667 kg m^-3. Find the height to which the liquid will rise. [Given that surface tension of the liquid = (1/20) N m^-1 and angle of contact = 60°]

1. 0.02 m
2. 0.01 m
3. 0.05 m
4. 0.04 m

Answer: 4. 0.04 m

The rise of tire liquid (h) in a capillary is given by

⇒ \(T=\frac{r h \rho g}{2 \cos \theta}\)

⇒ \(h=\frac{2 T \cos \theta}{r \rho g}\)

On substituting the given values,

⇒ \(h=\frac{2\left(\frac{1}{20} \mathrm{~N} \mathrm{~m}^{-1}\right) \cos 60^{\circ}}{\left(0.15 \times 10^{-3} \mathrm{~m}\right)\left(667 \mathrm{~kg} \mathrm{~m}^{-3}\right)\left(10 \mathrm{~m} \mathrm{~s}^{-2}\right)}\)

= 0.05m.

Question 94. The pressure inside two soap bubbles is 1.01 atm and 1.02 atm. The ratio of their volumes is

1. 4:1
2. 8:1
3. 1:8
4. 1:4

Answer: 2. 8:1

The excess pressure (p_i -p₀) in a soap bubble is \(\frac{4T}{R}\)

Hence, \(\frac{4 T}{R_1}\) = 1.01 atm^-1 atm

= 0.01 atm

and \(\frac{4 T}{R_2}\) 1.02 atm^-1 atm = 0.02 atm.

∴ \(\frac{R_1}{R_2}=\frac{0.02 \mathrm{~atm}}{0.01 \mathrm{~atm}}\)

= 2.

⇒ \(\frac{V_1}{V_2}=\left(\frac{R_1}{R_2}\right)^3=2^3\)

=8

V₁:V₂ =8:1

Question 95. A capillary tube made of glass and of radius 0.15 mm is dipped vertically in a beaker filled with a liquid of surface tension 5 x 10^-2 Nm ^-1 and density 667 kg m^-3. The capillary rise is h. It is observed that tangents drawn from the meniscus from two diametrically opposite points are inclined at 60°. The value of h is

1. 0.049 m
2. 0.172 m
3. 0.087 m
4. 0.137 m

Answer: 3. 0.087 m

Capillary rise = h = \(\frac{2 T \cos \theta}{r \rho g}\)

= \(\frac{2\left(5 \times 10^{-2} \mathrm{~N} \mathrm{~m}^{-1}\right)\left(\cos 30^{\circ}\right)}{\left(0.15 \times 10^{-3} \mathrm{~m}\right)\left(667 \mathrm{~kg} \mathrm{~m}^{-3}\right)\left(10 \mathrm{~m} \mathrm{~s}^{-2}\right)}\)

⇒ \(\frac{1.732 \times 5 \times 10^{-2}}{0.15 \times 667 \times 10^{-2}} \mathrm{~m}\)

= 0.087 m.

Question 96. A capillary tube of radius r is immersed vertically in water, and the water rises to a height of h. The mass of the water in the capillary is 5 g. Another capillary tube of radius 2r is immersed in water. The mass of the water that will rise in this tube is

1. 5.0 g
2. 10.0 g
3. 20.0 g
4. 2.5 g

Answer: 2. 10.0 g

The weight of the liquid column in the capillary tube is balanced by the force due to surface tension.

Hence, 2πrTcos θ = mg.

For the capillary tube of radius 2r, let the mass of the liquid that rises be m’.

∴ 2π(2r)Tcos θ = m’g.

Hence, \(\frac{m^{\prime}}{m}=2\)

=> m’ = 2m

= 2(5 g)

=10g.

Question 97. Ablockofdensitydx and massMmovesdownwards with a uniform speed in glycerine of density d₂. What is the viscous force acting on the die block?

1. Mgd₁
2. Mgd₂
3. \(M g\left(1-\frac{d_1}{d_2}\right)\)
4. \(M g\left(1-\frac{d_2}{d_1}\right)\)

Answer: 4. \(M g\left(1-\frac{d_2}{d_1}\right)\)

Weight of the block (acting downwards) = Mg,

weight of the liquid = buoyant force (acting upwards)

⇒ \(M^{\prime} g=V d_2 g=\left(\frac{M}{d_1}\right) d_2 g\)

and f = viscous force (upward).

Since the motion is uniform, net force = 0.

∴ \(M g-M\left(\frac{d_2}{d_1}\right) g-f=0\)

⇒ \(f=M g\left(1-\frac{d_2}{d_1}\right)\)

Question 98. An object is moving through a liquid. The viscous damping force acting on it is proportional to the velocity. The dimensions of the constant of proportionality are

1. ML^-1T^-1
2. MLT^-1
3. M₀LT^-1
4. ML₀T^-1

Answer: 4. ML₀T-1

Viscous damping force = f = kv.

∴ \([k]=\frac{[f]}{[v]}\)

= \(\frac{\mathrm{MLT}^{-2}}{\mathrm{LT}^{-1}}\)

= \(\mathrm{ML}^0 \mathrm{~T}^{-1}\)

Question 99. Which of the following is not a dimensional quantity?

1. Acceleration due to gravity
2. Surface tension
3. Reynolds number
4. Velocity of light

Answer: 3. Reynolds number

The critical velocity is given by \(v_c=\frac{k \eta}{\rho R}\) where the Reynolds number k is dimensionless.

Question 100. The Reynolds number for fluid flow through a pipe is independent of the

1. Viscosity of the fluid
2. Velocity of the fluid
3. Length of the pipe
4. Diameter of the pipe

Answer: 3. Length of the pipe

In a fluid flow, critical velocity = \(v_c=\frac{k \eta}{\rho D}\) where the Reynolds number

k is independent of the length of the pipe.

Question 101. When a steel ball is dropped in an oil,

1. The ball attains a constant velocity after some time
2. The speed of the ball will keep on increasing
3. The ball stops after some time
4. None of the above takes place

Answer: 1. The ball attains a constant velocity after some time

A steel ball dropped in oil will experience its’ weight (downward), buoyant force (upward), and viscous force (upward). The viscous force gradually increases with speed, and the net force becomes zero when the ball finally attains a constant terminal velocity.

Question 102. The ratio of the terminal velocities of two drops of radii R and R/2 is

1. 1:1
2. 2:1
3. 4:1
4. 1:4

Answer: 3. 4:1

Terminal velocity = \(v=\frac{2}{9} \cdot \frac{(\rho-r) r^2}{\eta}\)

∴ v ∝ r²

⇒ \(\frac{v_1}{v_2}=\frac{R^2}{(R / 2)^2}\)

= \(\frac{4}{1}\)

v₁: v₂ = 4: 1

Question 103. The volume of a liquid flowing per unit of time through a tube of radius r and length l whose ends are maintained at a pressure difference P is given by \(V=\frac{\pi Q P r^4}{\eta l}\) where t is the coefficient of viscosity and Q is equal to

1. 8
2. \(\frac{1}{8}\)
3. 16
4. \(\frac{1}{16}\)

Answer: 2. \(\frac{1}{8}\)

The volume flowing per unit of time is given by Poiseuille’s equation, i.e,

⇒ \(V=\frac{\pi P r^4}{8 \eta l}\)

Comparing this with the given equation, we have Q = \(\frac{1}{8}\)

Question 104. The radii of two drops of a liquid are in the ratio of 3: 2. Their terminal velocities are in the ratio

1. 2:3
2. 3:2
3. 9:4
4. 4:9

Answer: 3. 9:4

The ratio of the terminal velocities is

⇒ \(\frac{v_1}{v_2}=\frac{r_1^2}{r_2^2}\)

= \(\left(\frac{3}{2}\right)^2\)

= \(\frac{9}{4}\)

Question 105. The coefficient of viscosity of hot air

1. Is Greater Than The Coefficient Of Viscosity Of Cold Air
2. Is The Same As The Coefficient Of Viscosity Of Cold Air
3. Is Smaller Than The Coefficient Of Viscosity Of Cold Air
4. Increases or decreases depending on the external pressure

Answer: 1. Is Greater Than The Coefficient Of Viscosity Of Cold Air

An increase in gas temperature causes the gas molecules to collide more often and thus increases the gas viscosity. Hence, hot air is more viscous than cold air.

Question 106. The viscous force exerted by a liquid flowing between two plates in a streamline flow depends upon

1. The velocity gradient in the direction perpendicular to the plates only
2. The areas of the plates only
3. The coefficient of viscosity of the plates only
4. All of these

Answer: 4. All of these

The viscous force exerted by a liquid flowing between two horizontal plates is given by

⇒ \(F=-\eta A \frac{d v}{d z}\)

which depends on all the three factors given in options (1), (2), and (3).

Question 107. Under a constant-pressure head, the volume flowing per unit time through a tube is V. If the length of the tube is doubled and the diameter of the bore is halved, the rate of flow would become

1. \(\frac{V}{4}\)
2. 16V
3. \(\frac{V}{8}\)
4. \(\frac{V}{32}\)

Answer: 4. \(\frac{V}{32}\)

The rate of flow is given by

⇒ \(V=\frac{\pi P r^4}{8 \eta l}\)

Hence, the new rate of flow will be

⇒ \(V^{\prime}=\frac{\pi P}{8 \eta} \cdot \frac{\left(\frac{r}{2}\right)^4}{2 l}\)

= \(\frac{\pi P r^4}{8 \eta l} \cdot \frac{1}{32}\)

= \(\frac{V}{32}\)

Question 108. A spherical ball is dropped in a p-long column of liquid. Which of the following three plots (P, Q, and R) represents the variation of gravitational force with time, that of viscous force with time, and that of the net force acting on the ball with time respectively?

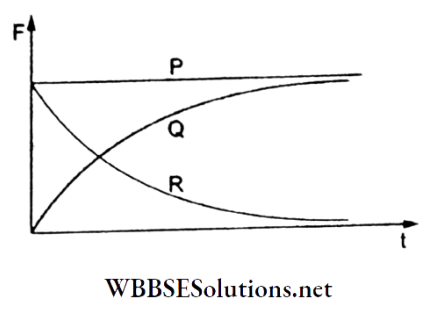
1. Q, R, and P
2. R, Q, and P
3. P, Q, and R
4. R, P, and Q

Answer: 3. P, Q, and R

The gravitational force (= weight = mg) remains constant (given by the plot P). The viscous force gradually increases with time and becomes constant when the terminal velocity is reached (represented by the plot Q). The net force gradually decreases and has a zero value when the motion becomes uniform (shown by plot R).

Question 109. A lead shot of 1 mm diameter falls through a long column of glycerine. The variation of its velocity (v) with the distance covered (s) is represented by

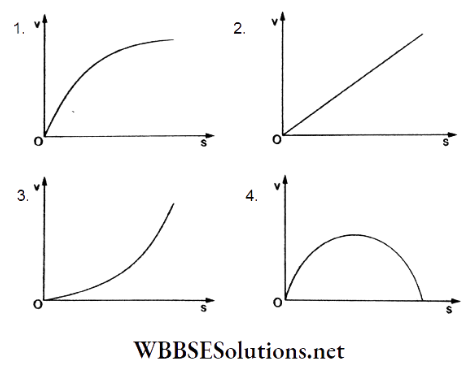
Answer: 1.

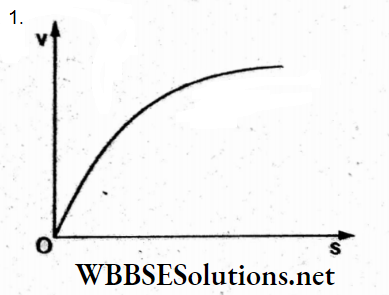
Initially, the velocity increases due to gravity. Simultaneously, a viscous force acts, so the lead shot attains a constant terminal velocity, as shown in the graph in option (1).

Question 110. A sphere of mass M and radius R is falling in a viscous fluid. The terminal velocity attained by the falling object will be proportional to

1. R²
2. R
3. \(\frac{1}{R}\)
4. \(\frac{1}{R^2}\)

Answer: 1. R²

The terminal velocity of a spherical body falling under gravity in a viscous fluid is given by

⇒ \(v=\frac{2}{9} \cdot \frac{\rho-\sigma}{\eta} \cdot R^2\)

⇒ \(v \propto R^2\)

Question 111. A raindrop reaching the ground with a terminal velocity has a momentum of p. Another drop of twice its radius, also reaching the ground with a terminal velocity, will have a momentum of

1. 4p
2. 16p
3. 8p
4. 32p

Answer: 4. 32p

The terminal velocity is given by v ∝ r², and the mass is given by

⇒ \(m=\frac{4}{3} \pi r^3 \rho \propto r^3\)

Hence, the momentum is given by p ∝ r³.

For the smaller drop, p = kr⁵.

For a larger drop, p’ = k(2r) = 32kr⁵.

∴ \(\frac{p^{\prime}}{p}=32\)

p’ = 32p

Question 112. A cylindrical drum, open at the top, contains 30 liters of water. It drains out through a small hole at the bottom. 10 liters of water comes out in a time of the next 10 liters in a further time t₂ and the last 10 liters in a further time t₃. Then,

1. t₁ = t₂ = t₃
2. t₁>t₂<t₃
3. t₁<t₂<t₃
4. \(\frac{1}{t_1}+\frac{1}{t_2}=\frac{1}{t_3}\)

Answer: 3. t₁<t₂<t₃

The velocity of efflux is given by \(v=\sqrt{2 g x}\), where x is the height of the liquid. As the water drains out, x reduces, so v reduces. This reduces the rate of drainage. Hence, it requires a longer time to drain out the same volume of water. Thus,

t₁<t₂<t₃

Question 113. A solid sphere of radius R acquires a terminal velocity while falling under gravity through a viscous fluid having the coefficient of viscosity r₁. The sphere is broken into 27 identical solid spheres. If each of these small spheres acquires a terminal velocity of v₂ while falling through the same fluid, the ratio v₁/v₁ equals

1. 9
2. \(
3. 27
4. [latex]\frac{1}{9}\)

Answer: 1. 9

The terminal velocity of a sphere falling through a viscous medium under gravity is given by

⇒ \(v=\frac{2}{9} \cdot \frac{r^2(\rho-\sigma) g}{\eta}\)

Thus, v₁ = kr₂….(1)

When the drop is split up into 27 droplets of equal radii r0 then,

⇒ \(\frac{4}{3} \pi r^3=27 \cdot \frac{4}{3} \pi r_0^3\)

Hence, r₀ = \(\frac{r}{3}\) and its terminal velocity is

⇒ \(v_2=k r_0^2=k\left(\frac{r}{3}\right)^2\)…..(2)

∴ \(\frac{v_1}{v_2}=\frac{r^2}{\left(\frac{r}{3}\right)^2}=9\)

Question 114. A small hole of area of cross-section 2 mm² is punched near the bottom of a fully filled open tank of height 2 m. Taking =10 m s^-2, the rate of flow of water through the open hole would be nearly

1. 12.6 x 10^-6 m³ s^-1
2. 8.9 x 10^-6 m³ s^-1
3. 2.23 x 10^-6 m³s^-1
4. 6.4 x 10^-6 m³ s^-1

Answer: 1. 12.6 x 10^-6 m³ s^-1

According to Torricelli’s law, the efflux speed of a liquid at a depth of h is \(v=\sqrt{2 g h}\) and thus the volume flowing per unit time is

V = (area) (velocity)

= A\(v=\sqrt{2 g h}\)

= \(\left(2 \times 10^{-6} \mathrm{~m}^2\right) \sqrt{2\left(10 \mathrm{~m} \mathrm{~s}^{-2}\right)(2 \mathrm{~m})}\)

= \(=4 \times 10^{-6} \times \sqrt{10} \mathrm{~m}^3 \mathrm{~s}^{-1}\)

= 12.6 x 10^-6 m³ s^-1.

Question 115. Water from a pipe is coming at a rate of 100 litres per minute. If the radius of the pipe is 5 cm, the Reynolds number for the flow is of the order of (given that density of water = 1000 kg m^-3 and coefficient of viscosity of water =1 mPa s)

1. 10⁶
2. 10³
3. 10⁴
4. 10²

Answer: 3. 10⁴

The volume flowing per unit time is given by V = (area ofcross section)(velocity) = Av.

Since critical velocity = \(v_{\mathrm{c}}=\frac{k \eta}{\rho R}\)

∴ \(\frac{V}{A}=\frac{k \eta}{\rho R}\)

Hence, Reynolds number = k\(\frac{V \rho R}{A \eta}\) ,

Substituting the proper radius, we obtain

⇒ \(k=\frac{\left(100 \mathrm{~L} \mathrm{~min}^{-1}\right)\left(10^3 \mathrm{~kg} \mathrm{~m}^{-3}\right)}{\pi\left(5 \times 10^{-2} \mathrm{~m}\right)\left(10^{-3} \mathrm{~Pa} \mathrm{~s}\right)} \approx \frac{\left(\frac{100 \times 10^{-3}}{60}\right)\left(10^3\right)}{(3.14)\left(5 \times 10^{-5}\right)}\)

⇒ \(\frac{10^7}{3.14 \times 300} \approx 10^4\)

Question 116. Water flows into a large tank with a flat bottom at the rate of 10^-4 m³ s^-1 Water is also leaking out of a hole of area 1 cm² at its bottom. If the height of the water in the tank remains steady then this height is close to

1. 4 cm
2. 3 cm
3. 5 cm
4. 1.7 cm

Answer: 3. 5 cm

The water level will remain steady if in flow rate = outflow rate.

Hence, V = Av = A\(\sqrt{2 g h}\)

⇒ \(h=\frac{V^2}{2 A^2 g}=\frac{\left(10^{-4} \mathrm{~m}^3 \mathrm{~s}^{-1}\right)^2}{2\left(10^{-4} \mathrm{~m}^2\right)^2\left(10 \mathrm{~m} \mathrm{~s}^{-2}\right)}\)

⇒ \(\frac{10^{-8}}{2 \times 10^{-7}} \mathrm{~m}\)

= \(\frac{1}{20}\)

= 5 cm.

Question 117. A spherical ball of radius r and density p is released from a height h above the surface of a viscous liquid. If the terminal velocity gained by the ball in die liquid is the same as that before entering the liquid then h is proportional to

1. r⁴
2. r³
3. r²
4. r

Answer: 1. r⁴

The velocity of the ball just before entering the liquid is v = \(\sqrt{2 g h}\)

Its terminal velocity in the liquid will be

⇒ \(v_{\mathrm{t}}=\frac{2}{9} \cdot \frac{r^2(\rho-\sigma) g}{\eta}=k r^2\)

∴ \(\sqrt{2 g h}=k r^2\)

⇒ \(h=\frac{k^2 r^4}{2 g} \propto r^4\)

Fluid Mechanics

Question 1. Two nonmixing liquids of densities p and np (where n > 1) are put in a container. The height of each liquid is h. A solid cylinder of length L and density d is put in this container. The cylinder floats keeping its axis vertical so that a length of pL (where p < 1) is in the denser liquid. The density d is equal to

1. [2 + (n + 1)p]p
2. [2 + (n- 1)p]p
3. [1 + (n-1)p]p
4. [1 + (n + 1)p]p

Answer: 3. [1 + (n-1)p]p

Let A be the cross-sectional area of the cylindrical block. weight of the cylinder = ALdg (downward),

buoyant force due to the lower part of the liquid = ApLnpg (upward),

and that due to the upper part of the liquid =A(1 -p)Lpg (upward).

For equilibrium, when the cylinder floats,

ALdg= ApLnpg + A(1 -p)Lpg

=> d = pnp + (1-p)p = [1 + (n-1)p]p.

Question 2. A candle of diameter d is floating in a liquid kept in a cylindrical container of diameter D (where D = d), as shown in the figure. It is burning at the rate of 2 cm h^-1. Then, the top of the candle will

1. Remain at the same height
2. Fall at the rate of 1 cm h^-1
3. Fall at the rate of 2 cm h^-1
4. Go up at the rate of 1 cm h^-1

Answer: 2. Fall at the rate of 1 cm h^-1

Let p_c and p_liq be the densities of the candle and liquid respectively. For the candle to float initially, weigh to f the candle- buoyant force

A.2L.p_cg = ALp_liqg

p_liq = 2p_c….(i)

Thus, the candle’s length is equally distributed below and above foe liquid surface.

If 2 an of the candle is burnt out, for length above for liquid level will be

⇒ \(\frac{2 L-2 \mathrm{~cm}}{2}=L-1 \mathrm{~cm}\)

which is reduced by 1 cm.

So, for top footcandle will fall at a rate of 1 cm h^-1

Question 3. By sucking through a straw a person can reduce the pressure in his lungs to 750 mmHg (density of mercury being 13.6 g cm^-3). Using the straw, he can drink water from a glass up to a maximum depth of

1. 10 cm
2. 13.6 cm
3. 75 cm
4. 1.36 cm

Answer: 2. 13.6 cm

Standard atmospheric pressure = 760 mmHg.

The pressure in the lungs is reduced to 750 mmHg.

∴ pressure difference =10 mmHg.

Fewfoesame pressure difference, let ft = height of the water column.

∴ h (1 g cm^-33)g = (10 mm) (13.6 g cm^-3)g

= 136 mm Hg

= 13.6 cmHg.

Hence, for required depth is 13.6 cm

Question 4. A solid sphere of volume V and density p floats at the interface of two immiscible liquids of densities p₁, and p₂ respectively. If p₁ < p < p₂ then the ratio of the volume of the parts of the sphere in the upper and lower liquids is

1. \(\frac{\rho-\rho_1}{\rho_2-\rho}\)
2. \(\frac{\rho_2-\rho}{\rho-\rho_1}\)
3. \(\frac{\rho+\rho_1}{\rho+\rho_2}\)
4. \(\frac{\rho+\rho_2}{\rho+\rho_1}\)

Answer: 2. \(\frac{\rho_2-\rho}{\rho-\rho_1}\)

Let V₁ be the volume of the sphere immersed in the liquid of density p₁ and V₂ be the volume immersed in the liquid of density p₂.

Total weight of the sphere = Mg = (V₁ + V₂)pg (downward) and buoyant force due to the liquids displaced

= V₁p₁g + V₂p₂g

= (V₁ P₁ + V₂ P₂)g (upward)

For equilibrium,

(V₁ + V₂)pg = (V₁p₁ + V₂p₂)g

⇒  V₁(p-p₁) = V₂(p₂-p)

⇒ \(\frac{V_1}{V_2}=\frac{\rho_2-\rho}{\rho-\rho_1}\)

Question 5. If water is used to construct a barometer, what would be the approximate height of the water column in the tube at the standard atmospheric pressure (76 cm Hg)?

1. 2m
2. 4 m
3. 6 m
4. 10 m

Answer: 4. 10 m

For the same pressure,

⇒ \(h_{\mathrm{w}} \rho_{\mathrm{w}} g=h_{\mathrm{Hg}} \rho_{\mathrm{Hg}} g\)

⇒ \(h_w=\frac{h_{\mathrm{Hg}} \rho_{\mathrm{Hg}}}{\rho_{\mathrm{w}}}\)

⇒ \(\frac{(76 \mathrm{~cm})\left(13.6 \times 10^3 \mathrm{~kg} \mathrm{~m}^{-3}\right)}{1 \times 10^3 \mathrm{~kg} \mathrm{~m}^{-3}}\)

= 1033.6 cm ≈ 10 m.

Question 6. A cube of ice floats partly in water and partly in an oil l as shown in the figure. If the relative densities of the oil, ice, and water are 0.8, 0.9, and 1.0 then the ratio of the volumes of ice immersed in water to that in the oil will be

1. 8:9
2. 9:8
3. 1:1
4. None of these

Answer: 3. 1:1

From the law of floatation, the weight of the ice block = the weight of the liquids displaced.

Now, the weight of the ice block = Vp_iceg = (V_w + V_o) p_iceg,

the weight of the displaced water = V_wp_wg,

and the weight of the displaced oil = V_op_og.

For equilibrium,

⇒ \(\left(V_{\mathrm{w}}+V_{\mathrm{o}}\right) \cdot \rho_{\mathrm{ice}}=V_{\mathrm{w}} \rho_{\mathrm{w}}+V_{\mathrm{o}} \rho_{\mathrm{o}}\)

V_w (P_ice – P_w) = V_o (p_o – P_ice)

⇒ \(\frac{V_w}{V_0}=\frac{\rho_0-\rho_{\text {ice }}}{\rho_{\text {ice }}-\rho_w}\)

= \(\frac{0.8-0.9}{0.9-1.0}=\frac{-0.1}{-0.1}=1\)

=> V_w:V_o =1:1.

Question 7. A thick spherical shell of inner and outer radii R and 2R respectively, floats half-submerged in a liquid of density p0. The density of the material of the spherical shell is

1. \(\frac{\rho_0}{2}\)
2. p₀
3. \(\frac{4}{3} \rho_0\)
4. \(\frac{4}{7} \rho_0\)

Answer: 4. \(\frac{4}{7} \rho_0\)

The volume of the material of the shell is

⇒ \(\frac{4}{3} \pi\left[(2 R)^3-R^3\right]=\frac{4}{3} \pi\left(7 R^3\right)\)

∴ its mass is \(M=\frac{4}{3} \pi\left(7 R^3\right) p\)

∴ the buoyant force (due to the displaced liquid) is

⇒ \(\frac{1}{2}\left[\frac{4}{3} \pi(2 R)^3\right] \rho_0 g\)

For equilibrium,

⇒ \(\frac{4}{3} \pi\left(7 R^3\right) \rho g=\frac{1}{2} \cdot \frac{4}{3} \pi\left(8 R^3\right) \rho_0 \cdot g\)

⇒ \(\rho=\frac{4}{7} \rho_0 .\)

Question 8. A U-tube containing a liquid is accelerated horizontally with a constant acceleration a0. If the separation between the vertical arms is l then the difference in the heights of the liquid columns in the two arms will be

1. \(\frac{l g}{a_0}\)
2. \(\frac{l a_0}{g}\)
3. \(l\left(1+\frac{a_0}{g}\right)\)
4. \(i\left(1-\frac{a_0}{g}\right)\)

Answer: 2. \(\frac{l a_0}{g}\)

Let A be the area of the cross-section of the tube t and p be the density of the liquid. Consider section AB of the tube.

Mass of the liquid in AB = volume x density = Alp.

The pressures at A and B due to the liquid column are h₂pg and h₁pg respectively.

∴ the net force on the liquid in AB to the right is (h₂– h₁) pgA.

(h₂-h₁)pgA = Ma₀ = Alpa₀.

Hence, the difference in height is

(h₂– h₁) = la₀/g

Question 9. A beaker containing a liquid of density p moves vertically upwards with a uniform acceleration a. The hydrostatic pressure due to the liquid column at a depth h below the free liquid surface is

1. hpg
2. hp (g – a)
3. hp (g + a)
4. \(h \rho g\left(\frac{g-a}{g+a}\right)\)

Answer: 3. hp (g + a)

Consider the forces acting on an element of the liquid of cross-section A and height h. The force on the upper section is F₁ = P₀A and that on the lower section is F₂ = (p₀ + p)A, where p = pressure due to the liquid column.

∵ weight = mg = Vpg = Ahpg,

∴ upward net force =F₂– F₁– mg = ma.

∴ (p₀+p)A – p₀A – Ahpg = Ahpa

Hence,p = hp(g + a)

Question 10. The area of the cross-section of two arms of a hydraulic press is A and nA (where n >1) respectively, as shown in the figure. A force F is applied to the liquid in the thinner arm. In order to maintain the equilibrium of the liquid, the force F’ to be applied on the liquid in the thicker arm is

1. \(\frac{F}{n}\)
2. nF
3. \(\frac{F}{n+1}\)
4. (n + 1)F

Answer: 2. nF

In equilibrium, the pressures at the two surfaces are equal as they lie in the same horizontal plane.

∴ \(p_{\mathrm{atm}}+\frac{F}{A}=p_{\mathrm{atm}}+\frac{F^{\prime}}{n A}\)

⇒ \(F=\frac{F^{\prime}}{n}\)

⇒ F’=nF

Question 11. A wind blows parallel to the roof of a house at a speed of 40 m s^-1. The area of the roof is 250 m². Assuming the pressure inside the house to die standard atmospheric pressure, the force exerted by the wind on the roof and the direction of the force will be (given that pair = 1.2 kg m³)

1. 4,8 x 10⁵ N (downward)
2. 4.8 x 10⁵ N (upward)
3. 2.4 x 10⁵ N (upward)
4. 2.4 x 10⁵ N (downward)

Answer: 3. 2.4 x 10⁵ N (upward)

According to Bernoulli’s theorem,

⇒ \(p_1+\frac{1}{2} \rho v_1^2+h \rho g=p_2+\frac{1}{2} \rho v_2^2+h \rho g\)

So, the pressure difference inside and outside the roof is

AP = P₂ ~ P₁

⇒ \(\frac{1}{2} \rho\left(v_2^2-v_1^2\right)\)

Substituting the values,

⇒ \(\Delta p=\frac{1}{2}\left(1.2 \mathrm{~kg} \mathrm{~m}^{-3}\right)\left[\left(40 \mathrm{~m} \mathrm{~s}^{-1}\right)^2-0\right]\)

= 960 N m^-2.

∴ the force acting on the roof upwards is

ΔpA = (960 N m^-2) (250 m²)

= 240000 N

= 2.4 X 10⁵N.

Question 12. The cylindrical tube of a spray pump has a radius of R. One end of the pump has n fine holes, each of radius r. If the speed of the liquid in the tube is v, the speed with which the liquid is ejected through the hole is

1. \(\frac{v R^2}{n^2 r^2}\)
2. \(\frac{v R^2}{n r^2}\)
3. \(\frac{v R^2}{n^3 r^2}\)
4. \(\frac{v^2 R}{n r}\)

Answer: 2. \(\frac{v R^2}{n r^2}\)

According to the equation of continuity,

A₁v₁ = A₂v₂

⇒ πR²v = n(πr²)v’

⇒ \(v^{\prime}=\frac{v R^2}{n r^2}\)

Question 13. Bernoulli’s theorem is a consequence of the conservation of

1. Energy
2. Linear momentum
3. Angular momentum
4. Mass

Answer: 1. \(\frac{v R^2}{n^2 r^2}\)

Bernoulli’s theorem is a consequence of the conservation of energy in fluids, which have their KE, PE, and pressure energy

Question 14. In old age, arteries carrying blood in the human body become narrow resulting in an increase in blood pressure. This follows from

1. Stokes’ law
2. Archimedes’ principle
3. Pascal’s law
4. Bernoulli’s principle

Answer: 4. Bernoulli’s principle

According to the equation of continuity,

av = constant.

So, an increase in area (a₂ >a₁ decreases the velocity (v₂ < v₁)

From Bernoulli’s theorem,

⇒ \(p_1+\frac{1}{2} \rho v_1^2=p_2+\frac{1}{2} \rho v_2^2\)

Hence, increase in pressure = \(p_2-p_1=\frac{1}{2} \rho\left(v_1^2-v_2^2\right)\)

Question 15. A liquid kept in a cylindrical vessel is rotated about a vertical axis through the centre of the circular base. If the radius of the vessel is r and the angular velocity of rotation is co, the difference in the heights of the liquid at the centre of the vessel and the edge is

1. \(\frac{r \omega}{2 g}\)
2. \(\frac{r^2 \omega^2}{2 g}\)
3. \(\sqrt{2 g \omega r}\)
4. \(\frac{\omega^2}{2 g r^2}\)

Answer: 2. \(\frac{r^2 \omega^2}{2 g}\)

At the point P, let

So, N cos θ = mg and N sinθ = \(\frac{m v^2}{x}\)

⇒ \(\tan \theta=\frac{v^2}{x g}=\frac{d y}{d x}\)

Integrating,

⇒ \(\int_0^h d y=\int_0^r \frac{\omega^2 x}{g} d x\) [∵ v = xwww]

⇒ \(h=\frac{r^2 \omega^2}{2 g}\)

Alternative method:

According to Bernoulli’s theorem,

⇒ \(p+\frac{1}{2} \rho v^2\) = constant.

At the sides, the speed (v = m) is large, so the pressure is low. However, the pressure at a given horizontal level in a liquid is constant. Hence, the liquid rises such that

⇒ \(\frac{1}{2} \rho v^2=\rho g h \text { and } h=\frac{r^2 \omega^2}{2 g}\)

Question 16. The velocity of water flowing through a cylindrical tube of diameter Semis 4 m s^-1. It is connected to a pipe with an end tip of a diameter of 2 cm. The velocity of water at the free end is

1. 4 ms^-1
2. 8 ms^-1
3. 32 ms^-1
4. 64 ms^-1

Answer: 4. 64 ms^-1

From the equation of continuity,

A₁V₁ = A₂V₂

⇒ \(\pi R_1^2 v_1=\pi R_2^2 v_2\)

⇒ \(v_2=v_1\left(\frac{R_1}{R_2}\right)^2\)

= \(\left(4 \mathrm{~m} \mathrm{~s}^{-1}\right)\left(\frac{8 \mathrm{~cm}}{2 \mathrm{~cm}}\right)^2\)

= \(64 \mathrm{~m} \mathrm{~s}^{-1}\)

Question 17. The pressure head in the Bernoulli equation is

1. \(\frac{p p}{g}\)
2. \(\frac{p}{pg}\)
3. ppg
4. Pg

Answer: 2. \(\frac{p}{pg}\)[/latex]

From Bernoulli’s theorem,

⇒ \(\frac{1}{2} \rho v^2+\rho g h+p=\text { constant }\)

⇒ \(\frac{v^2}{2 g}+h+\frac{p}{\rho g}=\text { constant. }\)

Hence, the pressure head is \(\frac{p}{p g}\)

Question 18. Water flows through a horizontal tube with a constriction at B, as shown in the figure. The pressure difference between the regions A and B is 600 Pa, while the cross-sectional areas are in the ratio 2:1. The speed of flow (v_A) in the region A is

1. 2ms^-1
2. √2 ms^-1
3. 4 ms^-1
4. √0.4 ms^-1

Answer: 4. √0.4 ms^-1

From the equation of continuity,

v_Aa_A = v_Ba_B

⇒ \(v_{\mathrm{B}}=v_{\mathrm{A}} \cdot \frac{a_{\mathrm{A}}}{a_{\mathrm{B}}}=v_{\mathrm{A}} \cdot \frac{2}{1}=2 v_{\mathrm{A}}\)

From Bernoulli’s theorem,

⇒ \(p_{\mathrm{A}}+\frac{1}{2} \rho v_{\mathrm{A}}^2=p_{\mathrm{B}}+\frac{1}{2} \rho v_{\mathrm{B}}^2\)

⇒ \(p_{\mathrm{A}}-p_{\mathrm{B}}=\frac{1}{2} \rho\left(v_{\mathrm{B}}^2-v_{\mathrm{A}}^2\right)\)

⇒ \(\frac{1}{2} \rho\left(4 v_{\mathrm{A}}^2-v_{\mathrm{A}}^2\right)=\frac{3}{2} \rho v_{\mathrm{A}}^2\)

⇒ \(v_{\mathrm{A}}=\sqrt{\frac{2}{3 \rho}\left(p_{\mathrm{A}}-p_{\mathrm{B}}\right)}\)

= \(\sqrt{\frac{2}{3} \cdot \frac{600 \mathrm{~N} \mathrm{~m}^{-2}}{10^3 \mathrm{~kg} \mathrm{~m}^{-3}}}\)

= \(\sqrt{0.4} \mathrm{~m} \mathrm{~s}^{-1}\)

Question 19. Water and mercury are filled in two cylindrical vessels up to the same height. Both vessels have a hole in the wall near the bottom. The velocity of water and mercury coming out of the holes are v₁ and v₂ respectively, then

1. v₁ = 13.6v₂
2. \(v_1=\frac{v_2}{13.6}\)
3. v₁ = v₂
4. \(v_1=\sqrt{13.6} v_2\)

Answer: 3. v₁ = v₂

Efflux speed \(v=\sqrt{2 g h}\), which is independent of the density of the liquid. So, for the same height h, the efflux speed will always be the same.

Question 20. There is a small hole near the bottom of an open tank filled with a liquid. The speed of the liquid ejected out through the hole does not depend on the

1. Area of the hole
2. Density of the liquid
3. Acceleration due to gravity
4. Height of the liquid column from the hole

Answer: 1. Area of the hole

Efflux speed \(v=\sqrt{2 g h}\), which is independent of the area of the hole.

Question 21. The efflux speed of the liquid coming out of a small hole of a large vessel containing two different liquids of densities 2p and p (as shown 2h in the figure) will be

1. \(\sqrt{g h}\)
2. \(2 \sqrt{g h}\)
3. \(\sqrt{8 g h}\)
4. \(\sqrt{6 g h}\)

Answer: 2. \(2 \sqrt{g h}\)

A liquid column of height 2h with a density of p is equivalent to a liquid column of height h with a density of 2p, as they produce the same pressure.- Hence, the equivalent height H of the top surface from the hole is

H = h + h = 2h,

and the efflux speed is \(v=\sqrt{2 g(2 h)}=2 \sqrt{g h}\)

Question 22. A block of silver of mass 4 kg hanging from a string is immersed in a liquid of relative density 0.72. If the relative density of silver is 10, what will be the tension in the string?

1. 73 N
2. 21 N
3. 42.5 N
4. 37.2 N

Answer: 4. 37.2 N

Tension in the string = true weight- upthrust

⇒ \((4 \mathrm{~kg})\left(10 \mathrm{~m} \mathrm{~s}^{-2}\right)-\frac{(4 \mathrm{~kg})\left(0.72 \times 10^3 \mathrm{~kg} \mathrm{~m}^{-3}\right)\left(10 \mathrm{~m} \mathrm{~s}^{-2}\right)}{10 \times 10^3 \mathrm{~kg} \mathrm{~m}^{-3}}\)

= 40 N – 2.8 N

= 37.2 N.

Question 23. An incompressible fluid of density p is filled in two identical containers of base area A. On opening the l valve V connecting the containers, the levels become equal. The loss in the gravitational potential energy during the process is

1. \(\frac{A p g}{4}\left(x_1-x_2\right)^2\)
2. \(\frac{A \rho g}{4}\left(x_1+x_2\right)^2\)
3. \(\frac{A \rho g}{4}\left(x_1^2-x_2^2\right)\)
4. \(\frac{A \rho g}{4}\left(x_1^2+x_2^2\right)\)

Answer: 1. \(\frac{A p g}{4}\left(x_1-x_2\right)^2\)

The initial potential energy of the system is

U_i = m₁gh₁ + m₂gh₂

⇒ \(\left(\rho A x_1, \frac{x_1}{2}+\rho A x_2 \cdot \frac{x_2}{2}\right) g\)

⇒ \(\left(x_1^2+x_2^2\right)\left(\frac{A p g}{2}\right)\)

When the connecting valve is opened, the fluid attains the same height \(x=\frac{\left(x_1+x_2\right)}{2}\) in both containers.

Hence, the final potential energy is

⇒ \(U_f=2\left(\frac{x_1+x_2}{2}\right)^2\left(\frac{A p g}{2}\right)\)

⇒ \(\left(x_1+x_2\right)^2\left(\frac{A \rho g}{4}\right)\)

∴ loss in \(\mathrm{PE}=U_1-U_f=\frac{A \rho g}{2}\left[x_1^2+x_2^2-\frac{\left(x_1+x_2\right)^2}{2}\right]\)

⇒ \(\frac{A \rho g}{4}\left(x-x_2\right)^2\)

Question 24. A shell of relative density 27/9 relative to water is just submerged in water. If its inner and outer radii are r and R respectively, r/R is equal to

1. \(\left(\frac{1}{3}\right)^{\frac{1}{3}}\)
2. \(\left(\frac{2}{3}\right)^{\frac{1}{3}}\)
3. \(\left(\frac{3}{4}\right)^{\frac{1}{3}}\)
4. \(\left(\frac{5}{9}\right)^{\frac{1}{3}}\)

Answer: 2. \(\left(\frac{2}{3}\right)^{\frac{1}{3}}\)

For equilibrium,

mass of shell = buoyant force

⇒ \(\frac{4}{3} \pi\left(R^3-r^3\right) \rho_{\mathrm{sh}} g=\frac{4}{3} \pi R^3 \rho_{\mathrm{w}} g\)

⇒ \(\left(R^3-r^3\right) \cdot 3=R^3 \Rightarrow 1-\left(\frac{r}{R}\right)^3=\frac{1}{3}\)

⇒ \(\left(\frac{r}{R}\right)^3=\frac{2}{3} \Rightarrow \frac{r}{R}=\left(\frac{2}{3}\right)^{\frac{1}{3}}\)

Heat And Thermodynamics Synopsis

• Heat is a form of energy that exists in matter due to the constant random motion of molecules. Its SI unit is the joule (symbol: J) and cgs unit is the calorie (symbol: cal).1 cal = 4.2 J and 1 kcal = 4200 J.
• The temperature of a body indicates its thermal state and determines the direction of the flow of heat Heat always flows from a higher to a lower temperature.
• The absolute zero, or OK, corresponds to a temperature of -273.15 degrees on the Celsius temperature scale. The lower and upper fixed points in the Celsius scale correspond to 273.15 K and 373.15 K respectively.
• The triple point for pure water is 0.01°C (273.16 K) at 611 Pa, and is used to calibrate thermometers.
• Ideal gas equation of state: pV= nRT, where p = pressure (in pascals or newtons per meter squared), V = volume (in m³), n = amount of substance (in moles), and R = gas constant ≈ 8.3 J K^-1 mole^-1.
• van der Waals equation of state for real gases:
  \(\left(p+\frac{a}{V^2}\right)\) (V – b) = nRT, where a and b are constants.
• Thermal expansion:
  • For length, L_2θ = L_v(1 + αθ).
  • For area, A_θ = A₀(1 + βθ).
  • For volume, V_θ = V₀(1 + γθ).
  • 6α = 3β = 2γ.
• Molar mass M = wNA, where m = mass of each molecule and NA = Avogadro constant ≈ 6.022 x 10²³ mol^-1.
• Boltzmann constant,
  \(k=\frac{R}{N_{\mathrm{A}}} \approx \frac{8.3 \mathrm{~J} \mathrm{~K}^{-1} \mathrm{~mol}^{-1}}{6.022 \times 10^{23} \mathrm{~mol}^{-1}} \approx 1.38 \times 10^{-23} \mathrm{~J} \mathrm{~K}^{-1}\)
• Gaseous pressure,
  \(p=\frac{1}{3}\left(\frac{M}{V}\right) c_{\mathrm{rms}}^2=\frac{1}{3} \rho c_{\mathrm{rms}}^2\).
  
• In this equation:
  • RMS speed, \(c_{\mathrm{rms}}=\sqrt{\frac{3 p}{\rho}}=\sqrt{\frac{3 p V}{M}}=\sqrt{\frac{3 n R T}{M}}=\sqrt{\frac{3 k T}{m}}\), where
    m = mass of each molecule;
  • mean speed, \(\bar{c}=\sqrt{\frac{8 R T}{\pi M}}=\sqrt{\frac{8 k T}{\pi m}}\)
• Most probable velocity, \(c_{\mathrm{mp}}=\sqrt{\frac{2 R T}{M}}=\sqrt{\frac{2 k T}{m}}\)
• Thus, cmp < vmean < crms.
• Heat absorbed (or expelled) Q = mcAT, where Q is in joules, specific heat capacity (unit: J kg^-1K^-1), and AT = change in temperature (in°C or K).
• Specific latent heat = L (SI unit: J kg^-1).
• During a phase change, Q = mL.
• The molar heat capacity C (SI unit: J mol^-1C^-1) of gases is process dependent and is given by the equation Q = nCAT, where n = amount of substance (in moles).
  • At a constant volume, \(C_V=\left.\frac{Q}{n \Delta T}\right|_{V=\text { constant }}\)
  • At a constant pressure, \(C_p=\left.\frac{Q}{n \Delta T}\right|_{p=\text { constant }}\)
• \(C_p-C_V=R, \frac{C_p}{C_V}=\gamma, C_p=\left(\frac{R \gamma}{\gamma-1}\right), p V^\gamma=\text { constant }\)
• Change in internal energy, AU = nC_vΔT.
• The first law of thermodynamics: ΔQ = ΔU + ΔW.
• Thermodynamic processes:

• Molar heat capacity of a mixture of gases:
  • \(\left(C_V\right)_{\text {mix }}=\frac{n_1 C_{V_1}+n_2 C_{V_2}+\ldots}{n_1+n_2+\ldots}\)
  • \(\left(C_p\right)_{{mix}}=\frac{n_1 C_{p_1}+n_2 C_{p_2}+\ldots}{n_1+n_2+\ldots}\)
  • \((\gamma)_{{mix}}=\frac{n_1 C_{p_1}+n_2 C_{p_2}+\ldots}{n_1 C_{V_1}+n_2 C_{V_2}+\ldots}\)
• Equipartition law: This law states that the average energy of a molecule in a gas associated with each degree of freedom is \(\frac{1}{2}\)kT, where k = Boltzmann constant and T = temperature (in kelvin).

Thermal Expansion and Calorimetry

Question 1. A Celsius thermometer and a Fahrenheit thermometer are dipped in boiling water. The water temperature is lowered until the Fahrenheit thermometer registers 140 °F. What is the fall in temperature as registered by the Celsius thermometer?

1. 80°C
2. 30 °C
3. 60°C
4. 40 °C

Answer: 4. 40 °C

Given that F = 140°.

Putting this value of F in the relation

⇒ \(\frac{F-32}{9}=\frac{C}{5}\), we get

⇒ \(\frac{140-32}{9}=\frac{C}{5}\)

or, \(C=60^{\circ} \mathrm{C}\)

The fall in temperature in the Celsius scale is

AC = 100°C – 60 °C

= 40°C

Question 2. On a new scale of temperature (which is linear), called the W-scale, the freezing and boiling points of water are 39 °W and 239°W respectively. What will be the temperature on the new W-scale, corresponding to a temperature of 39 °C on the Celsius scale?

1. 200°W
2. 117°W
3. 78 °W
4. 139°W

Answer: 2. 117°W

Comparing the two scales, we get

⇒ \(\frac{x-39}{239-39}=\frac{39-0}{100-0}\)

x = 117°W

Question 3. The coefficients of linear expansion of a brass rod and a steel rod are 04 and their lengths are l₁ and l₂ respectively. If (l₂– l₁) is maintained the same at all temperatures, which of the following relations holds good?

1. \(\alpha_1^2 l_2=\alpha_2{ }^2 l_1\)
2. \(\alpha_1 l_1=\alpha_2 l_2\)
3. \(\alpha_1 l_2=\alpha_2 l_1\)
4. \(\alpha_1 l_2^2=\alpha_2 l_1^2\)

Answer: 2. \(\alpha_1 l_1=\alpha_2 l_2\)

Coefficient of linear expansion \(\alpha=\frac{l_2-l_1}{l_1 \Delta T}\)

Increase in length = (l₂– l₁) = l₁αΔT.

Let the initial lengths of brass and steel be l₁ and l₂ and on increasing the temperature by AT let their lengths be l’₁ and l’₂.

So, (l’₁ – l₁)= α₁ΔT and (l₂ —l₂) = l₂α₂ΔT.

Subtracting, (l’₁ – l₂) – (l₁ – l₂) = (l₁α₁ -l₂α₂)AT.

Since the change in length (l₂– l₁) is maintained the same,

l₁α₁ – l₂α₂ = 0

α₁l₁ = α₂l₂

Question 4. The coefficient of volume expansion of glycerine is 5 x 10^-4 K^-1. The fractional change in the density of glycerine for a rise of 40°C in its temperature is

1. 0.025
2. 0.015
3. 0.010
4. 0.020

Answer: 4. 0.020

Since the mass remains constant,

V_θp_θ = V_θp₀

or, \(\frac{\rho_\theta}{\rho_0}=\frac{V_0}{V_\theta}=\frac{V_0}{V_0(1+\gamma \theta)}\)

= \((1-\gamma \theta)\)

or, \(\frac{\rho_0-\rho_\theta}{\rho_0}=\gamma \theta\)

∴ the fractional change in density is

⇒ \(\frac{\Delta \rho}{\rho}=\frac{\rho_0-\rho_\theta}{\rho_0}=\gamma \theta\)

= \(\left(5 \times 10^{-4} \mathrm{~K}^{-1}\right)(40 \mathrm{~K})\)

= 0.020.

Question 5. The density of water at 20°C is 998 kg m^-3 and at 40°C it is 992 kg m^-3. The coefficient of volume expansion of water is

1. 3 x 10^-4 °C^-1
2. 2 x 10^-4 °C^-1
3. 6 x 10^-4 °C^-1
4. 10^-4 °C ^-1

Answer: 1. 3 x 10^-4 °C^-1

Since the fractional change in the density of a liquid is

⇒ \(\frac{\Delta \rho}{\rho}=\gamma \theta \Rightarrow \frac{\rho_{20^{\circ} \mathrm{C}}-\rho_{40^{\circ} \mathrm{C}}}{\rho_{20^{\circ} \mathrm{C}}}\)

= \(\gamma\left(40^{\circ} \mathrm{C}-20^{\circ} \mathrm{C}\right)\)

\(\frac{998 \mathrm{~kg} \mathrm{~m}^{-3}-992 \mathrm{~kg} \mathrm{~m}^{-3}}{998 \mathrm{~kg} \mathrm{~m}^{-3}}\)

= \(\gamma \times 20^{\circ} \mathrm{C}\)

Hence, the coefficient of volume expansion of water is

⇒ \(\gamma=\frac{6}{20^{\circ} \mathrm{C}(998)}=\frac{3}{10(998)^{\circ} \mathrm{C}} \approx 3 \times 10^{-4}{ }^{\circ} \mathrm{C}^{-1}\)

Question 6. 4.0 g of a gas occupies 22.4 L at stp. The molar heat capacity of the gas at constant volume is 5.0 J mol^-1 K^-1. If the speed of sound in this gas at NTP is 952 m s^-1 then the molar heat capacity of that gas at constant pressure is (take R = 8.3 J K^-1 mol^-1)

1. 8.0 J K^-1 mol^-1
2. 8.5 J K^-1 mol^-1
3. 7.0 J K^-1 mol^-1
4. 7.5 J K^-1 mol^-1

Answer: 1. 8.0 J K^-1 mol^-1

Given that mass m- 4 g; volume V = 22.4 L; Cv = 5 J 10^-1 mol^-1; velocity
of sound v = 952 ms^-1.

∵ velocity of sound, v = \(v=\sqrt{\frac{\gamma p}{\rho}}=\sqrt{\frac{\gamma p V}{M}}\)

∴ \(\frac{C_p}{C_V}=\gamma=\frac{M}{p V} v^2\)

⇒ \(C_p=C_V \frac{M}{p V} v^2=\left(5 \mathrm{~J} \mathrm{~mol}^{-1} \mathrm{~K}^{-1}\right) \frac{\left(4 \times 10^{-3} \mathrm{~kg}\right)\left(952 \mathrm{~m} \mathrm{~s}^{-1}\right)^2}{\left(10^5 \mathrm{~Pa}\right)\left(\left(22.4 \times 10^{-3} \mathrm{~m}^3\right)\right.}\)

= 8.09 J mol^-1K^-1

Question 7. 1.0 g of ice at its melting point is mixed with 1.0 g of steam. At thermal equilibrium, the resultant temperature of the mixture is

1. 240°C
2. 100°C
3. 120°C
4. 200 °C

Answer: 2. 100°C

Heat required to convert 1.0 g of ice (at 0°C) to water (at 100°C) is

H = mL_ice + mC_water (100°C – θ)

= (1.0 g)(80 cal g^-1) + (1.0 g) (1 cal g^-1 °C^-1)(100°C)

= 80 cal + 100 cal

= 180 cal.

The heat released by 1.0 g of steam to convert itself into water at 100°C is 540 cal, which is greater than 180 cal. Hence, the temperature of the mixture will be100°C.

Question 8. If 10 g of ice is added to 40 g of water at 15 °C then the temperature of the mixture is (C_water = 4.2 x 10³ J kg^-1 K^-1, L_ice = 3.36 x 10s J kg^-1)

1. 10°C
2. 12°C
3. 0°C
4. 15°C

Answer: 3. 0°C

The heat required to melt 10 g of ice (at 0°C) to water (at 0°C) is

H₁ = mL_ice =(10 x 10^-3 kg)(3.36 x 10⁵ J kg^-1)

= 3360J.

Heatlostby 40 g of water to cool down from 15°C to 0°C is

H₂ = mC_water Δθ

= (40 x 10^-3 kg) (4.2 x 10³ J kg^-1 K^-1)(15 °C – 0°C)

=2520J.

Since H₂ <  H₁ heat lost by water is not sufficient to melt all the ice, the
the temperature of the mixture, containing some ice and water will be 0°C.

Question 9. A body initially at 80°C cools down to 64°C in 5 min and 52°C in 10 min. The temperature of the surroundings is

1. 16 °C
2. 36 °C
3. 40 °C
4. 26 °C

Answer: 1. 16 °C

According to Newton’s law of cooling, the rate of cooling = the difference in temperature between the body and the surroundings

⇒ \(\frac{\theta_1-\theta_2}{t}=k\left(\frac{\theta_1+\theta_2}{2}-\theta_0\right)\)

⇒ \(\frac{80^{\circ} \mathrm{C}-64^{\circ} \mathrm{C}}{5}=k\left(\frac{80+64}{2}-\theta_0\right)\)

⇒ \(\frac{16}{5}=k\left(72-\theta_0\right)\)….(1)

Similarly, \(\frac{64-52}{5}=k\left(58-\theta_0\right)\)

⇒ \(\frac{12}{5}=k\left(58-\theta_0\right)\)….(2)

Taking the ratio of (1) and (2),

⇒ \(\frac{16}{5} \times \frac{5}{12}=\frac{72-\theta_0}{58-\theta_0}\)

⇒ \(\frac{4}{3}=\frac{72-\theta_0}{58-\theta_0}\)

θ₀ =16 °C

Question 10. A beaker full of hot water is kept in a room. If it cools from 80 °C to i 75 °C in t₁ min, from 75°C to 70°C in t₂ min and from 70 °C to 65 °C in t₃ min then

t₁ = t₂ = t₃

t₁< t₂ = t₃

t₁<t₂<t₃

t₁>t₂> t₃

Answer: 3. t₁<t₂<t₃

The cooling curve (temperature 0 vs time t) is shown in the figure. The slope of this curve \(\left(\frac{d \theta}{d t}\right)\) gives the instantaneous rate of cooling, which decreases as time increases. So, for the same fall in temperature successively more time is required. Hence, t₁<t₂< t₃.

Question 11. Water cools from 70 °C to 60°C in the first 5 min and to 54°C in the next 5 min. The temperature of the surroundings is

1. 45 °C
2. 10 °C
3. 20 °C
4. 42°C

Answer: 1. 45 °C

According to the law of cooling,

⇒ \(\frac{70-60}{5}=k\left(\frac{70+60}{2}-\theta_0\right)\)

or 2 = k(65 – θ₀),…..(1)

Similarly,

⇒ \(\frac{60-54}{5}=k\left(\frac{60+54}{2}-\theta_0\right)\)

or, \(\frac{6}{5}=k\left(57-\theta_0\right)\)…..(2)

Dividing (1) by (2),

⇒ \(\frac{5}{3}=\frac{65-\theta_0}{57-\theta_0}\)

θ₀ = 45C.

Question 12. A body cools from temperature 3T to 2T in 10 min. The room temperature is T. Assuming that Newton’s law of cooling is applicable, the temperature of the body at the end of the next 10 minutes will be

1. \(\frac{7}{4}\)T
2. \(\frac{3}{2}\)T
3. \(\frac{4}{3}\)T
4. T

Answer: 2. \(\frac{3}{2}\)T

From the law of cooling, \(\frac{3 T-2 T}{10 \min }=k(1.5 \mathrm{~T})\)

\(k=\left(\frac{2}{30 \min }\right)\)

After the next 10 min, let x be the temperature of the body. So,

⇒ \(\frac{2 T-x}{10 \min }=k\left(\frac{2 T+x}{2}-T\right)=\left(\frac{2}{30 \min }\right)\left(\frac{x}{2}\right)\)

⇒ \(2 T-x=\frac{x}{3} \Rightarrow x=\frac{3 T}{2}\)

Question 13. A piece of ice falls from a height h so that it melts completely. Only one quarter of the heat produced is absorbed by the ice and all the energy of the ice gets converted into heat during its fall. The value of ft is (Lice = 3.4 X 10⁵ J kg^-1, g = 10 N kg^-1)

1. 34 km
2. 68 km
3. 136 km
4. 544 km

Answer: 3. 136 km

Loss in potential energy mgh is spent in the form of heat, i.e., H = mgh.

Since one-quarter of the heat is used in melting the ice,

⇒ \(\frac{1}{4}\)mgh = mLice.

height = \(h=\frac{4\left(L_{\text {ice }}\right)}{g}=\frac{4\left(3.4 \times 10^5 \mathrm{~J} \mathrm{~kg}^{-1}\right)}{\left(10 \mathrm{~N} \mathrm{~kg}^{-1}\right)}\)

= 136 x 10³ m

= 136 km.

Question 14. Steam at 100°C is passed into 20 g of water at10°C. When the water acquires a temperature of 80°C, the mass of the water present will be (C_water =1 cal g^-1 °C^-1, L_steam– 540 cal g )

1. 24 g
2. 31.5 g
3. 5g
4. 22.5 g

Answer: 4. 22.5 g

Let m be the mass of steam (at 100°C), which is condensed into water at a temperature of 80°C.

∴ heat lost by steam is

⇒ \(H_1=m L_{\text {steam }}+m C_{\text {water }} \Delta \theta\)

= m(540 cal g^-1) + m(1 cal g^-1 °C^-1)(100°C – 80°C)

= 560m cal g^-1

Heat gained by water to increase its temperature is

H₂ = m_water Cwater A0 = (20 g)(l cal g^-1 °C^-1)(80°C-10 °C)

= 1400 cal.

Since heat gained- heat lost, H₁ = H₂.

∴ 560m cal g^-1 = 1400 cal

m = 2.5 g.

∴ total mass of water present = 22.5 g.

Question 15. Ice cubes of mass 10 g are released at 0°C in a calorimeter (water equivalent = 55 g) at 40°C. Assuming no loss of heat to the surroundings, the temperature of water in the calorimeter becomes (Lice = 80 cal g^-1)

1. 30 °C
2. 20°C
3. 22°C
4. 15°C

Answer: 3. 22°C

Let the final temperature of the system be 0.

Heat lost by ice is

H₁ = m_iceL_ice + m_ice C_water (θ  – 0°C)

= (10 g)(80 cal g^-1) + (10 g)(1 cal g^-1 °C^-1)0

= 800 cal + 100 cal.

Heat lost by calorimeter is

H₂ = (water equivalent)(l cal g-1 °C^-1)(40 °C – θ)

= (55 g)(l cal g^-1 °C^-1)(40°C- θ).

Equating H₁ and H₂,

800 + 10θ = 55(40-θ)

0 = 21.54°C ≈ 22°C

Question 16. The amount of heat energy required to raise the temperature of 1g of helium at stp from T₁ K to T₂ K is

1. \(\frac{3}{8} N_{\mathrm{A}} k_{\mathrm{B}}\left(T_2-T_1\right)\)
2. \(\frac{3}{2} N_{\mathrm{A}} k_{\mathrm{B}}\left(T_2-T_1\right)\)
3. \(\frac{3}{4} N_{\mathrm{A}} k_{\mathrm{B}}\left(T_2-T_1\right)\)
4. \(\frac{3}{4} N_{\mathrm{A}} k_{\mathrm{B}} \frac{T_2}{T_1}\)

Answer: 1. \(\frac{3}{8} N_{\mathrm{A}} k_{\mathrm{B}}\left(T_2-T_1\right)\)

Helium is monatomic and has three degrees of freedom for translational motion.

Further,

⇒ \(1 \mathrm{~g} \text { of } \mathrm{He}=\frac{1}{4} \mathrm{~mol}\)

⇒ \(U_{\mathrm{i}}=\left(\frac{1}{2} k_{\mathrm{B}} T_1\right) \frac{3}{4} N_{\mathrm{A}} \text { and } U_{\mathrm{f}}\)

= \(\left(\frac{1}{2} k_{\mathrm{B}} T_2\right) \frac{3}{4} N_{\mathrm{A}}\)

energy required = \(\Delta U=U_{\mathrm{f}}-U_{\mathrm{i}}\)

= \(\frac{3}{8} N_{\mathrm{A}} k_{\mathrm{B}}\left(T_2-T_1\right)\)

Question 17. At 10°C, the value of the density of a fixed mass of an ideal gas divided by its pressure is x. At 110°C this ratio is

1. x
2. \(\frac{283}{383} x\)
3. \(\frac{10}{110} x\)
4. \(\frac{383}{283} x\)

Answer: 2. \(\frac{283}{383} x\)

From the gas equation,

pV = nRT

or, pV = \(\frac{m}{M}\)RT, where m = mass ofgas and M = molar mass

or, \(p\left(\frac{V}{m}\right)=\left(\frac{R}{M}\right) T\)

or, \(\frac{p}{p}\) = constant x T.

∴ \(\frac{\rho}{p} \propto \frac{1}{T}\)

In the given situations,

⇒ \(\frac{\rho_1 / p_1}{\rho_2 / p_2}=\frac{T_2}{T_1}\)

or, \(\frac{x}{\rho_2 / p_2}=\frac{110+273}{10+273}=\frac{383}{283}\)

∴ required ratio, \(\frac{\rho_2}{p_2}=\frac{283}{383} x\)

Question 18. The equation of state for 5g of oxygen at a pressure p and temperature T, when occupying a volume V, will be

1. pV = \(\frac{5}{32}\) RT
2. pV = 5 RT
3. pV = \(\frac{5}{2}\) RT
4. pV = \(\frac{5}{16}\) RT

Answer: 1. pV = \(\frac{5}{32}\) RT

For oxygen, molar mass = M = 32 g.

∴ number of moles in5 g is \(n=\frac{m}{M}=\frac{5}{32}\)

For gas equation,

pV=nRT

or PV = \(\frac{5}{32}\)

Question 19. A thermometer graduated according to a linear scale reads a value x₀ when in contact with boiling water and x₀/3 when in contact with ice. What is the temperature of an object in °C if this thermometer in contact with the object reads x₀/2?

1. 40
2. 60
3. 35
4. 25

Answer: 4. 25

Since the graduation is linear, we have from the given scale,

⇒ \(\frac{100^{\circ} \mathrm{C}-0^{\circ} \mathrm{C}}{\theta-0^{\circ} \mathrm{C}}=\frac{x_0-\frac{x_0}{3}}{\frac{x_0}{2}-\frac{x_0}{3}}\)

or, \(\frac{100^{\circ} \mathrm{C}}{\theta}=\frac{2}{3} \times \frac{6}{1}=4\)

0 = 25°C

fig

Question 20. Two rods A and B of identical dimensions are at a temperature 30 °C. If A is heated up to 180°C and B up to 0°C then their new lengths are found to be the same. If the ratio of the coefficients of linear expansion of A and B is 4: 3 then the value of 0 is

1. 220 °C
2. 270 °C
3. 230°C
4. 250 °C

Answer: 3. 230°C

Since the change in length is the same for both A and B,

ΔL_A = ΔL_B

or α_AL(180°C – 30°C) = α_BL(θ – 30°C)

or, \(\frac{\alpha_{\mathrm{A}}}{\alpha_{\mathrm{B}}}\left(150^{\circ} \mathrm{C}\right)=\theta-30^{\circ} \mathrm{C}\)

Hence, θ = \(\frac{4}{3}\) x 150°C + 30°C

= 230°C.

Question 21. A thermally insulated vessel contains 150 g of water at 0°C. Then, die air from the vessel is pumped out adiabatically when a fraction of water turns into ice and the rest evaporates at 0°C itself. The mass of evaporated water will be closest to (given that Lice = 3.36 x 10⁵ J kg^-1, Lwater = 2.10 x 10⁶J kg^-1)

1. 130 g
2. 35g
3. 20 g
4. 150 g

Answer: 3. 20 g

Let x = mass of water frozen twice and y = mass of water that evaporates.

Thus, x + y = 150 g……(1)

The amount of heat Q₁ released during freezing will be sufficient to evaporate water.

Thus,

xL_ice = yL_water => x(3.36 x 10⁵) = y(2.10 x 10⁶)

x = \(\frac{21.0}{3.36}\)y

= 6.25y.

Substituting in (1),

6.25y + y = 150g

y = 20.6 g

= 20g.

Question 22. When Mj g of ice at -10 °C (specific heat capacity = 0.5 cal g^-1 °C^-1) is added to M2 g of water at 50°C, finally no ice is left and the final temperature of the mixture is 0°C. The latent heat of ice (in cal g^-1) is

1. \(\frac{5 M_1}{M_2}-50\)
2. \(\frac{50 M_2}{M_1}\)
3. \(\frac{5 M_2}{M_1}-5\)
4. \(\frac{50 M_2}{M_1}-5\)

Answer: 4. \(\frac{50 M_2}{M_1}-5\)

Heat gained by ice,

Q1 = M₁C_ice(Δθ) + M₁L_ice

⇒ \(M_1\left(\frac{1}{2} {cal~g}^{-1}{ }^{\circ} \mathrm{C}^{-1}\right)\left(10^{\circ} \mathrm{C}\right)+M_1 L \mathrm{cal} \mathrm{g}^{-1}\)

= 5M₁cal g^-1 + M₁L cal g^-1

= M₁(L + 5) cal g^-1.

Heat lost by water,

Q₂ = M₂C_water Aθ

= M₂(1 cal g^-1 °C)50°C

= 50 M₂cal g^-1.

Since heat lost = heat gained,

Ml(L + 5) = 50M₂

⇒ \(L=\frac{50 M_2}{M_1}-5\)

Question 23. A rod is heated from 0°C to 10°C so that its length is changed by 0.02%. What is the percentage change in its mass density?

1. 0.02
2. 0.08
3. 0.04
4. 0.06

Answer: 4. 0.06

⇒ \(\alpha=\frac{1}{L} \frac{\Delta L}{\Delta T}\)

= \(\frac{1}{\Delta T}\left(\frac{\Delta L}{L}\right)\)

= \(\frac{1}{10^{\circ} \mathrm{C}}\left(\frac{0.02}{100}\right)\)

= \(2 \times 10^{-5}{ }^{\circ} \mathrm{C}^{-1}\)

∴ y = 3a = 6 x 10^-5 °C^-1.

Density \(=\rho=\frac{M}{V}\) Hence, the fractional change in density is,

⇒ \(\frac{\Delta \rho}{\rho}\)

= \(-\frac{\Delta V}{V}=-\gamma \Delta T\)

= \(-6 \times 10^{-5} \times 10\)

Hence, percentage change in density = 6 x 10^-4 x 100

= 6 x 10^-2

= 0.06.

Question 24. A cubical block at 273 K is compressed by an external pressure p uniformly from all directions. To bring the cube back to its original size by heating, the rise in temperature is (take α = coefficient of linear expansion, B = bulk modulus of elasticity)

1. \(\frac{p}{2 \alpha B}\)
2. \(\frac{p}{4 \alpha B}\)
3. \(\frac{p}{3 \alpha B}\)
4. \(\frac{p}{\alpha B}\)

Answer: 3. \(\frac{p}{3 \alpha B}\)

Bulk modulus is

⇒ \(B=\frac{\text { stress }}{\text { volume strain }}\)

= \(\frac{p}{\Delta V / V}\)

decrease in volume = AV = \(\frac{pV}{B}\)…..(1)

If ATbe the rise in temperature regains its original volume,

ΔV = yVΔT….(2)

Equating (1) and (2),

⇒ \(\gamma V \Delta T=\frac{p V}{B}\)

⇒ \(\Delta T=\frac{p}{\gamma B}=\frac{p}{3 \alpha B}\)

Question 25. Two rods of the same cross-section and of lengths l₁ and l₂ have coefficients of linear expansion α₁ and α₂ respectively. The equivalent coefficient of the linear expansion for their combination in series is

1. \(\frac{\alpha_1+\alpha_2}{2}\)
2. \(\sqrt{\alpha_1 \alpha_2}\)
3. \(\frac{\alpha_1 L_1+\alpha_2 L_2}{L_1+L_2}\)
4. \(\frac{\alpha_1 L_2+\alpha_2 L_1}{L_1+L_2}\)

Answer: 3. \(\frac{\alpha_1 L_1+\alpha_2 L_2}{L_1+L_2}\)

L = L₂ + L₁; ΔL = ΔL₁ + ΔL₂.

But ΔL₁ = L₁α₁ΔT and ΔL₂ = L₂α₂AT

∴ ΔL = ΔL₁ + ΔL₂

= (L₁α₁ + L₂α₂)ΔT…..(1)

For the series combination,

ΔL = (L₁ + L₂)α_eqΔT……(2)

From (1) and (2),

⇒ \(\alpha_{e q}=\frac{L_1 \alpha_1+L_2 \alpha_2}{L_1+L_2}\)

Kinetic Theory

Question 1. A gas behaves as an ideal gas at

1. High pressure and low temperature
2. Low pressure and high temperature
3. High pressure and high temperature
4. Low pressure and low temperature

Answer: 2. Low pressure and high temperature

For an ideal gas, no intermolecular force exists. Since at low pressure and high temperature, the molecules in a gas are far apart, the gas behaves like an ideal gas.

Question 2. According to the kinetic theory of gases, molecules of a gas behave like

1. Inelastic spheres
2. Perfectly elastic rigid spheres
3. Inelastic nonrigid spheres
4. Perfectly elastic nonrigid spheres

Answer: 2. Perfectly elastic rigid spheres

According to the fundamental postulates of kinetic theory, gas molecules undergo perfectly elastic collisions and thus act like perfectly elastic rigid shapers

Question 3. The graph of molar heat capacity at constant volume (Cy) for a monatomic gas is given by

Answer: 3.

For an ideal monatomic gas, the internal energy for 1 molar temperature T is given by

⇒ \(U=\left(\frac{1}{2} k T\right)(3 N)=\frac{3}{2} \frac{R}{N} T N=\frac{3}{2} R T\)

But \(C_V=\frac{d U}{d T}=\frac{3}{2} R\)

Question 4. The degree(s) of freedom for a polyatomic gas molecule is

1. < 4
2. ≥ 5
3. ≥ 6
4. >7

Answer: 3. <= 6

The motion of a polyatomic gas molecule consists of 3 degrees of freedom of translational motion, 3 degrees of freedom of rotational motion, and a number of degrees of freedom for vibrational motion.

Thus, the total number is more than 6.

Question 5. The average kinetic energy of a gas molecule at 27 °C is 6.21 x 10^-21 J. Its average KE at 227 °C will be

1. 52.2 x 10^-21 J
2. 10.35 x 10^-21 J
3. 5.22 x 10^-21 J
4. 11.35 x 101 J

Answer: 2. 10.35 x 10^-21 J

The kinetic energy of a gas molecule is given by

⇒ \(E=\frac{3}{2} k T \text { or } E \propto T\)

∴ \(\frac{E_1}{E_2}=\frac{T_1}{T_2}\)

⇒ \(\frac{6.21 \times 10^{-21} \mathrm{~J}}{E_2}\)

= \(\frac{27+273}{227+273}\)

= \(\frac{300}{500}\)

⇒ \(E_2=\frac{5}{3} \times 6.21 \times 10^{-21} \mathrm{~J}\)

= 10.35 x 10^-21 J

Question 6. A perfect gas is contained in a closed cylindrical vessel kept in f vacuum. If the cylinder suddenly bursts then the temperature of the gas

1. Is increased
2. Becomes zero K
3. Remains unchanged
4. Is decreased

Answer: 4. Is decreased

Sudden expansion or compression is an adiabatic process. In an adiabatic expansion, cooling is produced; consequently, the temperature of the gas decreases.

Question 7. An ideal gas is heated from 27°C to 627°C at constant pressure. If its initial volume was 4 m³ then the final volume Of the gas will be

1. 6 m³
2. 4 m³
3. 12 m³
4. 2 m³

Answer: 3. 12 m³

According to Charles’s law, V ∝ T, when pressure is constant.

Thus,

⇒ \(\frac{V_1}{V_2}=\frac{T_1}{T_2}\)

or, \(\quad \frac{4 \mathrm{~m}^3}{V_2}\)

= \(\frac{27+273}{627+273}\)

= \(\frac{300}{900}\)

=\(\frac{1}{3}\)

V₂ = 3(4m³)

=12m³

Question 8. Let a gas sample be heated from 27°C to 327°C when the initial KE of the molecules is E. What will be the average KE after heating?

1. 2E
2. 327E
3. 300E
4. V2E

Answer: 1. 2E

Given, T₁ = (273 + 27) K = 300 K

and T₂ = (327 + 273) K = 600 K.

Since average KE ∝ T, we have

⇒ \(\frac{E}{E_{\mathrm{f}}}=\frac{300}{600}\)

⇒ \(E_{\mathrm{f}}=2 E\)

Question 9. The temperature corresponding to the energy of 1 eV is approximately

1. 7.6 x 10³ K
2. 7.2 x 10³ K
3. 7.7 x 10³ K
4. 7.1 x 10^-2 K

Answer: 3. 7.7 x 10³ K

Given that average KE = 1 eV = 1.6 x 10-19 J.

The average KE of a molecule of a monatomic gas is \(\frac{3}{2}\), where k = Boltzmann constant = 1.38 x 10^-23 J K^-1 and T tires absolute temperature.

∴ \(\frac{3}{2} k T=1.6 \times 10^{-19} \mathrm{~J} \quad\)

or, \(\quad T=\frac{2\left(1.6 \times 10^{-19} \mathrm{~J}\right)}{3\left(1.38 \times 10^{-23} \mathrm{JK}^{-1}\right)}\)

= \(7.73 \times 10^3 \mathrm{~K}\)

Question 10. A constant-pressure air thermometer gave a reading of 47.5 units of volume when immersed in ice-cold water, and 67 units in a boiling liquid. The boiling point of the liquid is

1. 135°C
2. 112°C
3. 100°C
4. 125°C

Answer: 2. 112°C

According to the pressure law, V ∝ T, when the pressure is constant.

Hence,

⇒ \(\frac{V_1}{V_2}=\frac{T_1}{T_2}\)

or, \(\frac{47.5}{67}=\frac{0+273}{\theta+273}\)

0 = 112°C

Question 11. A bulb contains one mole of hydrogen mixed with one mole of oxygen at temperature T. The ratio of rms values of the velocity of hydrogen molecules to that of oxygen molecules is

1. 1:4
2. 1:16
3. 16:1
4. 4:1

Answer: 4. 4:1

According to the kinetic theory of gaseous pressure,

⇒ \(p=\frac{1}{3} \frac{M}{V} c_{\mathrm{rms}}^2 \quad \text { or } \quad p V=\frac{1}{3} M c_{\mathrm{rms}}^2=R T\)

⇒ \(c_{\mathrm{rms}}^2=\frac{3 R T}{M} \quad\)

or, \(\quad c_{\mathrm{rms}} \propto \frac{1}{\sqrt{M}}\)

Hence,

⇒ \(\frac{\left(v_{\mathrm{rms}}\right)_{\mathrm{H}_2}}{\left(v_{\mathrm{rms}}\right)_{\mathrm{O}_2}}=\sqrt{\frac{(M)_{\mathrm{O}_2}}{(M)_{\mathrm{H}_2}}}\)

= \(\sqrt{\frac{32}{2}}=4\)

= \(\frac{4}{1}\)

Question 12. In a closed vessel, a gas is at pressure p. Ifthemass of all the molecules is halved and their speed is doubled then the resultant pressure will be

1. p
2. 2p
3. 4p
4. \(\frac{p}{2}\)

Answer: 2. 2p

Gaseous pressure is \(p=\frac{1}{3} \frac{M}{V} c_{\mathrm{rms}}^2\).

If the mass of each molecule is m and N is the number of molecules then M = mN.

∴ \(p=\frac{1}{3} \frac{m N}{V} c_{\mathrm{rms}}^2 \propto m c_{\mathrm{rms}}^2\)

Let \(m_1=m \text { and } m_2=\frac{m}{2}\)

∴ \(\left(c_{r m s}\right)_1=v \text { and }\left(c_{r m s}\right)_2=2 v \Rightarrow \frac{p_1}{p_2}=\frac{m v^2}{\frac{m}{2}(2 v)^2}=\frac{1}{2}\)

Hence, the resultant pressure = p₂ = 2p₁ = 2p.

Question 13. The temperature of a gas is held constant while its volume is decreased. The pressure exerted by the gas on the walls of the container increases because its molecules

1. Strike the walls more frequently
2. Strike the walls with higher velocities
3. Are in contact with the wall for a shorter time
4. Strike die walls with a larger force

Answer: 1. Strike the walls more frequently

The pressure exerted by the gas molecules on the walls of the container arises due to their impact on the walls. This impact causes the transfer of momentum from the molecules to the walls and hence, pressure is produced. With the decrease in volume, the impact is much more frequent which leads to an increase in gaseous pressure.

Question 14. A column of mercury of length 10 cm is contained in the middle of a narrow horizontal tube of length 1 m closed at both ends. The air in both halves of the tube is under the pressure of 76 cm of mercury column. The tube is now slowly made vertical. The distance moved by the mercury will be

1. 2.5 cm
2. 4.5 cm
3. 3.0 cm
4. 1.2 cm

Answer: 3. 3.0 cm

In the horizontal position of the tube, the volume of air on both sides of the mercury pellet is 45 A and the pressure is 76 cm of the Hg column. In the vertical position, let the Hg pellet drop by x so the volume of air above mercury = (45 cm + x)A and the new pressure = p₁

Similarly, for air below the Hg pellet,

volume = (45 cm- x)A and pressure = p₂.

Applying Boyle’s law

76(45 cm)A = p₁(45 cm + x)A

=> p₁ = \(p_1=\frac{76 \times(45 \mathrm{~cm})}{(45 \mathrm{~cm}+x)}\)

Similarly, for the lower portion,

76(45 cm)A = p₂(45 cm- x)A

p₂ = \(\frac{76(45 \mathrm{~cm})}{(45 \mathrm{~cm}-x)}\)

Now, p₂ > P₁, so the difference (p₂– p₁) is the pressure produced by the Hg column.

⇒ \((10 \mathrm{~cm})=\frac{76 \times 45 \mathrm{~cm}}{(45 \mathrm{~cm}-x)}-\frac{76 \times 45 \mathrm{~cm}}{(45 \mathrm{~cm}+x)}\)

= \(\frac{(76 \times 45) 2 x}{45^2-x^2}\)

Solving, we get,

x = 2.9cm ≈ 3 cm.

Question 15. 3 mol of hydrogen is mixed with 1 mol of neon. The molar heat capacity at constant pressure is

1. \(\frac{9}{4}\) R
2. \(\frac{13}{2}\) R
3. \(\frac{9}{2}\) R
4. \(\frac{13}{4}\) R

Answer: 4. \(\frac{13}{4}\) R

For hydrogen: n = 3 mol, Cv = latex]\frac{5}{2}[/latex]R (diatomic).

For neon: n =1 mol, CV = \(\frac{3}{2}\)R (monatomic).

For the mixture,

⇒ \(C_V=\frac{n_1 C_{V_1}+n_2 C_{V_2}}{3+1}=\frac{3\left(\frac{5}{2} R\right)+1\left(\frac{3}{2} R\right)}{4}=\frac{9 R}{4}\)

Now, \(C_p-C_V=R\)

⇒ \(C_p=C_V+R\)

= \(\frac{9 R}{4}+R\)

= \(\frac{13}{4} R\)

Question 16. If vrms, vav, and Vmp be rms, average speed, and most probable speed of molecules of a gas obeying Maxwellian velocity distribution then which of the following statements is correct?

1. v_rms < v_av < v_mp
2. v_mp > v_rms > v_av
3. v_rms > v_av > v_mp
4. v_mp < v_rms < v_av

Answer: 3. v_rms > v_av > v_mp

From Maxwell’s distribution, the expressions for velocities are as under:

RMS velocity = \(\sqrt{\frac{3 k T}{m}}=1.73 \sqrt{\frac{k T}{m}}\)

Average velocity = \(=\sqrt{\frac{8 k T}{\pi m}}\)

= \(\sqrt{\frac{8}{3.14}} \sqrt{\frac{k T}{m}}\)

= \(1.6 \sqrt{\frac{k T}{m}}\)

Most probable velocity = \(\sqrt{\frac{2 k T}{m}}=1.41 \sqrt{\frac{k T}{m}}\)

Thus, v_rms>v_av>v_mp

Question 17. One mole of hydrogen gas is contained in a box of volume V = 1.00 m3 at T- 300 K. It is heated to 3000 K and gets converted to a gas of hydrogen atoms. The final pressure would be (assume the gas to be ideal)

1. Same as the initial pressure
2. Twice the initial pressure
3. Ten times the initial pressure
4. Twenty times the initial pressure

Answer: 4. Twenty times the initial pressure

From gas laws,pV = nRT

=> p₁V₁ = n₁RT₁; p₂V₂ = n₂RT₂

Hence = \(\frac{p_1 V_1}{p_2 V_2}=\frac{n_1 T_1}{n_2 T_2}\)

V₁ = V₂ = V; n₁ =1, n₂ = 2 (as H2 molecule splitsinto atoms)

T₁ = 300K, T₂ = 3000 K.

These substitutions in (1) give

⇒ \(\frac{p_1}{p_2}=\frac{300}{2(3000)}\)

= \(\frac{1}{20}\)

⇒ \(p_2=20 p_1\)

Question 18. Two balloons are filled, one with pure He gas and the other with air. If the pressure and temperature of these balloons are the same then the number of molecules per unit volume is

1. More in the He-filled balloon
2. The same in both balloons
3. More in an air-filled balloon
4. In file ratio 1: 2

Answer: 2. The same in both balloons

From the gas laws, \(\frac{p_1 V_1}{T_1}=\frac{p_2 V_2}{T_2}\)

Since p₁ = p₂ and T₁ = T₂, the volumes V₁ = V₂.

Again, pV = nRT.

Hence, for the same values of p, V, and T, both hydrogen and air will have an equal number of molecules per unit volume.

Question 19. A gas mixture contains one mole of O₂ and one mole of He gas. Find the ratio of the specific heat at constant pressure to that at constant volume of the gaseous mixture.

1. 2.5
2. 1.5
3. 2
4. 4

Answer: 2. 1.5

For oxygen: n = 1, C_v = \(\frac{5}{2}\)(diatomic).

For helium: n = 1, C_v = \(\frac{3}{2}\)R (monatomic).

⇒ \(\left(C_V\right)_{\text {mixture }}=\frac{\frac{5}{2} R+\frac{3}{2} R}{1+1}=2 R\)

(Cp)_mixture = (CV)_mixture + R = 2R + R = 3R.

⇒ \(\frac{C_p}{C_V}=\frac{3 R}{2 R}\)

= 1.5

Question 20. A closed cylindrical vessel contains 60 g of Ne and 64 g of 02. If the pressure of the gaseous mixture in the cylinder be 30 bar then the partial pressure of O₂ (in bar) in the cylinder is

1. 15
2. 30
3. 12
4. 20

Answer: 3. 12

Number of moles2R in Ne = \(\frac{60 \mathrm{~g}}{20 \mathrm{~g} \mathrm{~mol}^{-1}}\) = 3 mol.

Number of moles in O₂ = \(\frac{64 \mathrm{~g}}{32 \mathrm{~g} \mathrm{~mol}^{-1}}\) = 2 mol.

Total number of moles = (3 + 2) mol

= 5 mol.

∴ the partial pressure of oxygen

(P) = mole fraction x (32 bar)

= \(\frac{2}{5}\) (30 bar)

= 12 bar.

Question 21. A certain gas is taken to the five states represented by dots in the graph. The plotted curves are isothermals. The order of the most probable speed-up of the molecules at these five states is

1. v_p at 3 > v_p at 1 = v_p at 2 > v_p at 4 = v_p at 5
2. v_p at 1 > v_p at 2 = v_p at 3 > v_p at 4 = v_p at 5
3. v_p at 3 > v_p at 2 = v_p at 4 > v_p at 1 = v_p at 5
4. v_p at 3 > v_p at 2 = v_p at 4 > v_p at 1 = v_p at 5

Answer: 1. v_p at 3 > v_p at 1 = v_p at 2 > v_p at 4 = v_p at 5

Let T₁ T₂, …, T₅ represent the temperatures and \(v_{p_1}, v_{p_2}, \ldots, v_{p_5}\) the corresponding values of the most probable velocities at these temperatures.

From the given set of isothermals, T₁ = T₂, T₄ = T₅, and T₃ is the maximum temperature.

Thus, T₃ > T₁ = T₂ > T₄

= T₅

Since v_p ∝ √T, hence v_p3 > v_p1 = v_p2 > v_p4 = v_p5.

Question 22. The molecules of a given mass of a gas have an rms velocity of 200 ms’1 at 27°C and 1.0 x 105 N m_2 pressure. When the temperature and pressure of the gas are 127 °C and 0.05 x 105 N m“2 respectively, the rms velocity of its molecules in m s-1 is

1. \(\frac{100 \sqrt{2}}{3}\)
2. 100V2
3. \(\frac{100}{3}\)
4. \(\frac{400}{\sqrt{3}}\)

Answer: 4. \(\frac{400}{\sqrt{3}}\)

At T₁ = 27 °C = (27 + 273) K = 300K, p₁= 105 N m^-1, v_rms = 200m s^-1.

At T₂ = 127 ºC = (127 + 273) K = 400 K, p₂ = 0.05N m^-2.

v_rms = ?

From kinetic theory, \(v_{\mathrm{rms}} \propto \sqrt{T}\)

⇒ \(\frac{v_2}{v_1}=\sqrt{\frac{T_2}{T_1}} \Rightarrow \frac{v_2}{200 \mathrm{~m} \mathrm{~s}^{-1}}=\sqrt{\frac{400}{300}}\)

∴ \(v_2=\left(200 \mathrm{~m} \mathrm{~s}^{-1}\right) \sqrt{\frac{4}{3}}=\frac{400}{\sqrt{3}} \mathrm{~m} \mathrm{~s}^{-1}\)

Question 23. A given sample of an ideal gas occupies a volume V at pressure p and absolute temperature T. The mass of each molecule of the gas is m. Which of the following gives the density of the gas?

1. \(\frac{p}{kT}\)
2. \(\frac{mp}{kT}\)
3. \(\frac{p}{kTV}\)
4. mkT

Answer: 2. \(\frac{mp}{kT}\)

From the gas equation, pV = nRT.

∴ \(\frac{p V}{R T}=n \text { (number of moles) }=\frac{\text { mass }}{\text { molar mass }}\)

Density = \(\rho=\frac{\text { mass }}{\text { volume }}\)

= \(\frac{p(\text { molar mass })}{R T}\)

= \(\frac{p}{R T} \cdot\left(m N_{\mathrm{A}}\right)\)

= \(\frac{m p}{T\left(R / N_{\mathrm{A}}\right)}\)

= \(\frac{m p}{k T}\)

Question 24. Keeping the volume constant, if the temperature of a .gas is increased, the

1. Collision on the walls will be less
2. The number of collisions per unit of time will increase
3. Collisions will be in straight lines
4. The rate of collision will not change

Answer: 2. Number of collisions per unit of time will increase

With the increase in temperature, the molecules move more rapidly at random and this leads to an increase in the collision rate.

Question 25. A polyatomic gas with n degrees of freedom has a mean energy per molecule given by

1. \(\frac{n k T}{2}\)
2. \(\frac{n k T}{2N}\)
3. \(\frac{3 k T}{2}\)
4. \(\frac{n k T}{N}\)

Answer: 1. \(\frac{n k T}{2}\)

According to the law of equipartition of energy, energy per degree of

freedom = \(\frac{1}{2}\)KT.

In a polyatomic gas, each molecule has n degrees of freedom (given),

So the average energy per molecule is,

⇒  \(\left(\frac{1}{2} k T\right) n=\frac{n k T}{2}\)

Question 26. According to the kinetic theory of gases, at absolute zero temperature,

1. Water freezes
2. Liquid helium freezes
3. Molecular motion stops
4. Liquid hydrogen freezes

Answer: 3. Molecular motion stops

According to the kinetic theory,

⇒  \(p=\frac{1}{3} \frac{m}{V} C_{\mathrm{rms}}^2 \Rightarrow p V=R T=\frac{1}{3} M C_{\mathrm{rms}}^2\)

At absolute zero,

⇒ \(C_{\mathrm{rms}}^2=0 \Rightarrow \frac{1}{n}\left(C_1^2+C_2^2+C_3^2+\ldots+C_n^2\right)=0\)

This is possible if C₁ = C₂ = C₃ = ….. = 0.

Hence, molecular motion of all types stops.

Question 27. In Maxwell’s speed distribution curve for nitrogen, the average relative velocity between two molecules at 300 K will be

1. 300 ms^-1
2. 920 ms^-1
3. 606 ms^-1
4. zero

Answer: 3. 606 ms^-1

The magnitude of relative velocity between two molecules is

⇒ \(\left|\vec{v}_{\text {rel }}\right|=\sqrt{v^2+v^2-2 v^2 \cos \theta}=2 v \sin \frac{\theta}{2}\)

Average value is

⇒ \(\mid \vec{v}_{\mathrm{rel}} \mathrm{lav}_{\mathrm{av}}=\frac{1}{\pi} \int_0^\pi 2 v \sin \frac{\theta}{2} d \theta=\frac{4 v_{\mathrm{av}}}{\pi}\)

According to.Maxwell’s velocity distribution,

⇒ \(v_{\mathrm{av}}=\sqrt{\frac{8 R T}{\pi m}}\)

average relative velocity

⇒ \(=\frac{4}{\pi} \sqrt{\frac{8 R T}{\pi m}}\)

⇒ \(\frac{4}{3.14} \sqrt{\frac{8\left(8.3 \mathrm{~J} \mathrm{~mol}^{-1} \mathrm{~K}^{-1}\right)(300 \mathrm{~K})}{3.14\left(28 \times 10^{-3} \mathrm{~kg} \mathrm{~mol}^{-1}\right)}}\)

= \(606 \mathrm{~m} \mathrm{~s}^{-1}\)

Question 28. The radius of an oxygen molecule is 40 A. Its time of relaxation at atmospheric pressure and a temperature of 27°C will be

1. 10^-12 s
2. 10^-10 s
3. 10^-16 s
4. 10^-14 s

Answer: 1. 10^-12s

The rms velocity of gaseous molecules is

⇒ \(v_{\mathrm{rms}}=\frac{\text { mean free path }(\lambda)}{\text { relaxation time }(\tau)}\)

⇒ \(\tau=\frac{\lambda}{v_{\mathrm{rms}}}\)

= \(\frac{1}{\sqrt{2} \pi n d^2} \sqrt{\frac{m_{\mathrm{O}}}{3 R T}}\)

Now, number density = \(\), where p = number of moles.

But \(n=\frac{N}{V}=\frac{\mu N_A}{V}\)

∴ \(p V=\mu R T \text { or } \frac{\mu}{V}=\frac{p}{R T} \text {. Hence, } n=\frac{N_{\mathrm{A}} p}{R T}\)

Substituting the given values, x = 0.01 x 10^-10s

= 10^-12 s.

Question 29. If 10²²molecules of a gas, each of mass 10^-26 kg, collide elastically and perpendicularly with a surface per second over an area of 1 m² with a speed of 104 m s^-1, the pressure exerted by the gas molecules will be of the order of

1. 4 Pa
2. 3 Pa
3. 2 Pa
4. 5 Pa

Answer: 3. 2 Pa

For an elastic collision, the change in momentum per collision = 2mu,

and in 1 s, n molecules collide. Hence, net force F = 2mun.

This force acts on an area of 1 m². Hence, pressure is

⇒ \(p=\frac{F}{A}\)

= \(\frac{2 m u n}{1 \mathrm{~m}^2}\)

= \(\frac{2\left(10^{-26} \mathrm{~kg}\right)\left(10^4 \mathrm{~m} \mathrm{~s}^{-1}\right)\left(10^{22}\right)}{1 \mathrm{~m}^2}\)

= \(2 \mathrm{Nm}^{-2}\)

= 2Pa.

Question 30. 15 g of nitrogen is enclosed in a vessel at a temperature of 27°C. For the rms speed of its molecules to get doubled, the amount of heat absorbed by the gas is about

1. 10 kJ
2. 14 kJ
3. 6 kJ
4. 0.5 kJ

Answer: 1. 10 kJ

The rms velocity \(v_{\mathrm{rms}}=\sqrt{\frac{3 R T}{M}}\)

When v_rms is doubled, temperature increases from T to 4T.

Hence, increase in temperature = AT = 4T-T

= 3T

= 3(273 + 27) K

= 900 K.

Heat absorbed (at constant volume) = ΔQ = nCvΔT.

Here,

n = \(\frac{15g}{28g}\)

= \(\frac{15}{28}, C_V=\frac{5}{2} R\)

⇒ \(\Delta Q=\frac{15}{28} \cdot \frac{5}{2}\) (8-3).900

= 104J

= 10kJ.

Question 31. An increase in the temperature of a gas-filled in container would lead to

1. An increase in its mass
2. An increase in its kinetic energy
3. A decrease in its pressure
4. A decrease in intermolecular separation

Answer: 2. An increase in its kinetic energy

The energy of an ideal gas is purely kinetic, and is \(\frac{1}{2}\)kT per degree of freedom.

Hence, an increase in temperature leads to an increase in its kinetic energy.

Question 32. A gas mixture consists of 3 mol of oxygen and 5 mol of argon at temperature T. Considering only translational and rotational modes, the total internal energy of the gaseous mixture will be

1. 12 RT
2. 20 RT
3. 15 RT
4. 4 RT

Answer: 3. 15 RT

Oxygen is diatomic, so it has 5 degrees of freedom for each molecule.

Hence, 3 mol of oxygen has total degrees of freedom = (3NA)5 = 15NA.

Similarly, argon (monatomic) has 3 degrees of freedom per molecule, and thus for 5 mol, the number of degrees of freedom = (5NA)3 = 15NA.

∴ total numberofdegrees offreedom = 30NA.

According to the equipartition law, the total energy is

⇒ \(E=\left(30 N_A\right)\left(\frac{1}{2} k T\right)\)

Question 33. An ideal gas occupies a volume of 2 m³ at a pressure of 3 x 10⁶ Pa. The energy of the gas is

1. 6 x 10⁴ J
2. 9 x 10⁶ J
3. 3 x 10³ J
4. 10⁷J

Answer: 2. 9 x 10⁶ J

According to the kinetic theory, the pressure is

⇒ \(p=\frac{1}{3} \frac{M}{V} C_{\mathrm{rms}}^2\)

= \(\frac{2}{3 V}\left(\frac{1}{2} M C_{\mathrm{rms}}^2\right)\)

= \(\frac{2}{3 V}(\mathrm{KE})\)

∴ energy of the gas = E = \(\frac{3}{2}\) pV.

Substituting the given values,

E = \(\frac{3}{2}\) (3 x 10⁶ Pa)(2 m³)

= 9 x 10⁶ J.

Question 34. The temperature at which the rms speed of hydrogen molecules equals their escape velocity from the earth is closest to (given that Boltzmann constant, kB = 1.38 x 10^-23 J K-1; Avogadro’s number, NA = 6.02 x 10²³ mol^-1; earth’s radius = 6400 km; acceleration due to gravity = 10 m s^-2)

1. 10⁴K
2. 650 K
3. 800 K
4. 3 x 10⁵ K

Answer: 1. 10⁴K

The rms speed \(C_{\mathrm{rms}}=\sqrt{\frac{3 R T}{M}}\)

where M = molar mass ofhydrogen = 2 g mol^-1 = 2 x 10³ kgmol^-1.

Given that = escape velocity = \(\sqrt{2 g r}\), where r is earth’s radius

Substituting the given values,

⇒ \(\sqrt{\frac{3 R T}{M}}=\sqrt{2 g r}\)

⇒ \(T=\frac{2 M g r}{3 R}\)

1.02 x 10⁴K ≈ 10⁴K

Question 35. If the temperature of an ideal gas enclosed in a box is increased then the

1. Mean free path decreases
2. The mean free path remains unchanged
3. Relaxation time decreases
4. Relaxation time remains unchanged

Answer: 2. Mean free path remains unchanged

Mean free path = \(\lambda=\frac{1}{\sqrt{2} \pi n d^2}, \text { where } n=\frac{N}{V}\) = number density,

d = diameter of molecule.

With the increase in temperature, the rate of collision increases, so relaxation time decreases, but the mean free path remains unchanged.

Thermodynamic Processes

Question 1. A thermodynamic system is taken from state A to state B along ACB and brought back to A along BDA as shown in the adjoining p-V diagram. The net work done during the complete cycle is given by the area

1. p₁ACBp₂p₁
2. ACBB’A’A
3. ACBDA
4. ADBB’A’A

Answer: 3. ACBDA

Work done in a cyclic process is the area enclosed by the cycle on the p-V diagram.

Thus, W = area ACBDA. Note that work done is positive for a clockwise cycle and negative for an anticlockwise process in the p-V diagram.

Question 2. An ideal gas undergoing an adiabatic process has a pressure-temperature relationship

1. p^γ-1T^γ = constant
2. p^γT^γ-1 = constant
3. p^γT^1-γ = constant
4. p^1-γT^γ = constant

Answer: 4. p^1-γT^γ = constant

For an adiabatic process, the relation between p and V is pV^γ = constant. From the equation of state pV = RT, we get

⇒ \(V=\frac{R T}{p}\)

or, \(p\left(\frac{R T}{p}\right)^\gamma=\text { constant or } p^{1-\gamma} T^\gamma=\text { constant. }\)

Question 3. An ideal gas at 27 °C is compressed adiabatically to 8/27 of its original volume. The rise in temperature is (take y = 5/3)

1. 475°C
2. 402°C
3. 275°C
4. 375°C

Answer: 4. 375°C

For adiabatic compression,

⇒ \(T_1 V_1^{\gamma-1}=T_2 V_2^{\gamma-1}\)

∴ \((27+273) V^{\frac{5}{3}-1}=T_2\left(\frac{8}{27} V\right)^{\frac{5}{3}-1}\)

∴ \(300 V^{\frac{2}{3}}=\left(T_2\right)\left(\frac{2}{3}\right)^2 V^{\frac{2}{3}}\)

9(300) = 4T₂

∴ \(T_2=\frac{9 \times 300}{4}\)

= 675K.

Hence, the rise in temperature is T₂ – T₁ = 675 – 300

= 375°C.

Question 4. The molar heat capacity at constant pressure of an ideal gas is \(\frac{7}{2}\) R. The ratio of the molar heat capacity at constant pressure to that at constant volume is

1. \(\frac{7}{5}\)
2. \(\frac{8}{7}\)
3. \(\frac{5}{7}\)
4. \(\frac{9}{7}\)

Answer: 1. \(\frac{7}{5}\)

Given that,

⇒ \(C_p=\frac{7}{2} R, \text { but } C_p-C_V=R \text { or } C_V=\frac{7}{2} R-R=\frac{5}{2} R\)

∴ \(\frac{C_p}{C_V}=\frac{\frac{7 R}{2}}{\frac{5 R}{2}}=\frac{7}{5}\)

Question 5. A thermodynamic system is taken through a cyclic process ABCDA as shown in the figure. Heat expelled by the gas during the cycle is

1. 2pV
2. 4pV
3. \(\frac{1}{2}\) pV
4. pV

Answer: 1. 2pV

From the first law of thermodynamics, dQ = dU + dW, and the change in internal energy in a cyclic process is dU = 0.

dQ = dW = area of cyclic process = (2p-p)(3V- V)

= 2pV.

Since the cycle is anticlockwise, work done is negative; hence heat expelled is 2pV

Question 6. A gas is taken through the cycle ABCA as shown in the figure. What is the net work done by the gas?

1. 2000 J
2. 1000 J
3. -200 J
4. Zero

Answer: 2. 1000 J

Net work done is the area enclosed by the cycle in the p-V diagram.

Hence,

⇒ \(W=\frac{1}{2}(A C)(B C)=\frac{1}{2}\left(5 \times 10^{-3} \mathrm{~m}^3\right)\left(4 \times 10^5 \frac{\mathrm{N}}{\mathrm{m}^2}\right)\)

= 1000J.

Question 7. The molar heat capacities of an ideal gas at constant pressure and constant volume are C_p and C_v respectively. If \(\gamma=\frac{C_p}{C_V}\) and R is the universal gas constant then C_v is equal to

1. \(\frac{1+\gamma}{1-\gamma}\)
2. \(\frac{R}{\gamma-1}\)
3. \(\frac{\gamma-1}{R}\)
4. yR

Answer: 2. \(\frac{R}{\gamma-1}\)

For a gas, C_p -C_v = R.

Dividing throughout by C_v,

⇒ \(\frac{C_p}{C_V}-1=\frac{R}{C_V}\)

⇒ \(\gamma-1=\frac{R}{C_V}\)

⇒ \(C_V=\frac{R}{\gamma-1}\)

Question 8. In the given (V-T) diagram, what is the relation between pressures p₁ and p₂?

1. p₂ = p₁
2. p₂ > p₁
3. p₂ < P₁
4. Cannot be predicted

Answer: 3. p₂ < P₁

From the gas laws, pV = nRT or V = \(V=\left(\frac{n R}{p}\right) T\)

Hence, the slope of the V-Tline is inversely proportional to the pressure

⇒ \(\left(\text { slope } \propto \frac{1}{p}\right)\),

Hence p₂ < P₁.

Question 9. During an adiabatic process, the pressure of a gas is found to be proportional to the cube of its temperature. The ratio C_p/C_v for the gas is

1. \(\frac{4}{3}\)
2. 2
3. \(\frac{5}{3}\)
4. \(\frac{3}{2}\)

Answer: 4. \(\frac{3}{2}\)

For an adiabatic process,

p^1-γT^γ = constant

⇒ \(p T^{\frac{\gamma}{1-\gamma}}=\text { constant }\)

⇒ \(\frac{p}{T^{\frac{\gamma}{\gamma-1}}}=\text { constant }\)

⇒ \(p \propto T^{\frac{\gamma}{\gamma-1}}\)…..(1)

Comparing (1) with the given condition p ∝ T₃, we conclude that

⇒ \(\frac{\gamma}{\gamma-1}=3, \text { thus } \gamma=\frac{3}{2}\)

Question 10. One mole of an ideal diatomic gas undergoes a transition from A to B along the straight path AB as shown in the figure. The change in internal energy of the gas during the transition is

1. 20 kJ
2. -20 kJ
3. 20 J
4. -12 kJ

Answer: 2. -20 kJ

For a diatomic gas, Cv = \(\frac{5}{2}\) R, and change in internal energy is

⇒ \(\Delta U=n C_V \Delta T=\frac{5}{2} R\left(T_2-T_1\right)\) [∵ n = l].

From the gas laws, pV = RT, hence T = \(\frac{pV}{R}\)

∴ \(\Delta U=\frac{5}{2} R \frac{\left(p_2 V_2-p_1 V_1\right)}{R}=\frac{5}{2}\left(p_2 V_2-p_1 V_1\right)\)

Substituting the values from the given graph,

⇒ \(\Delta U=\frac{5}{2}\left[\left(2 \times 10^3 \mathrm{~Pa}\right)\left(6 \mathrm{~m}^3\right)-\left(5 \times 10^3 \mathrm{~Pa}\right)\left(4 \mathrm{~m}^3\right)\right]\)

= \(\frac{5}{2}(-8 \mathrm{~kJ})\)

= -20 kJ.

Question 11. An ideal gas is compressed to half its initial volume by means of several processes. Which of the processes results in the work done on the gas?

1. Isochoric
2. Adiabatic
3. Isobaric
4. Isothermal

Answer: 2. Adiabatic

For the gas undergoing an isochoric process (volume constant), no work is done by the gas. The work done on the gas is the area under the p-V diagram. Hence, according to the given figures,

Question 12. A thermodynamic system undergoes a cyclic process ABCDA as shown in the figure. The work done by the system in one cycle is

1. p₀V₀
2. 2p₀V₀
3. \(\frac{p_0 V_0}{2}\)
4. Zero

Answer: 4. Zero

For a cyclic process, the net work done by the system is equal to the area enclosed in the p-V diagram. The given process comprises two closed areas, AODA and COBC. The first is clockwise, so the work done is +ve while that for the second is -ve (being anticlockwise).

∴ W_net = area AOD- area COB

⇒ \(\frac{1}{2}\left(2 V_0-V_0\right)\left(2 p_0-p_0\right)-\frac{1}{2}\left(2 V_0-V_0\right)\left(3 p_0-2 p_0\right)\)

⇒ \(\frac{1}{2} p_0 V_0-\frac{1}{2} p_0 V_0\)

= 0.

Question 13. One mole of an ideal monatomic gas undergoes a process described by the equation (pV³ = constant). The heat capacity of the gas during this process is

1. \(\frac{3}{2}\) R
2. \(\frac{5}{2}\) R
3. 2R
4. R

Answer: 4. R

For a polytropic process (pVn = constant), the molar heat capacity is

⇒ \(C=C_V+\frac{R}{1-n}\)

In the given process, pV³ = constant, we have n = 3

∴ \(C=C_V+\frac{R}{1-3}=\frac{3}{2} R-\frac{R}{2}=R\) [∵ \(C_V=\frac{3}{2} R\)]

Question 14. One mole of an ideal gas undergoes a process from an initial state A to the final state B via two processes. It first undergoes isothermal expansion from volume V to 3V and then its volume is reduced from 3V to V at constant pressure. The correct p-V diagram representing the two processes is

Answer: 4.

The isothermal expansion from state A (volume V) to state C (volume 3V) is represented by the rectangular hyperbola AC in (4).

In the second process, the isobaric compression from state C to final state B is shown by the line CB in (4).

Hence, the correct p-V is (4).

Question 15. The ratio of the molar heat capacities \(\frac{C_p}{C_V}=\gamma\) in terms of degrees of freedom (f) is given by

1. 1 + \(\frac{1}{f}\)
2. 1 + \(\frac{f}{3}\)
3. 1 + \(\frac{2}{f}\)
4. 1 + \(\frac{f}{2}\)

Answer: 3. 1 + \(\frac{2}{f}\)

If the total number of degrees of freedom of a polyatomic gas molecule is f, the internal energy per mole of the gas will be

U = \(\frac{f}{2}\) RT.

∴ \(C_V=\frac{d U}{d T}=\frac{1}{2} f R, \text { hence } C_p=C_V+R=\left(\frac{f}{2}+1\right) R\)

∴ \(\frac{C_p}{C_V}=\frac{\left(\frac{f}{2}+1\right) R}{\frac{f_2}{2} R}=1+\frac{2}{f}\)

Question 16. A gas undergoes two processes to reach the final state C from the initial state A as shown in the p-V diagram. In the process, AB, 400 J of heat is absorbed by the gas, and in the process BC, 100 J of heat is absorbed. The heat absorbed by the system in the process of AC will be

1. 380 J
2. 500 J
3. 460 J
4. 300 J

Answer: 3. 460 J

Since the initial state (A) and the final state (C) are the same for the two processes, the change in internal energy AU will be the same.

For the process ABC,

ΔQ_ABC = W_AB + W_BC + AU

=> 400J +100J = 0(isochoric) + 6 x 20J + AU

=> 500 J = 120 J + AU……(1)

Similarly, for the process AC,

ΔQ_AC = AU + AW = AU + \(\frac{1}{2}\)(8 x 20)J

⇒ ΔQ_AC = 80J + AU…..(2)

Subtracting (2) from (1),

500J – ΔQ_AC = 40J

ΔQ_AC = 500 J- 40 J

= 460 J.

Question 17. A monatomic gas at pressure p and volume Vexpands isothermally to a volume 2V and then adiabatically to a volume of 16V. The final pressure of the gas is (take y = 5/3)

1. 64p
2. 32p
3. \(\frac{p}{34}\)
4. 16p

Answer: 3. \(\frac{p}{34}\)

For the isothermal process, pV = \(\frac{p}{2}\)(2V).

For the adiabatic process, pV^γ = constant.

∴ \(\frac{p}{2}(2 V)^{5 / 3}=p^{\prime}(16 V)^{5 / 3} \Rightarrow p \cdot 2^{2 / 3}=p^{\prime} \cdot 2^{20 / 3}\)

∴ final pressure = \(\frac{p}{2^6}=\frac{p}{64}\)

Question 18. If All and AW represent the increase in internal energy and work done by the system respectively in a thermodynamical process, which of the following is true?

1. ΔU = -ΔW in an adiabatic process
2. ΔU = ΔW in an isothermal process
3. ΔU = ΔW in an adiabatic process
4. ΔU = -ΔW in an isothermal process

Answer: 1. ΔU = -ΔW in an adiabatic process

For an isothermal process, ΔU = 0,

So, options (2) and (4) are incorrect.

For an adiabatic process,

ΔQ = ΔU + ΔW

⇒ ΔU + ΔW = 0

⇒ ΔU = – ΔW is correct.

Question 19. In a thermodynamic process, which of the following statements is not true?

1. In an adiabatic process, the system is insulated from the surroundings.
2. In an isochoric process, pressure remains constant.
3. In an isothermal process, the temperature remains constant.
4. In an adiabatic process, pV^γ = constant.

Answer: 2. In an isochoric process, pressure remains constant.

For an adiabatic process, there is no exchange of heat between the body and the surroundings and the process follows the law pV^γ = constant.

Hence, (1) and (4) are true.

For an isothermal process, temperature remains constant, so (3) is true.

Finally, for an isochoric process, the volume remains constant and not the pressure.

So, option (4) is incorrect.

Question 20. The internal energy change in a system that has absorbed 2 kcal of heat and done 500 J of work is

1. 8900 J
2. 6400 J
3. 5400 J
4. 7900 J

Answer: 4. 7900 J

From the first law of thermodynamics, ΔQ = ΔU + ΔW.

Given, ΔQ = 2000 cal

= 2000 x 4.2J

= 8400 J and ΔW

= 500 J.

∴ ΔU = ΔQ – ΔW

= 8400 J- 500 J

= 7900 J

Question 21. In the cyclic process shown in the V-p diagram, the magnitude of work done is

1. \(\frac{\pi}{4}\left(p_2-p_1\right)^2\)
2. \(\frac{\pi}{4}\left(V_2-V_1\right)^2\)
3. \(\frac{\pi}{4}\left(p_2-p_1\right)\left(V_2-V_1\right)\)
4. \(\pi\left(p_2 V_2-p_1 V_1\right)\)

Answer: 3. \(\frac{\pi}{4}\left(p_2-p_1\right)\left(V_2-V_1\right)\)

For a cyclic process plotted in the p-V diagram, the work done = area inside the closed curve. We can treat the circle as an ellipse ot

semimajor axis \(\frac{1}{2}\)(P₂ – P₁) semimmor axis \(\frac{1}{2}\)(V₂ – V₁).

∴ work done \(\pi \frac{1}{2}\left(p_2-p_1\right) \frac{1}{2}\left(V_2-V_1\right)=\frac{\pi}{4}\left(p_2-p_1\right)\left(V_2-V_1\right)\)

Question 22. A gas with \(\frac{C_p}{C_V}=\gamma\) goes from an initial state (p₁, V₁, T₁) to a final state (P₂, V₂, T₂) Enough an adiabatic process. The work done by the gas is

1. \(\frac{p_1 V_1-p_2 V_2}{\gamma-1}\)
2. \(\frac{p_1 V_1+p_2 V_2}{\gamma+1}\)
3. \(\frac{n R\left(T_1+T_2\right)}{\gamma-1}\)
4. nyR(T1 – T2)

Answer: 1. \(\frac{p_1 V_1-p_2 V_2}{\gamma-1}\)

Work done during the adiabatic expansion from state A (p₁, V₁, T₁) to state B (p₂, V₂, T₂) is given by

⇒ \(W=\frac{n R}{\gamma-1}\left(T_1-T_2\right)=\frac{1}{\gamma-1}\left(n R T_1-n R T_2\right)\)

⇒ \(\frac{1}{\gamma-1}\left(p_1 V_1-p_2 V_2\right)\) [∵ pV = nRT]

Question 23. A cyclic process ABCA is shown in the p-T diagram. Which of the following diagrams shows the same process in the p-V diagram?

Answer: 2.

In the given cyclic process, consider the three processes individually.

(1) Process AB: p oc T(for the straight line) The process is isochoric (volume constant) as in options (1) and (2).

(2) Process BC: Isobaric compression as in options (a) and (b).

(3) Process GA: Isothermal process (T – constant), so according to Boyle’s law (pV = constant) which gives a rectangular hyperbola, option (2) is true.

Question 24. A cyclic process ABC is shown in the p-T diagram. Which of the following curves shows the same process in a V-T diagram?

Answer: 3.

Process AB: pecT, hence V = constant with T increasing: option (3).

Process BC: Isothermal expansion (T=constant), T constant and volume increasing: option (3).

Process CA: Isobaric (p = constant), volume decreases linearly with temperature as in option (3).

Hence (3) is the correct representation.

Question 25. A sample of n moles of a gas undergoes isothermal expansion from volume V₁ to V₂ at temperature T. The work done by the gas is

1. \(n R T \ln \left(\frac{V_2}{V_1}+1\right)\)
2. \(n R T \ln \left(\frac{V_2}{V_1}-1\right)\)
3. \(n R T \ln \frac{V_2}{V_1}\)
4. \(n R T\left(\frac{V_2}{V_1}\right)\)

Answer: 4. \(n R T\left(\frac{V_2}{V_1}\right)\)

Work done during the isothermal expansion is

⇒ \(W=n R T \ln \frac{V_2}{V_1}\)

Question 26. A system is taken from state A to state B along two different paths, 1 and 2. The heat absorbed and work done by the system along these two paths are Q₁ and Q₂, and W₁ and W₂ respectively. Which of the following is true?

1. Q₁– Q₂
2. W₁ = W₂
3. Q₁-Q₂ = W₁-W₂
4. Q₁ + W₁ = Q₂ + W₂

Answer: 3. Q₁-Q₂ = W₁-W₂

The internal energy function is a state function, which depends only on the initial and final states.

For A: Q₁ = W₂ + ΔU.

For B: Q₂ = W₂ + ΔU, where ΔU= U_B-U_A.

∴ Q₁ – W₁ = Q₂ – W₂

Q₁ – Q₂ = W₁ – W₂

Question 27. If the ratio of the specific heat capacity of a gas at constant pressure to that at constant volume is y then the change in internal energy of a given mass of the gas when its volume changes from V to 2V at constant pressure p is

1. \(\frac{R}{\gamma-1}\)
2. pV
3. \(\frac{pV}{\gamma-1}\)
4. \(\frac{\gamma p V}{\gamma-1}\)

Answer: 3. \(\frac{pV}{\gamma-1}\)

Change in internal energy is

⇒ \(\Delta U=n C_V \Delta T=n\left(\frac{R}{\gamma-1}\right)\left(T_2-T_1\right)\)

⇒ \(\frac{1}{\gamma-1}\left(n R T_2-n R T_1\right)=\frac{1}{\gamma-1}\left(p_2 V_2-p_1 V_1\right)\)

Given that p₁ = p₂ = p, V₂ = 2V, V₁ = V, so

⇒ \(\Delta U=\frac{p}{\gamma-1}(2 V-V)=\frac{p V}{\gamma-1}\)

Question 28. An ideal gas A and a real gas B have their volumes increased from V to 2V under isothermal conditions. The increase in internal energy

1. Will be the same in both A and B
2. There will be zero in both gases
3. Of B will be more than that of A
4. Of A will be more than that of B

Answer: 2. Will be zero in both the gases

Under isothermal conditions temperature T remains constant, so dT = 0. The change in internal energy = AU = nC_vdT = 0. Hence, the increase in internal energy in both A and B is zero.

Question 29. The temperature inside a refrigerator is maintained at 4°C and the temperature of the atmosphere is 15°C. If the gas enclosed undergoes the Carnot process in its working, find the Carnot efficiency.

1. 0.028
2. 0.038
3. 0.072
4. 0.054

Answer: 2. 0.038

During the Carnot cyclic process, efficiency

⇒ \(\eta=1-\frac{T_2}{T_1}=1-\frac{(273+4) \mathrm{K}}{(273+15) \mathrm{K}}\)

⇒ \(1-\frac{277}{288}=\frac{11}{288}\)

= 0.038.

Question 30. A conducting, closed container of capacity 100 litres contains an ideal gas at a high-pressure p0. Using an exhaust pump the gas from the container is pumped out at a constant rate of 5 litres per second. Find the time in which the pressure inside the container is reduced to \(\frac{p_0}{100}\) (Assume isothermal conditions.)

1. 92 s
2. 110 s
3. 45 s
4. 150 s

Answer: 1. 92 s

For an isothermal process,

pV = constant

pdV + Vdp = 0

⇒ \(\frac{d p}{p}=-\frac{d V}{V}\)……(1)

Given that V = 100 L = 100 x 10^-3 m³, initial pressure = p₀,

⇒ \(\frac{d V}{d t}=5 \mathrm{~L} \mathrm{~s}^{-1}\)

= \(5 \times 10^{-3} \mathrm{~m}^3 \mathrm{~s}^{-1}\)

⇒ \(d V=\left(5 \times 10^{-3} d t\right) \mathrm{m}^3 \mathrm{~s}^{-1}\)

Final pressure = \(\frac{p_0}{100}\)

Integrating (1),

⇒ \([\ln p]_{p_0}^{p_0 / 100}=-\int_0^t \frac{5 \times 10^{-3} d t}{100 \times 10^{-3}}\)

⇒ \(\ln p_0-\ln \frac{p_0}{100}=5 \times 10^{-2} t\)

∴ time \(t=\frac{1}{5 \times 10^{-2}}(2 \ln 10)\)

= 0.4 x 100 x 2.3

= 92 s.

Question 31. The given p-V indicator diagram p shows four processes: isochoric, isobaric, isothermal, and adiabatic. The correct assignment of the processes in the same order is given

1. ADCB
2. ADBC
3. DABC
4. DBCA

Answer: 3. DABC

Process A: Isobaric (p constant)

Process B: Isothermal (T constant)

Process C: Adiabatic (greater slope, ΔQ = 0)

Process D: Isochoric (V constant)

Hence, option (3)

Question 32. In an isobaric process, work done by a diatomic gas is 10 J. The heat given to the gas will be

1. 35 J
2. 25 J
3. 45 J
4. 30 J

Answer: 1. 35 J

For an isobaric process (p constant),

pV =nRT

=> pdV = nRdT.

Work done = dW = pdV = nRdT……(1)

Heat given to the gas is

⇒ \(d Q=n C_p d T=n\left(\frac{7}{2} R\right) d T\) [∵ \(C_p=\frac{7}{2} R\)]

⇒ \(\frac{7}{2}(n R d T)=\frac{7}{2}(d W)\) [from (1)]

= \(\frac{7}{2}\) (10J)

= 35J.

Question 33. An ideal gas initially at a pressure of 1 bar is compressed from 30 m³ to 10 m³ and its temperature decreases from 320 K to 280K. The final pressure will be

1. 3.4 bar
2. 1.25 bar
3. 2.625 bar
4. 4.36 bar

Answer: 3. 2.625 bar

From the gas laws, \(\frac{p_1 V_1}{T_1}=\frac{p_2 V_2}{T_2}\)

⇒ \(\frac{(1 \mathrm{bar})\left(30 \mathrm{~m}^3\right)}{(320 \mathrm{~K})}=\frac{p_2\left(10 \mathrm{~m}^3\right)}{(280 \mathrm{~K})}\)

final pressure = p₂ = 2.625 bar.

Question 34. One mole of nitrogen is heated isobarically from 300 K to 600 K. The change in its entropy is

1. 20 J K^-1
2. 30 JK^-1
3. 40 JK^-1
4. 50 JK^-1

Answer: 1. 20 J K^-1

Change in entropy \(\Delta S=\int \frac{d Q}{T}\)

But \(d Q=n C_p d T=1\left(\frac{7}{2} R\right) d T\)

⇒ \(\Delta S=\frac{7}{2} R \int_{300 \mathrm{~K}}^{600 \mathrm{~K}} \frac{d T}{T}\)

= \(\frac{7 R}{2} \ln 2\)

= \(\frac{7}{2}(8.3)(0.693) \mathrm{JK}^{-1}\)

= 20JK^-1

Question 35. One mole of an ideal gas undergoes the process A → B → C. Given p A that TA = 40 K, Tc = 400 K, and \(\frac{p_{\mathrm{B}}}{p_{\mathrm{A}}}=\frac{1}{5}\). The heat supplied to the gas is

1. 2059.2 J
2. 2659.2 J
3. 3659.2 J
4. 2259.2 J

Answer: 2. 2659.2 J

For isochoric compression A → B, heat expelled = nC_vdT = nC_v(T_B– T_A)

and for isobaric expansion B → C, heat absorbed = nC_pdT = nC_p(T_C– T_B).

∴ net heat absorbed is

ΔQ = nC_pdT + nC_vdT

= nC_p(T_C – T_B) + nC_v(T_B– T_A).

Given that

⇒ \(\frac{p_{\mathrm{B}}}{p_{\mathrm{A}}}=\frac{1}{5}=\frac{T_{\mathrm{B}}}{T_{\mathrm{A}}}\)

⇒ \(T_{\mathrm{B}}=\frac{T_{\mathrm{A}}}{5}=\frac{400 \mathrm{~K}}{5}\)

∴ ΔQ = (T_C – T_B)C_p-C_v(T_C-T_B) [∵ T_A = T_C (given)]

⇒ \(\left(400-\frac{400}{5}\right) R\)

= \(\frac{4}{5}(400)(8.31) \mathrm{J}\)

= 2659.2J

Question 36. C_p/C_v for a mixture of 11 g of CO₂ and 14 g of N₂ is

1. \(\frac{7}{5}\)
2. \(\frac{11}{5}\)
3. \(\frac{4}{3}\)
4. \(\frac{11}{8}\)

Answer: 4. \(\frac{11}{8}\)

For CO₂: \(n=\frac{1}{4}, C_V=3 R, C_p=4 R\)

For N₂: \(n=\frac{1}{2}, C_V=\frac{5}{2} R, C_p=\frac{7}{2} R\)

∴ \(\gamma_{\text {mixture }}=\frac{n_1 C_{p_1}+n_2 C_{p_2}}{n_1 C_{V_1}+n_2 C_{V_2}}=\frac{\frac{1}{4} \times 4 R+\frac{1}{2} \times \frac{7}{2} R}{\frac{1}{4} \times 3 R+\frac{1}{2} \times \frac{5}{2} R}=\frac{11}{8}\)

Question 37. For the given cyclic process CABC for a gas shown in the p-V indicator diagram, the work done by the gas is

1. 5 J
2. 1 J
3. 30 J
4. 10 J

Answer: 4. 10 J

Work done by the gas during the process

C → A (expansion) = area under CA

WCA = (6 Pa)(5 m³-1 m³) = 24J.

WAB = zero, for the isochoric process.

WBC = area under BC.(compression)

= –\(\frac{1}{2}\)(6 Pa +1 Pa)(5 m³-1 m³)

= -14J.

Net work done during one complete cycle is

W = 24J + (-14) J

= 10J.

Question 38. When heat Q is supplied to a diatomic gas of rigid molecules at constant volume, its temperature increases by AT. The heat required to produce the same change in temperature at a constant pressure is

1. \(\frac{7Q}{5}\)
2. \(\frac{5Q}{3}\)
3. \(\frac{3Q}{2}\)
4. \(\frac{2Q}{3}\)

Answer: 1. \(\frac{7Q}{5}\)

For a diatomic gas, \(C_V=\frac{5}{2} R, C_p=\frac{7}{2} R\)

At constant volume, \(Q=n C_V \Delta T=n \frac{5}{2} R \Delta T\)

At constant pressure, \(Q^{\prime}=n C_p \Delta T=n \cdot \frac{7}{2} R \Delta T\)

∴ \(\frac{Q^{\prime}}{Q}=\frac{7}{5}, \text { hence } Q^{\prime}=\frac{7}{5} Q\)

Question 39. A diatomic gas with rigid molecules does 10 J of work when expanded at constant pressure. The amount of heat absorbed during the process will be

1. 40 J
2. 35 J
3. 30 J
4. 25J

Answer: 2. 35 J

Work done at constant pressure is

dW= pdV= nRdT = 10J …..(1)

Heat absorbed = dQ = dU + dW.

For a diatomic gas, C = \(\frac{5}{2}\)R.

Hence, change in internal energy is

⇒ \(d U=n C_V d t=\frac{5}{2} R n d T=\frac{5}{2}(10 \mathrm{~J})\) [from (1)]

= 25J.

Substituting in (2), heat absorbed is

dQ = 25J +10J

= 35J.

Question 40. A cylinder with a fixed capacity of 67.2 L contains helium gas at the stop. The amount of heat needed to raise the temperature of the gas by 20°C is (given that R = 8.31 J mol^-1 K^-1)

1. 748 J
2. 700 J
3. 374 J
4. 350 J

Answer: 1. 748 J

Helium is monatomic, for which Cv = \(\frac{3}{2}\)R.

Number of moles = \(\frac{67.2 \mathrm{~L}}{22.4 \mathrm{~L}}\) = 3.

The amount of heat required is

⇒ \(\Delta Q=n C_V \Delta T=3 \mathrm{~mol}\left(\frac{3}{2} R\right) \Delta T\)

= \(\frac{9}{2}\) mol (8.31 J mol^-1 K^-1)(20 K)

= 747.9 J ≈ 748 J.

Question 41. A gas is taken from state A to state P B via two different paths, ACB and c ADB. When path ACB is used, 60 J of heat flows into the system and the system does 30 J of work. If the path ADB is used, work done by the system is 10 J. The heat which flows A into the system for the path ADB is

1. 80 J
2. 40 J
3. 100 J
4. 20 J

Answer: 2. 40 J

Internal energy is a state function and depends only on the initial and final states—it is path-independent.

From the first law of thermodynamics,

ΔQ = ΔU + ΔW

⇒ ΔU = ΔQ – ΔW.

For process ACB, ΔU = 60 J-30 J

= 30 J.

For process ADB, ΔU = ΔQ-10 J.

Since AU is the same for both,

ΔQ – 10J = 30J

⇒ ΔQ = 40J.

Question 42. A sample of an ideal gas is taken through a cyclic process abca as p shown in the figure. The change in the internal energy of the gas along the path ca is -180 J. The gas absorbs 250 J of heat along the path ab and 60 J along the path be. The work done by the gas along the 0L path abc is

1. 120 J
2. 140 J
3. 100 J
4. 130 J

Answer: 4. 130 J

Consider the values of ΔQ, ΔU, and ΔW given in the following table.

For the process b → c (isochoric),

ΔW = 0,

so, (ΔU)_bc = (dQ)_bc = 60J.

In the total cyclic process, ΔU = 0.

So,(ΔU)_ab + (ΔU)_bc + (ΔU)_ca = 0

=> (ΔU)_ab + 60J – 180J = 0

=> (ΔU)_ab = 120J.

For a → b, ΔW = ΔQ – ΔU

= 250 J-120 J

= 130 J.

∴ W_abc = W_ab + W_bc

= 130 J + 0

= 130 J.

Question 43. Two moles of helium gas is mixed with three moles of hydrogen molecules. The molar heat capacity of the mixture at constant volume is (given that = 8.3 J KT^-1 mol^-1)

1. 21.6 J mol^-1 K^-1
2. 15.7 J mol^-1 K^-1
3. 19.7 J mol^-1 K^-1
4. 17.4 J mol^-1 K^-1

Answer: 4. 17.4 J mol^-1 K^-1

Helium → monatomic \(\left(C_V=\frac{3}{2} R\right)\)

Hydrogen → diatomic \(\left(C_V=\frac{5}{2} R\right)\)

∴ \(\left(C_V\right)_{\text {mixture }}=\frac{n_1 C_{V_1}+n_2 C_{V_2}}{n_1+n_2}\)

⇒ \(\frac{2\left(\frac{3}{2} R\right)+3\left(\frac{5}{2} R\right)}{2+3}=\frac{21}{10} R\)

= \(\frac{21}{10}\)(8.3Jmol^-1K^-1)

= 17.43 J mol^-1 K^-1.

Question 44. A sample of n moles of an ideal gas with heat capacity at constant volume Cv undergoes an isobaric expansion by a certain volume. The ratio of the work done in the process to the heat supplied is

1. \(\frac{4 n R}{C_V+n R}\)
2. \(\frac{n R}{C_V+n R}\)
3. \(\frac{n R}{C_V-n R}\)
4. \(\frac{4 n R}{C_V-n R}\)

Answer: 2. \(\frac{n R}{C_V+n R}\)

Heat capacity of n moles of a gas at constant volume = nC_v-C_v (given).

Work done = ΔW = pΔV = nRΔT,

Heat absorbed = nC_pΔT = n(C_v + R)ΔT

⇒ (nC_v + nR)ΔT = (C₂ + nR)ΔT.

∴ ratio = \(\frac{\Delta W}{\Delta Q}=\frac{n R \Delta T}{\left(C_V+n R\right) \Delta T}=\frac{n R}{C_V+n R}\)

Question 45. Half a mole of an ideal monatomic gas is heated at a constant pressure of 1 atm from 20°C to 90°C. The work done by the gas is close to (take R = 8.31 J mol^-1 K^-1)

1. 291 J
2. 581 J
3. 146 J
4. 73 J

Answer: 1. 291 J

Work done by a gas is ΔW = pΔV = nRΔT.

Substituting the given values,

AW = (\(\frac{1}{2}\) mol) (8.31 J mol^-1K^-1)(70 K)

= 291 J

Question 46. A diatomic ideal gas undergoes an adiabatic process at room temperature. The relation between temperature and volume for this process is TVn = constant. The value of n is

1. \(\frac{2}{5}\)
2. \(\frac{3}{5}\)
3. \(\frac{5}{3}\)
4. \(\frac{2}{3}\)

Answer: 1. \(\frac{2}{5}\)

For an adiabatic process, TV^γ-1 = constant.

For a diatomic gas, \(C_V=\frac{5 R}{2}, C_p=\frac{7 R}{2}\)

∴ y = \(\frac{7}{5}\)

Given that TVⁿ = constant.

∴ \(n=\gamma-1=\frac{7}{5}-1=\frac{2}{5}\)

Question 47. Three moles’ of oxygen is mixed with 5 moles of argon, both at temperature T. The total internal energy of the mixture is

1. 15RT
2. 12RT
3. 19RT
4. 10RT

Answer: 1. 15RT

Oxygen is diatomic, meaning that f = 5, and argon is monatomic, implying f = 3.

Total internal energy \(U=\left(\frac{1}{2} k T\right)(3 N) 5+\left(\frac{1}{2} k T\right)(5 N) 3\)

= 15NkT

= 15JRT.

Question 48. The internal energy of 1 mol of a nonlinear triatomic molecule at temperature T is

1. \(\frac{9}{2}\) RT
2. \(\frac{3}{2}\) RT
3. 3RT
4. \(\frac{5}{2}\) RT

Answer: 3. 3RT

For a nonlinear triatomic molecule,f = 6.

∴ \(U=\left(\frac{1}{2} k T\right)(6 N)\)

= 3 RT

Question 49. 0.1 mol of a gas at 200 K is mixed with 0.05 mol of the same gas at 400 K. If the final temperature of the mixture is 10T₀, the value of T₀ is (in kelvin)

1. 20.44
2. 26.66
3. 22.44
4. 25.15

Answer: 2. 26.66

Conserving internal energy,

⇒ \(n_1 C_V T_1+n_2 C_V T_2=\left(n_1+n_2\right) C_V T_0\)

⇒ (0.1)(200 K) + (0.05)(400 K) = (0.15)(10T₀)

⇒ \(T_0=\frac{20 \mathrm{~K}+20 \mathrm{~K}}{0.15}\)

= 26.66 K

Question 50. The absorption of 160 J of heat by an ideal gas at constant pressure increases its temperature by50°C. For the same gas, the temperature rises by 100°C when 240 J of heat is absorbed at constant volume. The number of degrees of freedom of each of its molecules is

1. 5
2. 3
3. 6
4. 7

Answer: 3. 6

At constant pressure, ΔQ = nC_pΔT

⇒ 160 J = nC_p 50.

At constant volume, 240 J = nC_v .100

∴ \(\frac{C_p}{C_V}=\gamma\)

= \(\frac{160}{240} \times \frac{100}{50}\)

= \(\frac{4}{3}\)

But y =1 + \(\frac{2}{f}\)

⇒ f = 6.

Question 51. An ideal diatomic gas undergoes an adiabatic process in which its density is increased to 32 times its original value. If the pressure increases to n times its original value, the value of n will be

1. 4
2. 8
3. 64
4. 128

Answer: 4. 128

For an adiabatic process, pV^γ = p’V’^γ

⇒ \(p\left(\frac{M}{\rho}\right)^\gamma=p^{\prime}\left(\frac{M}{\rho^{\prime}}\right)^\gamma\)

⇒ \(p\left(\frac{1}{\rho}\right)^\gamma=(n p)\left(\frac{1}{32 \rho}\right)^\gamma\)

=> n = 32^γ

= (25)^7/5

= 128 [ ∵ y =\(\frac{7}{5}\)]

Question 52. A bullet of 5 g moving at 210 m s^-1 strikes a fixed wooden target. Half of its kinetic energy is converted into heat in the bullet while the remaining half is absorbed by wood. What is the rise in temperature of the bullet? (Given that the specific heat capacity of the bullet’s metal = 0.03 cal g^-1 °C^-1 and 1 cal = 4.2 J)

1. 83.3°C
2. 38.4°C
3. 87.5 °C
4. 110°C

Answer: 3. 87.5 °C

Kinetic energy, E = \(\frac{1}{2}\)mv²;

Heat absorbed = H = \({E}{2}\) = \(\frac{1}{4}\)mv²

= mcΔθ.

Hence, rise in temperature,

⇒ \(\Delta \theta=\frac{v^2}{4 c}\)

= \(\frac{\left(210 \mathrm{~m} \mathrm{~s}^{-1}\right)^2}{4\left(0.03 \times 4200 \mathrm{~J} \mathrm{~kg}^{-1}{ }^{\circ} \mathrm{C}^{-1}\right)}\)

= 87.5°C.

Question 53. A helium-filled balloon at 32°C and 1.7 atm suddenly burst. Immediately after it bursts, the expansion of helium can be considered as

1. Irreversible isothermal
2. Irreversible adiabatic
3. Reversible adiabatic
4. Reversible isothermal

Answer: 2. Irreversible adiabatic

An isothermal process is slow but bursting is fast and sudden. Hence, the bursting of the balloon is an irreversible adiabatic process.

Heat Engine Refrigerator

Question 1. The efficiency of a Carnot engine operating between the temperatures of 100°C and -23°C will be

1. \(\frac{100-23}{273}\)
2. \(\frac{100+23}{373}\)
3. \(\frac{100+23}{100}\)
4. \(\frac{100-23}{100}\)

Answer: 2. \(\frac{100+23}{373}\)

Temperature of source = T₁ = (273 + 100) K

= 373 K,

Temperature of sin k = T₂ = (273 – 23 K)

= 250 K.

The efficiency of the Carnot engine is

⇒ \(\eta=1-\frac{T_2}{T_1}\)

= \(\frac{T_1-T_2}{T_1}\)

= \(\frac{123}{373}\)

= \(\frac{100+23}{373}\)

Question 2. An engine takes heat from a reservoir and converts \(\frac{1}{6}\) of it into work. If the temperature of the sink is reduced by 62 °C, the efficiency of the engine becomes double. The temperatures of the source and sink must be

1. 90°C, 37°C
2. 99°C,37°C
3. 372°C,37°C
4. 206°C, 37°C

Answer: 2. 99°C,37°C

Efficiency = \(\frac{\text { output }}{\text { input }}=\frac{\text { work done }(W)}{\text { heat absorbed }(Q)}\)

⇒ \(1-\frac{T_2}{T_1}=\frac{1}{6}\)…..(1) [∵ W = \(\frac{Q}{6}\)]

When the temperature T₂ of the sink is reduced by 62°C, the new temperature T₂ = T₂– 62, and

⇒ \(\eta^{\prime}=1-\frac{T_2^{\prime}}{T_1}\)

= \(2 \eta=2\left(\frac{1}{6}\right)\)

= \(\frac{1}{3}\)

⇒ \(1-\left(\frac{T_2-62}{T_1}\right)\)

= \(\frac{1}{3}\)…(2)

From(1), \(\frac{T_2}{T_1}=\frac{5}{6}\) and from(2), \(\frac{T_2}{T_1}=\frac{2}{3}+\frac{62}{T_1}\)

Equating \(\frac{T_2}{T_1}\) as mentioned above, \(\frac{5}{6}=\frac{2}{3}+\frac{62}{T_1}\)

T₁ = 372K

= 99°C,

and \(T_2=\frac{5}{6} T_1\)

= 310K

= 37°C.

∴ The required temperatures are 99 °C and 37°C.

Question 3. The temperatures of the source and sink of a heat engine are 127°C and 27°C respectively. A technician claims its efficiency to be 26%.

1. It is impossible.
2. It is possible with high probability.
3. It is possible with low probability.
4. The data is insufficient.

Answer: 1. It is impossible.

Given that T₁ = (273 + 127) K

= 400 K

T₂ = (273 + 27) K

= 300 K.

The efficiency of an ideal engine (Carnot engine),

⇒ \(\eta=1-\frac{T_2}{T_1}\)

= \(1-\frac{300}{400}\)

= \(\frac{1}{4}\)

= 25%

No engine can have an efficiency more than that of a Carnot engine.

Hence, the claim of 26% efficiency is impossible.

Question 4. The efficiency of a Carnot engine is 50% and the temperature of the sink is 500 K. If the temperature of the source is kept constant and the efficiency of the engine is to be raised to 60% then the required temperature of the sink will be

1. 600 K
2. 500 K
3. 400 K
4. 100 K

Answer: 3. 400 K

Efficiency \(\eta=1-\frac{T_2}{T_1}\)

Given that T₂ = 500 K and \(\eta=50 \%=\frac{1}{2} \Rightarrow \frac{1}{2}=1-\frac{500}{T_1}\)

hence T₁ = 1000 K.

For 60% efficiency, let the temperature of the sink be T₂

⇒ \(\frac{60}{100}=1-\frac{T_2^{\prime}}{T_1}=1-\frac{T_2^{\prime}}{1000}\)

∴ T₂ = 400 K

Question 5. An ideal gas heat engine operates as a Carnot cycle between 227°C and 127°C. It absorbs 6 kcal of heat at a higher temperature. The amount of heat (in kcal) converted into work is equal to

1. 1.6
2. 1.2
3. 4.8
4. 3.5

Answer: 2. 1.2

Temperature of source = T₁ = (227 + 273) K

= 500 K.

Temperature ofsink = T₂ = (127 + 273) K

= 400 K.

∴ efficiency \(\eta=1-\frac{T_2}{T_1}\)

= \(1-\frac{400}{500}\)

= \(\frac{1}{5}\)

But \(\eta=\frac{\text { output }(W)}{\text { input }(Q)}\)

= \(\frac{W}{6 \mathrm{kcal}}\)

= \(\frac{1}{5}\)

∴ work W = \(\frac{6kcal}{5}\)

= 1.2 kcal

Question 6. An engine has an efficiency of \(\frac{1}{6}\) When the temperature of the sink is reduced by 62°C, its efficiency is doubled. The temperature of the source is

1. 120°C
2. 35°C
3. 99°C
4. 65°C

Answer: 3. 99°C

Let T₁ = temperature of the source.

Given that efficiency n = \(\frac{1}{6}\)

⇒ \(\eta=1-\frac{T_2}{T_1} \Rightarrow \frac{1}{6}=1-\frac{T_2}{T_1}\)….(1)

When T₂ is reduced to T₂– 62, efficiency gets doubled. So,

⇒ \(\frac{2}{6}=1-\frac{T_2-62}{T_1}\)…..(2)

Solving (1) and (2), T₁ = 372 K = 99°C.

Question 7. A Carnot engine whose sink is at 300 K has an efficiency of 40%. By how much should the temperature of the source be increased so as to increase its efficiency by 50% of the original efficiency?

1. 250 K
2. 370 K
3. 270 K
4. 390 K

Answer: 1. 250 K

Efficiency of Carnot engine is \(\eta=1-\frac{T_2}{T_1}\)

Given that \(\eta=40 \%=\frac{4}{10}\), the temperature of sink = T₂ = 300 K.

Temperature of source = T₁.

∴ \(\frac{4}{10}=1-\frac{300}{T_1}\)

⇒ T₁ = 500 K

Let the temperature T₁ of the source be increased by ΔT₁ so that the increased efficiency becomes

⇒ \(\eta^{\prime}=40 \%+50 \% \text { of } \eta=\frac{40}{100}+\frac{50}{100} \times \frac{40}{100}=\frac{60}{100}\)

Hence,

⇒ \(\eta^{\prime}=\frac{60}{100}\)

= \(1-\frac{T_2}{T_1+\Delta T_1}\)

⇒ \(\frac{60}{100}=1-\frac{300}{500+\Delta T_1}\)

⇒ \(\Delta T_1=250 \mathrm{~K}\)

Question 8. Two Carnot engines A and B are operated in series. Engine A receives heat from the source at temperature T₁, and rejects heat to the sink at temperature T. The second engine receives heat at temperature T and rejects a part of it to its sink at temperature T₂. For what value of T are the efficiencies of the two engines equal?

1. \(\frac{T_1+T_2}{2}\)
2. \(\frac{T_1-T_2}{2}\)
3. \(\sqrt{T_1 T_2}\)
4. \(\frac{\sqrt{T_1 T_2}}{2}\)

Answer: 3. \(\sqrt{T_1 T_2}\)

For Carnot engine A, efficiency is \(\eta_{\mathrm{A}}=1-\frac{T}{T_1}\) and for Carnot engine B,

it is \(\eta_{\mathrm{B}}=1-\frac{T_2}{T}\)

Given that \(\eta_{\mathrm{A}}=\eta_{\mathrm{B}}\)

⇒ \(1-\frac{T}{T_1}=1-\frac{T_2}{T}\)

⇒ \(\frac{T}{T_1}=\frac{T_2}{T}\)

Hence, \(T=\sqrt{T_1 T_2}\)

Question 9. The coefficient of performance of a refrigerator is 5. If the temperature inside the freezer is -20°C, the temperature of the surroundings to which it rejects heat is

1. 42 °C
2. 31 °C
3. 21 °C
4. 15°C

Answer: 2. 31 °C

The coefficient of performance (k) of a refrigerator is expressed as

⇒ \(k=\frac{T_2}{T_1-T_2}\)

where T₂ = temperature of the refrigerated space,

and T₁ = temperature of surroundings.

Given that T₂ = -20°C = 253 K.

For k = 5,

⇒ \(5=\frac{253}{T_1-253}\)

⇒ \(T_1=303.6 \mathrm{~K} \approx 31^{\circ} \mathrm{C}\)

Question 10. A Carnot engine having an efficiency of \(\frac{1}{10}\) as a heat engine is used as a refrigerator. If the work done on the system is 10 J, the amount of energy absorbed from the reservoir at a lower temperature is

1. 110 J
2. 90 J
3. 95 J
4. 10 J

Answer: 2. 90 J

When used as a Carnot engine, efficiency \(\eta=1-\frac{T_2}{T_1}\)

Given that n = \(\frac{1}{10}\)

Hence, \(\frac{T_2}{T_1}=\frac{9}{10}\)……(1)

When used as a refrigerator, its coefficient of performance is

⇒ heat extracted from the reservoir (Q₂) / work done on the system

Question 11. A refrigerator works between 4°C and 30°C, It is required to remove 600 calories of heat every second in order to keep the temperature of the refrigerated space constant. The power required is

1. 24.65 W
2. 236.5 W
3. 230 W
4. 2.365 W

Answer: 2. 236.5 W

For the refrigerator, T₂ = (4 + 273) K = 277 K.

T₁ = (30 + 273) K

= 303 K.

The coefficient of performance is

⇒ \(\frac{Q_2 \text { (heat absorbed) }}{W(\text { Work done })}=\frac{Q_2}{Q_1-Q_2}\)

= \(\frac{1}{\frac{Q_1}{Q_2}-1}\)

⇒ \(\frac{Q_2}{W}=\frac{1}{\frac{T_1}{T_2}-1}\)

= \(\frac{T_2}{T_1-T_2}\)

Given that Q₂ = 600 cal s^-1 = 600 x 4.2 J s^-1.

∴ required power is,

⇒ \(W=Q_2 \frac{\left(T_1-T_2\right)}{T_2}\)

= \(\frac{\left(600 \times 4.2 \mathrm{~J} \mathrm{~s}^{-1}\right)(26 \mathrm{~K})}{(277 \mathrm{~K})}\)

= 236.5 W.

Question 12. The temperature inside a refrigerator is t₂°C and the room temperature is t₁°C. The amount of heat delivered to the room for each joule of electrical energy consumed ideally will be

1. \(\frac{t_1+t_2}{t_1+273} \mathrm{~J}\)
2. \(\frac{t_1+273}{t_1-t_2} \mathrm{~J}\)
3. \(\frac{t_1}{t_1-t_2} \mathrm{~J}\)
4. \(\frac{t_1+273}{t_1-273} \mathrm{~J}\)

Answer: 2. \(\frac{t_1+273}{t_1-t_2} \mathrm{~J}\)

For a refrigerator, the ratio

⇒ \(\frac{Q_1 \text { (heat delivered to the room) }}{Q_2 \text { (heat absorbed) }}=\frac{T_1}{T_2}\)

But Q₁– Q₂ = W, so Q₂ = Q₁– W, where W = electrical energy is consumed.

∴ \(\frac{Q_1}{Q_1-W}=\frac{T_1}{T_2} \Rightarrow \frac{1}{1-\frac{W}{Q_1}}=\frac{T_1}{T_2} \Rightarrow Q_1=\frac{W T_1}{T_1-T_2}\)

Given that T₁ = 273 + t₁, T₂ = 273 + t₂, W = 1 J.

∴ \(Q_1=\frac{(1 \mathrm{~J})\left(273+t_1\right)}{\left(t_1-t_2\right)}=\frac{273+t_1}{t_1-t_2} \mathrm{~J}\)

Question 13. Determine the efficiency of a Carnot engine if, during its adiabatic expansion, the volume is increased to 3 times the initial volume and y = 1.5.

1. \(1-\frac{1}{\sqrt{2}}\)
2. \(1-\frac{1}{\sqrt{3}}\)
3. \(1-\frac{1}{\sqrt{2}}\)
4. \(1-\frac{1}{\sqrt{3}}\)

Answer: 2. \(1-\frac{1}{\sqrt{3}}\)

During the adiabatic expansion,

⇒ \(T_1 V_1^{\gamma-1}=T_2 V_2^{\gamma-1}\)

⇒ \(\frac{T_2}{T_1}=\left(\frac{V_1}{V_2}\right)^{\gamma-1}\)

⇒ \(\frac{T_2}{T_1}=\left(\frac{1}{3}\right)^{1.5-1}\)

= \(\frac{1}{\sqrt{3}}\)

Hence, efficiency is \(\eta=1-\frac{T_2}{T_1}=1-\frac{1}{\sqrt{3}}\)

Question 14. In a refrigerator, the heat absorbed from the source is 800 J and the heat supplied to the sink is 500 J. The coefficient of performance is

1. \(\frac{5}{8}\)
2. \(\frac{8}{5}\)
3. \(\frac{5}{3}\)
4. \(\frac{3}{5}\)

Answer: 3. \(\frac{5}{3}\)

The coefficient of performance of a refrigerator is defined as

⇒ \(k=\frac{\text { heat absorbed }\left(Q_2\right)}{\text { work done on the coolant }(W)}\)

⇒ \(\frac{Q_2}{W}=\frac{Q_2}{Q_1-Q_2}\)

Given that Q₁ = 800J,

Q₂ = 500J,

Hence k = \(\frac{5}{3}\)

Question 15. A Carnot engine works between 27°C and127°C. The heat supplied by the source is 500 J. The heat delivered to the sink is

1. 100 J
2. 667 J
3. 375 J
4. 500 J

Answer: 3. 375 J

In a Carnot engine,

⇒ \(\frac{Q_2}{Q_1}=\frac{T_2}{T_1}\)

= \(\frac{27+273}{127+273}\)

= \(\frac{3}{4}\)

∴ heat delivered to the sink,

Q₂ = \(\frac{3}{4}\)

Q₁ = \(\frac{3}{4}\) x 500J

= 375J.

Question 16. A Carnot engine has an efficiency of \(\frac{1}{6}\). When the temperature of the sink is reduced by 62°C, its efficiency is doubled. The temperatures of the source and the sink are respectively

1. 99°C, 37°C
2. 124°C, 62°C
3. 37°C, 99°C
4. 62°C, 124°C

Answer: 1. 99°C,37°C

The efficiency of the Carnot engine is

⇒ \(\eta=\frac{1}{6}=1-\frac{T_2}{T_1}\)

⇒ \(\frac{T_2}{T_1}=\frac{5}{6}\)

When T₂ is reduced by 62°C, the efficiency becomes

⇒ \(\eta^{\prime}=2 \eta\)

= \(\frac{1}{3}\)

= \(1-\frac{T_2-62}{T_1}\)

⇒ \(\frac{T_2-62}{T_1}=\frac{2}{3}\)

Dividing (2) by (1),

⇒ \(\frac{T_2-62}{T_2}=\frac{2}{3} \times \frac{6}{5}\)

= \(\frac{4}{5}\)

=> 5T₂ – 310 = 4T₂

=> T₂ = 310 K

= (310- 273) °C

= 37°C.

⇒ \(T_1=\frac{6}{5} T_2\)

= \(\frac{6}{5}(310 \mathrm{~K})\)

= 372 K

= (372- 273) °C

= 99°C

Question 17. TwoCarnotenginesAand B areoperatedinseries. EngineAreceives heat at T₁ (= 600 K) and rejects it to a reservoir at temperature T₂. Engine B receives heat rejected by Engine A and in turn, rejects heat to a heat reservoir at T₃ (= 400 K). If the work outputs by the two engines are equal, the temperature T₂ is equal to

1. 600 K
2. 500 K
3. 400 K
4. 300 K

Answer: 2. 500 K

The diagram shows the working of Carnot engines A and B in series.

For A: Q₁ = Q₂ + W₁,

So, W₁ = Q₁– Q₂.

For B: Q₂ = Q₃ + W₂,

So W₂ = Q₂-Q₃.

Given that work, the output is the same for both A and B, hence W₁ = W₂

Q₁ – Q₂ = Q₂-Q₃

=> Q₁ + Q₃ = 2Q₂

⇒ \(\frac{Q_1}{Q_2}+\frac{Q_3}{Q_2}=2\)

⇒ \(\frac{T_1}{T_2}+\frac{T_3}{T_2}=2\)

Substituting, \(\frac{600 \mathrm{~K}}{T_2}+\frac{400 \mathrm{~K}}{T_2}=2\)

⇒ T₂ = 500 K.

Sound Waves Synopsis

• General Equations Of Wave Motion:
  • In the positive y = f(vt- x); and in the negative r-direction, y = f(vt + x).
  • Here v = velocity of wave propagation, x = position, and t = time at which the function y (displacement) is measured.
  • In the sinusoidal form, y = Asin(ωt ± kx), where A = amplitude (maximum value of the periodic function), ω = angular frequency = \(\frac{2 \pi}{T}=2 \pi f\), T = time period (in s), and f = frequency
• Particle Velocity And Slope: The particle velocity is given by
  \(v_{\text {particle }}=\frac{d y}{d t}=A \omega \cos (\omega t \pm k x)=v_{\max } \cos (\omega t \pm k x)\)
  The slope of a waveform is \(\frac{d y}{d x}= \pm A k \cos (\omega t \pm k x)\)
  Thus, \(v_{\text {particle }}=v_{\text {wave }} \cdot \mid \text { slope } \mid\)
• The resultant amplitude A with a phase difference Φ is given by
  \(A^2=A_1^2+A_2^2+2 A_1 A_2 \cos \phi\)
  • For maxima, \(\phi=\left(\frac{2 \pi}{\lambda}\right) x= \pm 2 n \pi\)
  • For minima, \(\phi=\left(\frac{2 \pi}{\lambda}\right) x= \pm(2 n+1) \pi\)
• Standing waves are produced by the superposition of identical waves traveling in opposite directions.
  y = A sin(ωt – kx) + A sin(ωt + kx)
  = 2A sin ωt cos kx
  = (2A cos kx) sin ωf.
  In a standing wave, all particles execute an SHM about their mean position with die same frequency but with the amplitude A(x) = 2A cos kx, which is position-dependent
• Transverse Vibrations Of A String:
  Velocity of wave = \(v=\sqrt{\frac{F}{\mu}}\) = where F = tension in string (in N) and
  μ = mass per unit length (in kg m^-1).
• Modes Of Vibrations In A Stretched String:
  • Fundamental mode = first harmonic: \(f_1=\frac{1}{2 l} \sqrt{\frac{F}{\mu}}\)
  • First overtone = second harmonic: \(f_2=\frac{2}{2 l} \sqrt{\frac{F}{\mu}}=2 f_1\)
  • Second overtone = third harmonic: \(f_3=\frac{3}{2 l} \sqrt{\frac{F}{\mu}}=3 f_1\)
  • (p-l)th overtone = pth harmonic: \(f_{\mathrm{P}}=\frac{p}{2 l} \sqrt{\frac{F}{\mu}}=p f_1\)

• Speed Of Sound Waves In A Gaseous Medium:
  \(\hat{v}=\sqrt{\frac{\gamma p}{\rho}}, \text { where } \gamma=\frac{c_p}{c_v}\), where \(\gamma=\frac{c_p}{c_v}\), p = pressure and p = density = \(\frac{M}{V}\)
  Hence, \(v=\sqrt{\frac{\gamma p V}{M}}=\sqrt{\frac{\gamma R T}{M_0}}\), where M₀ = molar mass.
• Sound Waves Are Pressure Wives: The excess pressure as a function
  of x and t is
  p = p₀cos (ωt- kx), where p₀ = pressure amplitude.
  Instanding waves, die pressure nodes and displacement antinodes are coincident.
• The Vibration Of An Air Column (organ pipe):
  • Close Organ Pipe: In a closed pipe, the open end is the pressure node as well as the displacement antinode.

The Following Are The Modes Of Vibrations In A Closed Pipe:

• Fundamental Or First Harmonic: f₁ = \(\frac{v}{4l}\)
• First Overtone Or Third Harmonic: \(f_3=3\left(\frac{v}{4 l}\right)=3 f_1\)
• Second Overtone Or Fifth Harmonic: \(f_5=5\left(\frac{v}{4 l}\right)=5 f_1\)

• Open Organ Pipe:
• The Modes Of Vibrations In An Open Pipe Are As Follows:
  • Fundamental Or First Harmonic: \(f_1=\frac{v}{2 l}\)
  • First Overtone Or Second Harmonic: \(f_2=2\left(\frac{v}{2 l}\right)=2 f_1\)
  • Second Overtone Or Third Harmonic: \(f_3=3\left(\frac{v}{2 l}\right)=3 f_1\)
• Note that, in a closed pipe only odd harmonics are present, while an open pipe contains all the harmonics. The richness of overtones in an open pipe makes the note melodious.
• Doppler Effect: The apparent change in frequency due to the relative motion between the source of waves and the receiver is called the Doppler effect. A decrease in separation leads to an apparent increase in frequency. The general equation for the apparent frequency is
  \(f^{\prime}=\left(\frac{v \pm v_0}{v \pm v_s}\right) f\)
• where v = velocity of the sound wave, v₀ = velocity of the observer, v_s = velocity of the source,f = true frequency, and f’ = apparent frequency.
• Beats: Beats are the rhythmic variation of loudness at a point due to the superposition of waves having a small difference in their frequencies. This may be regarded as an interference in time (with the path difference fixed).
• Beat frequency = difference in frequencies.
• The Intensity Of Sound Waves: It is the amount of energy passing through a unit area per unit of time perpendicular to the area element. Thus, intensity = \(I=\frac{\Delta U}{\Delta A \Delta t}\) (SI Unit: W m^-2), and it is proportional
• to the square of pressure amplitude.
• Loudness: It is what we perceive as the volume of a sound.
• The loudness level (β) is defined by the relation
• \(\beta=\log \frac{I}{I_0} B=10 \log \frac{I}{I_0} \mathrm{~dB}\)
• The minimum intensity (l₀) which is audible to the normal human ear is I₀ = 10^-12W m^-2.

Sound Waves

Wave Equation:

Question 1. A wave travelling in the positive having displacement along the y-direction with amplitude 1 m, wavelength 2π m and frequency of \(\frac{1}{\pi}\) Hz is represented by

1. y = sin(10πx- 20nt)
2. y = sin(2πx + 2nt)
3. y = sin(x-2t)
4. y = sin (2πx- 2nt)

Answer: 3. y = sin(x-2t)

The standard equation of a progressive wave travelling along the +ve x-direction is given by

y = A sin(kx – ωt),

where A = displacement amplitude (=1 m),

⇒ \(k=\frac{2 \pi}{\lambda}\) = angular wavenumber \(\left(=\frac{2 \pi}{(2 \pi) m}\right)\), and

ω = angular frequency \(\left[=\frac{2 \pi}{T}=2 \pi f=2 \pi\left(\frac{1}{\pi} \mathrm{Hz}\right)\right]\)

∴ y = (1 m) sin(x-2t)

= sin(x- 2t).

Question 2. The equation of a simple harmonic wave is given by y = 3 sin \(\frac{\pi}{2}\) (50t- x), where x and y are in metres and t is in seconds. The ratio of maximum particle velocity to the wave velocity is

1. 2π
2. \(\frac{3}{2}\)n
3. 3π
4. \(\frac{2}{3}\)n

Answer: 2. \(\frac{3}{2}\)n

For a wave equation, y = Asin (ωt-kx),

particle velocity = \(\frac{d y}{d t}=A \omega \cos (\omega t-k x) \Rightarrow\left(v_{\text {particle }}\right)_{\max }=A \omega\)

Wave velocity = \(f \lambda=\frac{\frac{2 \pi}{T}}{\frac{2 \pi}{\lambda}}=\frac{\omega}{k}\)

In the given equation, \(y=3 \sin \left(25 \pi t-\frac{\pi}{2} x\right)\)

A = 3m, ω = 25n and \(k=\frac{\pi}{2}\)

∴ particle, \(\frac{\left(v_{\text {particle }}\right)_{\max }}{v_{\text {wave }}}=\frac{A \omega}{\omega / k}=A k=(3 \mathrm{~m})\left(\frac{\pi}{2} \mathrm{~m}^{-1}\right)=\frac{3 \pi}{2}\)

Question 3. Two waves are represented by the equations y₁ = asin (ωt + kx + 0.57) and y₂ = acos (ωt + kx), where x is in metres and f is in seconds. The phase difference between them is

1. 1.0 rad
2. 1.25 rad
3. 1.57 rad
4. 0.57 rad

Answer: 1. 1.0 rad

Given, y₁ = asin(ωf+ kt + 0.57) and

⇒ \(y_2=a \cos (\omega t+k x)=a \sin \left(\omega t+k x+\frac{\pi}{2}\right)\)

phase difference = \(\phi=\frac{\pi}{2}\) – 0.57

= 1.57 – 0.57

= 1.0 rad

Question 4. A transverse wave is represented by y = Asin(ωt – kx). For what value of the wavelength is the wave velocity equal to the maximum particle velocity?

1. \(\frac{\pi}{2}\)A
2. πA
3. 2πA
4. A

Answer: 3. 2πA

The given wave equation is y = Asin(ω- kx), wave velocity = \(\frac{ω}{k}\), and maximum particle velocity = Aω.

Since both velocities are given as equal,

⇒ \(\frac{ω}{k}\) = Aω

⇒ \(\frac{\lambda}{2 \pi}=A\)

⇒ λ = 2πA

Question 5. A wave is described by y = 0.25sin (10πx- 2πt), where x and y are in metres and t is in seconds. The wave is travelling along

1. +x-direction with frequency 1 Hz and wavelength λ = 0.2 m
2. -x-direction with amplitude 0.25 m and wavelength λ = 0.2 m
3. -x-direction with frequency 1 Hz
4. +x-direction with frequency n Hz and wavelength λ = 0.2 m

Answer: 1. +x-direction with frequency 1 Hz and wavelength λ = 0.2 m

Comparing the given equation y = (0.25 m)sin(10πr – 2πt) with the standard wave equation travelling along the +ve x-direction,

y= Asin(kx-ωt),

we conclude that

(1) the wave is travelling along the +x-direction,

(2) \(k=\frac{2 \pi}{\lambda}\) =10K, λ = 0.2m, and

(3) ω = 2πf = 2π, f = 1 Hz.

Question 6. A transverse wave propagating along the x-axis is represented by \(y(x, t)=8.0 \sin \left(0.5 \pi x-4 \pi t-\frac{\pi}{4}\right)\) where x is in metres and t is in seconds. The speed of the wave is

1. 4k m s^-1
2. 0.5k m s^-1
3. 8 ms^-1
4. – m s^-1

Answer: 3. 8 ms^-1

Given, y = 8.0sin\(\left(0.5 \pi x-4 \pi t-\frac{\pi}{4}\right)\), where k = \(\frac{2 \pi}{\lambda}\) = 0.5π, and

ω = 2πf

= 4π.

∴ speed r of the wave is v = \(\frac{\omega}{k}=\frac{4 \pi}{0.5 \pi}\)

= 8 m s^-1.

Question 7. A wave is travelling along the positive x-direction with a = 0.2 m, velocity = 360 m s^-1 and λ = 60 m. The correct expression for the wave motion is

1. \(y=0.2 \sin 2 \pi\left(6 t+\frac{x}{60}\right)\)
2. \(y=0.2 \sin \pi\left(6 t+\frac{x}{60}\right)\)
3. \(y=0.2 \sin 2 \pi\left(6 t-\frac{x}{60}\right)\)
4. \(y=0.2 \sin \pi\left(6 t-\frac{x}{60}\right)\)

Answer: 3. \(y=0.2 \sin 2 \pi\left(6 t-\frac{x}{60}\right)\)

Given that amplitude fl = 0.2m and velocity = \(\frac{ω}{k}\) = 360 m s^-1.

λ = 60m

⇒ \(k=\frac{2 \pi}{60} \mathrm{~m}^{-1}\),

So, \(\omega=360 \times \frac{2 \pi}{60}\)

= 12π.

∴ the required wave equation is

y = asin(ωt-kx)

⇒ \(0.2 \sin \left(12 \pi t-\frac{2 \pi}{60} x\right)\)

⇒ \(0.2 \sin 2 \pi\left(6 t-\frac{x}{60}\right)\)

Question 8. The equation of a progressive wave is given by \(y=4 \sin \left[\pi\left(\frac{t}{5}-\frac{x}{9}\right)+\frac{\pi}{6}\right]\) where x, y are in centimetres and t is in seconds. Which of the following is correct?

1. a = 0.04 cm
2. λ = 18 cm
3. f = 50Hz
4. v = 5 cm s^-1

Answer: 2. λ = 18 cm

In the equation \(y=4 \sin \left(\frac{\pi t}{5}-\frac{\pi}{9} x+\frac{\pi}{6}\right)\), x and y are in centimetres and t is in seconds.

∴ amplitude = a = 4.0 cm.

⇒ \(\omega=\frac{2 \pi}{T}=\frac{\pi}{5}\)

⇒ \(f=\frac{1}{10} \mathrm{~s}^{-1}\)

⇒ \(k=\frac{2 \pi}{\lambda}\)

= \(\frac{\pi}{9}\)

⇒ wavelength= y =18cm

Question 9. The equation of a progressive wave is given by y = 5sin (100πt- 0.4πλ), where x and y are in metres and t is in seconds.

(1) The amplitude of the dying wave is 5 m.
(2) The wavelength of the wave is 5 m.
(3) The frequency of the wave is 50 Hz.
(4) The velocity of the wave is 250 m s^-1.

Which of the above statements is correct?

1. (1), (2) and (3)
2. (2) and (3)
3. (1) and (4)
4. All are correct

Answer: 4. All are correct

In the equation y= 5 sin(100πt – 0.4πλ), with x and y in metres, J we have

amplitude = 5m, ω = 2πf = 100π,f = 50Hz,

⇒ \(k=\frac{2 \pi}{\lambda}=0.4 \pi, \lambda=\frac{2}{0.4}\) = 5 m.

∴ velocity = \(\partial=\frac{\omega}{k}=\frac{100 \pi}{0.4 \pi}\)

= 250m s^-1.

Question 10. The graph between wavenumber λ and angular frequency ω is

Answer: 1.

Wavenumber \(\bar{\lambda}=\frac{1}{\lambda}=\frac{f}{v}=\frac{2 \pi f}{2 \pi v}=\frac{\omega}{2 \pi v}\)

∴ \(\omega=(2 \pi 0) \bar{\lambda}\)

Thus the equation connecting ω and \(\bar{\lambda}\). is of the form y= mx which is a straight line passing through the origin as shown in option (1).

Question 11. The wave equation is expressed as \(y=10 \sin \left(\frac{2 \pi t}{30}+\alpha\right)\). If the displacement is 5 cm at t = 0 then the total phase at t = 7.5 s will be

1. \(\frac{2 \pi}{3} \mathrm{rad}\)
2. \(\frac{\pi}{3} \mathrm{rad}\)
3. \(\frac{\pi}{2} \mathrm{rad}\)
4. \(\frac{2 \pi}{5} \mathrm{rad}\)

Answer: 1. \(\frac{2 \pi}{3} \mathrm{rad}\)

In the equation \(y=10 \sin \left(\frac{2 \pi t}{30}+\alpha\right)\), y = 5 cm at t = 0.

Thus,

⇒ \(5 \mathrm{~cm}=10 \mathrm{~cm} \sin \left(\frac{2 \pi}{30} \cdot 0+\alpha\right)\)

⇒ \(\sin \alpha=\frac{1}{2}\)

Hence, \(\alpha=30^{\circ}=\frac{\pi}{6}\)

∴ total phase angle at t = 7.5s is

⇒ \(\phi=\frac{2 \pi}{30}(7.5)+\frac{\pi}{6}=\frac{\pi}{2}+\frac{\pi}{6}=\frac{2 \pi}{3} \mathrm{rad}\)

Question 12. The equations of two progressive waves are given by \(y_1=a \sin \left(\omega t+\phi_1\right) \text { and } y_2=a \sin \left(\omega t+\phi_2\right)\). If the amplitude and time period of the resultant wave are the same as those of the component waves then \(\left(\phi_1-\phi_2\right)\) is

1. \(\frac{\pi}{3}\)
2. \(\frac{2 \pi}{3}\)
3. \(\frac{\pi}{6}\)
4. \(\frac{\pi}{4}\)

Answer: 2. \(\frac{2 \pi}{3}\)

The equations of component waves are y₁ = asin(ωt + Φ₁) and y₂ = asin(ωt + Φ₂).

The superposition leads to the resultant displacement y = y₁ + y₂, in which die results in an amplitude A = a

=> A² = a² + a² +2a. acosΦ

=> a² = 2a²(1 + cos Φ)

∴ \(\left(\phi_1-\phi_2\right)=\phi\)

= \(120^{\circ}=\frac{2 \pi}{3}\)

Question 13. A progressive wave is expressed by y = 2.0 cos 2π(10t-8 x 10^-3x+ 0.45), where x and y are in centimetres and t is in seconds. The phase difference between two points separated by a distance of 4m is

1. 1.2K rad
2. 3.2K rad
3. 6.4K rad
4. \(\frac{2 \pi}{3} \mathrm{rad}\)

Answer: 3. 6.4K rad

In the given equation,

y = 2.0cos2π(10f- 8 x 10^-3x + 0.45).

∴ ω = 20π and 16π x 10-3 = k = \(\frac{2 \pi}{\lambda}\)

y = 8 x 10^-3 cm.

Phase difference= Φ = \(\frac{2 \pi}{\lambda}\) (path difference)

= (167t x 10^-3 cm^-1)(400 cm)

= 6.4n rad.

Question 14. The equation \(y=A \cos ^2\left(2 \pi f t-\frac{2 \pi}{\lambda} x\right)\) represents a wave in which

1. Amplitude = A, frequency = f and wavelength = λ
2. Amplitude = A, frequency = 2f and wavelength = 2λ
3. Amplitude = \(\frac{A}{2}\), frequency = 2f and wavelength = λ
4. Amplitude = \(\frac{A}{2}\), frequency = 2f and wavelength = \(\frac{λ}{2}\)

Answer: 4. Amplitude = \(\frac{A}{2}\), frequency = 2f and wavelength = \(\frac{λ}{2}\)

For the given equation

⇒ \(y=A \cos ^2\left(2 \pi f t-\frac{2 \pi}{\lambda} t\right)\)

⇒ \(\frac{A}{2} 2 \cos ^2\left(2 \pi f t-\frac{2 \pi}{\lambda} t\right)\)

⇒ \(\frac{A}{2}\left[1+\cos 2\left(2 \pi f t-\frac{2 \pi}{\lambda} t\right)\right]\)

⇒ \(\frac{A}{2}+\frac{A}{2} \cos \left[2 \pi(2 f) t-\frac{2 \pi}{\frac{\lambda}{2}} t\right]\)

Thus, amplitude = \(\frac{A}{2}\), frequency= 2f and wavelength = \(\frac{\lambda}{2}\)

Question 15. A progressive wave travelling along the positive is represented by y(x, t) = Asin(kx- ωt + Φ). Its amplitude at x = 0 is given in the figure. For this wave the initial phase is

1. π
2. \(\frac{\pi}{2}\)
3. –\(\frac{\pi}{2}\)
4. 0

Answer: 1. π

Given that y = Asin(kx- ωt + Φ ).

Slope of the waveforms, \(\frac{d y}{d x}=A k \cos (k x-\omega t+\phi)\)

At t = 0, \(\frac{d y}{d x}=A k \cos (k x+\phi)\)

Since the slope is negative, = π.

Question 16. A travelling harmonic wave is represented by the equation y(x, t) = 10^-3 sin(50t + 2x), where x and y are in metres and t is in seconds. Which of the following statements is correct?

1. The wave is propagating along the negative x-axis with a speed of 25 ms^-1.
2. The wave is propagating along the positive x-axis with a speed of 25 ms^-1.
3. The waves propagate along the positive x-axis with a speed of 100 ms^-1.
4. The wave is propagating along the negative x-axis with a speed of 100 ms^-1.

Answer: 1. The wave is propagating along the negative x-axis with a speed of 25 ms^-1.

The equation of a harmonic wave travelling along the negative x-axis is given by y = Asin (ωt + kx).

Comparing this with the given equation y = 10^-3 sin(50t + 2x), we conclude that the wave represented by this equation is travelling

along the negative x-axis with speed v = \(\frac{\omega}{k}=\frac{50 \mathrm{~s}^{-1}}{2 \mathrm{~m}^{-1}}\)

= 25 m s^-1.

Doppler Effect:

Question 17. Two cars moving in opposite directions approach each other with speeds of 22 m s^-1 and 16.5 m s^-1 respectively. The driver of the first car blows a horn having a frequency of 400Hz. The frequency heard by the driver of the second car is (velocity of sound = 340 m s^-1)

1. 361 Hz
2. 411 Hz
3. 448 Hz
4. 350 Hz

Answer: 3. 448 Hz

When the source (v_s) and observer (v_o) approach each other, the apparent frequency = \(f^{\prime}=\frac{v+v_0}{v-v_{\mathrm{s}}} f\), where the velocity of sound = v = 340 m s^-1.

Velocity of source (first car) = v_s = 22 m s^-1.

Velocity of observer (2nd car) = v_o = 16.5m s^-1.

True frequency = f = 400 Hz.

∴ \(f^{\prime}=\frac{340+16.5}{340-22} \times 400\)

= 448 Hz.

Question 18. The driver of a car travelling at 108 km h^-1 towards a hill sounds a horn of frequency 600 Hz. If the velocity of sound air is 330 m s^-1, the frequency of the reflected sound as heard by the driver is

1. 550 Hz
2. 555.5 Hz
3. 720 Hz
4. 500 Hz

Answer: 3. 720 Hz

Frequency of the sound reaching the hill is \(f^{\prime}=\frac{v}{v-v_{\mathrm{s}}} f\), where v_s = v_car This sound is reflected to the car (acts like an observer). The resultant frequency heard by the drivers

⇒ \(f^{\prime \prime}=\frac{\left(v+v_{\mathrm{car}}\right)}{v} f^{\prime}\)

= \(\frac{v+v_{\mathrm{car}}}{v-v_{\mathrm{car}}} f\)

⇒ \(\frac{330+30}{330-30} \times 600 \mathrm{~Hz}\)

= 720 Hz (∵ 108kmh^-1 = 30m s^-1).

Question 19. Two trains move towards each other at the same speed. The speed of sound is 340 m s^-1. If the height of the tone of the whistle of one of them heard the other changes by \(\frac{9}{8}\) times then the speed of each car should be

1. 20 ms^-1
2. 2 ms^-1
3. 200 ms^-1
4. 2000 ms^-1

Answer: 1. 20 ms^-1

The apparent frequency in the case of two trains approaching each other is

⇒ \(f^{\prime}=\frac{v+v_{\text {train }}}{v-v_{\text {train }}} f\)

⇒ \(\frac{f^{\prime}}{f}=\frac{v+v_{\text {train }}}{v-v_{\text {train }}}\)

Given, \(\frac{f^{\prime}}{f}=\frac{9}{8}\), velocity of sound = v = 340 m s^-1 and

velocity of train = v_train = ?

Substituting the values, \(\frac{9}{8}=\frac{340+v_{\text {train }}}{340-v_{\text {train }}}\)

v_train = 20 ms^-1.

Question 20. A star emitting radiation of wavelength 500 nm is approaching the earth with a velocity of 1.50 x 10⁶ m s^-1. The change in wavelength of the radiation as received on the earth is

1. 0.25 A
2. 2.5 A
3. 25 A
4. 250 A

Answer: 3. 25 A

According to the Doppler effect, there is an apparent increase in frequency and an apparent decrease in the wavelength of light radiated from the star approaching the Earth. The decrease in wavelength is given by

⇒ \(\Delta \lambda=\lambda\left(\frac{v}{c}\right)\)

= \((500 \mathrm{~nm})\left(\frac{1.5 \times 10^6 \mathrm{~ms}^{-1}}{3 \times 10^8 \mathrm{~ms}^{-1}}\right)\)

= 2.5nm

= 25 A.

Question 21. A whistle is made to revolve in a circle with angular velocity ω = 20 rad s^-1 using a string of length 50 cm. If the actual frequency of the sound of the whistle is 385 Hz then the minimum frequency heard by a listener far away from the centre is (given that velocity of sound in air = 340 m s^-1)

1. 385 Hz
2. 374 Hz
3. 399 Hz
4. 333 Hz

Answer: 2. 374 Hz

The tangential velocity of the whistle,

v_s = ωr = (20 rad s^-1)(50 x 10^-2m)

= 10m s^-1.

The apparent frequency heard by the listener will be minimum (at A) when the source is moving away and maximum when at B.

∴ \(f_{\min }=\frac{v}{v+v_s} f=\frac{340}{340+10} \times 385 \mathrm{~Hz}\)

= 374 Hz.

Question 22. A car is approaching a high hill. The driver of the car sounds a horn of frequency f. The reflected sound heard by the driver has a frequency of 2f. If the velocity of sound in air is v then the velocity of the car, in the same velocity units, will be

1. \(\frac{v}{\sqrt{2}}\)
2. \(\frac{v}{3}\)
3. \(\frac{v}{4}\)
4. \(\frac{v}{2}\)

Answer: 2. \(\frac{v}{3}\)

Apparent frequency reaching the hill, \(f^{\prime}=\frac{v}{v-v_{\text {car }}}\). The hill now acts as a sound source emitting sound waves of frequency f’.

Now, the apparent frequency of the car approaching the hill is

⇒ \(f^{\prime \prime}=\frac{v+v_{\mathrm{car}}}{v} f^{\prime}=\frac{v+v_{\mathrm{car}}}{v} \cdot \frac{v}{v-v_{\mathrm{car}}} f\)

Given that \(f^{\prime \prime}=2 f \Rightarrow \frac{v+v_{\mathrm{car}}}{v-v_{\mathrm{car}}}\)

= 2

∴ velocity of the car =v_car = \(\frac{v}{3}\)

Question 23. A listener moves towards a stationary source of sound with a speed \(\frac{1}{5}\) th of the speed of sound. The true values of the frequency and wavelength of the sound emitted are f and y respectively. The apparent frequency and wavelength recorded by the listener are respectively

1. f, 12λ
2. 0.8f, 0.8λ
3. 1.2f, 1.2λ
4. 1.2f, λ

Answer: 4. 1.2f, λ

When a listener moves towards a stationary source, the apparent frequency is \(f^{\prime}=\frac{v+v_e}{v} f\)

Given that \(v_0=\frac{v}{5} \Rightarrow f^{\prime}=\frac{v+\frac{v}{5}}{v} f=\frac{6}{5} f=1.2 f\)

= 1.2 f.

The motion of the listener does not affect the wavelength.

Hence, f’ = 1.2f, λ’ = λ

Question 24. A siren emitting a sound of frequency 800 Hz moves away from a listener towards a cliff at a speed of 15 m s^-1. Then, the frequency of sound that the listener hears in the echo reflected from the cliffs (given that velocity of sound in air = 330 m s^-1)

1. 800 Hz
2. 885 Hz
3. 838 Hz
4. 760 Hz

Answer: 3. 838 Hz

Frequency of sound waves reaching the cliffs

⇒ \(f^{\prime}=\frac{v}{v-v_s} f=\frac{330}{330-15} \times 800\)

= 838 Hz.

This is the apparent frequency of waves reflected from the cliff and heard by the standing listener.

Question 25. A source of sound S emitting waves of frequency 100 Hz and a listener O is located at some distance from each other. The source is moving at a speed of 19.4 m s^-1 at an angle of 60° with the source-listener tine as shown in the figure. The listener is at rest. The apparent frequency heard by the listener is (velocity of sound in air = 330 m s^-1)

1. 110 Hz
2. 103 Hz
3. 106 Hz
4. 97 Hz

Answer: 2. 103 Hz

The component of the velocity of the source towards the listener is

⇒ \(v_{\mathrm{s}}^{\prime}=v_5 \cos 60^{\circ}=\frac{v_{\mathrm{s}}}{2}\)

As the separation between the source and the listener decreases, there will be an apparent increase in frequency.

Hence,

⇒ \(f^{\prime}=\frac{v}{v-\frac{v_s}{2}} f\)

= \(\left(\frac{330}{330-\frac{19.4}{2}}\right) 100 \mathrm{~Hz}\)

= \(\frac{330 \times 100}{320.3} \mathrm{~Hz}\)

= 103 Hz.

Question 26. A speeding motorcyclist sees a traffic jam ahead of him. He slows down to 36 km h^-1. He finds that traffic has eased and a car moving ahead of him at 18 km h^-1 is honking at a frequency of 1392 Hz. If the speed of sound in air is 343 m s^-1, the frequency of the honk as heard by him will be

1. 1332Hz
2. 1372 Hz
3. 1412 Hz
4. 1454Hz

Answer: 3. 1412 Hz

Velocity of the motorcyclist= 36 x \(\frac{5}{18}\) m s^-1

= 10 m s^-1.

Velocity of the car moving away =18 x \(\frac{5}{18}\)m s^-1

= 5m s^-1.

Apparent frequency as heard by the motorcyclist,

⇒ \(f^{\prime}=\frac{v+v_{\text {bike }}}{v+v_{\text {car }}} f\)

= \(\frac{343+10}{343+5} \times 1392 \mathrm{~Hz}\)

= 1412 Hz

Question 27. Two sources of sound S₁ and S₂ are moving towards and away from a stationary listener with the same speed. If 3 beats per second are heard by the listener, find the speed of the sources. (Given that f₁ = f₂ = 500 Hz, speed of soundin air = 330 m s^-1.)

1. 2.5 ms^-1
2. 1 ms^-1
3. 3.2 ms^-1
4. 2 ms^-1

Answer: 2. 1 ms^-1

Apparent frequencies heard by the listener are

⇒ \(f_1=\frac{V}{V-v} f \text { and } f_2=\frac{V}{V+v} f \text {, }\)

where V= velocity ofsound= 330m s^-1,

v = speed of sources, and

f= true frequency = 500Hz.

Now, f₁-f₂ = 3S^-1

⇒ \(\frac{V}{V-v} f-\frac{V}{V+v} f=3 \mathrm{~s}^{-1}\)

⇒ \(\frac{V f}{V\left(1-\frac{v}{V}\right)}-\frac{V f}{V\left(1+\frac{v}{V}\right)}=3 \mathrm{~s}^{-1}\)

⇒ \(\left(1+\frac{v}{V}\right) f-\left(1-\frac{v}{V}\right) f=3 \mathrm{~s}^{-1}\)

⇒ \(v=\frac{3 \mathrm{~s}^{-1} V}{2 f}\)

= \(\frac{3 \mathrm{~s}^{-1}\left(330 \mathrm{~ms}^{-1}\right)}{2\left(500 \mathrm{~s}^{-1}\right)}\)

= 0.99ms^-1

= 1 ms^-1.

Question 28. A submarine travelling at 18 km h^-1 is being chased by another submarine travelling at 27 km h^-1 along the line of its velocity. B sends a sonar of 500Hz to detect A and receives a reflected sound of frequency f. The value of f is close to (speed of sound in water = 1500m s^-1)

1. 504Hz
2. 499 Hz
3. 507 Hz
4. 502 Hz

Answer: 4. 502 Hz

The velocity of A is v_A = 5 m s^-1 and that of B is v_B = \(\frac{15}{2}\) m s^-1. The apparent frequency detected by A is \(f^{\prime}=\frac{v-v_{\mathrm{A}}}{v-v_{\mathrm{B}}} f\) when A is the detector and B is the source.

The apparent frequency detected by B for the wave which gets reflected from A is

⇒ \(f^{\prime \prime}=\frac{v+v_{\mathrm{B}}}{v+v_{\mathrm{A}}} f^{\prime}\) (when B is the detector and A is the source)

⇒ \(\left(\frac{v+v_{\mathrm{B}}}{v+v_{\mathrm{A}}}\right)\left(\frac{v-v_{\mathrm{A}}}{v-v_{\mathrm{B}}}\right) f\)

= \(\left(\frac{1500+\frac{15}{2}}{1500+5}\right) \times\left(\frac{1500-5}{1500-\frac{15}{2}}\right) 500 \mathrm{~Hz}\)

= 502 Hz.

Question 29. A source of sound S is moving with a velocity of 50m s^-1 towards a stationary listener. The listener detects the frequency of the source as 1000Hz. What will be the source’s apparent frequency when it moves away from the listener after crossing him? (Velocity of sound in air = 350 m s^-1.)

1. 1140 Hz
2. 750 Hz
3. 850 Hz
4. 805 Hz

Answer: 2. 750 Hz

The apparent frequency detected by the listener is

⇒ \(f^{\prime}=\frac{v}{v-v_{\mathrm{s}}} f\)

⇒ \(1000 \mathrm{~Hz}=\frac{350 \mathrm{~m} \mathrm{~s}^{-1}}{350 \mathrm{~m} \mathrm{~s}^{-1}-50 \mathrm{~m} \mathrm{~s}^{-1}} \times f=\frac{350}{300} f\)

When the moving source has crossed the listener and is moving away,

⇒ \(f^{\prime \prime}=\frac{350}{350+50} f=\frac{350}{400} f\)

∴ \(\frac{f^{\prime \prime}}{1000}=\frac{350}{400} \times \frac{300}{350}=\frac{3}{4}\)

Hence f” = 750Hz.

Question 30. Two sources of sound S₁ and S₂ produce sound waves of the same frequency of 600 Hz. A listener is moving from source S₁ towards source S₂ with a constant speed u m s^-1 and hears 10 beats per second. The velocity of sound in air is 330m s^-1. The value of u is

1. 10.30 ms^-1
2. 5.56 ms^-1
3. 2.75 ms^-1
4. 15.35 ms^-1

Answer: 3. 2.75 ms^-1

Apparent frequencies detected by the listener are

⇒ \(f_1=\frac{v}{v-u} f=\left(1-\frac{u}{v}\right)^{-1} f \sim\left(1+\frac{u}{v}\right) f\)

and \(f_2=\frac{v}{v+u} f\)

= \(\left(1+\frac{u}{v}\right)^{-1} f \sim\left(1-\frac{u}{v}\right) f\)

Sincebeat frequency = difference in frequency,

⇒ \(10 \mathrm{~s}^{-1}=f_1-f_2\)

= \(2 \frac{u}{v} f\)

= \(\frac{(2 u)\left(600 \mathrm{~s}^{-1}\right)}{330 \mathrm{~m} \mathrm{~s}^{-1}}\)

Hence, \(\frac{330}{120}\) ms^-1

= 2.75 m s^-1.

Question 31. A stationary source emits sound waves of frequency 500 Hz. Two listeners moving along a line passing through the source detect the sound to be of frequency 480 Hz and 530 Hz. Their respective speeds (in m s^-1) are (given that speed of sound = 300 ms^-1)

1. 12, 18
2. 8, 18
3. 16, 14
4. 12, 16

Answer: 1. 12, 18

For the listener moving away from the source, the apparent frequency decreases and for the listener moving towards the sources the apparent frequency increases.

Hence,

⇒ \(480 \mathrm{~Hz}=\left(\frac{v-u_1}{v}\right) f \text { and } 530 \mathrm{~Hz}=\left(\frac{v+u_2}{v}\right) f\)

where u₁ and u₂ are the velocities of the two listeners. Substituting the
values,

⇒ \(480=\left(1-\frac{u_1}{300}\right) 500 \Rightarrow u_1=12 \mathrm{~m} \mathrm{~s}^{-1}\)

and \(530=\left(1+\frac{u_2}{300}\right) 500 \Rightarrow u_2=18 \mathrm{~m} \mathrm{~s}^{-1}\)

Question 32. A train moves towards a stationary listener with a uniform speed of 34 m s^-1. The train sounds a whistle and its frequency detected by the listener is f₁ If the speed of the train is reduced to 17 m s^-1, the frequency detected is f₂. If the speed of sound is 340 m s^-1, the ratio is

1. \(\frac{19}{18}\)
2. \(\frac{18}{17}\)
3. \(\frac{20}{19}\)
4. \(\frac{21}{20}\)

Answer: 1. \(\frac{19}{18}\)

The apparent frequency detected by the approaching train is \(f^{\prime}=\frac{v}{v-v_{\mathrm{T}}} f\)

When \(v_{\mathrm{T}}=34 \mathrm{~m} \mathrm{~s}^{-1}, f_1=\frac{340}{340-34} f\)

When \(v_{\mathrm{T}}=17 \mathrm{~m} \mathrm{~s}^{-1}, f_2=\frac{340}{340-17}\)

∴ \(\frac{f_1}{f_2}=\frac{323}{306}\)

= \(\frac{17 \times 19}{17 \times 18}\)

= \(\frac{19}{18}\)

Question 33. A person standing on open ground hears the sound of a jet aeroplane coming from the north at an angle of 60° with the ground. But he finds the aeroplane right vertically above his position. If v is the speed of sound then the speed of the plane is

1. \(\frac{\sqrt{3}}{2} v\)
2. v
3. \(\frac{v}{2}\)
4. \(\frac{2}{\sqrt{3}} v\)

Answer: 3. \(\frac{v}{2}\)

At time t = 0, the sound from the jet plane propagates along the line P₀O towards the person at O. During the time interval t, the jet plane has moved through P₀P and is directly overhead.

Thus,

P₀P = (velocity of jetplane, v_p)(time t) and

P₀O = (velocity of sound in air, v_a)(time t).

⇒ \(\frac{P_0 P}{P_0 O}=\frac{v_{\mathrm{p}}}{v_{\mathrm{a}}}=\cos 60^{\circ}\)

Hence, v_p = \(\frac{v}{2}\)

Question 34. Two cars A and B are moving away from each other in opposite directions. Both the cars are moving at a speed of 20 m s^-1 relative to the ground. If a listener in car A detects a frequency of 2 kHz of the sound coming from car B, what is the true frequency of the sound source in car B? (Given that speed of sound in air = 340 m s^-1.)

1. 2250 Hz
2. 250 Hz
3. 300 Hz
4. 2060 Hz

Answer: 1. 2250 Hz

The apparent frequency detected by a listener in car A will be

⇒ \(f^{\prime}=\frac{v-v_{\mathrm{A}}}{v+v_{\mathrm{B}}} f\)

=> 2kHz = \(\frac{340-20}{340+20} f=\frac{320}{360}\)

true frequency = f = (2000Hz) \(\frac{9}{8}\) = 2250Hz

Question 35. The driver of a car moving with velocity v towards a hill blows a horn which emits a sound of frequency 420 Hz. The frequency of the reflected sound detected by the driver was found to be 490 Hz. The velocity of the car is (given that velocity of sound in air = 330 ms^-1)

1. 71 km h^-1
2. 61 km h^-1
3. 91 km h^-1
4. 81 km h^-1

Answer: 3. 91 km h^-1

The apparent frequency of a sound wave reaching the hill (as receiver),

⇒ \(f^{\prime}=\frac{v}{v-v_{\mathrm{car}}} f\)

The apparent frequency detected by the driver (observer) moving towards the hill (source) is

⇒ \(f^{\prime \prime}=\left(\frac{v+v_{\mathrm{car}}}{v}\right) f^{\prime}=\left(\frac{v+v_{\mathrm{car}}}{v-v_{\mathrm{car}}}\right) f\)

⇒ \(490=\frac{330+v_{\mathrm{car}}}{330-v_{\mathrm{car}}} \times 420\)

⇒ \(v_{\mathrm{car}}=\frac{330}{13} \mathrm{~m} \mathrm{~s}^{-1}\)

= 91 km h^-1

Vibration of Air Column: Organ Pipe

Question 36. The two nearest harmonics of a tube dosed at one end and open at the other end are 220 Hz and 260 Hz. What is the fundamental frequency of the system?

1. 20Hz
2. 30Hz
3. 40Hz
4. 10Hz

Answer: 1. 20Hz

In a closed organ pipe (open at one end only), only odd harmonics are present with fundamental (or first harmonics) = f₁ = \(\frac{v}{4l}\). Two consecutive harmonics are (2n-1)f₁ and (2n +1)f₁.

Thus, their difference is

(2n + 1)f₁– (2n-1)f₁

= 260Hz – 220Hz

= 40Hz

or 2f₁ = 40Hz. Hence the fundamental frequency is f₁ = 20Hz.

Question 37. An air column, closed at one end and open at the other, resonates with a tuning for k when the smallest length of the column is 50 cm. The next larger length of the column resonating with the same tuning for k is

1. 150 cm
2. 100 cm
3. 200 cm
4. 66.7 cm

Answer: 1. 150 cm

In a closed organ pipe, let be the length of the air column for the 1st resonance; then \(l_1=\frac{\lambda}{4}\)

For the 2nd resonance, \(l_2=\frac{3 \lambda}{4}\)

⇒ \(\frac{l_2}{l_1}=\frac{\frac{3 \lambda}{4}}{\frac{\lambda}{4}}=3, \text { thus } l_2=3 l_1\)

= 3(50 cm)

= 150 cm,

Question 38. The second overtone of an open organ pipe has the same frequency as the first overtone of a closed pipe metres long. The length of the open pipe will be

1. 2L
2. L
3. \(\frac{L}{2}\)
4. 4L

Answer: 1. 2L

In an open organ pipe, the second overtone is the third harmonic for which \(f_3=3 f_1=3\left(\frac{v}{2 L_0}\right)\),

where P = speed of sound, and L₀ = length of the open pipe.

The first overtone of the closed pipe is the third harmonic

⇒ \(f_3^{\prime}=3\left(\frac{v}{4 L_c}\right)=3 \frac{v}{4 L}\) (∵ L_c = L).

Equating f₃ and f’₃,

⇒ \(\frac{3 v}{2 L_0}=\frac{3 v}{4 L}\)

=> L₀ = 2L

Question 39. The fundamental frequency of a closed organ pipe of length 20 cm is equal to the second overtone of an organ pipe open at both ends. The length of the organ pipe open at both ends is

1. 80 cm
2. 140 cm
3. 100 cm
4. 120 cm

Answer: 4. 120 cm

Given: length of the closed pipe = 20 cm.

Its fundamental frequency is \(f_{\mathrm{c}}=\frac{v}{4 l}=\frac{v}{80 \mathrm{~cm}}\)

The second overtone of the open pipe is the third harmonic for which

⇒ \(f_3=3 f_1=3\left(\frac{v}{2 l_0}\right)\)

Since \(f_{\mathrm{c}}=f_3, \text { so } \frac{3 v}{2 l_0}=\frac{v}{80 \mathrm{~cm}}\)

∴ length of the pipe is l₀ = 120 cm.

Question 40. A closed organ pipe (closed at one end) is excited to support the third overtone. It is found that the air column in the pipe has

1. Three nodes and three antinodes
2. Three nodes and four antinodes
3. Four nodes and three antinodes
4. Four nodes and four antinodes

Answer: 4. Four nodes and four antinodes

In a closed organ pipe, 1st, 3rd, 5th, 7th, … harmonics are present.

So, the 3rd overtone is the 7th harmonic, which will consist of four nodes and four antinodes as shown in the adjoining figure.

Question 41. The number of possible natural oscillations of an air column in a pipe closed at one end of length 85 cm whose frequencies lie below 1250Hz is (velocity of sound = 340 m s^-1)

1. 4
2. 5
3. 7
4. 6

Answer: 4. 6

The fundamental frequency in the closed pipe is

⇒ \(f_1=\frac{v}{4 l}=\frac{340 \mathrm{~m} \mathrm{~s}^{-1}}{4\left(85 \times 10^{-2} \mathrm{~m}\right)}\) = 100Hz.

Since only odd harmonics are present, the harmonics below 1250 Hz are(in Hz) 100, 300, 500, 700, 900 and 1100.

Hence, there are 6 possible harmonics

Question 42. If we study the vibration of a pipe open at both ends, which of the following statements is not true?

1. The open ends will be antinodes.
2. Odd harmonics of the fundamental frequency will be present.
3. All harmonics of the fundamental frequency will be generated.
4. Pressure change will be maximum at both ends.

Answer: 4. Pressure change will be maximum at both ends.

During the longitudinal vibration of an air column, the displacements of antinodes and pressure nodes are coincident. At open ends in the pipe, there exist displacement antinodes. Hence, pressure nodes exist where a change of pressure is not maximum.

Question 43. A cylindrical resonance tube open at both ends has a fundamental frequency f in air. If half of the length is dipped vertically in water, the fundamental frequency of the air column will be

1. 2f
2. \(\frac{3}{2}\)
3. f
4. \(\frac{f}{2}\)

Answer: 4. \(\frac{f}{2}\)

Let L be the length of the open organ pipe, for which the fundamental frequency is f = \(\frac{v}{2L}\) When half the length of the pipe is dipped

vertically in water, it becomes a closed organ pipe with length = \(\frac{L}{2}\)

The fundamental frequency of this dosed pipe is

⇒ \(f^{\prime}=\frac{v}{4\left(\frac{L}{2}\right)}\)

= \(\frac{v}{2 L}\)

= f.

Question 44. An organ pipe closed at one end has a fundamental frequency of 1500 Hz. The maximum number of overtones generated by this pipe which a normal person can hear is

1. 14
2. 13
3. 6
4. 9

Answer: 4. 9

The audible range is 20 Hz to 20 kHz.In a dosed organ pipe, only odd harmonics are produced. Since the fundamental frequency is f₁ = 1500 Hz, the overtones are 3f₁, 5f₁…

∴ 20,000 = n x 1500

n ≈ 13.

Hence, the maximum number of possible harmonics is seven, the harmonics being the 1st, 3rd, 5th, 7th, 9th, 11th and 13th.

The number of overtones = 7-1

= 6.

Question 45. In a pipe, the die fundamental frequency is 50 Hz and the next successive frequencies are 150 Hz and 250 Hz. Then, the pipe is

1. Closed at both ends
2. Closed at one end
3. An open pipe
4. A stretched pipe

Answer: 2. Closed at one end

Since 50 Hz is the fundamental (1st harmonic), the higher harmonics are 150 Hz = 3 x 50 Hz = 3f₁, and 250 Hz = 5 x 50 Hz = 5f₁.

The presence of only odd harmonics indicates that the pipe is dosed at one end.

Question 46. A tube closed at one end produces the fundamental note of frequency 512 Hz. If it is open at both ends, the fundamental frequency will be

1. 1024 Hz
2. 256 Hz
3. 1250 Hz
4. 768 Hz

Answer: 1. 1024 Hz

The frequency of the fundamental note in a closed pipe is f₁ = 512 Hz

= \(\frac{2}{4l}\) . When the ends are open, the fundamental frequency is

⇒ \(\frac{v}{2 l}=2\left(\frac{v}{4 l}\right)\)

= 2(512 Hz)

= 1024 Hz

Question 47. A pipe open at both ends suddenly dosed at one end, as a result of which the frequency of the third harmonic of the dosed pipe is found to be higher by 100 Hz than the fundamental frequency of the open pipe. The fundamental frequency of the open pipe is

1. 200 Hz
2. 240 Hz
3. 450 Hz
4. 300 Hz

Answer: 1. 200 Hz

The fundamental frequency of the open pipe is fo = \(\frac{v}{2l}\) and the 3rd harmonic of the dosed pipe is \(f_{\mathrm{c}}=\frac{3 v}{4 l}=\frac{3}{2}\left(\frac{v}{2 l}\right)=\frac{3}{2} f_{\mathrm{o}}\)

Given that f_c = f_o + 100

⇒ \(\left(\frac{3}{2}-1\right) f_0=100 \mathrm{~Hz}\)

=> f_o = 200Hz.

Question 48. The third overtone of an open organ pipe of length has the same frequency as the third overtone of a dosed pipe of length The ratio \(\frac{L_0}{L_c}\) is

1. 2:1
2. 3:2
3. 5:3
4. 8:7

Answer: 4. 8:7

The third overtone of an open pipe is the fourth harmonic, which is

⇒ \(f_0=4\left(\frac{v}{2 L_0}\right)\)

The third overtone of the dosed pipe is the seventh harmonic, which is

⇒ \(f_{\mathrm{c}}=7\left(\frac{v}{4 L_{\mathrm{c}}}\right)\)

Since \(f_{\mathrm{o}}=f_{\mathrm{c}^{\prime}} \frac{2 v}{L_{\mathrm{o}}}\)

= \(\frac{7 v}{4 L_{\mathrm{c}}}\)

⇒ \(\frac{L_{\mathrm{o}}}{L_{\mathrm{c}}}=\frac{8}{7}\)

Question 49. A pipe of length 1 m is dosed at one end. The velocity of sound in air is 300 m s^-1. The air column in the pipe will not resonate for sound of frequency

1. 75 Hz
2. 225 Hz
3. 300 Hz
4. 378 Hz

Answer: 3. 300 Hz

In a dosed organ pipe only odd harmonics are produced. So the tube will resonate with lengths

⇒ \(l=\frac{\lambda}{4}, 3 \frac{\lambda}{4}, 5 \frac{\lambda}{4}, \ldots,(2 N+1) \frac{\lambda}{4}\)

⇒ \(\lambda=\frac{4 l}{2 N+1}\)

∴ the allowed frequencies are

⇒ \(f=\frac{v}{\lambda}\)

= \((2 N+1) \frac{v}{4 l}\)

= \((2 N+1) \frac{300 \mathrm{~m} \mathrm{~s}^{-1}}{4 \mathrm{~m}}\)

= 75(2N+ 1) Hz.

With N= 0,1, 2, …, f=? 75Hz, 225Hz, 375Hz, ….

So, 300 Hz will not resonate

Question 50. Find the number of tones present in an open organ pipe of length 1 m whose frequencies lie within 1 kHz. (Given that the speed of sound in air = 330m s^-1.)

1. 6
2. 4
3. 3
4. 5

Answer: 1. 6

Fundamental frequency is \(f_1=\frac{v}{2 l}=\frac{330 \mathrm{~m} \mathrm{~s}^{-1}}{2(1 \mathrm{~m})}=165 \mathrm{~s}^{-1}\)

The notes produced have frequencies f₁, 2f₁, …, so the number of tones

lying within 1 kHz will be n = \(\frac{1000 \mathrm{~Hz}}{165 \mathrm{~Hz}} \approx 6\)

Question 51. Second harmonics are produced by an open organ pipe of length 50 cm. A person moves towards the organ pipe at a speed of 10 km h^-1. If the speed of sound is 330m s^-1, the frequency heard by the person will be

1. 666 Hz
2. 500 Hz
3. 753 Hz
4. 333 Hz

Answer: 1. 666 Hz

In an open organ pipe, the frequency of the fundamental is f₁ = \(\frac{v}{2l}\)

For the 2nd harmonic, \(f_2=2 f_1=2\left(\frac{v}{2 l}\right)=\frac{330 \mathrm{~m} \mathrm{~s}^{-1}}{50 \mathrm{~cm}}\)

= 660 Hz.

Speed ofobserveris v₀ = 10km h^-1 = \(\frac{50}{18}\)m s^-1.

Because of the Doppler effect, the apparent frequency is

⇒ \(f^{\prime}=\left(\frac{v+v_{\Omega}}{v}\right) f=\left(1+\frac{v_{\Omega}}{v}\right) f_2\)

Substituting the values:

⇒ \(f^{\prime}=\left(1+\frac{50}{18 \times 330}\right) 660 \mathrm{~Hz}\)

= 660Hz + \(\frac{50}{9}\)Hz == 666 Hz

Question 52. A dosed organ pipe has a fundamental frequency of 1.5 kHz. The number of overtones that can be distinctly heard by a person from this organ pipe will be (given that audible range = 20Hz-20 kHz)

1. 8
2. 6
3. 4
4. 5

Answer: 2. 6

If the die-closed pipe has n overtones, the maximum frequency (2n + 1)f corresponds to the maximum frequency of the audible range (= 20 kHz).

Thus, (2n +1)(1.5 kHz) = 20kHz

or 2n = 12

⇒ number of overtones, n = 6.

Question 53. A tuning fork of frequency 480 Hz is used in an experiment for measuring the speed of sound (v) in air by the resonance tube method. Resonance is observed to occur at two successive lengths of the air column, l₁ = 30 cm and l₂ = 70 cm. Then v is equal to

1. 332 ms^-1
2. 371 ms^-1
3. 338 ms^-1
4. 384 m s^-1

Answer: 4. 384 m s^-1

For 1st resonance, \(\frac{\lambda}{4}=h_1+\epsilon\), and

for 2nd resonance, \(\frac{3 \lambda}{4}=l_2+\epsilon\)

⇒ \(\lambda=2\left(l_2-l_1\right)\)

∴ The velocity of sound is

v = fλ =(480s^-1) x 2 x (70cm – 30cm)

= (960 s^-1)(40 X 10^-2 m)

= 384 m s^-1.

Question 54. In a resonance tube experiment, when the tube is filled with water up to a height of 17.0 and from the bottom, it resonates with a given tuning fork. When the water level is raised, the next resonance with die same tuning fork occurs at a height of 24.5 cm. The frequency of the tuning fork is (given that the velocity of sound in air = 330m s^-1)

1. 2200 Hz
2. 3300 Hz
3. 1100 Hz
4. 550 Hz

Answer: 1. 2200 Hz

The difference between the lengths of the air columns at two consecutive resonances is \(\frac{λ}{2}\)

Hence, \(\frac{λ}{2}\) = (24.5 cm-17.0 cm)

= 7.5 cm.

∴ wavelength is λ = 15 cm.

Hence, frequency = \(f=\frac{v}{\lambda}=\frac{330 \mathrm{~m} \mathrm{~s}^{-1}}{15 \times 10^{-2} \mathrm{~m}}\)

= 2200 Hz.

Vibration of a String: Sonometer

Question 55. A stretched string resonates with a tuning fork of frequency 512Hz when the length of the string is 0.5m. The length of the string required for it to vibrate resonantly with a tuning fork of frequency 256Hz would be

1. 0.25 m
2. 0.5 m
3. 1 m
4. 2 m

Answer: 3. 1 m

In the transverse vibration of a stretched string, frequency \(f \propto \frac{1}{\text { length }(l)}\)

Thus, f₁l₁ = f₂l₂

=> (512Hz)(0.5 m)

= (256Hz)l₂

⇒  l₂ = 1.0 m.

Question 56. A uniform string of length 5.5m has a mass of 35 g. If the tension in the string is 77N, the speed of the wave on the string is

1. 110 ms^-1
2. 165 ms^-1
3. 77 ms^-1
4. 102 ms^-1

Answer: 1. 110 ms^-1

Given that mass m = 35 x 10^-3 kg, length l = 5.5 m, tension F = 77N.

Then, massperunitlengthis

⇒ \(\mu=\frac{m}{l}\)

= \(\frac{35 \times 10^{-3}}{5.5}\)

= \(\frac{70}{11} \times 10^{-3} \mathrm{~kg} \mathrm{~m}^{-1}\)

The speed of the wave is

⇒ \(v=\sqrt{\frac{F}{\mu}}\)

= \(\sqrt{\frac{77 \mathrm{~N}}{\frac{70}{11} \times 10^{-3} \mathrm{~kg} \mathrm{~m}^{-1}}}\)

= 110 ms^-1.

Question 57. A wave of frequency 100 Hz is sent along a string towards its fixed end. When these wave gels are reflected, a node is formed at a distance of 10 cm from the fixed end of the string. The speed of the incident and the reflected wave is

1. 5ms^-1
2. 10 m s^-1
3. 20 ms^-1
4. 40m s^-1

Answer: 3. 20 ms^-1

At the fixed end of the string, an ode is produced, so the distance between two consecutive nodes is \(\frac{\lambda}{2}\) = 10 cm

or λ = 20 cm

= 20 x 10^-2 m.

The speed of the incident and the reflected wave is

v = fλ = (100 s^-1)(20 x 10^-2m)

= 20m s^-1.

Question 58. A sonometer wire when vibrated over its entire length has frequency n. Now it is divided by bridges into a number of segments of lengths l₁, l₂, l₃,… When vibrated, these segments have frequencies n₁, n₂, n₃ …. Then the correct relation is

1. n = n₁ + n₂ + n₃ + ……..
2. \(n^2=n_1^2+n_2^2+n_3^2+\ldots\)
3. \(\frac{1}{n}=\frac{1}{n_1}+\frac{1}{n_2}+\frac{1}{n_3}+\ldots\)
4. \(\frac{1}{\sqrt{n}}=\frac{1}{\sqrt{n_1}}+\frac{1}{\sqrt{n_2}}+\frac{1}{\sqrt{n_3}}+\ldots\)

Answer: 3. \(\frac{1}{n}=\frac{1}{n_1}+\frac{1}{n_2}+\frac{1}{n_3}+\ldots\)

According to the law of length, frequency

⇒ \(n \propto \frac{1}{l}\)

⇒ \(n_1 l_1=n_2 l_2=\ldots=k\)

∵ total length l = l₁ + l₂+ l₃+ …

∴ \(\frac{k}{n}=\frac{k}{n_1}+\frac{k}{n_2}+\frac{k}{n_3}+\ldots\)

⇒ \(\frac{1}{n}=\frac{1}{n_1}+\frac{1}{n_2}+\frac{1}{n_3} \ldots\)

Question 59. Two strings A and B of lengths l_A and l_B have their upper ends fixed to rigid supports, They have masses M_A and M_B. If n_A and n_B are the frequencies of their vibrations and n_A = 2n_B then

1. l_A– 4l_B regardless of their masses
2. l_B = 4l_A, regardless of their masses
3. M_A = 2M_B, l_A = 2l_B
4. M_B = 2M_A, l_B– 2l_A

Answer: 2. l_B = 4l_A, regardless of their masses

Frequency of vibration in a suspended string,

⇒ \(n=\frac{1}{2 l} \sqrt{\frac{m g}{\mu}}=\frac{1}{2 l} \sqrt{\frac{m g}{\frac{m}{l}}}=\frac{1}{2 l} \sqrt{l g}=\frac{1}{2} \sqrt{\frac{l g}{l^2}}=\frac{1}{2} \sqrt{\frac{g}{l}}\)

Given that \(n_{\mathrm{A}}=2 n_{\mathrm{B}}\)

⇒ \(\frac{1}{2} \sqrt{\frac{g}{l_{\mathrm{A}}}}=2 \cdot \frac{1}{2} \sqrt{\frac{g}{l_{\mathrm{B}}}}\)

Hence, l_B = 4l_A which will not depend on the masses M_A and M_B.

Question 60. When a string is divided into three segments of lengths l₁, l₂ and l₃, the fundamental frequencies of these segments are f₁, f₂ and f₃ respectively. The original fundamental frequency f of the string is

1. \(\sqrt{f}=\sqrt{f_1}+\sqrt{f_2}+\sqrt{f_3}\)
2. f = f₁ + f₂ + f₃
3. \(\frac{1}{\sqrt{f}}=\frac{1}{\sqrt{f_1}}+\frac{1}{\sqrt{f_2}}+\frac{1}{\sqrt{f_3}}\)
4. \(\frac{1}{f}=\frac{1}{f_1}+\frac{1}{f_2}+\frac{1}{f_3}\)

Answer: 4. \(\frac{1}{f}=\frac{1}{f_1}+\frac{1}{f_2}+\frac{1}{f_3}\)

For the transverse vibration of a stretched string, frequency \(f=\frac{1}{2 l} \sqrt{\frac{F}{\mu}}\)

When F and p are constants,

fl=k

⇒ \(f l=f_1 l_1=f_2 l_2=f_3 l_3=k\)

⇒ \(l=\frac{k}{f}, l_1=\frac{k}{f_1}, l_2=\frac{k}{f_2}, l_3=\frac{k}{f_3}\)

∵ \(l=l_1+l_2+l_{3 f}\)

∴ \(\frac{1}{f}=\frac{1}{f_1}+\frac{1}{f_2}+\frac{1}{f_3}\)

Question 61. A uniform rope of length L and mass M hangs vertically from a rigid support. A block of mass m is attached to the free end of the rope. A transverse pulse of wavelength λ₁ is produced at the lower end of the rope. The wavelength of the pulse when it reaches the top of the rope is λ₂. The ratio \(\frac{\lambda_2}{\lambda_1}\) is

1. \(\sqrt{\frac{M+m}{m}}\)
2. \(\sqrt{\frac{m}{M}}\)
3. \(\sqrt{\frac{M+m}{M}}\)
4. \(\sqrt{\frac{M}{m}}\)

Answer: 1. \(\sqrt{\frac{M+m}{m}}\)

Since the rope has mass M, its tension will be different at different points. The tension at the free end will be mg (due to the mass m of the block) and that at the upper fixed end is (M + m)g. The frequency of the wave pulse will be the same everywhere on the rope as it depends only on the frequency of the source. The mass per unit length is also the same throughout.

⇒ \(\lambda=\frac{v}{f}=\frac{1}{f} \sqrt{\frac{F}{\mu}}\)

⇒ \(\lambda \propto \sqrt{F}\)

⇒ \(\frac{\lambda_2}{\lambda_1}=\sqrt{\frac{M+m}{m}}\)

Question 62. The tension in a piano Wire is 10 N. What should be the tensionin the wire to produce a note double the frequency?

1. 40 N
2. 5 N
3. 80 N
4. 20 N

Answer: 1. 40 N

Since \(f \propto \sqrt{F}\)

⇒ \(\frac{f_2}{f_1}=\sqrt{\frac{F_2}{F_1}}\)

⇒ \(\frac{2 f_1}{f_1}=\sqrt{\frac{F_2}{10 \mathrm{~N}}}\)

F₂ = 40 N

Question 63. Standing waves are produced in a 10-m-long stretched string. If the string vibrates in 5 segments and the wave velocity is 20m s^-1 its frequency is

1. 5 Hz
2. 4 Hz
3. 2 Hz
4. 10 Hz

Answer: 1. 5 Hz

Length of string = L = 10 m.

Length of each segment = \(\frac{\lambda}{2}\)

So, 5.\(\frac{\lambda}{2}\) = 10m

or, λ = 4m.

Frequesncy = \(f=\frac{v}{\lambda}=\frac{20 \mathrm{~m} \mathrm{~s}^{-1}}{4 \mathrm{~m}}\) = 5Hz.

Question 64. A transverse wave passes through a string with equation y = 10 sin π(0.02x – 2.0t), where x is in metres and t is in seconds. The maximum particle velocity in the wave motion is

1. 63
2. 78
3. 100
4. 121

Answer: 1. 63

The wave equation is y = 10π(0.02x- 2.0t).

∵ particle velocity= \(\frac{\partial y}{\partial t}=-20 \pi \cos \pi(0.02 t-2 t)\)

The maximum value of particle velocity is

⇒ \(\left(\frac{\partial y}{\partial t}\right)_{\max }=20 \pi \text { units }\)

= 63 units

Question 65. A string is stretched between fixed points separated by 75 cm. It is observed to have resonant frequencies of 420Hz and 315Hz and other resonant frequencies between these two. The lowest resonant frequency for this string is

1. 10.5 Hz
2. 105 Hz
3. 155 Hz
4. 205 Hz

Answer: 2. 105 Hz

The given resonant frequencies are

Hence, 315Hz = frequency of the 3rd harmonic and 420 is that of the 4th harmonic.

∴ the lowest (or 1st harmonic) frequency is

⇒ \(f_1=\frac{315}{3} \mathrm{~Hz}=\frac{420}{4} \mathrm{~Hz}\)

= 105Hz.

Question 66. The tension in a string is increased by 44%. If the frequency of vibration is to remain unchanged, its length must be increased by

1. 44%
2. V44%
3. 22%
4. 20%

Answer: 4. 20%

Frequency = \(f=\frac{1}{2 l} \sqrt{\frac{F}{\mu}}=\frac{1}{2(l+\Delta l)} \sqrt{\frac{F+\frac{44}{100} F}{\mu}}\)

⇒ \(\frac{l+\Delta l}{l}=\frac{12}{10}\)

⇒ \(1+\frac{\Delta l}{l}=1+\frac{2}{10}\)

∴ % increase in length \(\frac{\Delta l}{l} \times 100 \%=\frac{2}{10} \times 100 \%\)

= 20%.

Question 67. The equation of a wave travelling along a stretched string is given by y = (0.002 m)sin(300t- 15x). The mass density is μ = 0.1 kg m^-1. The tension in the strings

1. 30 N
2. 40 N
3. 10 N
4. 45 N

Answer: 2. 40 N

From the given wave equation, the velocity of the wave is

⇒ \(v=\frac{\omega}{k}=\frac{300 \mathrm{~s}^{-1}}{15 \mathrm{~m}^{-1}}=20 \mathrm{~m} \mathrm{~s}^{-1}\)

But \(v=\sqrt{\frac{F}{\mu}}\) hence the tension is F = μv² = (0.1 kg m^-1)(20m s^-1)²

= 40N.

Question 68. A 2.0-m-long string fixed at its ends is driven by a 240-Hz vibrator. The string vibrates in its third harmonic mode. The speed of the wave and its fundamental frequency are

1. 320 ms^-1, 80 Hz
2. 180 m s^-1 80 Hz
3. 320 ms^-1, 120 Hz
4. 180 ms^-1, 120 Hz

Answer: 1. 320 ms^-1, 80 Hz

Given that l = 2.0 m and the frequency of the 3rd harmonic is

f₃ = 3f₁ = 240 Hz.

∴ the frequencyofthe fundamental mode is = \(\frac{240}{3}\)Hz = 80 Hz.

For the 3rd harmonic, 3 \(\frac{λ}{2}\) = 2.0m

λ = \(\frac{4}{3}\) m.

Speed of the wave = v = fλ = (240 s^-1) (\(\frac{4}{3}\)m)

= 320 ms^-1.

Question 69. A string is damped at both ends and is vibrating in its 4th harmonic. The equation of the stationary wave is y = 0.3 sin(0.157x)cos(2007πt). The length of the string is (all quantities are in SI units)

1. 40 m
2. 25 m
3. 80 m
4. 60 m

Answer: 3. 80 m

The equation of a standing wave is given by y = 2Asin(kx)cos(ωt).

Comparing with the given equation,

y= 0.3sin(0.157x)cos(2007πt),

k = \(\frac{2 \pi}{\lambda}\)

= 0.157 m^-1 and

ω = 2πf = 200n s^-1.

wavelength λ = \(\frac{2 \pi}{0.157}\) m

= 40m.

Since the string vibrates in its 4thharmonic, the length of the string will be,

⇒ \(L=4\left(\frac{\lambda}{2}\right)=2 \lambda\)

= 80m.

Question 70. A string of length 1 m and mass 5g is fixed at both ends. The tension in the string is 8.0 N. The string is set into transverse vibration, using an external vibrator of frequency 100 Hz. The separation between the successive nodes on the string is due to

1. 16.6 cm
2. 33.3 cm
3. 20.0 cm
4. 10.0 cm

Answer: 3. 20.0 cm

Given that for a vibrating string, the linear mass density is μ = 5 x 10^-3 kg m^-1, the length of the string is l = 1 m, the tension in the string is F = 8 N and the frequency is f = 100 s. If the string vibrates in its pth harmonic, we have

⇒ \(f=\frac{p}{2 l} \sqrt{\frac{F}{\mu}}\)

⇒ \(p=(2 f l) \sqrt{\frac{\mu}{F}}\)

= \(2\left(100 \mathrm{~s}^{-1}\right)(1 \mathrm{~m}) \sqrt{\frac{5 \times 10^{-3} \mathrm{~kg}}{8 \mathrm{~N}}}\)

Hence, the string vibrates in 5 loops.

∴ \(5\left(\frac{\lambda}{2}\right)=100 \mathrm{~cm}\)

Hence, separationbetween two successivenodes = \(\frac{\lambda}{2}\) = 20 cm.

Question 71. The fundamental frequencies of two identical strings x and y are 450 Hz and 300 Hz respectively. The ratio of the tensions in them is

1. \(\sqrt{\frac{2}{3}}\)
2. \(\frac{9}{4}\)
3. \(\sqrt{\frac{4}{3}}\)
4. \(\sqrt{\frac{3}{2}}\)

Answer: 2. \(\frac{9}{4}\)

Frequency \(f \propto \sqrt{\text { tension }}\).

Hence,

⇒ \(\frac{f_1}{f_2}=\sqrt{\frac{F_1}{F_2}}\)

⇒ \(\frac{F_1}{F_2}=\left(\frac{f_1}{f_2}\right)^2\)

= \(\left(\frac{450 \mathrm{~Hz}}{300 \mathrm{~Hz}}\right)^2\)

= \(\frac{9}{4}\)

Question 72. A uniform string of mass 6 g is suspended from a rigid support and the lower end has a block of mass 2 g attached to it. A wave pulse of wavelength 6 cm produced at the bottom travels up along the string. The wavelength at the top of the string is

1. 6 cm
2. 18 cm
3. 12 cm
4. 24 cm

Answer: 3. 12 cm

Velocity = v = fλ = VF.

Since frequency f and mass per unit length remain constant,

⇒ \(\frac{\lambda_1}{\lambda_2}=\sqrt{\frac{F_1}{F_2}}\)

⇒ \(\frac{6 \mathrm{~cm}}{\lambda_2}=\sqrt{\frac{2 g \omega t}{(6+2) g \omega t}}\)

= \(\frac{1}{2}\)

⇒ \(\frac{6 \mathrm{~cm}}{\lambda_2}=\frac{1}{2}\)

λ₂ = 12 cm

Beats:

Question 73. A source of unknown frequency gives 4 beats per second when sounded with a source of known frequency 250 Hz. The second harmonic of the source of unknown frequency gives five beats per second when sounded with a source of frequency 513 Hz. The unknown frequency is

1. 240 Hz
2. 260 Hz
3. 254 Hz
4. 246 Hz

Answer: 3. 254 Hz

Let f be the unknown frequency of the given source. Since 4 beats per second are produced when sounded with a source of frequency = 250 Hz, therefore,

f = (250 ± 4) Hz

= 246 Hz or 254 Hz.

The second harmonic of this fundamental is 2f = 492 Hz or 508 Hz, which gives 5 beats per second when sounded with a source of 513 Hz.

This is possible with the 2ndharmonic of 508Hz = 2f.

∴ unknown frequency = f = 254Hz.

Question 74. Two identical piano wires, kept under the same tension, have a fundamental frequency of 600 Hz. The fractional increase in the tension in one of the wires which will lead to the occurrence of 6 beats per second when wires vibrate together would be

1. 0.01
2. 0.02
3. 0.03
4. 0.04

Answer: 2. 0.03

The frequency is \(f=\frac{1}{2 l}\left(\frac{F}{\mu}\right)\)

⇒ \(\frac{\Delta f}{f}=\frac{1}{2} \frac{\Delta F}{F}\) (∵ l and μ are constants).

∴ \(\frac{\Delta F}{F}=2 \frac{\Delta f}{f}=\frac{2}{f}\) (beat frequency)

⇒ \(\frac{2}{600}\) x 6

= 0.02.

Question 75. A tuning fork of frequency 512 Hz produces 4 beats per second with the vibrating string of a piano. The beat frequency decreases to 2 beats per second when the tension in the piano string is slightly increased. The frequency of the piano string before the increase in the tension was

1. 510 Hz
2. 514 Hz
3. 516 Hz
4. 508 Hz

Answer: 4. 508 Hz

Let f be the initial frequency of the piano wire. Since the beat frequency with a 512-Hz tuning fork gives 4 beats per second,

f = 512 ± 4

= 516Hz or 508Hz.

On increasing the tension, the beat frequency decreases to 2 beats per second (but the frequency of the guitar wire is increased).

Hence,

f’ = 512 ± 2

= (514 or 510)Hz.

Since f’ > f, the frequency of the guitar wire has increased from its initial value of 508 Hz to 510 Hz.

The frequency before increasing the tensionis 508Hz.

Question 76. Two sound waves with wavelengths 5.0 m and 5.5 m propagate in gas with a velocity of 330 m s^-1. The number of beats per second will be

1. 6
2. 12
3. 0
4. 1

Answer: 1. 6

Given, the velocity of sound = 330 m s^-1; the two wavelengths are λ₁ = 5.0 m and λ₂ = 5.5 m.

The corresponding frequencies are

⇒ \(f_1=\frac{v}{\lambda_1} \text { and } \lambda_2=\frac{v}{\lambda_2}\)

Beat frequency = \(\Delta f=f_1-f_2\)

= \(\frac{v}{\lambda_1}-\frac{v}{\lambda_2}\)

⇒ \(\frac{330}{5} \mathrm{~s}^{-1}-\frac{330}{5.5} \mathrm{~s}^{-1}\)

= \(330\left(\frac{1}{55}\right)\)

= 6 beats per second.

Question 77. Two waves of wavelengths 50 cm and 51 cm produce 12 beats per second. The velocity of sound in air is

1. 340 ms^-1
2. 331 ms^-1
3. 306 ms^-1
4. 360 ms^-1

Answer: 3. 306 ms^-1

Let v = velocity of sound in air.

∴ frequency, \(f_1=\frac{v}{\lambda_1} \text { and } f_2=\frac{v}{\lambda_2}\)

∴ beat frequency is \(12=f_1-f_2\)

= \(v\left(\frac{1}{\lambda_1}-\frac{1}{\lambda_2}\right)\)

⇒ \(v\left(\frac{100}{50 \mathrm{~m}}-\frac{100}{51 \mathrm{~m}}\right)=12 \mathrm{~s}^{-1}\)

⇒ \(v=\left(12 \mathrm{~s}^{-1}\right)\left(\frac{50 \times 51}{100}\right) \mathrm{m}=306 \mathrm{~m} \mathrm{~s}^{-1}\)

Question 78. Two vibrating tuning forks produce waves given by y₁ = 4sin 500πt and y₂ = 4sin 506πf. The number of beats produced in one minute is

1. 60
2. 3
3. 180
4. 360

Answer: 3. 180

The angular frequencies are

ω₁ = 500π = 2πf₁ and ω₂ = 506π = 2πf₂.

∴ f₁ = 250Hz, f₂ = 253Hz.

Hence, the number of beats in one second is f₂ – f₁ = 3 Hz.

So, in one minute, the number of beats = 3 x 60

= 180.

Question 79. Each of the two strings of lengths 51.6 cm and 49.1 cm are tensioned separately by a force of 20 N. The linear mass densities of both strings are the same and equal to 1.0 g m^-1. When both the strings vibrate together the beat frequency is

1. 7 s^-1
2. 3 s^-1
3. 8 s^-1
4. 5 s^-1

Answer: 1. 7 s^-1

Given, l₁ = 51.6 cm, l₂ = 49.1 cm, F₁ = F₂ = 20 N.

Linear mass density = μ₁ = μ₂

= 1.0 g m^-1

= 1 x 10^-3 kg m^-1.

Frequency due to the 1st string,

⇒ \(f_1=\frac{1}{2 l_1} \sqrt{\frac{F}{\mu}}\)

= \(\frac{1}{2\left(51,6 \times 10^{-2} \mathrm{~m}\right)} \sqrt{\frac{20 \mathrm{~N}}{10^{-3} \mathrm{~kg} \mathrm{~m}^{-1}}}\)

= 137 Hz

Similarly, \(f_2=\frac{1}{2 I_2} \sqrt{\frac{F}{\mu}}\)

= 144 Hz

=> beat frequency= Δf = f₂-f₁

= 7Hz

= 7 s^-1.

Question 80. Two sound sources are a finite distance apart. They emit sounds of wavelength λ. An observer situated between them on the line joining the sources approaches one source with speed u. Then the I number of beats heard per second by the observer will be

1. \(\frac{2u}{λ}\)
2. \(\frac{u}{λ}\)
3. \(\frac{u}{2λ}\)
4. \(\frac{λ}{u}\)

Answer: 1. \(\frac{2u}{λ}\)

Let the observer move towards the source S₂, so the apparent frequency will increase from the true frequency of \(f_2=\frac{v+u}{v} f\) and that due to

S₁ will decrease from \(f_1=\frac{v-u}{v} f\), where the velocity of sound v = fλ.

∴ beat frequency is

⇒ \(\Delta f=f_2-f_1\)

= \([(v+u)-(v-u)] \frac{f}{f \lambda}\)

= \(\frac{2 u}{\lambda}\)

Question 81. A closed organ pipe and an open organ pipe of the same length produce four beats per second when sounded together. If the length of the closed pipe is increased, the beat frequency will

1. Increase
2. Decrease
3. Remain the same
4. First, increase then remain constant

Answer: 1. Increase

The fundamental frequency of the openpipe is f_o = \(\frac{v}{2L}\), and that of the closed pipe, f_c = \(\frac{v}{4L}\)

Since f_o > f_c, beat frequency = f_o-f_c.

If the length of the closed pipe is increased, fc will decrease. So the frequency difference (=beat frequency) will increase

Question 82. Two closed organ pipes of lengths 100 cm and 101 cm produce 16 beats in 20 seconds when each pipe is sounded in fundamental mode. The velocity of sound in air is

1. 303 ms^-1
2. 332 ms^-1
3. 323.2 ms^-1
4. 300 ms^-1

Answer: 3. 323.2 ms^-1

The lengths of the closed pipes are l₁ = 100 cm = 1.0 m and l₂ = 101 cm

= 1.01 m.

The frequencies of their fundamental modes of vibration are,

⇒ \(f_1=\frac{v}{4 l_1} \text { and } f_2=\frac{v}{4 l_2}\), where v = speed of sound.

Given thatbeat frequency = \(\frac{16 \text { beats }}{20 \text { seconds }}\)

⇒ \(f_1-f_2=\frac{16}{10}\)

⇒ \(\frac{v}{4}\left(\frac{1}{1.0 \mathrm{~m}}-\frac{1}{1.01 \mathrm{~m}}\right)=\frac{16}{20} \mathrm{~s}^{-1}\)

Simplifying, we get v = 323m s^-1.

Question 83. In a guitar, two strings A and B made of the same material are slightly out of tune and produce beats of frequency 6Hz. When the tension in B is slightly decreased, the beat frequency increases to 7 Hz. If the frequency of A is 530 Hz, the original frequency of B will be

1. 524 Hz
2. 536 Hz
3. 537 Hz
4. 523 Hz

Answer: 1. 524 Hz

Beat frequency = difference in frequencies.

Hence, f_A – f_B = 6Hz or f_B-f_A = 6Hz.

The decrease in tension in string B will decrease f_B, which will increase the beat frequency to 7.

Hence the first option is true.

f_A – f_B = 6Hz

=> 530Hz – f_B = 6Hz

=> f_B = 524Hz

Intensity: Loudness

Question 84. When we hear a sound, we can identify its source from the

1. Frequency of the sound
2. Wavelength of the sound
3. Overtones present in the sound
4. Amplitude of the sound

Answer: 3. Overtones present in the sound

It is the richness of overtones (harmonics) that changes the quality of sound and the source of sound can be identified.

Question 85. Consider sound waves originating from two identical sources S₁ and S₂ reaching a point P in the same phase and producing an intensity I0. If the power of S₁ is reduced by 64% and the phase difference between S₁ and S₂ is varied continuously, the ratio of the maximum and minimum intensities recorded at P will be

1. 16:3
2. 4:3
3. 4:1
4. 16:1

Answer: 4. 16:1

Let l be the intensity originating from two identical sources.

Superposition in the same phase (- 0) produces intensity

⇒ \(I_0=I+I+2 \sqrt{I} \sqrt{I} \cos 0^{\circ}=4 I\)

Next, the changed intensity of one source is reduced to 36%I = 0.361 and for maximum intensity,

⇒ \(I_{\max }=I+0.36 I+2 I \sqrt{0.36} \cos 0^{\circ}\)

⇒ \(=I\left(1.36+\frac{2 \times 6}{10}\right)=2.56 I\)

And, \(I_{\min }=I(1.36-1.20)=0.16 I\)

∴ \(\frac{I_{\max }}{I_{\min }}=\frac{2.56}{0.16}=16\)

Question 86. The intensity of sound from a point source is 1.0 x 10^-8 W m^-2 at a distance of 5.0 m from the source. The intensity at a distance of 25m from the source will be

1. 4.0 x 10^-8 W m^-2
2. 4.0 x 10^-9 Wm^-2
3. 2.0 x 10^-8 W m^-2
4. 4.0 x 10^-10 W m^-2

Answer: 4. 4.0 x 10^-10 W m^-2

Intensity \(I \propto \frac{1}{r^2}, \text { so } \frac{I_1}{I_2}=\frac{r_2^2}{r_1^2}\)

⇒ \(\frac{1.0 \times 10^{-8} \mathrm{~W} \mathrm{~m}^{-2}}{I_2}=\frac{(25 \mathrm{~m})^2}{(5.0 \mathrm{~m})^2}=25\)

⇒ \(I_2=\frac{10^{-8} \mathrm{~W} \mathrm{~m}^{-2}}{25}=4.0 \times 10^{-10} \mathrm{~W} \mathrm{~m}^{-2}\)

Question 87. The sound level at a point 5.0m away from a point source is 40 dB. The sound level at a point 50m away from the source will be

1. 10 dB
2. 20 dB
3. 30 dB
4. 5 dB

Answer: 2. 20 dB

β₁ = 40 dB = 10 log I₁ and β₂ = ? = 10 1og I₂.

∴ β₁ – β₂ = 40 dB – β₂ = \(10 \log \frac{I_1}{I_2} \mathrm{~dB}\)

But \(\frac{I_1}{I_2}=\frac{r_2^2}{r_1^2}=\left(\frac{50 \mathrm{~m}}{5 \mathrm{~m}}\right)^2\) = 100

∴ 40 dB – β₂ = 10 log 10² dB = 20 log 10 = 20 dB.

∴β₂ = 20 dB.

Question 88. If the intensity of sound is tripled, the level of loudness increases by L decibels, where L is

1. 3
2. 4.77
3. 4
4. 4.7

Answer: 2. 4.77

Loudness level = \(\beta=10 \log \frac{I}{I_0}\)

∴ \(\beta_1=10 \log \frac{I}{I_0}\)

and \(\beta_2=10 \log \frac{I_2}{I_0}\)

= \(10 \log \frac{3 I}{I_0}\)

∴ increase in loudness level,

⇒ \(\beta_2-\beta_1=L\)

= \(10\left(\log \frac{3 I}{I_0}-\log \frac{I}{I_0}\right)\)

= \(10 \log 3\)

= 10(0.477)

= 4.77.

Question 89. The sound level at a point 5.0m away from a point source is 40 dB. At what distance from the same source will the loudness reduce to 20 dB?

1. 30 m
2. 10 m
3. 20 m
4. 50 m

Answer: 4. 50 m

Given, L₁ = 10 \(\log \frac{I_1}{I_0}=40 \mathrm{~dB}\). At a distance of x, let the intensity be I₂.

‍∴ \(L_2=10 \log \frac{I_2}{I_0}=20 \mathrm{~dB}\)

Change in level, \(L_1-L_2=10 \log \frac{I_1}{I_2}\)

⇒ \(L_1-L_2=(40-20) \mathrm{dB}=10 \log \left(\frac{x^2}{25 \mathrm{~m}^2}\right)\)

⇒ \(20=10 \log \left(\frac{x}{5 \mathrm{~m}}\right)^2\)

⇒ \(\left(\frac{x}{5 \mathrm{~m}}\right)^2=100\)

x = 50m

Question 90. The loudness level at a point is increased from 50 dB to 60 dB. By what factor is the pressure amplitude increased?

1. √5
2. √10
3. 4
4. 3

Answer: 2. √10

Increase in loudness level,

⇒ \(\beta_2-\beta_1=60 \mathrm{~dB}-50 \mathrm{~dB}=10 \log \frac{I_2}{I_1}\)

⇒ \(10=10 \log \frac{I_2}{I_1} \Rightarrow \frac{I_2}{I_1}=10\)

But as the intensity is proportional to the square of the pressure amplitude, we have

⇒ \(\frac{p_2}{p_1}=\sqrt{\frac{I_2}{I_1}}=\sqrt{10}\)

Question 91. If the pressure amplitude at a point is increased by a factor of (10)^3/2, the loudness level will increase by

1. 10 dB
2. 40 dB
3. 30 dB
4. 20 dB

Answer: 3. 30 dB

Increase in loudness level = \(\beta_2-\beta_1=10 \log \frac{I_1}{I_2}\)

But \(\frac{I_1}{I_2}=\left(\frac{p_1}{p_2}\right)^2=\left(10^{3 / 2}\right)^2=10^3\)

∴ β₂-β₁ = 10 log(10)³

= 30 log 10

= 30 dB

Question 92. A small speaker delivers 2 W of audio output. At what distance from the speaker will one detect 120 dB intensity of sound waves? (Given that the reference intensity of sound = 10^-12 W m^-2.)

1. 40 cm
2. 10 cm
3. 20 cm
4. 30 cm

Answer: 1. 40 cm

Loudness dB is \(\beta=10 \log \frac{I}{I_0} \mathrm{~dB}\)

∴ \(120 \mathrm{~dB}=10 \log \frac{I}{10^{-12}} \mathrm{~dB}\)

= \(10[12+\log I] \mathrm{dB}\)

=> log₁₀I= 0.

Hence,

I = 1 W m^-2.

Intensity at distances

⇒ \(I=\frac{P}{4 \pi r^2}\)

⇒ \(1 \mathrm{~W} \mathrm{~m}^{-2}=\frac{2 \mathrm{~W}}{4 \pi r^2}\)

⇒ \(r=\frac{1}{\sqrt{2 \pi}} \mathrm{m}\)

= 0.399 m

= 0.4m

= 40 cm.

Electrostatics Synopsis

• An electric charge (q or Q) is always associated with electrons and protons which are constituents of atoms.
• The SI unit of charge is the coulomb (symbol: C). The elementary charge is e = 1.6 x 10^-19 C.
• 1 C of charge is contained in 6.25 x 10¹⁸ electrons (or protons).
• A charge is quantized as Q = ne, where n = 1, 2, 3,…
• A charging process involves a gain or loss of electrons. An object becomes positively charged due to the loss of electrons and negatively charged due to the gain of electrons.
• Coulomb’s law: \(F=\frac{1}{4 \pi \varepsilon_0}\left(\frac{q_1 q_2}{r^2}\right)=K\left(\frac{q_1 q_2}{r^2}\right)\)
  where ε₀ = permittivity of free space
  = 8.85 x 10^-12 F m^-1 (or C² N^-1 m^-2)
  and \(K=\frac{1}{4 \pi \varepsilon_0}\)
• = \(9 \times 10^9 \mathrm{~N} \mathrm{~m}^2 \mathrm{C}^{-2}\)
• Principle of superposition: The net force on any charge q due to other charges in space equals the vector sum of all the forces on it on account of the constituent charges in space. Thus,
  ⇒ \(\vec{F}=\vec{F}_1+\vec{F}_2+\ldots=\frac{1}{4 \pi \varepsilon_0}\left[q\left(\frac{q_1}{r_1^2} \cdot \hat{r}_1+\frac{q_2}{r_2{ }^2} \cdot \hat{r}_2+\ldots\right)\right]\)
• The electric field (or field strength or field intensity) \(\vec{E}\) is defined as the force per unit test charge \(\left(\vec{E}=\frac{\vec{E}}{q_0}\right)\). Its SI unit is NC^-1(≡V m^-1).
  The electric field due to a single charge Q (monopole) is
  ⇒ \(\vec{E}=\frac{1}{4 \pi \varepsilon_0}\left(\frac{Q}{r^2} \cdot \hat{r}\right)\)
• Electric dipole: Two equal and opposite point charges separated at a short distance constitute an electric dipole.
• The electric dipole moment \((\vec{p})\) is the product of one charge |± q| and the separation (δl) between the charges. Thus,
  ⇒ \(|\vec{p}|=|q| \delta l \Rightarrow \vec{p}=|q| \vec{\delta} l\)
  Here \(\vec{p}\) is a vector directed from the negative charge to the positive charge, and its SI unit is the coulomb meter (C m).
• Dipole field:
  • At a point on the axis,
    ⇒ \(\vec{E}_{\text {axial }}=\frac{1}{4 \pi \varepsilon_0}\left[\frac{2 \vec{p} r}{\left(r^2-l^2\right)^2}\right] \approx \frac{1}{4 \pi \varepsilon_0}\left(\frac{2 \vec{p}}{r^3}\right)\)
  • At an equatorial point,
    ⇒ \(\vec{E}_{\text {equatorial }}=\frac{1}{4 \pi \varepsilon_0}\left[\frac{-\vec{p}}{\left(r^2+l^2\right)^{3 / 2}}\right] \approx \frac{1}{4 \pi \varepsilon_0}\left(\frac{-\vec{p}}{r^3}\right)\)
  • At any point P(r, θ),
    ⇒ \(E=\frac{1}{4 \pi \varepsilon_0}\left(\frac{p}{r^3} \sqrt{1+3 \cos ^2 \theta}\right)\)
• Torque on a dipole in a uniform field \(\vec{E}\):
  ⇒ \(\vec{\tau}=\vec{p} \times \vec{E} \Rightarrow \tau=p E \sin \theta\)
• Flux of an electric field:
  ⇒ \(\phi=\int \vec{E} \cdot \overrightarrow{d A}=\int E d A \cos \theta\)
  Φ is a scalar quantity, and its SI unit is V m (≡ N m² C^-1).
• Gauss’s law: \(\Phi=\frac{1}{\varepsilon_0}\) (net charge enclosed).
• Expressions for an electric field:
  • For a line charge, \(\vec{E}=\frac{\lambda}{2 \pi \varepsilon_0 r} \cdot \hat{r}\)
  • For a plane charge sheet, \(\vec{E}=\frac{\sigma}{2 \varepsilon_0} \cdot \hat{n}\)
  • For a uniformly charged spherical shell of radius R,
    ⇒ \(\vec{E}=\left\{\begin{array}{cc}
    \frac{1}{4 \pi \varepsilon_0}\left(\frac{Q}{r^2} \cdot \hat{r}\right) & \text { for } r>R \\
    \frac{1}{4 \pi \varepsilon_0}\left(\frac{Q}{R^2} \cdot \hat{R}\right) & \text { for } r=R \\
    0 & \text { for } r<R
    \end{array}\right.\)
  • For a solid sphere of radius R having a total charge of Q distributed uniformly,
    ⇒ \(\vec{E}=\left\{\begin{array}{l}
    \frac{1}{4 \pi \varepsilon_0}\left(\frac{Q}{r^2} \cdot \hat{r}\right) \text { for } r>R \\
    \frac{1}{4 \pi \varepsilon_0}\left(\frac{Q}{R^2} \cdot \hat{R}\right) \text { for } r=R \\
    \frac{1}{4 \pi \varepsilon_0}\left(\frac{Q r}{R^3} \cdot \hat{r}\right) \text { for } r<R
    \end{array}\right.\)
• Potential energy between two charges:
  ⇒ \(U=\frac{1}{4 \pi \varepsilon_0}\left(\frac{q_1 q_2}{r}\right)\)
• The potential energy of a system of three charges:
  ⇒ \(U_{\text {sys }}=U_{12}+U_{13}+U_{23}=\frac{1}{4 \pi \varepsilon_0}\left(\frac{q_1 q_2}{r_{12}}+\frac{q_1 q_3}{r_{13}}+\frac{q_2 q_3}{r_{23}}\right)\)
• Potential at a point in an electric field:
  V = \(\frac{U}{q}\) = potential energy per unit test charge.
  • The potential due to a monopole (single charge) Q is
    ⇒ \(V=\frac{1}{4 \pi \varepsilon_0}\left(\frac{Q}{r}\right)\)
  • The potential due to a system of charges is
    ⇒ \(V=V_1+V_2+\ldots=\frac{1}{4 \pi \varepsilon_0}\left(\frac{Q_1}{r_1}+\frac{Q_2}{r_2}+\ldots\right)\)
  • The potential due to a uniformly charged ring of radius R is
    ⇒ \(V=\left\{\begin{array}{l}
    \frac{1}{4 \pi \varepsilon_0}\left(\frac{Q}{\sqrt{R^2+r^2}}\right) \text { atP } \\
    \frac{1}{4 \pi \varepsilon_0}\left(\frac{Q}{R}\right) \text { atO }
    \end{array}\right.\)

• Potential due to an electric dipole:
  • At an axial point, V = \(V=\frac{1}{4 \pi \varepsilon_0}\left(\frac{p}{r^2}\right)\)
  • At an equatorial point, V = 0.
  • At any point \(\mathrm{P}(r, \theta), V=\frac{1}{4 \pi \varepsilon_0}\left(\frac{p \cos \theta}{r^2}\right)\)
• Potential due to a uniformly charged spherical shell of radius R:
  • At an external point, \(V=\frac{1}{4 \pi \varepsilon_0}\left(\frac{Q}{r}\right)\)
  • On the surface of the shell, \(V=\frac{1}{4 \pi \varepsilon_0}\left(\frac{Q}{R}\right)\)
  • Inside theshell, \(V=\frac{1}{4 \pi \varepsilon_0}\left(\frac{Q}{R}\right)\) (the same as thaton thesurface).
• Potential due to a spherical charge distribution:
  • At an external point, \(V=\frac{1}{4 \pi \varepsilon_0}\left(\frac{Q}{r}\right) \text { for } r>R\)
  • On the surface of the sphere, \(V=\frac{1}{4 \pi \varepsilon_0}\left(\frac{Q}{R}\right)\)
  • Inside the sphere at a distance r (where r < R) from the center,
    ⇒ \(V=\frac{1}{4 \pi \varepsilon_0}\left[\frac{Q\left(3 R^2-r^2\right)}{2 R^3}\right]\)
• The potential energy of an electric dipole in a uniform electric field \(\vec{E}\):
  ⇒ \(U=-\vec{p} \cdot \vec{E}=-p E \cos \theta\)
• The potential difference in an electric field:
  ⇒ \(V_2-V_1=-\int_1^2 \vec{E} \cdot \vec{d} r\)
• Relation between the field \(\vec{E}\) and the potential (V):
  ⇒ \(|\vec{E}|=-\frac{d V}{d r}, \text { where } E_x=-\frac{\partial V}{\partial x}, E_y=-\frac{\partial V}{\partial y} \text { and } E_z=-\frac{\partial V}{\partial z}\)
• The electric field in the material of a conductor is \(\vec{E}\) = 0, and that in a dielectric medium of dielectric constant K is \(\vec{E}=\frac{\vec{E}_0}{K} \text {, where } \vec{E}_0\) is the external field strength.
• Dielectric strength: A sufficient increase in the external electric field reduces the ability of atoms and molecules to hold the outer electrons, which get detached to cause a dielectric breakdown. The maximum electric field that- the dielectric can sustain without breaking down is called its dielectric strength. For dry air, the dielectric strength is around 3 x 10⁶ V m^-1.
• Electrical capacitance, C = \(\frac{Q}{V}\). Its SI unit is the farad (symbol F).
  Thus,
  1F = \(\frac{1C}{1V}\)
• The capacitance of a sphere of radius R is C = 4nε₀R
• Electrostatic energy, \(U=\frac{1}{2} C V^2=\frac{Q^2}{2 C}\)
• The capacitance of a parallel-plate capacitor:
  • With air, \(C_0=\frac{\varepsilon_0 A}{d}\)
  • With a dielectric, \(C=K C_0=\frac{K \varepsilon_0 A}{d}\)
  • With a thin dielectric plate, \(C=\frac{\varepsilon_0 A}{d-t+\frac{t}{K}} \text { for } t<d\)
  • With a thin metal plate, \(C=\frac{\varepsilon_0 A}{d-t} \text { for } t<\underline{d} \text { and } K_{\text {metal }}=\infty\)
• Equivalent capacitance:
  • In series, \(\frac{1}{C_s}=\frac{1}{C_1}+\frac{1}{C_2}+\ldots\)
  • In parallel, C_p = C₁ + C₂ + ….
    C_s is less than the least in the combination, C_p is greater than the greatest in the combination.