WBCHSE Class 12 Physics Notes For Chapter 2 Electric Field

Electrostatics

Electric Field Force Of Attraction Or Repulsion Between Two Charges Couloms’s Law

We know that two like charges repel each other and unlike charges attract each other. Coulomb’s law gives the force between two charges.

This law is valid:

1. Only for point charges, i.e., when the sizes of the charged bodies are negligible in comparison to their distance of separation,
2. Only for distances greater than 10^-15 m (nuclear distance)
3. For charges at rest with respect to the observer.

Coulomb’s law: The force of attraction or repulsion between two point charges at rest is directly proportional to the product of the charges and inversely proportional to the square of the distance between them.

The force acts along the line joining the two charges. It depends on the nature of the intervening medium.

Read and Learn More Class 12 Physics Notes

Suppose two point charges q₁ and q₂ are at rest at a distance r from each other. If F is the magnitude of the electrostatic force acting between them, then according to Coulomb’s law

F ∝ q₁q₂, when r is constant

and \(P \propto \frac{1}{r^2}\), when q1 and q2 are constant

∴ \(F \propto \frac{q_1 q_2}{r^2}\), when q1, q2 and r variables

or, \(F=k^{\prime} \frac{q_1 q_2}{r^2}\)

where k’ is a constant of proportionality. Its value depends on the nature of the intervening medium and also on the units. In which the quantities F, q₁,q₂ and r are measured. Sometimes the constant K’ is referred to as the electrostatic constant or Coulomb constant.

1. In CGS system:

⇒ \(F=\frac{q_1 q_2}{r^2}\) ( for vacuum or air)….(2)

2. In SI, For vacuum or air:

⇒ \(k^{\prime}=\frac{1}{4 \pi \epsilon_0}\)

where ∈₀ is the permittivity of vacuum or air or permittivity of free space.

Hence, \(F=\frac{1}{4 \pi \epsilon_0} \cdot \frac{q_1 q_2}{r^2}\) (for vacuum or air)…..(3)

i.e., \(\epsilon_0=\frac{1}{4 \pi} \cdot \frac{q_1 q_2}{F r^2}\)……(4)

In this system, the charge is expressed in coulomb (C), force in newton (N) and distance in metre (m).

In this case, ∈₀ = 8.854 x 10^-12 C2.N^-1.m^-2

∴ \(\frac{1}{4 \pi \epsilon_0}=9 \times 10^9 \mathrm{~N} \cdot \mathrm{m}^2 \cdot \mathrm{C}^{-2}\)

Hence, from equation (3) \(F=9 \times 10^9 \frac{q_1 q_2}{r^2}\)….(5)

For any other intervening medium,

⇒ \(\dot{F}=\frac{1}{4 \pi \epsilon} \cdot \frac{q_1 q_2}{r^2}\)….(6)

where e is the permittivity of the medium

Again, e = k∈₀, where K is the relative permittivity or dielectric constant of the medium

In the CGS system, ∈₀ = l, so, ∈ = K, i.e., the permittivity of a medium is numerically equal to its dielectric constant. But permittivity has the unit statC² dyn^-1 cm^-2.

For Example, the dielectric constant of mica is 5.4 and the permittivity of mica is 5.4 statC². dyn^-1.cm^-1.

But in SI, the value of ∈₀ is 8.854 x 10^-12 C².N^-1.m^-2 and Comparison between electrostatic force and grav- hence the magnitudes of the dielectric constant and the permit-rotational force: Both electrostatic and gravitational forces activity of a medium are not the same.

So, in SI, the permittivity of mica,

∈ = K ∈₀

= 5.4 x 8.854 x 10^-12 C². N^-1.m^-2

= 4.78 x 10^-11 C². N^-1.m^-2

Note that, the permittivity of any medium is greater than that of vacuum. So the force of attraction or repulsion between two charges in any medium is less than that in a vacuum.

Only dry air has experimentally been observed to have almost the same permittivity (∈₀) as that of vacuum.

Thus, the general formula of Coulomb’s law for any medium is given by

⇒ \(F=\frac{1}{4 \pi \kappa \epsilon_0} \cdot \frac{q_1 q_2}{r^2}\)…….(7)

From equation (4) we come to know the dimension of ∈₀,

⇒ \(\left[\epsilon_0\right]=\frac{\left[q_1 q_2\right]}{[F]\left[r^2\right]}\)

= \(\frac{(\mathrm{IT})^2}{\mathrm{MLT}^{-2} \cdot \mathrm{L}^2}\)

= \(\left.\mathrm{M}^{-1} \mathrm{~L}^{-3} \mathrm{~T}^4\right|^2\)

It may be noted that in static electricity Coulomb’s law is an analogue of Newton’s law of gravitation. Of course, in the case of gravitation, this force is always attractive in nature and the proportionality constant (G) is universal.

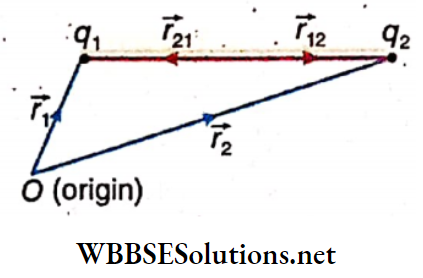
Vector form of Coulomb’s law: Suppose, \(\vec{r}_1\) are the position vectors of charges\(\vec{r}_2\), respectively. So the force acting on q₂ due to q₁,

⇒ \(\vec{F}_{12}=\frac{1}{4 \pi \epsilon_0} \cdot \frac{q_1 q_2}{r_{21}^2} \cdot \hat{r}_{21}\)

= \(\frac{1}{4 \pi \epsilon_0} \cdot \frac{q_1 q_2}{r_{21}^3} \cdot \vec{r}_{21}\)……(8)

where \(\vec{r}_12\) is the position vector of 42 with respect to q1,

i.e., \(\vec{r}_{21}=\vec{r}_2-\vec{r}_1=r_{21} \hat{r}_{21}\)

Similarly, the force acting on q₁ due to q₂,

⇒ \(\vec{F}_{21}=\frac{1}{4 \pi \epsilon_0} \cdot \frac{q_1 q_2}{r_{12}^2} \cdot \hat{r}_{12}\)

= \(\frac{1}{4 \pi \epsilon_0} \cdot \frac{q_1 q_2}{r_{12}^3} \cdot \vec{r}_{12}\)

Each of the equations (8) and (9) represents the vector form of Coulomb’s law.

As, \(\vec{r}_{12}=-\vec{r}_{21} \text {, and } \hat{r}_{12}=-\hat{r}_{21} \text {, we have } \vec{F}_{12}=-\vec{F}_{21}\)

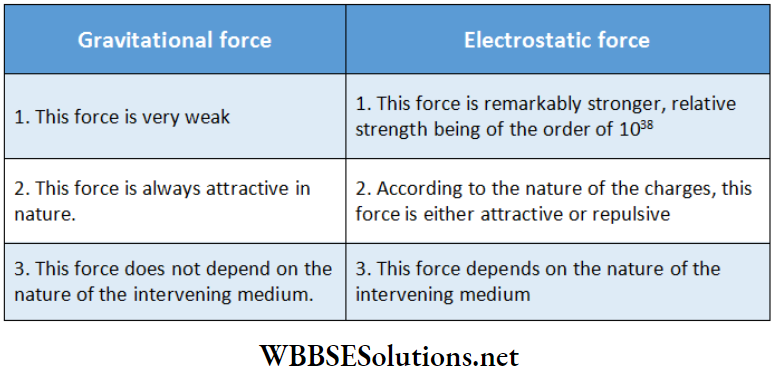
Comparison between electrostatic force and gravitational force:

Both electrostatic and gravitational forces act between two charged bodies. Similarities and dissimilarities between these two forces are given below.

Similarities:

1. Both forces are inversely proportional to the square of the distance between the two bodies.
2. Both the forces are conservative, Le., work done by these forces is independent of path.
3. Both the forces act in a vacuum as well.
4. Both the forces are central forces where the force is expressed as \(\vec{F}=-F(r) \hat{r}\)
5. Both the forces are mutually interactive, i.e., \(\vec{F}_{21}=-\vec{F}_{12}\)
6. Both the forces are spherically symmetric [function of r only].

Dissimilarities:

Unit of Charge:

CGS unit: Coulomb’s law, in the CGS system, is expressed as, \(F=\frac{q_1 q_2}{r^2}\) If we put F = 1 dyne, r = 1 cm, and q₁ = q₂ = q, we get from equation (2),

q₂=1  or, q = ±1

Definition: If an electric repulsive force of 1 dyne arises between two similar point charges kept 1 cm apart in air, then each point charge is called a unit charge. This unit is known as I esu or 1 statcoulomb (state).

SI: Coulomb’s law In SI for vacuum or air is expressed as

⇒ \(F=\frac{1}{4 \pi \epsilon_0} \cdot \frac{q_1 q_2}{r^2}\)

Now, putting F = 9 x 10⁹ N, r = 1 m,

q₁= q₂ = q and \(\frac{1}{4 \pi \epsilon_0}\) = 9 X 10⁹ N.m².C^-2 we get,

⇒ \(9 \times 10^9=9 \times 10^9 \times \frac{q \times q}{(1)^2}\)

or, q₂ = 1 or, q = ±1

Definition: If an electric repulsive force of 9 x 109 N arises between two similar point charges kept 1 m apart in vacuum or air, the amount of each charge is 1 C.

Relation between the units of charge:

1 C = 3 X 10⁹ state (precisely, 2.99792458 x 10⁹ esu)

An electromagnetic unit or emu is another unit of charge, defined in the discussions of electromagnetism. Its relations with the other units are,

1 emu of charge = 10 C = 3 X 10^-10 statC

In atomic physics, the charge of an electron or a proton is taken as the unit of measurement of charge. This is called an electronic charge unit (e).

1 electronic unit of charge is equal to the charge of an electron or a proton.

1e = 4.8 x 10^-10 statC = 1.6 X 10^-19 C

±e is the least amount of charge that can exist in nature. No charge exists which is not an integral multiple of ±e.

The force between Multiple Charges Superposition Principle:

The principle of superposition: The total force acting on a charge due to a number of interacting charges around it is the vector sum of the individual forces acting on that charge due to each of the other charges.

Individual forces are calculated on Coulomb’s law. The force with which two charges interact does not change by the presence of other charges. Coulomb’s law gives the electrostatic force between two point charges.

In this case, it is assumed that other charges are not present near the two charges. If there are a number of interacting charges, the force on a particular charge may be obtained by the principle of superposition.

Suppose a system contains n number of point charges q₁, q₂, q₃,….qn placed in vacuum or in air. So by the principle of superposition, the total force acting on qx exerted by all the other charges is given by

⇒ \(\vec{F}_1=\vec{F}_{21}+\vec{F}_{31}+\vec{F}_{41}+\cdots+\vec{F}_{n 1}\)

Here, \(\vec{F}_{21}\) = force acting on q₁ due to q₂

\(\vec{F}_{31}\) = force acting on q₁ due to q₃

………………………………………………..

\(\vec{F}_{n1}\) = force acting on q₁ due to q_n

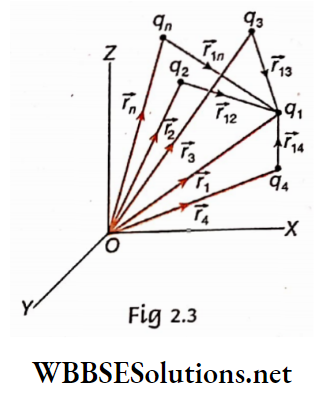
According to Coulomb’s law,

⇒ \(\vec{F}_{21}=\frac{1}{4 \pi \epsilon_0} \frac{q_1 q_2}{r_{12}^2} \hat{r}_{12}\)

⇒ \(\hat{r}_{12}\) is the position vector of charge q₁ with respect to q₂

Similarly,

\(\vec{F}_{31}=\frac{1}{4 \pi \epsilon_0} \frac{q_1 q_3}{r_{13}^2} \hat{r}_{13} \text {; }\) \(\vec{F}_{41}=\frac{1}{4 \pi \epsilon_0} \frac{q_1 q_4}{r_{14}^2} \hat{r}_{14}\)

……………………………………………………..

\(\vec{F}_{n 1}=\frac{1}{4 \pi \epsilon_0} \frac{q_1 q_n}{r_{1 n}^2} \hat{r}_{1 n}\)

∴ \(\vec{F}_1=\frac{1}{4 \pi \epsilon_0}\left[\frac{q_1 q_2}{r_{12}^2} \hat{r}_{12}+\frac{q_1 q_3}{r_{13}^2} \hat{r}_{13}+\cdots+\frac{q_1 q_n}{r_{1 n}^2} \hat{r}_{1 n}\right]\)….(1)

Similarly, the total force acting on q2 exerted by all the other charges is given by,

⇒ \(\vec{F}_2=\vec{F}_{12}+\vec{F}_{32}+\vec{F}_{42}+\cdots+\vec{F}_{n 2}\)

⇒ \(\begin{aligned}
\frac{1}{4 \pi \epsilon_0}\left[\frac{q_1 q_2}{r_{21}^2} \hat{r}_{21}+\frac{q_2 q_3}{r_{23}^2} \hat{r}_{23}+\frac{q_2 q_4}{r_{24}^2} \hat{r}_{24}\right. & \\
& \left.+\cdots+\frac{q_2 q_n}{r_{2 n}^2} \hat{r}_{2 n}\right]
\end{aligned}\)……(2)

In general, if \(\vec{F}_i\). be the total force on the i th charge of the configuration, then,

⇒ \(\vec{F}_i=\frac{1}{4 \pi \epsilon_0} \sum_{\substack{j=1 \\ j \neq i}}^n \frac{q_i q_j}{r_{j l}^2} \hat{r}_{j l}\)……(3)

Here, \(\hat{r}_{j i}\). is the position vector of the charge with respect to aj.

In the CGS system, the above equation is

⇒ \(\vec{F}_i=\sum_{\substack{j=1 \\ j \neq i}}^n \frac{q_i q_j}{r_{j i}^2} \hat{r}_{j i}\)….(4)

Force due to Continuous Charge Distribution:

Although charges are quantised we can consider a large collection of charges in a region as a continuous distribution.

The continuous distribution of charges may be of three types:

1. One dimensional,
2. Two-dimensional
3. Three-dimensional.

These distributions of charges are called linear charge distribution, surface charge distribution and volume charge distribution, respectively.

1. Linear charge distribution: Let there be a continuous distribution of charge along a straight line or a curved line placed in a vacuum or in air. The linear charge density = \(\left(\frac{\text { charge }}{\text { length }}\right)=\lambda\). Suppose, dl is an infinitesimally small element of this line charge distribution. Charge .on the element dl is dq = μdl.

Suppose, a test point charge q₁ is at a distance r from the small element dl.

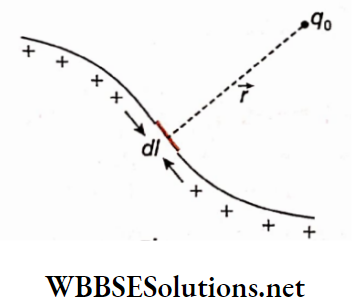
Now, the force acting on q₀ due to the charge dq is given by,

⇒ \(d \vec{F}=\frac{1}{4 \pi \epsilon_0} \frac{q_0 d q}{r^2} \hat{r} ; \text { where } \hat{r}=\frac{\vec{r}}{r}=\text { unit vector along } \vec{r}\)

⇒ \(\frac{1}{4 \pi \epsilon_0} \frac{q_0 \lambda d l}{r^2} \hat{r}\)

∴ The total force acting on q0 due to the whole line charge is given by

⇒ \(\vec{F}=\frac{q_0}{4 \pi \epsilon_0} \int_l \frac{\lambda}{r^2} \hat{r} d l\)…..(1)

l indicates the line along which the Integration extends.

In the CGS system, the above equation Is (replacing \(\epsilon_0 \text { by } \frac{1}{4 \pi}\))

⇒ \(\vec{F}=q_0 \int_l \frac{\lambda}{r^2} \hat{r} d l\)……(2)

Til Surface charge distribution: Let there be a continuous distribution of charge over a surface placed in a vacuum or in air. The surface charge density = \(\left(\frac{\text { charge }}{\text { area }}\right)=\sigma\) Suppose, ds be an infinitesimally small surface element of this surface distribution of charge. Charge on the surface element ds is dq = σds

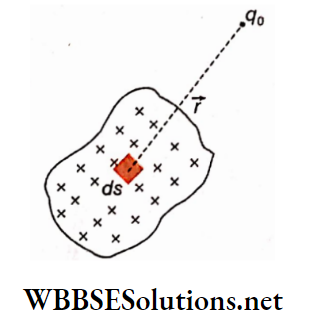
Suppose, a test point charge q0 is at a distance r from the small surface element ds.

Now, the force acting on qQ due to the charge dq is given by

⇒ \(d \vec{F}=\frac{1}{4 \pi \epsilon_0} \frac{q_0 d q}{r^2} \hat{r}=\frac{1}{4 \pi \epsilon_0} \frac{q_0 \sigma d s}{r^2} \hat{r}\)

∴ The total force acting on q0 due to the whole surface the charge distribution is given by,

⇒ \(\vec{F}=q_0 \int_s \frac{\sigma}{r^2} \hat{r} d s\)….(3)

s indicates the surface over which the integration extends.

In the CGS system, the above equation is,

⇒ \(\vec{F}=q_0 \int_s \frac{\sigma}{r^2} \hat{r} d s\)….(4)

3. Volume or spatial charge distribution: Let there be a continuous distribution of charge over a volume (such cube or a sphere) placed in a vacuum or in air. The volume density of charge \(\left(\frac{\text { charge }}{\text { volume }}\right)=\rho\)

Suppose, dv is an infinitesimally small volume element of till volume distribution of charge. Charge on the volume element dv is dq = pdv.

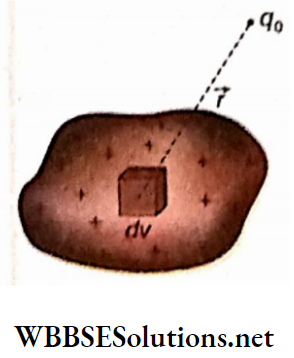
Suppose, a test point charge q₀ is at a distance r from the small volume element dv.

Now, the force acting on q₀ due to the charge dq is
given by,

⇒ \(d \vec{F}=\frac{1}{4 \pi \epsilon_0} \frac{q_0 d q^2}{r^2} \hat{r}=\frac{1}{4 \pi \epsilon_0} \frac{q_0 \rho d v}{r^2} \hat{r}\)

∴ The total force acting on q0 due to the whole volume of the charge distribution is given by

⇒ \(\vec{F}=\frac{q_0}{4 \pi \epsilon_0} \int_v \frac{\rho}{r^2} \hat{r} d v\)….(5)

v indicates the volume to which the integration extends.

In the CGS system, the above equation is,

⇒ \(\vec{F}=q_0 \int_v \frac{\rho}{r^2} \hat{r} d v\)……(6)

Electrostatics

Electric Field Numerical Examples

Example 1. A 196 dyn force acts between two point charges separated by a distance of 6 cm. If the magnitude of one charge is 4 times that of the other, calculate the charges on each.
Solution:

From Coulomb’s law, \(F=\frac{q_1 q_2}{r^2}\)

Suppose, q₁ = q; so, q₂ = 4q

Given, F = 196 dyn; r = 6cm

∴ \(196=\frac{q \times 4 q}{6^2} \text { or, } q^2=49 \times 6^2\)

or, q = 42 statC

∴ The other charge

4 X 42 = 168 statC.

Example 2. In free space, the force between two charges separated by a constant distance Is 9 dyn. When the charges are placed In a medium of dielectric constant K, the force between them becomes 4 dyn. Then the value of k.
Solution:

Let the two charges are q₁ and q₂, and they are separated by a distance r.

Prom Coulomb’s law, \(F_1=\frac{q_1 q_2}{4 \pi \epsilon r^2}\) [e = permittivity of the medium]

and \(F_2=\frac{q_1 q_2}{4 \pi \epsilon_0 r^2}\) [∈₀ = permittivity of free space]

∴ \(\frac{F_2}{F_1}=\frac{\epsilon}{\epsilon_0}=\kappa\)

or, k = \(\frac{9}{4}\)

= 2.25

Example 3. A pith ball having charge -20 esu and mass 0.1 g remains suspended at rest In air 2 cm below an Insulated charged sphere. What Is the amount of charge on the sphere and what is the nature of that charge? [Given, g = 980 cm s^-2 ]
Solution:

Suppose, the charge on the sphere = q

Since the pith ball is at rest, the weight of the pith ball = upward attractive force on the pith ball

or, \(0.1 \times 980=\frac{20 \times q}{(2)^2}\)

or, q = 19.6

Since the pith ball is at rest in space, the charge on the sphere is of the opposite nature to that of the pith ball. The nature of the charge on the pith ball is negative. So the charge on the sphere is positive.

∴ Charge on the sphere = +19.6 esu.

Example 4. The weight of each of the two small metal spheres having the same amount of charge is 3g. The two spheres are suspended with two threads of length 13 cm each from a point. Due to mutual repulsion, they are at rest at a distance of 10 cm. Determine the charge on each sphere and tension in the threads.
Solution:

Let the two spheres at rest be at A and B.

Here, AB = 10 cm. The length of each thread

= OA = OB

= 13 cm, 6 = angle made by each thread with the vertical,

T = tension in each thread and q = charge on each sphere

In equilibrium, three forces an on each ball These are

1. Weight of the ball = mg,

2. Tension in thread = T

3. Mutual force of electrostatic repulsion = F.

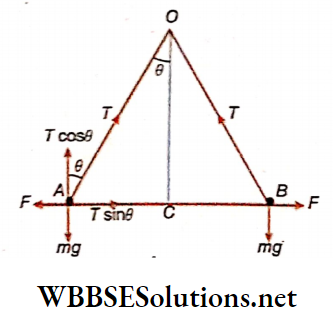
In the equilibrium of any sphere, the vertical component Tcosθ of the tension balances the weight mg of the sphere.

Tcosθ = mg

or, T x \(\frac{12}{13}\) = 3 x 980 [∵ \(\sin \theta=\frac{5}{13}, \cos \theta=\sqrt{1-\frac{25}{169}}=\frac{12}{13}\)]

or, \(T=\frac{3 \times 980 \times 13}{12}\) = 3185 dyn.

Again, the repulsive force F is balanced by the horizontal component Tsinθ of tension in the thread.

∴ F = Tsinθ

or, \(\frac{q \times q}{(10)^2}=3185 \times \frac{5}{13}\)

or, q₂ = 245 x 500

or, q = 350 esu

Example 5. Two similar balls are suspended from a point by two silk threads, each of length l. Each hall of mass m contains q amount of charge. If the angle between the two threads is very small, show that the distance between the centres of the two balls at equilibrium is \(x=\left(\frac{2 q^2 l}{m g}\right)^{1 / 3}\).
Solution:

Let A and B be the equilibrium positions of the two balls, OA = OB = l and AB = x. Suppose, the angle of inclination of the two threads with the vertical = 0.

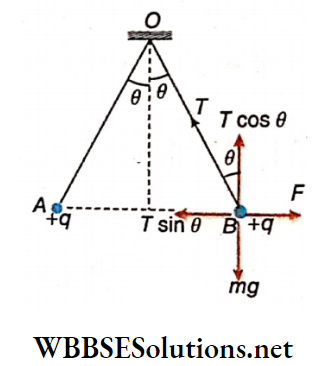
At equilibrium, three forces act on each ball:

1. Weight of the ball mg;

2. Tension in the string T

3. Multual repulsive force between the charged balls, F.

Here, \(T \sin \theta=F=\frac{q^2}{x^2}\); T cosθ = mg

∴ \(\tan \theta=\frac{q^2}{m g x^2}\)

or, \(\sin \theta=\frac{q^2}{m g x^2}\) [∵ θ is small, tan θ ≈ sinθ]

or, \(\frac{x / 2}{l}=\frac{q^2}{m g x^2} \quad \text { or, } x=\left(\frac{2 q^2 l}{m g}\right)^{1 / 3}\)

Example 6. A+250 esu of charge P. is on the line joining the other two charges Q (+50 esu of charge) and R (-300 esu of charge), in between them. The distance of P from Q is 5 cm and from R, it is 10 cm. What is the resultant force acting on P?

Solution:

The force acting on P due to the charge Q

⇒ \(\frac{50 \times 250}{(5)^2}=500 \text { dyn; along } \overrightarrow{P R}\)

The force acting on P due to the charge R

⇒ \(\frac{250 \times 300}{(10)^2}=750 \mathrm{dyn} ; \text { along } \overrightarrow{P R}\)

∴ The resultant force acting on the charge P

= 500 + 750 = 1250 dyn; along \(\overrightarrow{P R}\)

Example 7. Three positive charges 3esu, 4 esu and 5 esu are placed at the vertice of an equilateral triangle of side 10cm. What is the force acting on the largest charge?
Solution:

Three charges are placed at the comers of the equilateral triangle ABC. The largest charge, i.e., 5 esu is situated at point C.

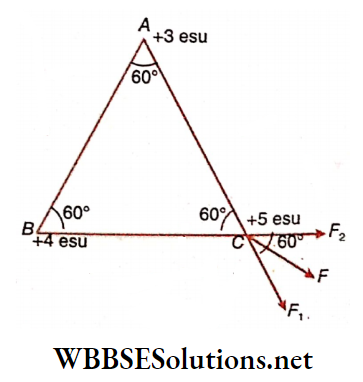
Force acting on the charge at C due to the charge at A,

⇒ \(F_1=\frac{3 \times 5}{(10)^2}=0.15 \text { dyn; along } \overrightarrow{A C}\)

Force acting on the charge at C due to the charge at B,

⇒ \(F_2=\frac{4 \times 5}{(10)^2}=0.2 \mathrm{dyn} \text {; along } \overrightarrow{B C}\)

∴ The resultant force acting on the charge at C,

⇒ \(F=\sqrt{F_1^2+F_2^2+2 F_1 F_2 \cos 60^{\circ}}\)

⇒ \(\sqrt{(0.15)^2+(0.2)^2+2 \times 0.15 \times 0.2 \times 0.5}\)

⇒ \(\sqrt{0.0925}\)

= 0.304 dyn

Example 8. Two particles are placed in the air at a distance of 10 cm. How will you distribute 20 esu charge between the two particles so that the mutual force of repulsion between them will be maximum? What is the magnitude of this maximum force?
Solution:

Suppose, the charges on the particles are q esu and (20- q ) esu.

The force of repulsion between the two particles,

⇒ \(F=\frac{q \times(20-q)}{(10)^2} \mathrm{dyn}\)

For maximum value of \(F, \frac{d F}{d q}=0\)

∴ \(\frac{d F}{d q}=\frac{1}{100} \frac{d}{d q}\left(20 q-q^2\right)\)

= 0

or, 20- 2q = 0 or, q = 10

So one particle contains 10 esu of charge and the other (20- 10) or 10 esu of charge, i.e., the total charge is equally shared between them.

Maximum force, \(F_{\max }=\frac{10 \times 10}{(10)^2}=1 \mathrm{dyn}\)

= 1 dyn

Example 9. Three identical small spheres each of mass 0.1 suspended by three silk threads each of length 20 cm from a certain point How much charge should be given to each sphere so that each thread will make an angle of 30° with the vertical? You may suppose that each sphere has an equal charge.
Solution:

The spheres are identical and each sphere has equal charge. They will repel each other with equal force.

So in the position of equilibrium, they will form an equilateral triangle ABC in the horizontal plane.

Here, the length of the thread OA = OB = OC = 20 cm, mass of each sphere,

m = 0.1 g, angle of inclination of the thread with the vertical, 6 = 30°.

Suppose, the charge of each sphere is q.

Clearly, the vertical line OG passes through the centre of gravity of the triangle ABC. Suppose, the length of each side of the triangle = a.

Median, \(A D=\sqrt{a^2-\frac{a^2}{4}}=\frac{\sqrt{3}}{2} a\)

Now, \(A G=\frac{2}{3} A D=\frac{2}{3} \cdot \frac{\sqrt{3}}{2} a=\frac{a}{\sqrt{3}}\)

Again, \(\sin 30^{\circ}=\frac{A G}{O A} \quad\)

or, \(A G=O A \sin 30^{\circ}\)

= \(20 \times \frac{1}{2}\)

= 10 cm

∴ \(\frac{a}{\sqrt{3}}=10\)

or, \(a=10 \sqrt{3}\)cm

Force of repulsion between any two balls, \(F=\frac{q^2}{a^2}\)

On sphere A two equal forces of repulsion F act due to the spheres B and C. Suppose, the resultant of these two equal forces is R.

∴ R² =F² + F² + 2F.F cos60° [∵ ABAC = 60° , .. internal angle between F and F = 60°

= F² + F² + F² = 3F²

or, \(R=\sqrt{3} F=\sqrt{3} \cdot \frac{q^2}{a^2}\)

Let the tension along AO = T.

∴ At equilibrium, T sin30° = R , and T cos30° = mg

∴ \(\tan 30^{\circ}=\frac{R}{m g}=\frac{\sqrt{3} q^2}{a^2 \cdot m g}\)

or, \(\frac{1}{\sqrt{3}}=\frac{\sqrt{3} q^2}{a^2 m g} \quad \text { or, } \quad q^2=\frac{a^2 m g}{3}\)

or, \(q=a \sqrt{\frac{m g}{3}}=10 \sqrt{3} \cdot \sqrt{\frac{0,1 \times 980}{3}}\)

= 99 esu (approx.)

Example 10. Three-point charges are lying along the x-axis. If two charges q₁ = -2C and q₂ = -3C are placed at x₁ = 2 m and x₂ = -1 m, respectively and the third positive charge is so located between the first two charges that the resultant force on it is zero, find the position of the third charge.
Solution:

In, two charges q₁ and q₂ are placed at points A and B along the x-axis. Suppose, the third charge q is placed in between q₁ and q₂ at C at a distance x m from the origin O so that the resultant force on it is zero.

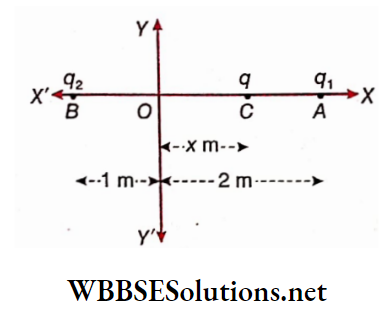
∴ \(\frac{1}{4 \pi \epsilon_0} \cdot \frac{q q_1}{(2-x)^2}=\frac{1}{4 \pi \epsilon_0} \cdot \frac{q q_2}{(1+x)^2}\)

or, \(\frac{-2}{(2-x)^2}=\frac{-3}{(1+x)^2}\)

or, 2(1 +x)² = 3(2-x)²

or, \(\sqrt{2}(1+x)=\sqrt{3}(2-x) \quad\)

or, \((\sqrt{2}+\sqrt{3}) x=2 \sqrt{3}-\sqrt{2}\)

∴\(x=\frac{2 \sqrt{3}-\sqrt{2}}{\sqrt{2}+\sqrt{3}}\)

= \(\frac{(2 \sqrt{3}-\sqrt{2})(\sqrt{3}-\sqrt{2})}{3-2}\)

= \(8+-3 \sqrt{6} \approx 0.65\)

Therefore, the third charge is to be placed at a distance of +0.65 m from the origin O.

Example 11. Two negative point charges each of magnitude 2 esu and another point charge q are lying on a straight line. Each of the charges are in equilibrium. Determine the position, value and the nature of the charge q.
Solution:

Since the two negative charges and the other charge q are in a straight line and in equilibrium, then the charge q must be positive. Also, the charge q should be placed in between the two negative charges such that the resultant force acting on q due to the two negative charges becomes zero.

The two 2 esu charges are placed at points A and B respectively and charge q is placed at point C.

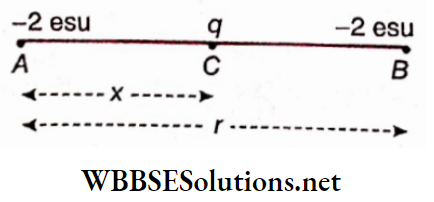
Let AB = r and AC = x

Now, the attractive force acting on +q due to the negative charge at

⇒ \(A=\frac{q \times 2}{x^2}\)

and the attractive force acting on +q due to the negative charge at

⇒ \(B=\frac{q \times 2}{(r-x)^2}\)

Now, from the condition of equilibrium of charge q,

⇒ \(\frac{q \times 2}{x^2}=\frac{q \times 2}{(r-x)^2} \quad \text { or, } x=\frac{r}{2}\)

Again, the repulsive force between the charges at A and B will try to push them apart, but the positive charge at C will attract them and maintain the equilibrium. Then the attractive force acting on any negative charge due to the positive charge q must be equal to the force of repulsion between two negative charges.

∴ \(\frac{q \times 2}{\left(\frac{r}{2}\right)^2}=\frac{2 \times 2}{r^2} \quad\)

or, q = \(\frac{1}{2}\)

= 0.5 esu

Example 12. A shower of protons from outer space deposits equal amounts of charge +q on the Earth and the moon. The electrostaticrepulsionthenexactlycounterbalancesthe counterbalanc the gravitational attraction. Find the amount of charge q.
Solution:

Let the mass of the moon be Mm and that of the earth be Mg and the distance between them be r.

The electrostatic repulsion between the moon and the earth

= \(\frac{q \times q}{r^2}=\frac{q^2}{r^2}\)

Gravitational attraction = \(\frac{G M_e M_m}{r^2}\)

According to the question,

⇒ \(\frac{q^2}{r^2}=\frac{G M_e M_m}{r^2}[/laetx]

or, [latex]q=\sqrt{G M_e M_m}\)

Example 13. The electrostatic force between two point charges and the gravitational attractive force between two point masses are inversely proportional to the square of the distance between the charges and the masses, respectively.

1.

1. Compare the electrostatic force (Fc) and the gravitational force (FG) between an electron and a proton,
2. Compare Fc and FG between two protons.

2. Find out the accelerations of an electron and of a proton due to the electrostatic force between them when they are placed at a distance of 1 A.
Solution:

1.

1. An electron and a proton have the same amount of charge e.

∴ Electrostatic force, \(F_C=\frac{1}{4 \pi \epsilon_0} \cdot \frac{e^2}{r^2}\)

Gravitational force, \(F_G=G \cdot \frac{m_e m_p}{r^2}\)

∴ \(\frac{F_C}{F_G}=\frac{1}{4 \pi \epsilon_0} \frac{e^2}{r^2} \cdot \frac{r^2}{G m_{e^2 m_p}}=\frac{1}{4 \pi \epsilon_0} \cdot \frac{e^2}{G m_{e^{m_p}}}\)

⇒ \(\begin{aligned}
& \frac{\left(9 \times 10^9\right) \times\left(1.6 \times 10^{-19}\right)^2}{\left(6.67 \times 10^{-11}\right) \times\left(9.1 \times 10^{-31}\right)} \\
& \times\left(1.67 \times 10^{-27}\right) \\
&
\end{aligned}\)

= 2.27 x 10³⁹

2. The electrostatic force between two protons,

⇒ \(F_C=\frac{1}{4 \pi \epsilon_0} \cdot \frac{e^2}{r^2}\)

The gravitational force between them,

⇒ \(F_G=\frac{G m_p^2}{r^2}\)

∴ \(\frac{F_C}{F_G}=\frac{1}{4 \pi \epsilon_0} \frac{e^2}{r^2} \cdot \frac{r^2}{G m_p^2}=\frac{1}{4 \pi \epsilon_0} \cdot \frac{e^2}{G m_p^2}\)

⇒ \(\left(9 \times 10^9\right) \times \frac{\left(1.6 \times 10^{-19}\right)^2}{\left(6.67 \times 10^{-11}\right) \times\left(1.67 \times 10^{-27}\right)^2}\)

= 1.24 x 10³⁹

2. The electrostatic force between an electron and a proton,

⇒ \(F_C=\frac{1}{4 \pi \epsilon_0} \cdot \frac{e^2}{r^2}=\left(9 \times 10^9\right) \times \frac{\left(1.6 \times 10^{-19}\right)^2}{\left(10^{-10}\right)^2}\) [∵ r = 1 A = 10^-10m]

= 2.3 X 10^-8 N

∴ Acceleration of the electron,

⇒ \(a_e=\frac{F_C}{m_e}=\frac{2.3 \times 10^{-8}}{9.1 \times 10^{-31}}=2.5 \times 10^{22} \mathrm{~m} \cdot \mathrm{s}^{-2}\)

Acceleration of the proton,

⇒ \(a_p=\frac{F_C}{m_p}=\frac{2.3 \times 10^{-8}}{1.67 \times 10^{-27}}=1.4 \times 10^{19} \mathrm{~m} \cdot \mathrm{s}^{-2}\)

Example 14. Three charges q, q and -q are kept at the three vertices of an equilateral triangle of side l. Find out the resultant force on each of the charges.
Solution:

Due to symmetry, the magnitude of the force between each pair of charges would be the same, given by

⇒ \(F=\frac{1}{4 \pi \epsilon_0} \frac{q^2}{l^2}\)

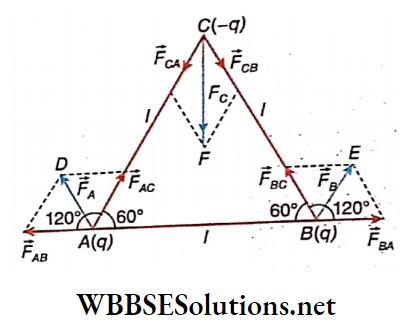
Force acting on charge q at A due to the charges at points. B and C,

⇒ \(F_A=\sqrt{F_{A B}^2+F_{A C}^2+2 F_{A B} \cdot F_{A C} \cos 120^{\circ}}\)

⇒ \(\sqrt{F_{A B}^2+F_{A C}^2-F_{A B} \cdot F_{A C}}=F_{A B}\) [∵ F_AB = F_AC]

⇒ \(\frac{1}{4 \pi \epsilon_0} \cdot \frac{q^2}{l^2} ; \text { along } \overrightarrow{A D}\)

Force acting on charge q at B due to the charges at points A and C,

⇒ \(F_B=\sqrt{F_{B A}^2+F_{B C}^2+2 F_{B A} \cdot F_{B C} \cos 120^{\circ}} .\)

⇒ \(\sqrt{F_{B A}^2+F_{B C}^2-F_{B A} \cdot F_{B C}}\)

= F_BA [∵ F_BA = F_BC]

⇒ \(\frac{1}{4 \pi \epsilon_0} \cdot \frac{q^2}{l^2} \text {; along } \overrightarrow{B E}\)

The force acting on charge -q at C due to the charges at points A and B,

⇒ \(F_C=\sqrt{F_{C A}^2+F_{C B}^2+2 F_{C A} \cdot F_{C B} \cos 60^{\circ}}\)

⇒ \(\sqrt{3} F_{C A}\) [∵ F_CA = F_CB]

⇒ \(\sqrt{3} \cdot \frac{1}{4 \pi \epsilon_0} \cdot \frac{q^2}{l^2} ; \text { along } \overrightarrow{C F}\)

The forces FA, FB and Fc are shown.

Let [latec]\vec{F}[/latex] be the resultant of the two forces \(\vec{F}_A\) and \(\vec{F}_B\)

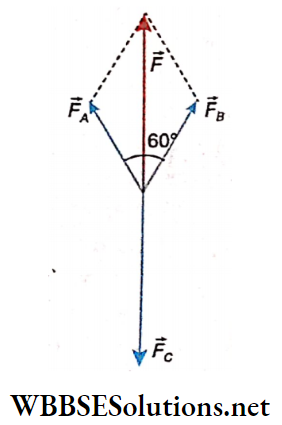
∴ \(F=\sqrt{F_A^2+F_B^2+2 F_A F_B \cos 60^{\circ}}\)

= \(\sqrt{3} F_A=\sqrt{3} \cdot \frac{1}{4 \pi \epsilon_0} \cdot \frac{q^2}{l^2}\)

Therefore, F and Fc have the same magnitude, and they are oppositely directed. So they cancel each other

∴ \(\vec{F}_A+\vec{F}_B+\vec{F}_C=\vec{F}+\vec{F}_C=-\vec{F}_C+\vec{F}_C=0\)

Example 15. Three charges are placed at the vertices of an equilateral triangle of side l. Each of the charges Is q. Find out the force on a charge Q placed at the centre of mass of the triangle.
Solution:

The centre of mass O is equidistant from the vertices A, B, and C of the equilateral triangle

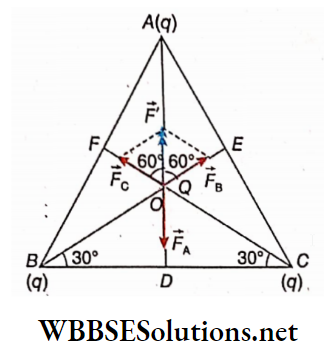
So, each of the charge q will exert equal force F on the charge Q at O, i.e., F_A = F_B = F_C = F

Now, two equal forces F_B and F_C act at O along with OE and OF, respectively. So their resultant Ff will act along OA, which is inclined at an angle of 60° with either OE or OF.

∴ F’ = F_Bcos60° + F_Ccos60° = \(F \cdot \frac{1}{2}+F \cdot \frac{1}{2}=F\)

As the force, F_A of magnitude F acts along OD, i.e., opposite to OA, the resultant of F’ and F_A will be zero.

∴ \(\vec{F}_A+\vec{F}_B+\vec{F}_C=\vec{F}_A+\vec{F}^{\prime}=-\vec{F}+\vec{F}=0\)

So, any charge Q placed at the centre of mass of a triangle will experience no force

Example 16. Two small metal spheres of equal volume are oppositely charged and are kept 0.5 m apart in a vacuum. The attractive force between them Is 0.144 N. But a repulsive force of 0.081 N acts between them after they are momentarily connected with a conducting wire. Find out the initial charges on the two spheres.
Solution:

Let the two initial charges be +q₁ and -q₂.

According to the question, the attractive force between the spheres = 0.144 N.

∴ \(\frac{1}{4 \pi \epsilon_0} \cdot \frac{q_1 q_2}{(0.5)^2}=0.144\)

or, \(q_1 q_2=\frac{0.144 \times 0.25}{9 \times 10^9}=4 \times 10^{-12}\)

The momentary connection brings the spheres to the same potential [See the chapter ‘Electric Potential’]. As the spheres have the same volume, their charges will also be the same. So, the charge on each sphere = \(\frac{1}{2}\left(q_1-q_2\right)\)

According to the question, the repulsive force between the spheres = 0.081 N

∴ \(\frac{1}{4 \pi \epsilon_0} \cdot \frac{\left(q_1-q_2\right)^2}{4 \times(0.5)^2}=0.081\)

or, \(\left(q_1-q_2\right)^2=\frac{0.081 \times 0.25 \times 4}{9 \times 10^9}\)

= \(9 \times 10^{-12}\)

or, q₁-q₂ = 3 X 10^-6 ….(1)

∴ \(q_1+q_2=\sqrt{\left(q_1-q_2\right)^2+4 q_1 q_2}\)

⇒ \(\sqrt{9 \times 10^{-12}+4 \times\left(4 \times 10^{-12}\right)}\)

= 5 X 10^-6 …(2)

From equations (1) and (2),

q₁ = 4 x 10^-6 C and q₂ = 1 x 10^-6 C

So, the initial charges on the spheres are +4 x 10^-6 C and -10^-6 C

Example 17. Two charges of equal magnitude 2 X 10^-8C separated by a silk thread of length lm are placed on an insulated, smooth, horizontal surface. Calculate the tension on the string.
Solution:

Tension on the string = force acting between the two charges

⇒ \(\frac{1}{4 \pi \epsilon_0} \cdot \frac{q_1 q_2}{r^2}\)

⇒\(9 \times 10^9 \times \frac{\left(2 \times 10^{-8}\right)^2}{(1)^2}\) [∵ q₁ = q₂ = 2 X 10^-8C , r = lm]

= 9 X 10⁹ x 4 x 1^-16

= 36 X 10^-7N

Example 18. Three charges -q₁, +q₂ and -q₃ are kept in vacuum. Show that the horizontal component of the resultant force in the charge -q₁ would be proportional to \(\frac{q_2}{b^2}+\frac{q_3}{a^2} \sin \theta\)

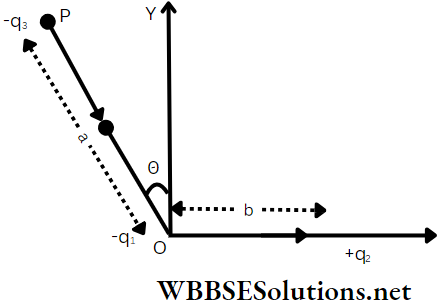
Solution:

The force on -q₁ due to +q₂ is

⇒ \(F_{12}=\frac{1}{4 \pi \epsilon_0} \cdot \frac{q_1 q_2}{b^2} ; \text { along } \overrightarrow{O X}\)

The force on -q₁ due to -q₃ is

⇒ \(F_{13}=\frac{1}{4 \pi \epsilon_0} \cdot \frac{q_1 q_3}{a^2} ; \text { along } \overrightarrow{P O}\)

The component of F13 along \(\overrightarrow{O X}\)

⇒ \(\left(F_{13}\right)_X=\frac{1}{4 \pi \epsilon_0} \cdot \frac{q_1 q_3}{a^2} \sin \theta\)

∴ The horizontal component of the resultant force on -q₁,

⇒ \(F_X=\frac{1}{4 \pi \epsilon_0} \cdot \frac{q_1 q_2}{b^2}+\frac{1}{4 \pi \epsilon_0} \cdot \frac{q_1 q_3}{a^2} \sin \theta\)

⇒ \(\frac{q_1}{4 \pi \epsilon_0}\left(\frac{q_2}{b^2}+\frac{q_3}{a^2} \sin \theta\right)\)

∴ \(F_X \propto\left(\frac{q_2}{b^2}+\frac{q_3}{a^2} \sin \theta\right)\)

Example 19. Four charges, each of -Q, are placed at the four corners of a square. Find out the value of a charge q placed at the centre of the square, such that all the charges would be at equilibrium.
Solution:

Let each side of the square = a

∴ \(A C=\sqrt{a^2+a^2}=\sqrt{2} a \text { and } A O=\frac{A C}{2}=\frac{\sqrt{2} a}{2}=\frac{a}{\sqrt{2}}\)

Force on the charge -Q at A due to the charge -Q at D,

⇒ \(F=\frac{1}{4 \pi \epsilon_0} \cdot \frac{Q^2}{a^2} ; \text { along } \overrightarrow{B A}\)

Similarly, the force on -Q at A due to -Q at D will also be F, but along \(\overrightarrow{D A}\)

The resultant of these two forces will be,

⇒ \(F_1=\sqrt{F^2+F^2}=\sqrt{2} F=\frac{1}{4 \pi \epsilon_0} \cdot \frac{\sqrt{2} Q^2}{a^2} ; \text { along } \overrightarrow{C A}\)

Again, force on -Q at A due to the charge -Q at C,

⇒ \(F_2=\frac{1}{4 \pi \epsilon_0} \cdot \frac{Q^2}{(\sqrt{2} a)^2}=\frac{1}{4 \pi \epsilon_0} \cdot \frac{Q^2}{2 a^2} ; \text { along } \overrightarrow{C A}\)

Now, force on -Q at A due to the charge q at O,

⇒ \(F_3=-\frac{1}{4 \pi \epsilon_0} \cdot \frac{q Q}{\left(\frac{a}{\sqrt{2}}\right)^2}=-\frac{1}{4 \pi \epsilon_0} \cdot \frac{2 q Q}{a^2} ; \text { along } \overrightarrow{C A}\)

At equilibrium, the resultant of these three forces along \(\) must be zero, i.e.,

⇒ \(F_1+F_2+F_3=0 \quad \text { or, } \frac{1}{4 \pi \epsilon_0}\left[\frac{\sqrt{2} Q^2}{a^2}+\frac{Q^2}{2 a^2}-\frac{2 q Q}{a^2}\right]=0\)

or, \(\frac{1}{4 \pi \epsilon_0} \cdot \frac{Q}{a^2}\left[Q\left(\sqrt{2}+\frac{1}{2}\right)-2 q\right]=0\)

or, \(Q\left(\sqrt{2}+\frac{1}{2}\right)-2 q=0\)

or, \(2 q=Q \frac{2 \sqrt{2}+1}{2} \quad\)

or, \(q=\frac{Q}{4}(2 \sqrt{2}+1)\)

Example 20. Two identically charged spheres are suspended by strings of equal length. The strings make an angle of 30° with each other. When suspended in a liquid of density 0.8 g.cm^-3, the angle remains the same. What is the dielectric constant of the liquid? The density of the material of the sphere is 1.6 g.cm^-3.
Solution:

Let A and B be the equilibrium positions of the two spheres in both cases. Suppose, AB = r, the charge of each sphere = q, the mass of each sphere = m.

When the system is in air, the tension in the string = Tx and repulsive force between the spheres,

⇒ \(F_1=\frac{1}{4 \pi \epsilon_0} \frac{q^2}{r^2}\)

At equilibrium,

F₁ = T₁sin15° …(1)

and mg = T₁ cos15°

From equations (1) and (2),

⇒ \(\frac{F_1}{m g}=\frac{T_1 \sin 15^{\circ}}{T_1 \cos 15^{\circ}}=\tan 15^{\circ}\)

or, F₁ = mgtan15°

⇒ \(\frac{1}{4 \pi \epsilon_0} \frac{q^2}{r^2}=m g \tan 15^{\circ}\)

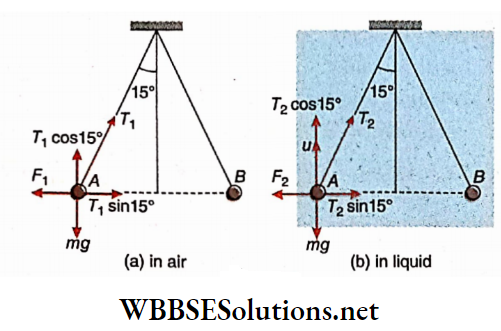
When the spheres are suspended in the liquid, tension in the string = T2, upthrust by the liquid on each sphere = u and repulsive force between the two spheres, \(F_2=\frac{1}{4 \pi \epsilon_0 \kappa} \frac{q^2}{r^2}\) [where k = dielectric constant of the medium]

Now at equilibrium,

F₂ = T₂sin15° …..(4)

and mg- u = T₂cos15° ….(5)

From equations (4) and (5),

⇒ \(\frac{F_2}{m g-u}=\tan 15^{\circ}\)

or, F₂ = (mg – u) tan15°

or, \(\frac{1}{4 \pi \epsilon_0 \kappa} \frac{q^2}{r^2}=(m g-u) \tan 15^{\circ}\)….(6)

From equations (3) and (6), we get

⇒ \(\kappa=\frac{m g}{m g-u}\)…..(7)

Let the volume of the sphere = V, the density of the material of the sphere = d and the density of the liquid = d_l

∴ m = Vd and u – Vd_l g

Hence from equation (7), we get \(\kappa=\frac{V d g}{V d g-V d_l g}=\frac{d}{d-d_l}\)

or, \(\kappa=\frac{1.6}{1.6-0.8}\) [∵ d = 1.6 g/cm³ and d₁ – 0.8 g/cm³]

or, k = 2

Hence, the required dielectric constant is 2.

Example 21. An infinite number of charges, each equal to 4μC are placed along the x-axis at x = 1m, 2m, 4m, 8m and so on. Find the total force on a charge of 1 C placed at the origin.
Solution:

Here, a charge of 1 C is placed at x = 0

Let, the total force on the charge at x = 0 due to an infinite number of charges of 4μC placed at distances x = lm, x = 2m, x = 4m, x = 8 m … up to x = ∞ be F.

∴ \(\begin{array}{r}
F=\frac{1}{4 \pi \epsilon_0}\left[\frac{1 \times 4 \times 10^{-6}}{1^2}+\frac{1 \times 4 \times 10^{-6}}{2^2}+\frac{1 \times 4 \times 10^{-6}}{4^2}\right. \\
\left.+\frac{1 \times 4 \times 10^{-6}}{8^2}+\cdots+\infty\right]
\end{array}\)

⇒ \(=9 \times 10^9 \times 4 \times 10^{-6}\left[\frac{1}{1^2}+\frac{1}{2^2}+\frac{1}{4^2}+\frac{1}{8^2}+\cdots+\infty\right]\)

⇒ \(36 \times 10^3\left[\frac{1}{1-\frac{1}{4}}\right]=36 \times 10^3 \times \frac{4}{3}\)

= 48 x 10³N

Hence, the total force acting on the charge at x = 0 is 48 x 10³ N.

Example 22. A small ball of mass 2 x 10^-3 kg having a charge of 1μC Is suspended by a string of length 0.8 m. Another identical ball having the same charge is kept at the point of suspension. Determine the minimum horizontal velocity which should be imparted to the lower ball, so that it can make a complete revolution.
Solution:

Let the charge of each ball – q, the radius of the vertical circular path = l and the velocity of the rotating ball at the highest and lowest point of that circular path be v and u respectively.

At the highest point A, three forces act simultaneously which are

1. Upward electrostatic force of repulsion, \(F_e=\frac{q^2}{l^2}\)[in CGS system],

2. Weight of the rotating ball, W = mg (downwards),

3. Tension in the string = T (downwards).

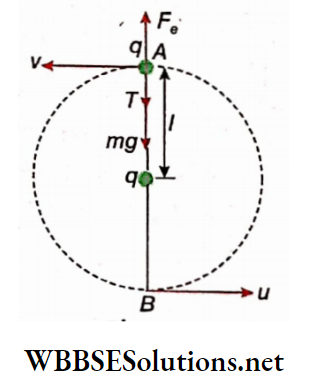
To move in a vertical circular path, the ball should have a minimum velocity at A. In that case, T = 0 and the necessary centripetal force is provided by (W – F_e).

∴ \(W-F_e=\frac{m v^2}{l}\)

∴ \(v^2=\frac{l}{m}\left[m g-\frac{q^2}{l^2}\right]\)

Applying the law of conservation of total mechanical energy at A and B we can write,

⇒ \(\frac{1}{2} m v^2+m g \times 2 l=\frac{1}{2} m u^2\)

or, \(u^2=v^2+4 g l=\frac{l}{m}\left[m g-\frac{q^2}{l^2}\right]+4 g l=5 g l-\frac{q^2}{m l}\)

∴ \(u=\sqrt{5 g l-\frac{q^2}{m l}}\)

Hence, the minimum horizontal velocity at the lowest point is

⇒ \(\sqrt{5 g l-\frac{q^2}{m l}}\)

Example 23. A rigid insulated wireframe in the die form of a right-angled triangle ABC is set in a vertical plane as shown in the figure. Two beads, each of mass m and carrying charges q₁ and q₂ are connected by a cord of length l and kept between the arms AB and AC of the triangle In such a way that they can slide without friction along the arms. When the beads arc stationary, determine

1. The angle a,
2. The tension in the cord and
3. The normal reaction on the beads.

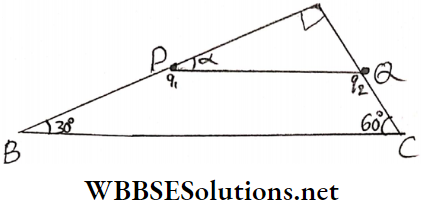
If the cord is now cut what are the values of charge for which the beads continue to remain stationary?
Solution:

Let us consider the forces acting on bead P. The forces are O weight mg acting vertically downward, Q tension T in the cord, G electric force between P and Q given by, \(F=\frac{1}{4 \pi \epsilon_0} \cdot \frac{q_1 q_2}{l^2}\), normal reaction \(N_{R_1}\)

When the bead P is at equilibrium,

mgcos60° = (7’- F)cosα…..(1)

and \(N_{R_1}=m g \cos 30^{\circ}+(T-F) \sin \alpha\)…(2)

For die bead Q, we have

mgsin60° = (T-F)sinα….(3)

and \(N_{R_2}=m g \cos 60^{\circ}+(T-F) \cos \alpha\)….(4)

1. Dividing (3) by (1), tanα = tan60°

∴ a = 60°

2. Again putting a = 60° in equation (3),

mgsin60° = (T-F)sin60°

or, \(T=F+m g=\frac{1}{4 \pi \epsilon_0} \cdot \frac{q_1 q_2}{l^2}+m g\)……(5)

3. From equation (4), T-F = mg

∴ \(N_{R_2}=m g \cos 60^{\circ}+m g \cos 60^{\circ}=m g\)

From equation (2),

⇒ \(N_{R_1}=m g \cos 30^{\circ}+m g \sin 60^{\circ}=\sqrt{3} m g\)

When the cord is cut, T=0

From equation (5) we get,

⇒ \(m g=-\frac{1}{4 \pi \epsilon_0} \cdot \frac{q_1 q_2}{l^2}\)

The right-hand side of the equation should be positive which is possible only if q₁ and q₂ have opposite signs. Thus for equilibrium, die beads must have unlike charges. The magnitude of the product of the charges is \(\left|q_1 q_2\right|=4 \pi \epsilon_0 m g l^2\)

Example 24. A particle A having a charge of 5.0 X 10~7 C Is fixed In a vertical wall. A second particle B of mass 100 g and having equal charge Is suspended by a silk thread of length 30 cm from the wall. The point of suspension Is located 30 cm vertically above the first particle. Find the angle of the thread with the vertical when It stays In equilibrium.
Solution:

The situation lets the point of suspension be O, where the tire thread makes an angle of θ with the vertical. Forces on the particle B are

1. Weight mg acting downward,
2. Tension T along the thread,
3. The electric force of repulsion F along AB.

At equilibrium, the sum of all these forces becomes zero.

From the given, \(O A=O B, \angle O B A=\angle O A B=\left(90^{\circ}-\frac{\theta}{2}\right)\)

Considering the components along BX, we get

⇒ \(F \cos \frac{\theta}{2}=m g \cos \left(90^{\circ}-\theta\right)=m g \sin \theta=2 m g \sin \frac{\theta}{2} \cos \frac{\theta}{2}\)

or, \(\sin \frac{\theta}{2}=\frac{F}{2 m g}\)

Now, \(F=\frac{1}{4 \pi \epsilon_0} \cdot \frac{q_1 q_2}{A B^2} \text { and } A B=2(O A) \sin \frac{\theta}{2}\)

∴ \(\sin \frac{\theta}{2}=\frac{1}{4 \pi \epsilon_0} \cdot \frac{q_1 q_2}{4(O A)^2 \sin ^2 \frac{\theta}{2}} \cdot \frac{1}{2 m g}\)

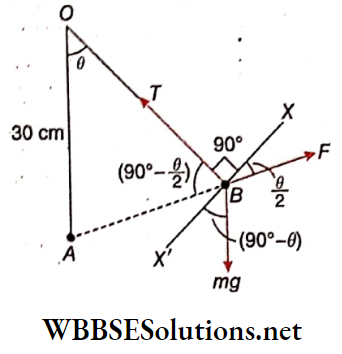
or, \(\sin ^3 \frac{\theta}{2}=\frac{1}{4 \pi \epsilon_0} \cdot \frac{q_1 q_2}{4(O A)^2 \sin ^2 \frac{\theta}{2}} \cdot \frac{1}{2 m g}\)

⇒ \(\begin{aligned}
& \left(9 \times 10^9\right) \times \frac{\left(5 \times 10^{-7}\right)^2}{4 \times\left(30 \times 10^{-2}\right)^2} \\
& \quad \times \frac{1}{2 \times\left(100 \times 10^{-3}\right) \times 9.8}
\end{aligned}\)

= 0.0032

or, \(\sin \frac{\theta}{2}=0.15\)

∴ 0 = 17°

Electrostatics

Electric Field Electric Field Intensity Of An Electric Field At A Point

Electric field: The space around any electric charge in which its influence can be felt, so that any other charge in that region is acted upon by a force, is known as the electric field of that charge.

Theoretically, the field extends up to infinity, but the limited sensitivity of detecting instruments predicts that charge exerts its influence (force of attraction or repulsion) only over a limited region.

Intensity of an electric field: The intensity or strength of an electric field at any point in the field is the force experienced by a unit positive charge placed at that point.

Obviously, the intensity of an electric field is a vector quantity. The direction of intensity is given by the direction of force acting on the positive charge. An electric field may be generated due to more than one charge.

Intensity at a point in such an electric field is determined by calculating the field at that point due to all the charges individually and then the resultant of all the field strengths are found out by vector addition.

The intensity or strength of the electric field at a point is generally denoted by the letter \(\vec{E}\). Often it is simply called an ‘electric field \(\vec{E}\)

Suppose, a test charge q₀ experiences a force of F when it is placed at a point in an electric field. Then, the electric field at that point is \(\vec{E}=\frac{\vec{F}}{q_0}\)

Here, the test charge q₀ should be very small so that it cannot alter the original electric field. So the correct definition of \(\vec{E}\) should be,

⇒ \(\vec{E}=\lim _{q_0 \rightarrow 0} \frac{\vec{F}}{q_0}\)

1. There is no problem as such in using the definition of \(\vec{E}\), except that we cannot make q₀ arbitrarily small, because in the real world, we have never observed a charge smaller than the electronic charge.

2. No problem arises however, if we take the definition of \(\vec{E}\) of a charge distribution at any point (x, y, z) , where the position vector is \(\vec{r}(=\hat{i} x+\hat{j} y+\hat{k} z)\) as

⇒ \(\vec{E}=\frac{1}{4 \pi \epsilon_0} \sum_{j=1}^n \frac{q_j \hat{r}_j}{r_j^2}\)

where \(\hat{r}_j\) is the unit vector from the jth charge to the point (\(\vec{r}\)) (x, y, z).

Unit: The unit of electric field intensity in the CGS system is dyn/esu of charge or dyn/statcoulomb and in SI the unit is newton/coulomb (N.C^-1).

An alternative unit of electric intensity in SI :

⇒ \(\frac{\mathrm{N}}{\mathrm{C}}=\frac{\mathrm{N} \times \mathrm{m}}{\mathrm{C} \times \mathrm{m}}=\frac{\mathrm{J}}{\mathrm{C} \times \mathrm{m}}=\frac{\mathrm{V}}{\mathrm{m}}=\mathrm{V} \cdot \mathrm{m}^{-1}\)

Dimension: \([E]=\frac{[F]}{[q]}=\frac{\mathrm{MLT}^{-2}}{\mathrm{IT}}=\mathrm{MLT}^{-3} \mathrm{I}^{-1}\)

Relation between CGS and SI units of intensity:

⇒ \(1 \mathrm{~N} \cdot \mathrm{C}^{-1}=\frac{10^5 \mathrm{dyn}}{3 \times 10^9 \text { esu of charge }}=\frac{1}{3 \times 10^4} \mathrm{dyn} \cdot \mathrm{statC}^{-1}\)

So, 1 esu electric intensity = 3 x 10⁴ N.C^-1

Electric field due to a point charge:

Let a point charge q be placed at a point in a medium of permittivity k. This charge will create an electric field around it.

To determine the electric field at a point at a distance r from the charge, a unit positive charge is imagined to be placed at that point.

The force experienced by the unit positive charge is the magnitude of the electric field at that point.

If charge q is placed at a point in a medium of permittivity E, the electric field at a distance r from the charge is given by,

⇒ \(E=\frac{1}{4 \pi \epsilon} \cdot \frac{q}{r^2}=\frac{1}{4 \pi \kappa \epsilon_0} \cdot \frac{q}{r^2}\)…..(1)

[K = dielectric constant of the medium]

For vacuum or air, K = 1

∴ \(E=\frac{1}{4 \pi \epsilon_0} \cdot \frac{q}{r^2}\) ….(2)

Therefore, an electric field due to a point charge is directly proportional; to the charge (q) and inversely proportional to the square of the distance (r) from it.

It is obvious that the electric field is different at different points of a field. Since we have assumed for definition that the test charge is positive, the electric field due to this will be directed away from the charge.

In case the source charge is negative, the field will be directed towards the charge

If E be the electric field at a point, the force acting on a charge q placed at that point, by definition is given by, F = qE i.e., force = charge x field intensity

The force acting on a charge in an electric field does not depend ‘ on its mass.

In the CGS system, for air or vacuum,

⇒ \(F=\frac{q}{r^2}\)

It should be noted that if the magnitude and direction of the field are the same at all points in the field, it is called a uniform electric field.

Electrostatics Electric Field Numerical Examples

Example 1. A negative charge of 20 units is placed at a distance 50 cm away from a positive charge of 80 units. Where will the electric field be zero on the line joining the two charges?
Solution:

Suppose, +80 unit and -20 unit of charges are placed at A and B respectively. The point where the electric field will be zero cannot lie in between A and B, because in that case intensity could be along the same direction, i.e., along \(\vec{AB}\) for both the charges.

As the charge at A is greater, the point where the resultant inequality is zero will be situated on the right side of B, say at P.

Suppose, BP = x

The electric field at P due to the charge at \(B=\frac{20}{x^2} \text {; along } \overrightarrow{P B}\)

The electric field at P due to the charge at \(A=\frac{80}{(50+x)^2}; \text { along } \overrightarrow{A P}\)

Since, the resultant intensity at P = 0

∴ \(\frac{20}{x^2}=\frac{80}{(50+x)^2} \quad \text { or, } x=50,-\frac{50}{3}\)

Now, \(x \neq-\frac{50}{3} \mathrm{~cm}\), because the point in that case would be situated in between A and B.

So, x = 50 cm; the point where the field Is zero at a distance of 50 cm from the -20 unit charge on its right side.

Example 2. At each of the four vertices of a square of side 10 cm, there is a + 20 esu of charge. Find the intensity of the electric field at the point of intersection of the two diagonals.
Solution:

The point of intersection O of the diagonals is equidistant from four equal charges. So the electric field at O due to each charge is the same in magnitude (=E, say).

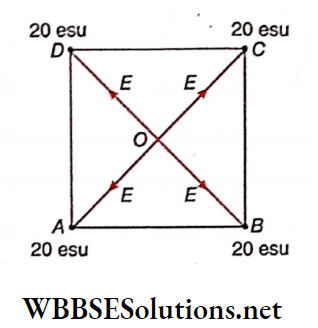
Now, the fields E along \(\overrightarrow{O C}\) and E along \(\overrightarrow{O A}\) cancel each other.

Similarly, E along \(\overrightarrow{O D}\) and E along \(\overrightarrow{O B}\) also canceL

Therefore, the intensity of the electric field at the point of intersection of the two diagonals of the square is zero.

Example 3. AB and CD are two perpendicular diameters of a circle of circumference 20πcm. There are +10 esu, +10esu, -10esu and -10esu of charges at A, B, C and D respectively. What is the intensity of the electric field at the centre O of the circle? What is the direction of the field?
Solution:

Suppose, O is the centre of the circle

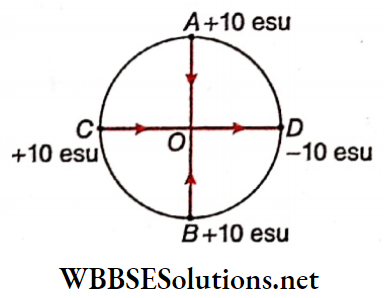
The radius of the circle = r

Here, 2πr = 20π

or, r = 10 cm

Intensities of the electric field at O due to the charges at A and B cancel each other because the two fields are equal and opposite.

Intensity at O due to the charge at C

⇒ \(=\frac{10}{(10)^2}=\frac{1}{10} \text { dyn } \cdot \operatorname{statC}^{-1} \text {; along } \overrightarrow{O D}\)

Intensity at O due to the charge at D

⇒ \(\frac{10}{(10)^2}=\frac{1}{10} \mathrm{dyn} \cdot \operatorname{statC}^{-1} \text {; along } \overrightarrow{O D}\)

∴ Resultant intensity at O

⇒ \(\left(\frac{1}{10}+\frac{1}{10}\right)=0.2 \mathrm{dyn} \cdot \operatorname{statC}^{-1} \text {; along } \overrightarrow{O D}\)

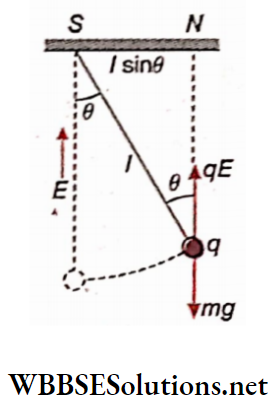
Example 4. The bob of a pendulum of weight 80 mg carries a charge of 2 x 10^-8 C. The bob is at rest in a horizontal electric field of magnitude 2 x 10⁴ V.m^-1. Determine the tension in the string and the angle of the string with the vertical. Given, g = 9.8 m.s^-2
Solution:

Suppose, the string of the pendulum is inclined at an angle of θ with the vertical

Let the horizontal electric field = E; tension in the string = T; charge on the bob = q. The forces acting on the bob.

At equilibrium,

Tsinθ = Eq; Tcosθ = mg

∴ \(\tan \theta=\frac{E q}{m g}\)

= \(\frac{2 \times 10^4 \times 2 \times 10^{-8}}{80 \times 10^{-6} \times 9.8}\)

= \(\frac{25}{49}\)

or, \(\theta=\tan ^{-1}\left(\frac{25}{49}\right) \approx 27^{\circ}\)

Now, cosθ = cos27° = 0.89

∴ \(T=\frac{m g}{\cos \theta}=\frac{80 \times 10^{-6} \times 9.8}{0.89}\)

= 8.8 x 10^-4 N

Example 5. A circular copper ring of radius r, placed in a vacuum, has a charge q on it. Find out the electric fields

1. At the center of the ring, and
2. On the axis of the ring at a distance x from its center,
3. For what value of x would the electric field be maximum?

Solution:

The charge q would be uniformly distributed along the circumference of the circular ring.

So, the linear density of charge along the ring,

⇒ \(\lambda=\frac{q}{2 \pi r}\)

1. The electric field at the centre of the ring due to the charge on the small element of the ring would be cancelled due to the charge on the diametrically opposite small element.

The whole ring is effectively an assembly of a large number. of such diametrically opposite pairs of elements. As a result, the resultant electric field at the die centre would be zero.

2. Let us take a small element of length dl on the ring at the position A. Charge on the element \(d l=\lambda d l=\frac{q}{2 \pi r} d l\). The electric field at the axial point P due to the charge on the element dl,

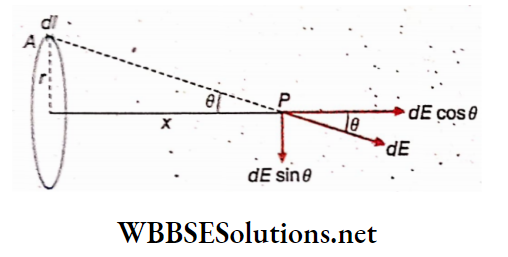
⇒ \(d E=\frac{1}{4 \pi \epsilon_0} \cdot \frac{\dot{\lambda} d l}{(A P)^2} ; \text { along } \overrightarrow{A P}\)

Its axial component is

⇒ \(d E \cos \theta=\frac{1}{4 \pi \epsilon_0} \cdot \frac{\lambda d l}{(A P)^2} \frac{x}{(A P)}=\frac{1}{4 \pi \epsilon_0} \cdot \frac{\lambda x d l}{(A P)^3}\)

The component of dE perpendicular to the axis = dEsind.

For charges distributed throughout the entire ring, \(\sum d E \sin \theta=0\), due to symmetry.

Therefore, the resultant axial electric field at the point P,

⇒ \(E=\sum d E \cos \theta=\sum \frac{1}{4 \pi \epsilon_0} \cdot \frac{\lambda x d l}{(A P)^3}\)

⇒ \(\frac{1}{4 \pi \epsilon_0} \cdot \frac{q}{2 \pi r} \cdot \frac{x}{\left(r^2+x^2\right)^{3 / 2}} \cdot \sum d l\) [∵ \(\lambda=\frac{q}{2 \pi r} \text { and } A P=\left(r^2+x^2\right)^{1 / 2}\)]

⇒ \(\frac{1}{4 \pi \epsilon_0} \cdot \frac{q}{2 \pi r} \cdot \frac{x}{\left(r^2+x^2\right)^{3 / 2}} \cdot 2 \pi r\)

⇒ \(\frac{1}{4 \pi \epsilon_0} \cdot \frac{q x}{\left(r^2+x^2\right)^{3 / 2}}\)

2. For E to be maximum, \(\frac{dE}{dx}\) = 0

Now, \(\frac{d E}{d x}=\frac{q}{4 \pi \epsilon_0} \cdot \frac{d}{d x}\left[x\left(r^2+x^2\right)^{-3 / 2}\right]\)

⇒ \(\begin{aligned}
& \frac{q}{4 \pi \epsilon_0}\left[1 \cdot\left(r^2+x^2\right)^{-3 / 2}\right. \\
& \left.\quad+x \cdot\left(-\frac{3}{2}\right) \cdot\left(r^2+x^2\right)^{-5 / 2} \cdot 2 x\right]
\end{aligned}\)

⇒ \(\frac{q}{4 \pi \epsilon_0}\left(r^2+x^2\right)^{-5 / 2}\left[\left(r^2+x^2\right)-3 x^2\right]\)

⇒ \(\frac{q}{4 \pi \epsilon_0}\left(r^2+x^2\right)^{-5 / 2}\left(r^2-2 x^2\right)\)

For maximum E,

⇒ \(\frac{d E}{d x}=0 \text { or, } r^2-2 x^2=0 \text { or, } x= \pm \frac{r}{\sqrt{2}}\)

The ‘±’ sign implies that the electric field on the die axis will be maximum at a distance \(\frac{r}{\sqrt{2}}\) on either side of the ring.

Example 6. Starting from rest, an electron of mass me and a proton of mass mp travel through a certain distance in a uniform electric field in times t₁ and t₂, respectively. Find out the ratio t₂/t₁, neglecting the influence of gravity.
Solution:

In a uniform electric field of intensity E, the force acting on a charge q is F = qE and its acceleration, a = \(\frac{qE}{m}\).

The distance travelled from rest in time t,

⇒ \(s:=\frac{1}{2} a t^2=\frac{1}{2}\left(\frac{q E}{m}\right) t^2\)

For electron, \(s_e=\frac{1}{2}\left(\frac{q E}{m_e}\right) t_1^2 ; \text { for proton, } s_p=\frac{1}{2}\left(\frac{q E}{m_p}\right) t_2^2\)

Given s_e = s_p; so we have,

⇒ \(\frac{1}{2}\left(\frac{q E}{m_e}\right) t_1^2=\frac{1}{2}\left(\frac{q E}{m_p}\right) t_2^2 \quad \text { or, } \frac{1}{m_e} t_1^2=\frac{1}{m_p} t_2^2\)

or, \(\frac{t_2^2}{t_1^2}=\frac{m_p}{m_e} \text { or; } \frac{t_2}{t_1}=\sqrt{\frac{m_p}{m_e}}\)

Example 7. Calculate the radius of a charged water drop which remains just suspended in equilibrium in the earth’s electric field. The charge in the water drop is equal to that of an electron. The magnitude of the earth’s electric field is 10^-2statC.cm^-1. [e = 4.805 x 10^-10 esu of charge; g = 980 cm.s^-2]
Solution:

Let the radius of the water drop be r cm and mass mg.

Here the intensity of the electric field,

E = 10^-2 statC.cm^-1

In equilibrium, electrical force on the charged water drop = weight of the water drop.

or, eE = mg

or, \(e E=\frac{4}{3} \pi r^3 \rho g \text { [denstiy of water, } \rho=1 \mathrm{~g} \cdot \mathrm{cm}^{-3} \text { ] }\)

or, \(r^3=\frac{3 e E}{4 \pi g}\)

= \(\frac{3 \times 4.805 \times 10^{-10} \times 10^{-2}}{4 \times \pi \times 980}\)

= 1.171 x 10^-15

∴ r = 1.054 x 10^-5 cm

Example 8. A simple pendulum consists of a small sphere of mass suspended by a thread of length l. The sphere carries a charge q. The pendulum Is placed in a uniform electric field of strength E directed vertically upwards. With what period will the pendulum oscillate if the electrostatic force acting on the sphere is less than the gravitational force?
Solution:

Let the pendulum be at an angle of θ with the vertical Net force acting on the bob = mg-qE Torque experienced by the bob about the point of suspension,

⇒ \(\tau\) = -(mg-qE)lsinθ (where the negative sign indicates that the E torque experienced is restoring torque)

As the amplitude of oscillation is small,

sinθ ≈ θ

Then, \(\tau=-(m g-q E) l \theta\)

Again \(\tau=\text { moment of inertia }(I) \times \text { angular acceleration }(\alpha)\)

∴ \(\alpha=\frac{\tau}{I}=\frac{-(m g-q E) l \theta}{I}=\frac{-(m g-q E) l \theta}{m l^2}\) [∵ I = ml²]

⇒ \(\frac{-\left(g-\frac{q E}{m}\right) \theta}{l}\)

∴ \(\alpha \propto-\theta\) [∵ \frac{\left(g-\frac{q E}{m}\right)}{l}=\text { constant }\right][/latex]

∴ The pendulum executes an SHM.

∴ Period of the pendulum

⇒ \(T=2 \pi \sqrt{\frac{\text { angular displacement }}{\text { angular acceleration }}}\)

= \(2 \pi \sqrt{\frac{\theta}{\alpha}}\)

∴ \(T=2 \pi \sqrt{\frac{l}{g-\frac{q E}{m}}}\)

Example 9. Find the electric field intensity at the centre of a semicircular arc of radius r, uniformly charged with a charge q.
Solution:

The length of the semicircular wire is πr.

Linear charge density, \(\lambda=\frac{q}{\pi r}\)

Let the charge of a small part dl of the wire be dq.

∴ \(d q=\lambda \cdot d l=\frac{q}{\pi r} \cdot d l\)

Field intensity at the centre O due to the small part,

⇒ \(d E=\frac{1}{4 \pi \epsilon_0} \cdot \frac{d q}{r^2}=\frac{1}{4 \pi \epsilon_0} \cdot \frac{q d l}{\pi r \cdot r^2}\)

⇒ \(\frac{1}{4 \pi \epsilon_0} \cdot \frac{q d \theta}{\pi r^2}\) [∵ dl = rdθ]

Now the field intensity dE is resolved into two components, one along the radius which is dEx = dEcosd and another particular dicular to the radius which is dEy = dEsind. Considering the whole wire, it is seen that all the dEx components get cancelled. Only the dEy components get to remain.

∴ Total field intensity at O,

⇒ \(E=\int_0^\pi d E \sin \theta=\frac{1}{4 \pi \epsilon_0} \cdot \frac{q}{\pi r^2} \int_0^\pi \sin \theta \cdot d \theta\)

⇒ \(\frac{1}{4 \pi \epsilon_0} \cdot \frac{q}{\pi r^2}(1-\cos \pi)\)

= \(\frac{1}{4 \pi \epsilon_0} \cdot \frac{2 q}{\pi r^2}\)

Example 10. An electron of charge 1.6 x 10^-19C and 9.1 X 10^-31 kg, travelling along the X-axis with a uniform velocity of 10⁶ m s^-1, enters in a uniform elecCrisfield of 10³ V.m^-1 acting perpendicular to the X-axis. If the electric field extends over a length of 2 cm along the X-axis, what will be the deflection of the electron along the direction of the field when it emerges from it?
Solution:

Here, the electric field E = 10³ V/m is directed along the Yaxis and effective up to a length L = 2 cm along the X-axis.

An electron is projected from O with an initial speed of v_x = 10⁶ m/s along + X-axis. Charge of the electron, e = 1.6 x 10^-19 C and mass of the electron, m = 9.1 x 10^-31 kg.

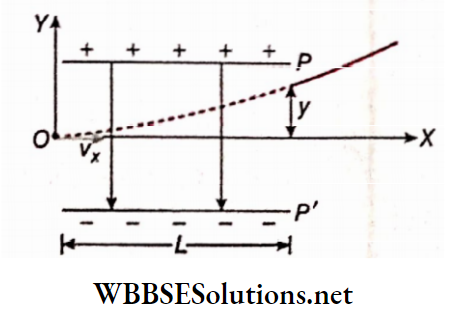
If we consider the motion of the electron along the Y-axis, the force on the electron due to the electric field, F_y = eE.

∴ Acceleration of the electron along 7-axis, ay = \(\frac{eE}{m}\)

Let the deflection of the electron when it is emitted from the electric field be y and the time taken to cover that vertical distance be t.

∴ \(y=\frac{1}{2} \cdot a_y t^2\)

or, \(y=\frac{1}{2} \cdot \frac{e E}{m} t^2\)….(1)

Along the X-axis, force on the electron, Fx = 0

∴ Acceleration, a_x = 0

∴ Velocity, v_x = 10⁶ m/s (constant)

Now distance covered along the X-axis in time t is L = v_xt

∴ \(t=\frac{L}{v_x}\)

From equation (1) and (2),

⇒ \(y=\frac{1}{2} \cdot \frac{e E}{m} \cdot \frac{L^2}{v_x^2}\)

⇒ \(\frac{1}{2} \times \frac{1.6 \times 10^{-19} \times 10^3 \times(.02)^2}{9.1 \times 10^{-31} \times\left(10^6\right)^2}\)

= 0.0351 m

= 3.51 cm

Thus, the required deflection is 3.51 cm.

Example 11. A charged cork ball of mass m is suspended on a light Y string in the adjacent figure. T When \(\vec{E}=\left(E_x \hat{i}+E_y \hat{j}\right) \hat{N} / \mathrm{C}\) the ball is in equilibrium at the angle θ. Find the charge of the ball and the tension in the string.

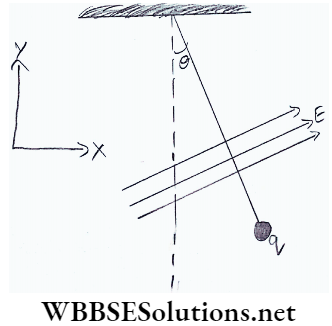
Solution:

In equilibrium, the net force acting on the ball = 0.

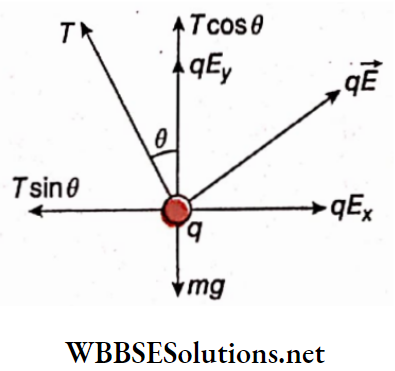
⇒ \(T \cos \theta \hat{j}-T \sin \theta \hat{i}+q E_x \hat{i}+q E_y \hat{j}-m g \hat{j}=0\)

∴ Tsinθ = qE_x….(1)

and Tcosθ = mg – qE_y….(2)

From equations (1) and (2) we get,

⇒ \(\tan \theta=\frac{q E_x}{m g-q E_y}\)….(3)

or, \(m g \tan \theta-q E_y \tan \theta=q E_x\)

∴ \(q=\frac{m g \tan \theta}{E_x+E_y \tan \theta}\)

Putting the value of q in equation (1) we get,

⇒ \(T \sin \theta=\frac{m g \tan \theta}{E_x+E_y \tan \theta} E_x\)

∴ \(T=\frac{m g E_x \sec \hat{\theta}}{E_x+E_y \tan \theta}\)

Example 12. Two point charges of 10 esu and 40 esu are located at points A and B separated by a distance of 4 cm. Find the electric field intensity at a point halfway between the charges.
Solution:

Since the charges are 4 cm apart, each of them is 2 cm away from the midpoint (O) joining them Electric field intensity at O due to the charge 10 esu,

⇒ \(E_1=\frac{10}{(2)^2}=2.5 \mathrm{dyn} / \mathrm{esu} ; \text { along } \overrightarrow{O B}\)

Electric field intensity at O due to the charge 40 esu,

⇒ \(E_2=\frac{40}{(2)^2}=10 \mathrm{dyn} / \mathrm{esu} ; \text { along } \overrightarrow{O A}\)

Since \(\overrightarrow{E_1} \text { and } \overrightarrow{E_2}\) are oppositely directed, the resultant field intensity at O,

E = E₂-E₁

= 10- 2.5

= 7.5 dyn/esu; along \(\vec{OA}\)

Example 13. Two negative charges each of 1 unit and a positive charge q are placed along a straight line. Find the magnitude and position of q for which all the charges will remain at equilibrium. Is the equilibrium stable, unstable or neutral in this case?
Solution:

The positive charge q has to be placed somewhere between the negative charges so that the attractive forces due to the negative charges may balance each other. Let the negative charges be placed at A and B and the charge +q be placed at C

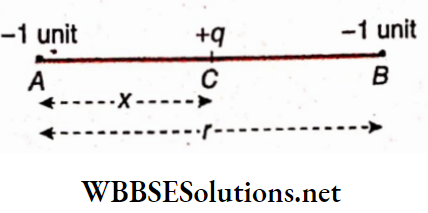
Let AB = r; AC = x

The attractive force on +q due to the negative charge at \(A=\frac{q}{x^2}\)

The attractive force on +q is due to the negative charge at

⇒ \(B=\frac{q}{(r-x)^2}\)

The charge q is at equilibrium, thus

⇒ \(\frac{q}{r^2}=\frac{q}{(r-x)^2}\)

or, \(x=\frac{r}{2}\)

The negative charges at A and B tend to move away from each other due to repulsion but are held at those points by the attractive forces of q.

At equilibrium, the attractive force between q and any negative charge = the repulsive force between two negative charges.

∴ \(\frac{q \times 1}{\left(\frac{r}{1}\right)^2}=\frac{1 \times 1}{r^2}\)

or, q = \(\frac{1}{4}\)

= 0.25 unit

This is an unstable equilibrium because a slight change in the position of any of the charges disturbs the equilibrium.

Example 14. Two identical balls each of mass m are hung from a point by two silk threads of length l. Each of them has a charge q. The angle between the two threads is negligibly small, showing that at equilibrium distance between the centres of the balls will be, \(x=\left(\frac{2 q^2 l}{m g}\right)^{1 / 3}\)
Solution:

Let at equilibrium, the two balls will be at A and B OA = OB = l and AB = x

Also, let the angle made by each thread with the vertical be d.

Three forces act on each ball at equilibrium

1. weight of the ball mg,

2. tension on thread T

3. repulsive force F acting between the balls.

Here, Tsinθ = F = \(\frac{q^2}{x^2}\); Tcosd = mg

∴ \(\tan \theta=\frac{q^2}{m g x^2} \quad\)

or, \(\sin \theta=\frac{q^2}{m g x^2}\) [∵ d is small, and = sinθ]

or, \(\frac{x}{2 l}=\frac{q^2}{m g x^2}\)

∴ \(x=\left(\frac{2 q^2 l}{m g}\right)^{1 / 3}\)

Example 15. Due to cosmic shower same amount of cations +q are stored in the atmosphere of the earth and the moon. If the gravitational attraction between the Earth and the moon is exactly equal to the electric repulsion between them. Then find the value of charge q stored in their atmosphere.
Solution:

Let the mass of the moon and the earth be Mm and Me respectively and the distance between them be r.

The repulsive force between them = \(\frac{q \times q}{r^2}=\frac{q^2}{r^2}\) and gravitational attractive force = \(=\frac{G M_e M_m}{r^2}\)

According to the problem,

⇒ \(\frac{q^2}{r^2}=\frac{G M_e M_m}{r^2}\)

or, \(q=\sqrt{G M_e M_m} \text { esu }\)

Example 16. The value of the electric field at a region of space is given by, E = Ar where A = 100 V.m-2 and r = distance (in m) from the origin inside the electric field. Find the amount of charge enclosed in a sphere of radius 20 cm centred at the origin. Given, \(\frac{1}{4 \pi \epsilon_0}=9 \times 10\) \(\frac{1}{4 \pi \epsilon_0}=9 \times 10^9 \mathrm{~N} \cdot \mathrm{m}^2 \cdot \mathrm{c}^{-2}\)
Solution:

We know, \(E=\frac{1}{4 \pi \epsilon_0} \cdot \frac{q}{r^2}\)

Again according to the problem,

E = Ar = 100 r V.m^-1

∴ \(100 r=9 \times 10^9 \cdot \frac{q}{r^2} \quad \text { or, } 100 r^3=9 \times 10^9 \times q\)

or, \(q=\frac{100 \times\left(20 \times 10^{-2}\right)^3}{9 \times 10^9}\)

= 888.8 x 10^-13 C

Electric Field at a Point Near a Charged Conductor:

Suppose, a spherical conductor of radius r placed in a medium of permittivity e has +Q amount of charge. The electric field is to be calculated at a point P close to the sphere.

If we place a unit positive charge at P, the force acting on it gives the electric field at P. It can be proved that to determine the electric field at an external point due to a charged spherical conductor, we can assume that the whole charge of the sphere is concentrated at the centre of the sphere.

So the distance between the charge concentrated at the centre O of the sphere and the unit positive charge at P may be taken to be equal to the radius of the sphere.

The electric field at P

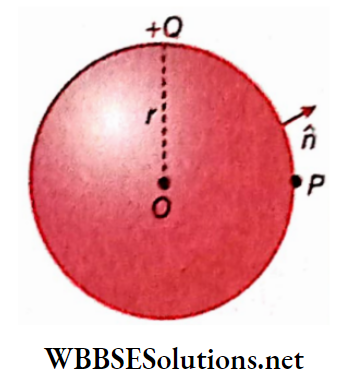
⇒ \(E=\frac{Q}{4 \pi \epsilon r^2}=\frac{Q}{4 \pi \kappa \epsilon_0 r^2}\) [K = dielectric constant of the medium]

⇒ \(\frac{4 \pi r^2 \sigma}{4 \pi \kappa \epsilon_0 r^2}\) [.. Q = 4πr2σ]

∴ \(E=\frac{\sigma}{\kappa \epsilon_0}\)

In vector form, \(\vec{E}=\frac{\sigma}{\kappa \epsilon_0} \hat{n}\)

where \(\hat{n}\) is the unit vector normal in the direction of \(\vec{E}\).

It may be noted that the electric field E does not depend on the radius of the sphere.

So the above equation applies not only to a spherical conductor but also to a conductor of any shape.

Electrostatics

Electric Field Electric Field Lines Or Electric Lines Of Force

Electric field intensity acts on every point of an electric field. This intensity has a definite magnitude and direction. But the magnitude and direction is different at different points.

If a free isolated unit positive charge is placed at a point in an electric field, it will experience a force along the tangent of the electric field line at that point and will move under its influence.

The path described by this unit’s positive charge is called the electric field line.

The direction of the force on it at that point gives the direction of the line. The direction is indicated by an arrowhead on the field line.

The tangent at any point on a field line gives the direction of the electric field intensity at that point.

Remember that the lines have no real existence. Scientist FaraDay introduced these imaginary lines to explain attraction and repulsion between two charged bodies and mentioned some definite properties of these lines

Properties of electric field fines:

1. Electric field lines start from a positive charge and end on a negative charge. There is no electric field line inside a conductor For an isolated positive charge, the lines start from it and diverge to infinity and for an isolated negative charge, the lines of force come from infinity and converge on the charge.
2. Two field lines can never intersect Because if they do so, then two tangents could be drawn at the point of intersection, which would indicate two directions of the electric field intensity at a single point But this is not possible.
3. Electric field lines are always normal to the surface of a charged conductor.
4. The electric field lines tend to contract longitudinally This phenomenon explains the attraction between two unit charges.
5. The lines tend to repel one another laterally. This explains the repulsion between two like charges.
6. At the two ends of each field line, there should be equal and opposite charges.
7. No line starts from a conductor and ends on it From this fact it may be concluded that there are no lines inside a low conductor
8. A region of closely spaced field lines indicates a strong electric field; sparsely spaced lines indicate a region of weak electric field.

Maps Of field lines: Maps of field lines are shown below for a few special cases.

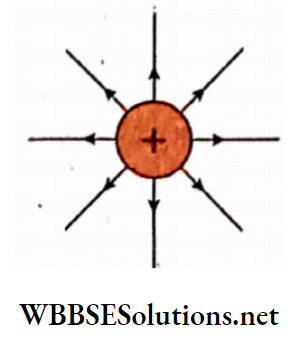
An isolated positive charge: In this case, the field lines are directed away from the charge and are arranged uniformly. If they are drawn backwards, they meet at the centre of the charge

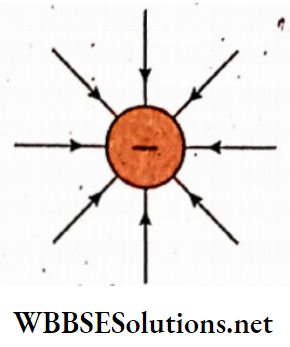
An isolated negative charge: In this case, the field lines are directed towards the charge and arranged uniformly. If the lines are extended, they meet at the centre of the charge. The lines are similar to those but are oppositely directed.

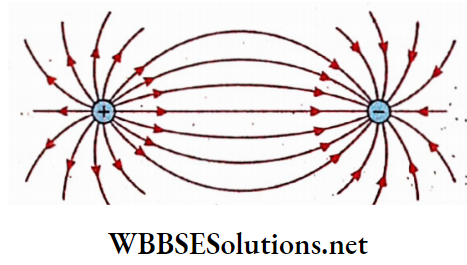
Two equal but opposite charges: Here the lines start from the positive charge and some of them end at the negative charge. Due to the longitudinal contractive tendency of the lines, two opposite charges attract each other. This mapping of the lines is similar to that of a bar magnet.

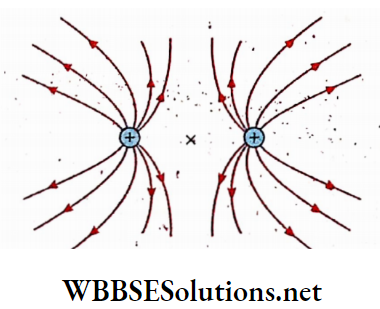
Two equal and similar charges: Here the field lines starting from the charges repel one another and travel off to an infinite distance. At the neutral point, denoted by the x sign, the resultant intensity is zero. At this point, due to both charges, the intensities are equal and opposite. As the lines repel laterally (i.e., sidewise), repulsion takes place between the two charges.

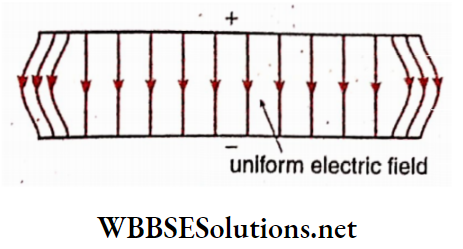
Uniform electric field: The field lines of a form electric field are represented by parallel equidistant straight lines.

It may be noted that all the electric fields are non-uniform.

Electric intensity in terms of field lines: According to scientist Maxwell, the number of lines emanating from a charge q placed in a medium of permittivity

⇒ \(\epsilon \text { is } \frac{q}{\epsilon} \text { (in CGS system } \frac{4 \pi q}{k} \text { ) }\).

Now imagine a sphere of radius r having a charge q at its centre. L field lines will cross the surface area of the sphere normally. Since the surface area of the sphere is 4πr2, the number of field lines passing normally per unit area of the sphere = \(\frac{q / \epsilon}{4 \pi r^2}\)

= \(\frac{q}{4 \pi \epsilon r^2}\)

But due to the charge q, the electric field at any point on the surface of the sphere = \(\frac{q}{4 \pi \epsilon r^2}\). So we can say that the electric field at a point is equal, to the number of field lines passing mally through the unit area surrounding that point.

Electrostatics

Electric Field Electric Dipole

Definition:

An electric dipole is a combination of two equal but opposite point charges separated by a small distance.

Example: The molecules of water (H₂O), ammonia (NH₃), hydrochloric acid (HCl), carbon dioxide (CO₂) etc. are electric dipoles. A water molecule and a hydrochloric acid molecule

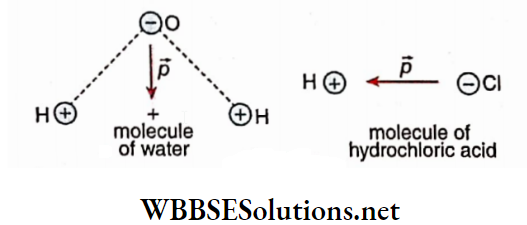
The total charge of the electric dipole is zero (as, +q- q = 0 ), but the field of the electric dipole is not zero.

Dipole moment: The moment of an electric dipole is the product of the magnitude of either charge (q) and the distance (21) between them.

According to, dipole moment, p = q.21…..(1)

The electric dipole moment is a vector quantity. It is directed along the axis of the dipole (the line joining the two charges) from the negative to the positive charge.

The vector form of dipole moment is \(\vec{p}=2 q \vec{l}\)

Unit and dimension: in the CGS system the unit of dipole moment is state. cm and in SI it is C.m . Its dimension is [p] = LTI

Field Intensity at a Point on the Axis of a Dipole:

Let the two charges -q and +q separated by a distance of 2l form an electric dipole AB. AB = 2l and dipole moment, p = 2lq. Electric field intensity at a point P situated on the axis of the dipole is to be calculated. This position of P concerning the dipole is called the end-on position or axial position.

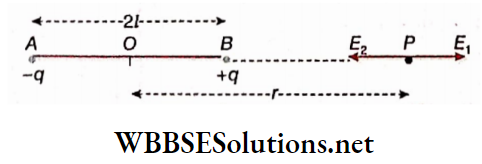
Let the distance of the point P from the centre 0 of the dipole be r.

Suppose, the dipole is placed in a medium of dielectric constant k. The permittivity of air medium or vacuum is ∈₀.

Now, field intensity at P due to the charge +q

⇒ \(E_1=\frac{q}{4 \pi \kappa \epsilon_0(r-l)^2} ; \text { along } \overrightarrow{O P}\)

Again, field intensity at P due to the charge -q,

⇒ \(E_2=\frac{q}{4 \pi \kappa \epsilon_0(r+l)^2} ; \text { along } \underset{;}{\overrightarrow{P O}}\)

As and E₂ acting in opposite directions and E₁> E₂, therefore, resultant field intensity at P,

E = E₁-E₂

⇒ \(\frac{q}{4 \pi \kappa \epsilon_0}\left[\frac{1}{(r-l)^2}-\frac{1}{(r+l)^2}\right]\)

⇒ \(\frac{q}{4 \pi \kappa \epsilon_0} \cdot \frac{4 r l}{\left(r^2-l^2\right)^2}\)

⇒ \(\frac{2 p r}{4 \pi \kappa \epsilon_0\left(r^2-l^2\right)^2}\) [… p = 2lp] ….(1)

The direction of E is along \(\vec{OP}\)

For air or vacuum, K = 1

∴ \(E=\frac{2 p r}{4 \pi \epsilon_0\left(r^2-l^2\right)^2}\)…(2)

The vector form of equation (1) is

⇒ \(\vec{E}=\frac{2 \vec{p} r}{4 \pi \kappa \epsilon_0\left(r^2-l^2\right)^2}\)

The corresponding CGS expression of equation (2) is

⇒ \(E=\frac{2 p r}{\left(r^2-l^2\right)^2}\)

Special case:

If the point P is far away from the centre O of the dipole i.e., if r >> l, then neglecting l₁ in comparison with r₂, we get from equations (1) and (2) respectively,

⇒ \(E=\frac{1}{4 \pi \kappa \epsilon_0} \cdot \frac{2 p}{r^3}\)….(3)

and \(E_v=\frac{1}{4 \pi \epsilon_0} \cdot \frac{2 p}{r^3}\) …(4)

For this end-on position, \(\vec{p}\) and \(\vec{E}\) are along the same direction.

Field Intensity at a Point on the Perpendicular Bisector of a Dipole:

Any line passing through the centre of the dipole and perpendicular to its axis is called its perpendicular bisector.

Let the two charges -q and +q separated by a distance of 21 form an electric dipole AB. AB = 21 and dipole moment, p = 2lq. Electric field intensity at a point P situated on the perpendicular bisector of the dipole is to be calculated.

This position of P concerning the dipole is called broadside on or equatorial position

Let the distance of the point P from the centre of the tire dipole be r.

Suppose, the dipole is placed in a medium of dielectric constant K.

The permittivity of air medium or vacuum is ∈₀.

Now, field intensity at P due to the charge +q,

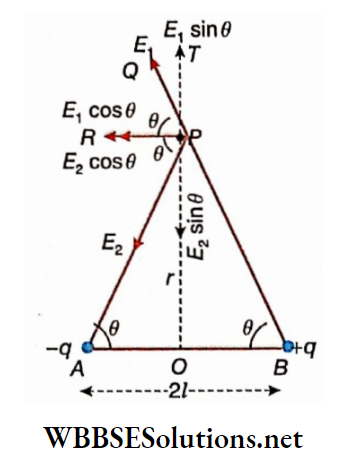
⇒ \(E_1=\frac{1}{4 \pi \kappa \epsilon_0} \cdot \frac{q}{(B P)^2} ; \text { along } \overrightarrow{B P}\)

Again, field intensity at P due to the charge -q,

⇒ \(E_2=\frac{1}{4 \pi \kappa \epsilon_0} \cdot \frac{q}{(A P)^2} \text {; along } \overrightarrow{P A}\)

Since BP = AP

∴ \(E_1=E_2 \text { and } \angle P A B=\angle P B A=\theta \text { (say) }\)

If PR be parallel to BA, then

∠QPR = ∠PBA = 0 and ∠RPA = ∠PAB = 0

Now, the field intensity E₁ is resolved into two perpendicular components along PR and PT (extended OP). The components are respectively E₁cosθ and E₁sinθ.

Similarly, the field intensity E₂ is resolved into two perpendicular components along PR and PO. The components are respectively E₂cosθ and E₂sinθ.

The sine components of PT and PO being equal and opposite balance each other. So the resultant field intensity at P is

E = E₁cosθ + E₂cosθ

= 2E₁ cosθ [∵ E₁ = E₂]

⇒ \(\frac{2}{4 \pi \kappa \epsilon_0} \cdot \frac{q}{(BP)^2} \cos \theta\)

⇒ \(\frac{2}{4 \pi \kappa \epsilon_0} \cdot \frac{q}{\left(r^2+l^2\right)} \cdot \frac{l}{\sqrt{r^2+l^2}}\) [∵ OP = r and OB = OA = l]

⇒ \(E=\frac{l}{4 \pi \kappa \epsilon_0} \cdot \frac{p}{\left(r^2+l^2\right)^{3 / 2}}\) [∵ p = 2lp]…(1)

The direction of E is along \(\vec{PR}\)

In air or vacuum, K = 1

∴ \(E=\frac{1}{4 \pi \epsilon_0} \cdot \frac{p}{\left(r^2+l^2\right)^{3 / 2}}\) ….(2)

The vector form of equation (1) is

⇒ \(\vec{E}=-\frac{1}{4 \pi \kappa \epsilon_0} \cdot \frac{\vec{p}}{\left(r^2+l^2\right)^{3 / 2}}\)

The corresponding CGS expression of equation (2) is

⇒ \(E=\frac{p}{\left(r^2+l^2\right)^{3 / 2}}\)

Special case:

If the point P is far away from the centre O of the dipole i.e., if r >> l, then neglecting l₂ in comparison with r₂, we get from equations (1) and (2) respectively,

⇒ \(E=\frac{1}{4 \pi \kappa \epsilon_0}: \frac{p}{r^3}\)….(3)

and \(E=\frac{1}{4 \pi \epsilon_0} \cdot \frac{p}{r^3}\)…(3)

For this broadside-on position, \(\vec{p}\) and \(\vec{E}\) are oppositely directed.

It is to be noted that, when point P is far away from the centre of the dipole, the electric field intensity at a point in the axial position is double the electric field intensity at a point in the equatorial position.

Field Intensity at any Point due to an Electric Dipole:

Suppose, the point P is situated at a distance r from the centre O of the dipole and the line OP makes an angle 0 with the axis of the dipole. The dipole moment \(\vec{p}\) acts along \(\vec{AB}\) and the length of the dipole is negligible in comparison to r.

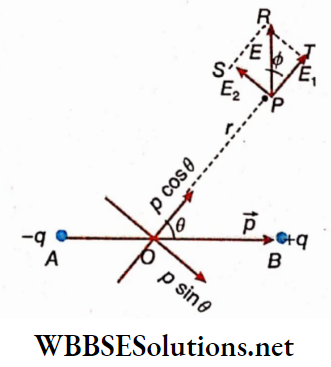
⇒ \(\vec{p}\) is resolved into two perpendicular components:

Pcosθ; along \(\vec{OP}\)

psinθ; along perpendicular to \(\vec{OP}\)

Hence, point P is situated on the axial line of a dipole of moment pcosθ, and the equatorial line of a dipole of moment psinθ.

Let \(\vec{E}_1 \text { and } \vec{E}_2\) be the Intensities at P due to the components pcosθ and psinθ respectively, and the resultant intensity at P be \(\vec{E}\)

So, \(\vec{E}=\vec{E}_1+\vec{E}_2\)

∴ \(E^2=E_1^2+E_2^2 \quad\)

or, \(E=\sqrt{E_1^2+E_2^2}\)

Suppose, the dipole is placed in a medium of dielectric constant K. The permittivity of air medium or vacuum is ∈₀.

From equation (3)

⇒ \(E_1=\frac{1}{4 \pi \kappa \epsilon_0} \cdot \frac{2 p \cos \theta}{r^3} \text {; along } \overrightarrow{P T}\)

and \(E_2=\frac{1}{4 \pi \kappa \epsilon_0} \cdot \frac{p \sin \theta}{r^3} \text {; along } \overrightarrow{P S}\)

∴ Resultant intensity at P,

⇒ \(E=\sqrt{\left(\frac{1}{4 \pi \kappa \epsilon_0}\right)^2 \cdot\left(\frac{p}{r^3}\right)^2\left[4 \cos ^2 \theta+\sin ^2 \theta\right]}\)

or, \(E=\frac{1}{4 \pi \kappa \epsilon_0} \cdot \frac{p}{r^3} \sqrt{3 \cos ^2 \theta+1}\)…(1)

The direction of E is along \(\vec{PR}\).

Suppose, \(\angle T P R=\phi\)

∴ \(\tan \phi=\frac{E_2}{E_1}=\frac{1}{2} \tan \theta\)…(2)

For air or vacuum, k = 1

∴ \(E=\frac{1}{4 \pi \epsilon_0} \cdot \frac{p}{r^3} \sqrt{3 \cos ^2 \theta+1}\)….(3)

The corresponding CGS expression of equation (3) is

⇒ \(E=\frac{p}{r^3} \sqrt{3 \cos ^2 \theta+1}\)

Special cases:

1. End-on position: If the point P is on the end-on position, i.e., on the axis of the dipole, θ = 0° or cosθ = 1.

Therefore, from equation (1) we get

⇒ \(E=\frac{1}{4 \pi \kappa \epsilon_0} \cdot \frac{p}{r^3} \sqrt{3+1}=\frac{1}{4 \pi \kappa \epsilon_0} \cdot \frac{2 p}{r^3}\)

2. Broadside-on position or equational position: If the point P is on the broadside-on position, i.e., on the perpendicular bisector of the dipole, 0 = 90° or, cosθ = 0.

Therefore, from equation (1) we get,

⇒ \(E=\frac{1}{4 \pi \kappa \epsilon_0} \cdot \frac{p}{r^3} \cdot \sqrt{0+1}=\frac{1}{4 \pi \kappa \epsilon_0} \cdot \frac{p}{r^3}\)

Torque Acting on an Electric Dipole in a Uniform Electric Field:

Consider an electric dipole consisting of charges -q at A and +q at B separated by a distance of 2l. So dipole moment, p = q 21. It is a vector quantity and its direction is from negative to positive charge along the axis of the dipole.

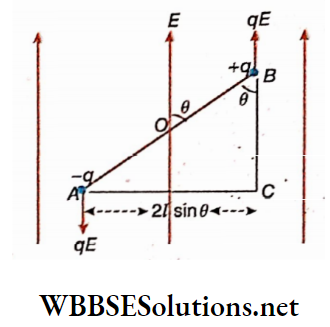
Suppose, the dipole makes an angle 6 with a uniform electric field of strength \(\vec{E}\). The force on charge +q is qE, along the direction of \(\vec{E}\) and the force on charge -q is qE, opposite to the direction of \(\vec{E}\).

These forces are equal in magnitude but opposite in direction.

Therefore, the net force on the dipole, \(\vec{F}_{\text {net }}=q \vec{E}-q \vec{E}=0\);

i.e., the net force on an electric dipole in a form electric field is zero.

Since these two forces are equal in magnitude, opposite in direction and act at different points, they constitute a couple. If the magnitude of the torque is \(\tau\), then

⇒ \(\tau\) = either force x perpendicular distance between the lines of action of the two forces

= qE x AC

= qE x 2lsinθ

= q x 2l x Esinθ

or, \(\tau\) = pEsinθ….(1)

The torque acting on the dipole tends to bring it along the direction of the electric field.

Special cases:

1. When θ = 90°, the magnitude of the torque becomes maximum, i.e., \(\tau\) = pE. In this case, the electric dipole is in stable equilibrium.

Now, if E = 1, \(\tau\) = p = dipole moment It provides an alternative definition of electric dipole moment

The moment of an electric dipole is defined as the torque acting on it when it is placed at right angles to a uniform electric field of unit strength.

2. When θ = 0° or 180° , the magnitude ofthe torque is minimum, i.e., r = 0. In this case, the electric dipole is in an unstable equilibrium.

Torque in vector form: Torque \(\vec{\tau}\) acting on a dipole of moment \(\vec{p}\) when placed in a uniform electric field \(\vec{E}\) is given in vector form as,

⇒ \(\vec{\tau}=\vec{p} \times \vec{E}\)….(2)

According to the rule of the cross product, \(\vec{\tau}\) is perpendicular to the plane containing \(\vec{p}\) and \(\vec{E}\)

Electrostatics

Electric Field Numerical Examples

Example 1. How many field lines would emanate from a 1 C positive charge placed in a vacuum?
Solution:

The number of lines emanated

⇒ \(\frac{1}{\epsilon_0}=\frac{1}{8.854 \times 10^{-12}}=1.129 \times 10^{11}\)

= 1.129 X 10¹¹

Example 2. A straight rod of length Z placed in a vacuum is charged uniformly with an amount q of charge. Calculate the electric field intensity in SI at a point on the axis of the rod at a distance x from Its nearer end. Write the result in the CGS system also.
Solution:

The length of the rod AB is Z. Let P be a point at a distance x from the end B. Since the rod is uniformly charged with q, the linear charge density of the rod is

⇒ \(\lambda=\frac{q}{l}\).

Consider a small length dr of the rod at a distance r from P.

Charge of this portion of length dr,

⇒ \(d q=\lambda d r=\frac{q}{l} d r\)

Electric field intensity at P due to this charge dq,

⇒ \(d E=\frac{1}{4 \pi \epsilon_0} \cdot \frac{\frac{q}{l}}{r^2} d r=\frac{1}{4 \pi \epsilon_0} \cdot \frac{q}{l} \frac{d r}{r^2}\)

Therefore, field intensity at P due to the whole charged rod,

⇒ \(E=\int d E=\frac{q}{4 \pi \epsilon_0 l} \int_x^{l+x} \frac{1}{r^2} d r=\frac{q}{4 \pi \epsilon_0 l} \cdot\left[-\frac{1}{r}\right]_x^{l+x}\)

⇒ \(\frac{q}{4 \pi \epsilon_0 l} \cdot\left[\frac{1}{x}-\frac{1}{l+x}\right]\)

⇒ \(\frac{q}{4 \pi \epsilon_0 l} \cdot \frac{l}{x(l+x)}=\frac{1}{4 \pi \epsilon_0} \cdot \frac{q}{x(l+x)} \text {; along } \overrightarrow{B P}\)

In CGS system, replacing \(\epsilon_0 \text { by } \frac{1}{4 \pi}\), the electric field intensity becomes

⇒ \(E=\frac{q}{x(l+x)} ; \text { along } \overrightarrow{B P}\)

Example 3. Two point charges are placed on the y-axis at y = +a and y = -a in vacuum. The magnitude of each charge is q. Determine the electric field intensity at P(x, 0) on the x-axis.
Solution:

Suppose, electric field intensity at the point P due to the charges at A and B are E₁ and E₂, respectively.

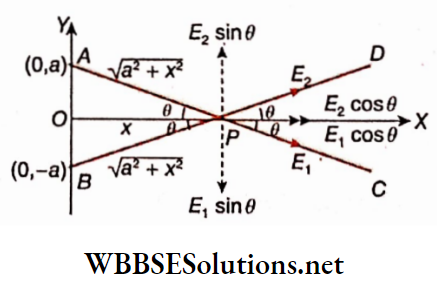
∴ \(E_1=\frac{1}{4 \pi \epsilon_0} \cdot \frac{q}{A P^2} ; \text { along } \overrightarrow{P C}\)

⇒ \(\frac{1}{4 \pi \epsilon_0} \cdot \frac{q}{a^2+x^2} \text {; along } \overrightarrow{P C}\)

⇒ \(E_2=\frac{1}{4 \pi \epsilon_0} \cdot \frac{q}{B P^2} ; \text { along } \overrightarrow{P D}\)

⇒ \(\frac{1}{4 \pi \epsilon_0} \cdot \frac{q}{a^2+x^2} \text {; along } \overrightarrow{P D}\)

∴ \(E_1=E_2 \text { (in magnitude) }=E \text { (say) }\)

Both E₁ and E₂ are resolved into two perpendicular components. It is seen that the sine components are equal and opposite, and balance each other. But the cosine components are added up and they act In the same direction.

∴ The field strength at P

⇒ \(2 E \cos \theta=2 \times \frac{1}{4 \pi \epsilon_0} \cdot \frac{q}{\left(a^2+x^2\right)} \cdot \frac{x}{\sqrt{a^2+x^2}}\)

⇒ \(\frac{1}{4 \pi \epsilon_0} \cdot \frac{2 q_x}{\left(a^2+x^2\right)^{3 / 2}}\)

Example 4. An electric dipole placed in a vacuum is formed by two equal but opposite charges each of magnitude 1μC separated by a distance of 2 cm. Calculate the electric field intensities in the following cases:

1. At a point on the axis of the dipole situated at a distance 60 cm away from its centre.
2. At a point on the perpendicular bisector of the dipole situated at a distance 60 cm away from its centre.

Solution:

Moment of the electric dipole,

p = q 21 = 1 x 10^-6 X 2 X 10^-6

= 2 x 10^-8 C.m

1. Electric field intensity on the axis of the dipole,

⇒ \(E_1=\frac{1}{4 \pi \epsilon_0} \cdot \frac{2 p}{r^3}=\frac{9 \times 10^9 \times 2 \times 2 \times 10^{-8}}{(0.6)^3}\)

= 1666.6N.C^-1; along the axis

2. Electric field intensity on the perpendicular bisector of the dipole

⇒ \(E_2=\frac{1}{4 \pi \epsilon_0} \cdot \frac{p}{r^3}=\frac{1}{2} E_1\)

= 833.3 N.C^-1; parallel to the axis of the dipole

Example 5. Three charges q, -2q and q are placed at three vertices of an equilateral triangle. Determine the equivalent dipole moment of the system
Solution:

The given system is a combination of two dipoles at an angle 60° with each other.

Let the dipole moment of one dipole is p₁ and that of another dipole is p₂, the length of each side of the equilateral triangle is a.

∴ Equivalent dipole moment,

p = p₁cos30° + p₂cos30° [∵ sine components cancel each other]

= 2qacos30° [∵ p₁ = p₂ = qa]

⇒ \(2 q a \times \frac{\sqrt{3}}{2}=\sqrt{3} q a\)

Example 6. Electric field intensities at two axial points of an electric dipole at distances 5 cm and 10cm from its centre are 2.5 x 10⁴ N.C^-1 and 2 X 10³ N.C^-1 respectively. The dipole is placed In the air. Determine the length of the dipole.
Solution:

Suppose, the length of the electric dipole = 21.

We know,

⇒ \(E=\frac{1}{4 \pi \epsilon_0} \cdot \frac{2 p r}{\left(r^2-l^2\right)^2}\)

According to the question

⇒ \(2.5 \times 10^4=9 \times 10^9 \cdot \frac{2 p \times 0.05}{\left[(0.05)^2-l^2\right]^2}\)…(1)

and \(2 \times 10^3=9 \times 10^9 \cdot \frac{2 p \times 0.1}{\left[(0.1)^2-l^2\right]^2}\)….(2)

Dividing (1) by (2) we get,

⇒ \(1.25 \times 10=\frac{\left[(0.1)^2-l^2\right]^2 \times 0.05}{\left[(0.05)^2-l^2\right]^2 \times 0.1}\)

or, \(25=\frac{\left[(0.1)^2-l^2\right]^2}{\left[(0.05)^2-l^2\right]^2} \quad\)

or, \(5=\frac{(0.1)^2-l^2}{(0.05)^2-l^2}\)

or, 2l = 0.05m

∴ The length of the electric dipole = 0.05 m.

Example 7. An electric dipole is formed by two equal but opposite charges, each of magnitude 1μC, separated by a distance of 5 cm. What is the magnitude of the torque required to place the dipole at right angles to an electric field of Intensity 3 x 10s N C^-1?
Solution:

Here,

p = q X 2l = 1 x 10^-6 x 5 x 10^-2

= 5 x 10^-8 C.m

E = 3 X 10⁵ N – C^-1 and θ = 90°

∴ The required torque,

⇒ \(\tau\) = pEsinθ

= 5 x 10^-8 x 3 x 10⁵ x sin90°

= 0.015 N.m

Example 8. An electric dipole is formed by placing charges ±20 x 10^-6 C at a distance of 2 min. Calculate the electric field at a point on the perpendicular bisector of the axis of the dipole situated at a distance of 10 cm from the midpoint of the dipole.
Solution:

Electric field, \(E=\frac{1}{4 \pi c_0} \cdot \frac{p}{\left(r^2+l^2\right)^{3 / 2}}\)

[here, l = 1 x 10^-3m , p = q x 21 = 20 X 10^-6 X 2 X 10^-3 =40 X 10^-9 cm]

∴ \(E=\frac{9 \times 10^9 \times 40 \times 10^{-9}}{\left[(10 \times 10)^{-2}+\left(1 \times 10^{-3}\right)^2\right]^{3 / 2}}\)

= \(\frac{360}{\left(10^{-2}+10^{-6}\right)^{3 / 2}}\)

= 36 X 10^-4 N.C^-1

Electrostatics

Electric Field Area vector Electric flux And Gauss Theorem

Area vectors: In different cases of physics, the surface area is treated as a vector. The magnitude of the area is represented by the length of the vector and the direction of the area vector is given by the outward drawn normal to the closed surface.

Suppose, dS is a small surface area on the surface S. A normal is drawn in the outward direction on the surface at dS. So \(d \vec{s}\) is an area vector.

Electric flux: Electric flux linked with a surface gives an idea of the number of field lines passing through the surface. The number of electric field lines passing normally through a surface Is called the electric flux through the surface. It is denoted by the symbol Ω. It is a scalar quantity.

Let us consider a small area element \(d \vec{S}\) on a surface S in an electric field of inequality \(\vec{E}\).

Let θ be the angle between E and \(d \vec{S}\).

Therefore, electric flux passes through the surface \(d \vec{S}\).

⇒ \(d \phi=\vec{E} \cdot d \vec{S}=E d S \cos \theta\)

= (Ecosθ)dS

= E_ndS….(1)

Here, E_n = Ecosθ = normal component of electric field intensity.

As the whole surface is the sum of a large number of such small-area elements, the electric flux passing through the surface S is given by

⇒ \(\phi=\int_S \vec{E} \cdot d \vec{S}=\int_S E_n d S=\int_S E d S \cos \theta\)….(2)

where \(\int_S\) represents the integral taken over the whole surface.

Special cases:

1. If an electric field \(\vec{E}\) is normal to a surface, then 0 = 0° . So the electric flux linked with the surface,

⇒ \(\phi=\int_S E d S \cos 0^{\circ}=E S \text { (maximum) }\)

2. If an electric field \(\vec{E}\) Is parallel to a surface, then 0 = 90°.

So the electric flux linked with the surface,

⇒ \(\phi=\int_S E d S \cos 90^{\circ}=0\)

Closed surface:

Now, let us consider a definite volume enclosed by a dosed surface S. Naturally, the electric flux linked with this surface S will be,

⇒ \(\phi=\oint_S \vec{E} \cdot d \vec{S}=\oint E d S \cos \theta\)….(3)

Here, the symbol \(\oint_S\) stands for integration across all the surface elements d\(\vec{S}\) which has different directions around the entire closed surface S.

Moreover, \(\vec{E}\) may be different at different points on the surface, So, none of 000 and E are constant when the entire surface is taken into account.

As a result, the integral in equation (3) is of ten very hard to compute. However, specific symmetries in connection with some special systems help us to tackle the integral effectively.

Positive electric flux:

The electric flux linked with a closed surface is taken as positive when the electric field vector is outwardly directed. Here, the volume acts as a source of field lines.

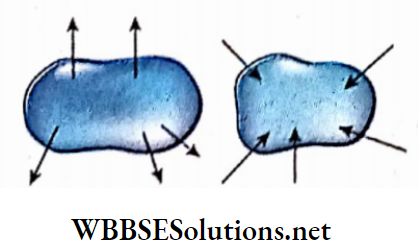
Negative electric flux:

The electric flux linked with a closed surface is taken as negative when the electric field vector is Inwardly directed. Here, the volume acts as a sink of field lines.

Unit and dimension of electric flux: In SI, a unit of electric flux is N.m².C^-1 or V m

Dimension of electric flux

= dimension of electric intensity x dimension of area

= MLT^-3l^-1 x L²

= ML³T^-3T^-1

Solid Angle:

If a surface is placed at any distance in front of a point, then it subtends a solid angle at that point. In this surface of area, S subtends a solid angle H at the point O.

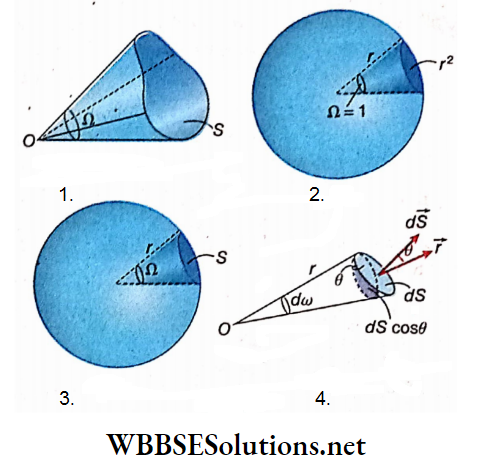
Unit solid angle:

The solid angle subtended by an area r2 on a sphere of radius r, at the centre of the sphere, is called a unit solid angle. This unit of solid angle is called steradian (sr).

The solid angle subtended by a surface of area S on a sphere of radius r, at the centre of the sphere, is given by,

⇒ \(\Omega=\frac{S}{r^2}=\frac{\text { area of this spherical surface }}{\text { (radius) }^2}\)

The dimension of Ω

⇒ \([\Omega]=\frac{\text { dimension of area }}{(\text { dimension of radius })^2}=\frac{\mathrm{L}^2}{\mathrm{~L}^2}\)

= 1

So, the solid angle is a dimensionless quantity. Steradian is a dimensionless unit.

The solid angle at the centre of a sphere of radius r due to the entire surface of the sphere

⇒ \(\frac{\text { area of the entire surface }}{r^2}\)

⇒ \(\frac{4 \pi r^2}{r^2}=4 \pi\)

If the surface is a closed surface (not necessarily a sphere), then it also subtends a solid angle of 4π at any of its internal points.

Now, let us take a surface dS at a distance r from point O. In general, dS is not normal to the radius vector \(\vec{r}\). If 000 be the angle between d\(\vec{S}\) and \(\vec{r}\), then the component of dS along the normal to r = dScosθ.

So, the solid angle subtended at O by the surface dS is,

⇒ \(d \omega=\frac{d S \cos \theta}{r^2}\)

Obviously, if a closed surface of any shape surrounds the point O, then the solid angle subtended at O by that closed surface,

⇒ \(\oint d \omega=\oint \frac{d S \cos \theta}{r^2}=4 \pi\)

Statement and Proof of Gauss’ Theorem:

Statement: The net electric flux linked with a closed surface is \(\frac{1}{∈}\) times the net charge within the surface.

Mathematically, \(\phi=\oint_S \vec{E} \cdot d \vec{S}:=\frac{q}{\epsilon}\)….(1)

Here q is the total charge enclosed by the surface and e is the permittivity of the medium.

For vacuum, \(\phi=\oint_S \vec{E} \cdot d \vec{S}=\frac{q}{\epsilon_0}\)

Proof:

Let S be a closed surface enclosing +q charge situated at O. P is a point situated on the surface and dS is a small area on the surface surrounding P. Suppose, OP = r.

Electric field intensity at P due to the charge +q,

⇒ \(E=\frac{1}{4 \pi \epsilon} \cdot \frac{q}{r^2}\)

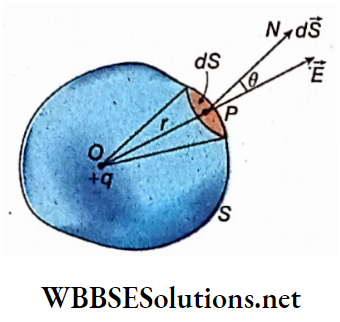
Let \(\vec{PN}\) be the normal at P on dS and 0 be the angle between \(\vec{E}\) and \(\vec{PN}\).

∴ The electric flux through the area dS

⇒ \(\vec{E} \cdot d \vec{S}=E d S \cos \theta\)

⇒ \(\frac{1}{4 \pi \epsilon} \cdot \frac{q}{r^2} d S \cos \theta\)

= \(\frac{q}{4 \pi \epsilon} \cdot \frac{d S \cos \theta}{r^2}\)

= \(\frac{q}{4 \pi \epsilon} \cdot d \omega\)

How, \(d \omega=\frac{d S \cos \theta}{r^2}\) = solid angle subtended at O by the area dS.

Therefore, net flux linked with the entire closed surface,

⇒ \(\phi=\oint_S \frac{q}{4 \pi \epsilon} d \omega\)

= \(\frac{q}{4 \pi \epsilon} \oint_S d \omega\)

= \(\frac{q}{4 \pi \epsilon} \cdot 4 \pi\)

= \(\frac{q}{\epsilon}\) [because a closed surface subtends a solid angle An at any point
within the surface]

If q₁, q₂ ….. qn be the charges lying within the closed surface and E₁, E₂,…, E_n be the corresponding electric fields, the net flux linked with the closed surface will be,

⇒ \(\phi=\oint_S \vec{E} \cdot d \vec{S}=\oint_S\left(\vec{E}_1+\vec{E}_2+\cdots+\vec{E}_n\right) \cdot d \vec{S}\)

⇒ \(\frac{1}{\epsilon}\left(q_1+q_2+\cdots+q_n\right)\)

= \(\frac{Q}{\epsilon}\)

where Q is the net charge inside the closed surface.

According to the nature of the charges (positive and negative), positive or negative signs are to be used in the sum of the charges.

Electric flux due to a charge lying outside a closed surface:

Let a charge of +q be placed at O outside the closed surface S. A cone of solid angle da) having its vertex at O intercepts small areas dS₁ and dS₂ on the closed surface. Let P be a point on dS₁ and Q be another point on dS₂. E₁ and E₂ are the electric field intensities at P and Q, respectively

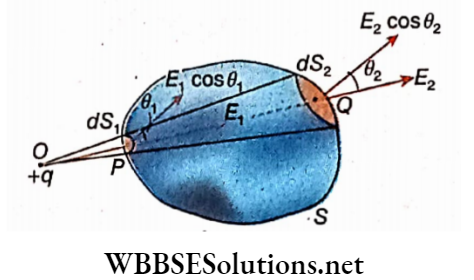
Suppose, OP = r₁ and OQ = r₂.

Component of E₁ along the normal to dS₁ = E₁cosθ₁ and a component of E₂ along the normal to dS₂ = E₂cosθ₂. Since electric flux through dS₁ directed inwards is negative, the flux through dS₂ directed outwards is positive.

Therefore, electric flux through dS₁ = -E₁cosθ₁dS₁ and electric flux through dS₂ = E₂cosθ₂dS₂

So, net electric flux through dS₁ and dS₂

= -E₁cosθ₁dS₁+E₂cosθ₂dS₂

⇒ \(-\frac{1}{4 \pi \epsilon} \cdot \frac{q}{r_1^2} \cos \theta_1 d S_1+\frac{1}{4 \pi \epsilon} \cdot \frac{q}{r_2^2} \cos \theta_2 d S_2\)

⇒ \(-\frac{q}{4 \pi \epsilon} \cdot \frac{d S_1 \cos \theta_1}{r_1^2}+\frac{q}{4 \pi \epsilon} \cdot \frac{d S_2 \cos \theta_2}{r_2^2}\)

⇒ \(-\frac{q}{4 \pi \epsilon} \cdot d \omega+\frac{q}{4 \pi \epsilon} \cdot d \omega\)

= 0

Clearly, this result is in accordance with equation (1), because in this case, q = charge within the closed surface = 0.

Discussions:

1. Only the charges inside a volume contribute to the electric flux linked with the surface enclosing that volume.
2. The net charge q is the algebric sum of all the charges, positive or negative, inside the closed surface.
3. If the net charge q is positive, the outward flux across the closed surface is greater than the inward flux; the enclosed volume acts as a source.
4. If the net charge q is negative, the inward flux exceeds the outward flux; the enclosed volume acts as a sink.
5. If the closed surface encloses equal amounts of positive and negative charges, then the net charge q is zero; consequently, the net flux linked with the surface is also zero.
6. For Example, if a closed surface encloses an electric dipole, the net electric flux through it is zero.
7. Charges outside the closed surface have no net contribution towards the electric flux linked with

Applications of Gauss’ Theorem:

Field intensity at a point due to a point charge: We have to calculate the electric field intensity at a point situated at a distance r from a point charge q.

Let us consider a spherical Gaussian surface of radius r having the point charge q at its centre. We can say from symmetry that electric field intensity due to a positive point charge is directed radially outwards.

So intensity at any point on the Gaussian surface is perpendicular to it Therefore, \(\vec{E}\) and d\(\vec{S}\) are parallel at each point.

∴ \(\vec{E} \cdot d \vec{S}=E d S \cos 0^{\circ}=E d S\)

According to Gauss’ theorem,

⇒ \(\phi=\oint_S \vec{E} \cdot d \vec{S}=\oint_S E d S=\frac{q}{\epsilon}\) [eee = permittivity of the medium]

By symmetry, E is constant over the entire Gaussian surface.

⇒ \(\oint_S E d S=E \oint_S d S=E \cdot 4 \pi r^2\) [4πr² = surface area of the sphere]

∴ \(E \cdot 4 \pi r^2=\frac{q}{\epsilon}\)

or, \(E=\frac{q}{4 \pi \epsilon r^2}=\frac{q}{4 \pi \kappa \epsilon_0 r^2}\)….(1)

where K and eee0 are respectively the dielectric constant of the medium and the permittivity of the vacuum.

In vector form, \(\vec{E}=\frac{q}{4 \pi \kappa \epsilon_0 r^2} \hat{r}\)

where \(\hat{r}\) is a unit vector along the outward drawn normal.

Note that if the point charge is negative, q is replaced by -q.

Then E would be negative, meaning that it would be radially inwards.

Field intensity at a point due to a uniformly charged thin spherical shell:

Point outside the spherical shell:

Suppose, a thin spherical shell of radius R is charged uniformly with +q

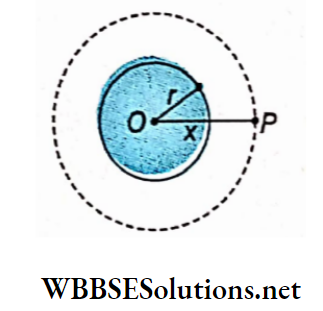
Electric field intensity at any external point P is to be calculated. Suppose, the distance of P from the centre O of the shell is r. Imagine a Gaussian spherical shell of radius r with centre at O.

We can say from symmetry that electric field intensity at each point on the surface of the spherical shell is equal and is directed along the outward drawn normal.

Suppose, the intensity at P is E. Electric flux linked with the imaginary surface of the spherical shell = E.4πr².

Since the Gaussian surface encloses the charge +q, the net flux according to Gauss’ theorem is \(\frac{q}{\epsilon}\)

∴ \(E \cdot 4 \pi r^2=\frac{q}{\epsilon}\)

or, \(E=\frac{1}{4 \pi \epsilon} \cdot \frac{q}{r^2}\)…(2)

In vector form, \(\vec{E}=\frac{1}{4 \pi \epsilon} \cdot \frac{q}{r^2} \hat{r}\)

where \(\hat{r}\) is a unit vector along the outward drawn normal.

Equation (2) shows that for an external point, a charged spherical shell behaves as though its total charge were concentrated at its centre.

Point inside the spherical shell:

The point P is situated inside the charged spherical shell. Imagine a Gaussian spherical shell of radius r with centre at O. Since the Gaussian surface does not enclose any charge (because the charge lies on the surface of the spherical shell), the flux linked with the imaginary surface of the spherical shell is given by,

E.4πr² = 0 [by Gauss’ theorem]

or, E = 0

i.e., there exists no field intensity inside a charged spherical shell.

Field intensity at a point due to an infinitely long Straight charged conducting Wire:

Consider an infinitely long thin straight wire charged uniformly.

Let +A be the linear charge density, i.e., a charge per unit length of the wire. Electric field intensity is to be calculated at P at a distance r from the wire.

Imagine a cylinder of radius r and length Z with the wire as its axis. Point P lies on the surface of such a cylinder. The surface of this cylinder will act as the Gaussian surface. All the points on the curved surface of this cylinder are equidistant from the wire.

From symmetry, it can be said that the electric field intensity at each point on the curved surface of the imaginary cylinder is equal and normally outwards.

No electric flux is linked with the two flat circular faces of the cylinder as the direction of electric field E is parallel to these two faces (i.e., the component of the electric field along the normal to the two flat circular faces is zero).

So, electric flux linked with the curved surface of the Gaussian cylinder = E 2πrl.

Now, since the cylinder encloses an amount of charge λl, by Gauss’ theorem, electric flux linked with the Gaussian cylinder = \(\frac{\lambda l}{\epsilon}\)

∴ \(E \cdot 2 \pi r l=\frac{\lambda l}{\epsilon} \text { or, } E=\frac{1}{4 \pi \epsilon} \cdot \frac{2 \lambda}{r}\)….(3)

In vector form, \(\vec{E}=\frac{1}{4 \pi \epsilon} \cdot \frac{2 \lambda}{r} \hat{r}\)

where \(\hat{r}\) is a unit vector along the outward drawn normal

Field intensity at a point due to an infinite nonconducting uniformly charged plane lamina:

Consider infinite nonconducting uniformly a surface charged plane lamina having face charge density +σ. A point P is taken in front of the plane lamina, where the electric field intensity is to be determined.

A small cylinder of cross-sectional area dS, normal to the lamina and having equal lengths on either side of it, is imagined such that P and p’ lie on its flat circular faces. The surface of this cylinder will act as the Gaussian surface.

Since the lamina is infinite, the electric field intensity at each point of the two flat circular faces of the Gaussian cylinder is equal and normally outwards due to symmetry.

The electric flux through the curved surface of the Gaussian cylinder is zero, since the direction of E is parallel to the curved surface (i.e., the component of E along the normal to the curved surface at every point is zero).

Now, electric flux through the two flat circular faces of the Gaussian cylinder = E.2dS

Now, since the cylinder encloses a number of charge cards, by Gauss’ theorem, electric flux linked with the cylinder = \(\frac{\sigma d S}{\epsilon}\)

∴ \(E \cdot 2 d S=\frac{\sigma d S}{\epsilon} \quad \text { or, } E=\frac{\sigma}{2 \epsilon}=\frac{\sigma}{2 \kappa \epsilon_0}\)….(4)

In vector form, \(\vec{E}=\frac{\sigma}{2 \epsilon} \hat{n}=\frac{\sigma}{2 \kappa \epsilon_0} \hat{n}\)

where \(\hat{n}\) is the unit vector directed normally away from the plane.

Field intensity at a point due to a charged conductor:

Let C be a charged conductor. The electric field intensity, developed due to it, at an external point P is to be determined. The distance of P from the conductor is much less than the dimensions of the conducting body.

Now, we consider a right circular cylinder of cross-sectional area dS, normal to the surface of the conductor, one of whose flat circular faces contains the point P.

The surface of this cylinder serves as the Gaussian surface in this case. We assume that the cross-sectional area dS is small enough that E is constant over the flat circular face. From symmetry, tire electric field intensity Is normally outwards with respect to the surface dS.

Thus, electric flux across the flat circular face around P is Eds.

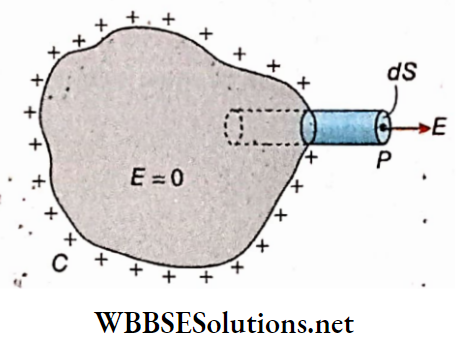
The curved surface of the cylinder does not contribute to any electric flux, since the direction of E is parallel to the curved surface at every point (i.e., the component of E along the normal to the curved surface at every point is zero). Also, the flat circular face inside the conducting body has no contribution, because the electric field inside a conductor is zero.

The entire charge resides on the outer surface of the conductor. In general, the curvature is not uniform around the surface. As a result, the charge would be non-uniformly distributed.

Let +cr be the surface charge density on a small area of dS on the surface just in front of P.

Now, since the cylinder encloses a number of charge σ dS, by Gauss’ theorem, electric flux linked with the Gaussian cylinder

= \(\frac{\sigma d S}{\epsilon}\)

∴ \(E d S=\frac{\sigma d S}{\epsilon} \quad \text { or, } E=\frac{\sigma}{\epsilon}\)

In vector form, \(\vec{E}=\frac{\sigma}{\epsilon_0} \hat{n}\)

where \(\hat{n}\) is the unit vector normal to the surface in the outward direction.

Electrostatics

Electric Field Numerical Example

Example 1. An electric field \((2 \hat{i}+3 \hat{j}) \mathrm{N} \cdot \mathrm{C}^{-1}\) exists In a region. Calculate the electric flux linked with a square plate of side 0.5 m held parallel to

1. yz -plane
2. xy -plane.

Solution:

Electric field, \(\vec{E}=2 \hat{i}+3 \hat{j}\); so it lies on the xy -plane. Area of the square plate, S = (0.5)² = 0.25 m²

1. The vector representation of the plate parallel to the yz -plane is, \(\vec{S}=S \hat{i}=0.25 \hat{i}\)

∴ Electric flux linked with the square plate

⇒ \(\vec{E} \cdot \vec{S}=(2 \hat{i}+3 \hat{j}) \cdot(0.25 \hat{i})\)

= 0.5 N.m².C^-1.

2. The vector representation of the plate parallel to the xy -plane is, \(\vec{S}=S \hat{k}=0.25 \hat{k}\)

∴ Electric flux linked with the square plate

⇒ \(\vec{E} \cdot \vec{S}=(2 \hat{i}+3 \hat{j}) \cdot(0.25 \hat{k})\)

= 0.

Example 2. In vacuum,

1. Find out the electric flux across, an area \(\vec{s}=10 \hat{j}\) placed in an electric flux \(\vec{E}=2 \hat{i}+4 \hat{j}+7 \hat{k}\)
2. How much electricity? charges are to be placed at a point such that 4400 electric lines of force will emerge from that point.
3. What will be the electric flux through any one of the faces of a cube of 10 cm if a charge of 1 eus is placed at the center of the cube?

Solution:

1. Electric flux, \(\phi=\vec{E} \cdot \vec{S}=(2 \hat{i}+4 \hat{j}+7 \hat{k}) \cdot 10 j\)

= 40 unit

2. In the CGS system, according to Gauss’ theorem, in a vacuum the net electric flux linked with a closed surface is 4 TC times the net charge within the surface.

∴ 4400 = 4πq

or, \(q=\frac{4400}{4 \pi}=\frac{4400 \times 7}{4 \times 22}\)

= 350 esu

3. Net electric flux = 4πq

= 4π.1

= 4n esu

As a cube has 6 faces, the electric flux linked with each face of the cube = \(\frac{1}{6} \times 4 \pi=\frac{2}{3} \pi \text { esu }\)

Example 3. An electric flux of 6.5 x 10³ N.m².C^-2 is linked with a sphere due to some charge placed In a vacuum Inside the sphere. Calculate the magnitude of the charge
Solution:

In this case, total flux linked = 6.5 x 10³ N.m².C^-1

According to Gauss’ theorem,

total flux linked with the sphere = \(\frac{1}{\epsilon_0} \cdot q\)

∴ \(\frac{1}{\epsilon_0} \cdot q=6.5 \times 10^3\)

or, q = 6.5 x 10³ x ∈0

= 6.5 x 10³ x 8.85 x 10^-12

= 5.75 X 10^-8 C.

Example 4. A spherical shell of radius 20cm has a 20μC charge placed in a vacuum. Calculate the electric field Intensity

1. At a distance of 15 cm
2. At a distance of 40 cm from the centre of the spherical shell

Solution:

1. Electric field intensity at a distance of 15 cm from the centre of the spherical shell = 0; because charge does not reside Inside a charged spherical shell.

2. Electric field Intensity at a distance of 40 cm or 0.4 m from the centre of the spherical shell

⇒ \(E=\frac{1}{4 \pi \epsilon_0} \cdot \frac{q}{x^2}=\frac{9 \times 10^9 \times 20 \times 10^{-6}}{(0.4)^2}\)

= 1.125 X 10⁶ N.C^-1

Example 5. A thin straight wire of length 40 cm placed in a vacuum has a 20μC charge. Calculate the electric field intensity at a distance of 15 cm from the wire.
Solution:

⇒ \(E=\frac{1}{4 \pi \epsilon_0} \cdot \frac{2 \lambda}{x}\) [ λ = charge per unit length of the wire = \(\frac{20 \times 10^{-6}}{40 \times 10^{-2}} \mathrm{C} \cdot \mathrm{m}^{-1}\) ]

= \(\frac{9 \times 10^9 \times 2 \times 20 \times 10^{-6}}{15 \times 10^{-2} \times 40 \times 10^{-2}}\)

= 6 x 10⁶ N C^-1

Example 6. A cube placed in a vacuum contains a charge of 9 x 10^-9 C. What will be the electric flux linked with each face of the cube?
Answer:

Electric flux linked with the 6 faces of the cube = \(\frac{q}{\epsilon_0}\) Due to symmetry, this flux is equally shared among the 6 faces. So, the electric flux linked with each face of the cube

⇒ \(\frac{1}{6} \cdot \frac{q}{\epsilon_0}=\frac{1}{6} \times \frac{9 \times 10^{-9}}{8.854 \times 10^{-12}}\)

= 169.41V.m

Example 7. An electric field is expressed as \(\vec{E}=(5 \hat{i}+3 \hat{j}+2 \hat{k})\) unit. Find out the electric flux across an area of 200 units on the yz-plane in that field.
Solution:

The yz-plane is perpendicular to the x-axis.

∴ The given area vector is \(\vec{S}=200 \hat{i} \text { unit. }\)

Then, the electric flux across that area,

⇒ \(\phi=\vec{E} \cdot \vec{S}=(5 \hat{i}+3 \hat{j}+2 \hat{k}) \cdot 200 \hat{i}\)

= 1000 units.

Example 8. The surface density of charge on a large, vertical positively charged plate Is σ C.m^-2. A string attaches a metal ball of mass M and charges +q with the plate. Find out the angle between the string and the plate In equilibrium
Solution:

Let the angle between the string and the plate in equilibrium = θ

Tension in the string = T;

The electric field at the position of the ball = E

= \(\frac{\sigma}{2 \epsilon_0}\)

In equilibrium,

⇒ \(T \sin \theta=q E=\frac{q \sigma}{2 \epsilon_0} ; T \cos \theta=M g\)

∴ \(\tan \theta=\frac{q \sigma}{2 \epsilon_0 M g}\)

or, \(\theta=\tan ^{-1}\left(\frac{q \sigma}{2 \epsilon_0 M g}\right)\)

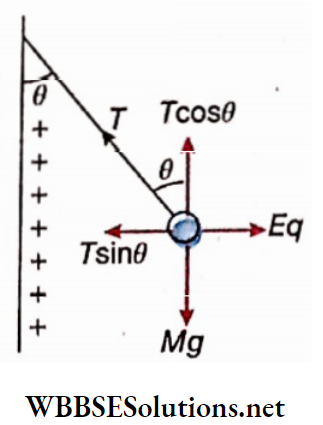
Example 9. The classical concept of atomic structure- is that negative charges are uniformly distributed inside a sphere of radius R keeping the nucleus of positive charge Ze at the centre of that sphere. An atom as a whole is electrically neutral. Find out the electric field at a distance r from the nucleus, according to this atomic model.
Solution:

Charge of the nucleus = +Ze.

∴ Amount of charge outside the nucleus = -Ze, as an atom is electrically neutral.

Volume density of negative charge inside the sphere,

⇒ \(\rho=\frac{-Z e}{\frac{4}{3} \pi R^3}=-\frac{3 Z e}{4 \pi R^3}\)

The electric field at external points (r > R):

Let A be any such point outside the sphere. A concentric sphere of radius r, passing through A, is a convenient Gaussian surface. The charge enclosed by this Gaussian surface, q = charge of the nucleus + charge distributed inside the sphere of radius R

= +Ze +(-Ze)

= 0

∴ From Gauss’ theorem, \(\oint_S \vec{E} \cdot d \vec{S}=\frac{q}{\epsilon_0}=0\)

∴ E = 0, at all external points.

The electric field at Internal points (r<R): Let B be any such point inside the sphere. A concentric sphere of radius r, passing through B is a convenient Gauislan surface.

It is to be noted that this surface encloses the entire positive charge +Ze of the nucleus, but a part of the negative charge -Ze remains outside it The amount of negative charge inside this Gaussian surface,

q’ = volume of this endorsed sphere x volume density p of negative charge

⇒ \(\frac{4}{3} \pi r^3\left(\frac{3 Z e}{4 \pi R^3}\right)=-Z e \frac{r^3}{R^3}\)

∴ Net charge enclosed by the sphere,

⇒ \(q=+Z e+q^{\prime}=Z e-Z e \frac{r^3}{R^3}=Z e\left(1-\frac{r^3}{R^3}\right)\)

For any surface element d\(\vec{S}\) on the Gaussian surface, the electric field \(\vec{E}\) and the area vector d\(\vec{S}\) are parallel to each other.

∴ \(\vec{E} \cdot d \vec{S}=E d S \cos 0^{\circ}=E d S\)

Again, from symmetry, £ is uniform in magnitude over the Gaussian surface. Then, from Gauss’ theorem,

⇒ \(\oint_S \vec{E} \cdot d \vec{S}=\frac{q}{\epsilon_0}\)

or, \(\oint_S E d S=\frac{q}{\epsilon_0} \text { or, } E \oint_S d S=\frac{q}{\epsilon_0} \quad \text { or, } E S=\frac{q}{\epsilon_0}\)

or, \(E \cdot 4 \pi r^2=\frac{1}{\epsilon_0} Z e\left(1-\frac{r^3}{R^3}\right)\)

or, \(E=\frac{Z e}{4 \pi \epsilon_0} \cdot \frac{1}{r^2}\left(1-\frac{r^3}{R^3}\right)=\frac{Z e}{4 \pi \epsilon_0}\left(\frac{1}{r^2}-\frac{r}{R^3}\right)\)

Example 10. A uniform electric field along the x-axis is given as,

⇒ \(\begin{aligned}
\vec{E} & =(200 \hat{i}) \mathrm{N} \cdot \mathrm{C}^{-1}, \text { for } x>0 \\
& =(-200 \hat{l}) \mathrm{N} \cdot \mathrm{C}^{-1}, \text { for } x<0
\end{aligned}\)

A cylinder of length 20 cm and radius 5 cm has Its center at the origin and axis along the x-axis and is placed in a vacuum. Find out

1. The electric flux across each of its circular faces,
2. The flux across hs curved surface,
3. The flux across its entire outer surface and
4. The net charge enclosed by it,

Solution:

Radius of the cylinder, r = 5 cm = 0.05 m

∴ Area of each circular face,

S = πr²

= 3.14 x (0.05)² m²

As the length of the cylinder is 20 cm or 0.2 m, the two circular faces are at x = +0.1 m and x = -0.1 m.

The area vectors representing the right and the left circular faces \(\vec{S}_1=\hat{i}\left(\pi r^2\right) \text { and } \vec{S}_2=-\hat{i}\left(\pi r^2\right)\) respectively.

The electric fields at the positions of these circular faces are, \(\) respectively

1. The electric flux across the right circular face,

⇒ \(\vec{E}_1=(200 \hat{i}) \mathrm{N} \cdot \mathrm{C}^{-1} \text { and } \vec{E}_2=(-200 \hat{i}) \mathrm{N} \cdot \mathrm{C}^{-1}\)

= 200 x 3.14 x (0.05)²

= 1.57 N.m².C^-1

Similarly, for the left circular face,

⇒ \(\phi_1=\vec{E}_1 \cdot \vec{S}_1=(200 \hat{i}) \cdot \hat{i}\left(\pi r^2\right)\)

= 200 x 3.14 X (0.05)²

= 1.57 N.m² – C^-1

∴ \(\phi_1=\phi_2\) i.e., equal flux passes across each of the two faces.

2. The curved surface is everywhere parallel to the electric field vector.

∴ The electric flux linked with the curved surface = 0

3. The entire outer surface consists of two circular faces and a curved surface. So, the flux linked with the entire surface,

∴ \(\phi=\phi_1+\phi_2+0=1.57+1.57+0\)

= 3.14 N.m².C^-1

4. FromchargeGauss’is, theorem, net flux, \(\phi=\frac{q}{\epsilon_0}\). So the endorsed closed is,

q = \(\phi \epsilon_0\) = 3.14 x 8.854 x 10^-12

= 2.78×10^-11C

Electrostatics

Electric Field Synopsis

• Coulomb’s law: The force of attraction or repulsion between two point charges is proportional to the product of the charges and inversely proportional to the square of the distance between them.
• SI unit of charge: If an electric repulsive force of 9 x 10⁹ N arises between two similar electric charges lm apart in air (or vacuum), the amount of each charge is 1C.

Units of charge in different unit systems:

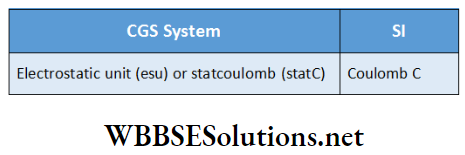
1 coulomb = 3 x 10⁹ esu of charge.

• The dielectric constant of a medium (k) is defined as the ratio of the permittivity of the medium (∈) to that of vacuum (∈₀). It is also known as relative permittivity.
  So, \(\kappa=\frac{\epsilon}{\epsilon_0}\)
• The electric field due to a charge distribution is the region in which its effect is felt. Any other charge, placed at any point in that region, experiences a force due to the original charge distribution.
• The intensity or strength of an electric field at any point in the field is the force experienced by a unit of positive charge placed at that point.

Unit of intensity of electric field:

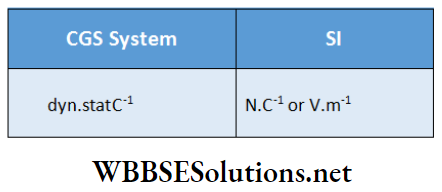
1 dyn statC^-1 = 3 x 10⁴ N.C^-1

• If the resultant intensity of the electric field at any point due to two or more charges is zero, then that point is called a neutral point.
• An electric dipole consists of a combination of two equal and opposite point charges separated by a very small distance.

Unit of dipole moment:

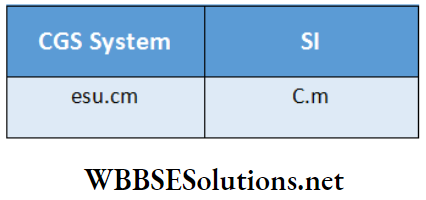
1 C.m = 3 x 10º esu.cm

• The electric dipole moment of an electric dipole is defined as the torque acting on it when it is placed at right angles to a uniform electric field of unit strength.
• The substances that have no free electrons cannot conduct electricity. They are called insulators or dielectrics.

According to the position of charge in the molecule of a dielectric, it is classified into two groups:

1. Nonpolar substance

2. Polar substance.

• The phenomenon of alignment of the molecules, which behave as electric dipoles, under the influence of an external electric field, is called electric polarisation.
• An electric field line is an imaginary line in an electric field along which a free and isolated positive charge would tend to move. A tangent at any point on the line gives the direction of the electric field at that point.
• The magnitude of the electric field intensity at any point is equal to the number of field lines passing normally through a unit surface area around that point.
• Electric flux is the number of field lines passing normally through a surface.

Unit of electric flux:

• SI: N.m².C^-1 or V m

Gauss’ theorem:

• The net electric flux linked with a closed surface is \(\frac{1}{\epsilon}\) times the total charge within the surface.

Electrostatics

Electric Field Useful Relations for Solving Numerical Problems

• The electrostatic force between two point charges,
  ⇒ \(F=\frac{q_1 q_2}{r^2}\) (In CGS system);
  ⇒ \(F=\frac{q_1 q_2}{4 \pi \epsilon_0 r^2}\)
  where e0 = permittivity of air or vacuum.
• Relation between K and e:
  ⇒ \(\kappa=\frac{\epsilon}{\epsilon_0}\) [where e0 = permittivity of vacuum (or air)
  = 8.854 x 10^-12 C².N^-1.m^-2.
• The electric intensity of a point at distance r from o point charge q,
  ⇒ \(E=\frac{1}{4 \pi \alpha^{\prime} C_0} \cdot \frac{q}{r^2}(\ln \mathrm{SI})\)
• Relation between electrostatic force and electric intensity,
  F = qE
• Electric dipole moment, p = 2lq, where, 2l = length of the dipole, q = charge of dipole It is a vector quantity defined as \(\), where \(\vec{l}\) is directed from the negative to the positive charge of the dipole.
• Electric field intensity at a point on the axis of a dipole,
  ⇒ \(E=\frac{2 p r}{4 \pi k \epsilon_0\left(r^2-l^2\right)^2}(\text { in SI })\)
  When \(r \gg l, E=\frac{1}{4 \pi \kappa \epsilon_0} \cdot \frac{2 p}{r^3}\);
• Electric field intensity at a point on the perpendicular bisector of a dipole
  ⇒ \(E=\frac{p}{4 \pi \kappa \epsilon_0\left(r^2+l^2\right)^{3 / 2}} \text { (in SI) }\)
  When \(r \gg l, E=\frac{1}{4 \pi \kappa \epsilon_0} \cdot \frac{p}{r^3} \text { (in SI) }\)
• Electric field intensity at any point due to an electric dipole,
  ⇒ \(E=\frac{1}{4 \pi \kappa \epsilon_0} \cdot \frac{p}{r^3} \sqrt{3 \cos ^2 \theta+1}(\text { in SI })\)
• The torque acting on an electric dipole inclined at an angle 0 with a uniform electric field, \(\tau=p E \sin \theta\)
• Electric flux through a surface d\(\vec{S}\) in an electric field of intensity \(\vec{E}\) is,
  ⇒ \(\phi=\int_S \vec{E} \cdot d \vec{S}\)
• According lo Gauss’ theorem, total electric flux linked with a closed surface is given by,
  ⇒ \(\oint_S \vec{E} \cdot d \vec{S}=\frac{q}{\epsilon} \text { (in SI) }\)
  where q is the charge enclosed by the closed surface and e is the permittivity of the medium.
• The electric field intensity at a point due to a point charge.
  ⇒ \(E=\frac{q}{4 \pi \epsilon r^2}(\text { in SI })\)
• The electric field intensity due to a uniformly charged thin spherical shell at a point outside the shell,
  ⇒ \(E=\frac{1}{4 \pi \epsilon} \cdot \frac{2 \lambda}{r}(\text { in SI })\)
• The electric field intensity at a point due to an infinitely long straight charged conducting wire,
  ⇒ \(E=\frac{\sigma}{2 \epsilon} \text { (in SI) }\) [where, λ = linear charge density of the wire]
• The electric field intensity at a point due to an infinite non-conducting uniformly charged plane lamina,
  ⇒ \(E=\frac{\sigma}{2 \epsilon} \text { (in SI) }\)
• The intensity of the electric field at a point very close to a charged conductor having a surface density of charge cr
  ⇒ \(E=\frac{\sigma}{\epsilon}(\text { in SI })\)

Electrostatics

Electric Field Very Short Answer Type Questions

Question 1. The force of interaction between two charges placed in vacuum is F. What will be the force between the charges placed at the same distance in a medium of dielectric constant k?
Answer: F/k

Question 2. What is the relation of esu of charge with coulomb?
Answer: 1C = 2 x 10⁹ esu of charge

Question 3. If 1μC = x esu of charge, what is the value of x?
Answer: 3 x 10^-3

Question 4. What is the order of the ratio of the gravitational force and the electrical force acting between two electrons?
Answer: 10⁴³

Question 5. What is the electric field intensity at a distance r from a charge q placed in a vacuum?
Answer: q/r²

Question 6. What is the unit of the intensity of the electric field in SI?
Answer: N.C^-1

Question 7. Which physical quantity has the unit V.m^-1 ? placed in a uniform electric field?
Answer: Intensity of the electric field

Question 8. Two point charges e₁ and e₂ are placed at a distance d from each other. In between them, there is no point where the electric field is zero. From this what conclusion can you
Answer: e₁ and e₂ are oppositely charged

Question 9. What is the intensity of the electric field at the centre of a
Answer: Zero

Question 10. The direction of electrostatic field intensity at a point on the surface of a charged conductor is along the tangent drawn on the surface at that point. Is the statement true or false?
Answer: False

Question 11. If a conductor is placed in an electric field, what change of the intensity of the electric field takes place inside the
Answer: Electric field intensity becomes zero

Question 12. what is the ratio of the intensity of the electric field at a particular distance on the axis to that on the perpendicular bisector of a very small dipole?
Answer: 2:1

Question 13. How should an electric dipole be kept in an electric field so that no torque acts on it?
Answer: If it remains parallel to the electric field

Question 14. is the dipole moment a scalar or a vector quantity?
Answer: Vector quantity

Question 15. What is the unit of dipole moment in SI?
Answer: C.m

Question 16. An electric dipole is inclined with a uniform electric field. State whether a force or a torque acts on the dipole. charge q placed in a vacuum?
Answer: Torque

Question 17. What is the resultant electric force on an electric dipole placed in a uniform electric field?
Answer: 0

Question 18. What is the dipole moment of a non-polar molecule?
Answer: 0

Question 19. If electric flux liked with a surface is maximum, what will be the angle of the field lines with the normal to the surface?
Answer: 0

Question 20. A square plate of side a is placed perpendicular to a uniform electric field of intensity E. What will be the flux linked with the plate?
Answer: Ea²

Question 21. What is the intensity of the electric field inside a uniformly charged spherical shell?
Answer: 0

Electrostatics

Electric Field Fill In The Blanks

1. Two point charges each equal to 1C exert a force 9 x 10⁹N mutually when they are placed 1 m apart in air

2. The electric field intensity at all points in a uniform electric field is equal

3. The field lines in a uniform electric field are equidistant parallel lines.

4. Three small spheres are placed on the circumference of a circle of radius r in such a way that an equilateral triangle is formed. If the charge of each sphere is q, the electric field intensity at the center of the circle will be zero

5. A few field lines moving from the left converge on the right side. The electric field intensity will be greater at the right side

6. The field fines of an isolated negative charge are radially inwards

7. The torque acting on an electric dipole placed in a uniform electric field is maximum when the dipole is perpendicular to the direction of the electric field.

8. An electric dipole of moment p is placed in an equilibrium position in a uniform electric field of intensity E. To rotate the dipole through an angle 6 from its initial position, the required moment of the torque is pE sinθ

9. If a charge exists outside a closed surface, the net electric flux linked with the closed surface will be zero

10. The direction of the area vector is perpendicular to the area

11. The flux linked with a surface perpendicular to the field lines in an electric field will be the maximum

12. According to Gauss’ theorem in the CGS system, the total electric flux linked with a closed surface is equal to 4π/k times the total charge lying within the surface.

13. Electric flux is a scalar quantity.

Electrostatics

Electric Field Assertion-Reason Type

Direction: These questions have statement 1 and statement 2. Of the four choices given below, choose the one that best describes the two statements.

1. Statement 1 is true, statement 2 is true; statement 2 is a correct explanation for statement 1.
2. Statement 1 is true, and statement 2 is true; statement 2 is not a correct explanation for statement 1.
3. Statement 1 is true, statement 2 is false.
4. Statement 1 is false, and statement 2 is true.

Question 1.

Statement 1: If a proton and an electron are placed in the same uniform electric field, they experience different accelerations.

Statement 2: Electric force on a test charge is independent of its mass.

Answer: 2. Statement 1 is true, and statement 2 is true; statement 2 is not a correct explanation for statement 1.

Question 2.

Statement 1: Electric dipole moment is a vector quantity.

Statement 2: The direction of electric dipole moment is from negative charge to the positive charge.

Answer: 1. Statement 1 is true, statement 2 is true; statement 2 is a correct explanation for statement 1.

Question 3.

Statement I: When an electric dipole is perpendicular to an electric field, maximum torque acts on it

Statement II : If 6 = 90°, then torque, r = pEsin90° = pE.

Answer: 1. Statement 1 is true, statement 2 is true; statement 2 is a correct explanation for statement 1.

Question 4.

Statement 1: A free electron suffers the same acceleration as a free proton when placed in a uniform field.

Statement 2: An electron and a proton have the same quantity of charge.

Answer: 4. Statement 1 is false, and statement 2 is true.

Question 5.

Statement 1: The electric field due to a dipole on its axial line at a distance r is E. The electric field due to the same dipole on the equatorial line at the same distance will be \(\frac{E}{2}\)

Statement 2: Electric field due to a dipole at a point varies inversely with distance.

Answer: 3. Statement 1 is true, statement 2 is false.

Electrostatics

Electric Field Match The Columns

Question 1. Some physical quantities are given in column I and their corresponding dimensions are given in column II

Answer: 1-C, 2-D, 3-A, 4-B

Question 2.

Answer: 1-C, 2-A, 3-D, 4-B

Question 3. Some physical quantities are given in column I and their corresponding dimensions are given in column II

Answer: 1-B, 2-D, 3-A, 4-C

Question 4. Charge inside the different types of closed surfaces are
given in column I and their corresponding values of flux
are given in column II.

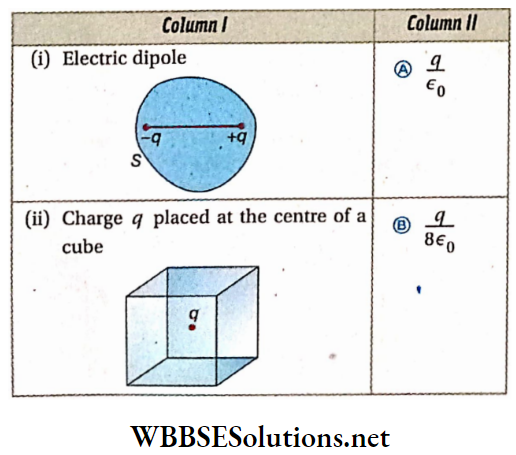
Answer: 1-D, 2-A, 3-B, 4-C, 5-D