WBCHSE Class 12 Physics Atom Question And Answers

Atom Long Question And Answers

Question 1. How many times does the electron— revolve in the first Bohr orbit of a hydrogen atom per second?
Answer:

The radius of the first Bohr orbit,

r₁ = 0.53 Å = 0.53 × 10^-8 cm

Again, the velocity of the electron in the first Bohr orbit

ν₁  = \(\frac{c}{137}=\frac{3 \times 10^{10}}{137} \mathrm{~cm} \cdot \mathrm{s}^{-1}\)

c = velocity of light in vacuum

So the electron revolutions of the electron in the first Bohr orbit in 1 second i.e the orbital frequency of the electron

f ₁ = \(\frac{{v}_1}{2 \pi r_1}=\frac{3 \times 10^{10}}{1.37 \times 2 \times \pi \times 0.53 \times 10^{-13}}\)

= 6.67 × 10 ^-27 s ^-1

Question 2. In a hydrogen atom, how many limes Is the energy of the electron In the fourth orbit compared to that In the second orbit?
Answer:

If the principal quantum of an orbit is n, the total energy

Hence, the ratio of light energies of electrons In the orbits is \(1: \frac{1}{4}: \frac{1}{9}: \frac{1}{16}\)

So, \(\frac{\text { energy of electron In the fourth orbit }}{\text { energy of electron In the second orbit }}=\frac{\frac{1}{16}}{\frac{1}{4}}\)

= \(\frac{1}{4}\)

Therefore, the energy of the electron In the fourth orbit is part of the energy of the electron In the second orbit

Question 3. The electron In a hydrogen atom makes a transition n₁ →n₂ -, where n₁ and n₂ are the principal quantum numbers of the two states. According to the Bohr model, the period of the electron In the initial stale Is eight times that In the final state. Which are possible values of n₁ and n₂?

1. 4,2
2. 8,2
3. 8,1
4. 6,3

Answer:

If the orbital frequency of the electron in the n-th orbit is f_n, then

f_n ∝ 1/n³

So, time period is (1/f) = \(T_n \propto n^3 \quad \text { or, } n \propto\left(T_n\right)^{1 / 3}\)

In the given question,

T₁  =  8T₂ = T₁ / T₂

= 8

∴ \(\frac{n_1}{n_2}=\left(\frac{T_1}{T_2}\right)^{1 / 3}\)

= (8)^1/3

= 2

n₁ = 2n₂

So, for the given values of n₁ and n₂, either (1) Or (4) are (4,2) Or ( 6,3)  is possible.

Question 4. An electron, in a hydrogen-like atom, is in an excited state. It has a total energy of -3.4 eV. Calculate the kinetic energy of the electron
Answer:

According to the Bohr model of the atom, when the total energy of an electron is -E, its kinetic energy = +E. So, in this case, the kinetic energy of the electron = 3.4 eV.

Question 4. As per the Bohr model, the minimum energy (In eV) required to remove an electron from the ground state of Li²⁺ Ion (Z = 3) Is:

1. 1.51
2. 13.6
3. 40.8
4. 122.4 which one is correct?

Answer:

In a hydrogen atom, the force of attraction (in CGS sys¬ tem) between the nucleus having charge +e and electron of charge -e at a distance r is e²/r²

Again, the ground state energy of the hydrogen atom,

E₁ = \(-\frac{2 \pi^2 m e^4}{h^2}\)

= -13.6 eV

In Li2+ ion, there is also one electron; the force of attraction between the nucleus having charge +Ze and the electron having charge’-e is  \(\frac{Z e^2}{r^2}\)

So, by putting Ze² in place of e² in the expression for the ground-state energy of the hydrogen atom, the ground-state energy of Li²⁺ can be obtained. This energy is

⇒ \(E_1^{\prime}=-\frac{2 \pi^2 m\left(Z e^2\right)^2}{h^2}=-\frac{2 \pi^2 m e^4}{h^2} \cdot Z^2\)

– 136 × 3² = -1224 eV

Question 5. The spectrum of sodium atoms resembles the spectrum of hydrogen atoms. In which way is this statement correct?
Answer:

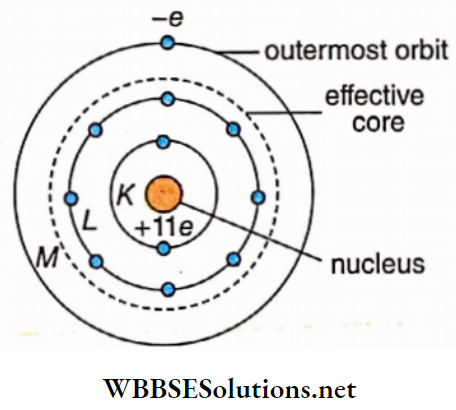
The charge of sodium (Z = 11) nucleus =+lle. There are 2 and 8 electrons, respectively in its K and L shells and there is only one electron in the outermost M shell. The nucleus, the K-orbit, and the L -orbit in this atom, all together form an effective core, and the electron in the Af-orbit revolves around this core. Moreover, the charge of the core = lie- (2 + 8)e = +e = charge of H-nucleus

So, this electron in the M-orbit resembles the electron revolving around the nucleus in a hydrogen atom. Thus, the spectrum of sodium atoms resembles the spectrum of hydrogen atoms.

Question 6. Determine the ratios of

1.  Time Periods and
2. Orbital frequencies of an electron in Bohr orbits of a hydrogen atom

Answer:

1.  Time Period:

T_n = \(=\frac{\text { circumference of the orbit }}{\text { velocity of electron }}\)

= \(\frac{2 \pi r_n}{v_n}\)

If the principal quantum number be n(- 1, 2, 3, ……………….. ) then

= \(r_n \propto n^2 \text { and } v_n \propto \frac{1}{n}\)

So, \(T_n \propto n^3 \text {, i.e., } T_1: T_2: T_3: \cdots=1: 8: 27\)

2. Orbital frequency, \(f_n=\frac{1}{T_n}\)

So, \(f_1: f_2: f_3: \cdots=1: \frac{1}{8}: \frac{1}{27}\)

Question 7. The wavelength of the K_α line of the X-ray of an element having atomic number Z = 11 is λ. For which atomic number will the wavelength of that line be 4 λ?
Answer:

Frequency f = \(\frac{c}{\lambda}\)

Or, \(\frac{f_1}{f_2}=\frac{\lambda_2}{\lambda_1}=\frac{4 \lambda}{\lambda}\)

= 4

According to Moseley’s law, (Z- 1)

∴ \(\frac{f_1}{f_2}=\left(\frac{Z_1-1}{Z_2-1}\right)^2\)

Or, \(\left(\frac{Z_1-1}{Z_2-1}\right)^2\) = 4

Here Z = 11

So, \(\left(Z_2-1\right)^2=\frac{1}{4} \times(11-1)^2=\frac{1}{4} \times 100\)

= 25

Z₂-1 = 5

Or, Z₂ = 6

Question 8. On which power of the principal quantum number (n) of the orbit, will the magnetic moment (μ) of an electron revolving in the Bohr orbit of an atom be directly proportional
Answer:

Due to the rotation of electrons in the n-th orbit, effective current, \(I_n=\frac{e}{T_n}\)

Now, time period, T_n∝ n³ ; hence \(I_n \propto \frac{1}{n^3}\)

Again, the area of the n-th orbit, A_n = π

Since, r_n ∝ n²  , A_n « ∝ n⁴

The magnetic moment of an electron in the n-th orbit,

So, \(\mu_n=I_n A_n\)

⇒ \(\mu_n \propto \frac{1}{n^3} \cdot n^4\)

Question 9. The three ascending energy states of an atom are A, B, and C. The wavelengths of emitted radiations due to transitions from C to B and from B to λ₁ are λ₂, respectively. What will be the wavelength of emitted radiation due to the transition from C to A?
Answer:

⇒ \(E_C-E_B=\frac{h c}{\lambda_1}\)

⇒ \(E_B-E_A=\frac{h c}{\lambda_2}\)

Now, if the wavelength of the radiation due to transition from C to A is λ, then

⇒ \(E_C-E_A=\frac{h c}{\lambda}\)

Or, \(\left(E_C-E_B\right)+\left(E_B-E_A\right)=\frac{h c}{\lambda}\)

⇒  \(\frac{h c}{\lambda} \text { or, } \frac{h c}{\lambda_1}+\frac{h c}{\lambda_2}=\frac{h c}{\lambda}\)

⇒  \(\frac{1}{\lambda}=\frac{1}{\lambda_1}+\frac{1}{\lambda_2}\)

Or, \(\frac{\lambda_1 \lambda_2}{\lambda_1+\lambda_2}\)

Question 10. Obtain the first Bohr radius and the ground state energy of a muonic hydrogen atom (i.e., an atom in which negatively charged muon (μ^–) of mass about 207m_e orbits around a proton).
Answer:

According to the question, the mass of muon.

m_m = 207 m_e

When all other quantities remain unchanged

⇒ \(r \propto \frac{n^2}{m} \text { and } E \propto \frac{m}{n^2}\)

⇒ \(r_m=\frac{r_e m_e}{m_m}=\frac{0.53 \times 10^3}{207}\)

= 2.56 × 10^-13 m

And \(E_m=\frac{E_e m_m}{m_e}\)

= -(13.6 ×  207)

= -3.8 keV

Question 11. The energy of a hydrogen atom In n given orbit In -3.4 cV. bind the radius of the orbit. (Given,e =  -1.610^-19 C, m_e = 9.1110^-31 kg , \(\frac{h}{2 \pi}=1.055 \times 10^{-34}\), \(\frac{1}{4 \pi c_0}=9 \times 10^9 \mathrm{~N} \cdot \mathrm{m}^2 \cdot \mathrm{C}^{-2} \mathrm{~J}\)
Answer:

We know, \(E_n \propto \frac{1}{n^2}\)

⇒ \(\frac{E_1}{E_2}=\frac{n_2^2}{n_1^2}\)

⇒  \(\frac{-13.6}{-3.4}=\frac{n_2^2}{1}\)

n₂ = 2

Again ,  \(r_n=\frac{\epsilon_0 n^2 h^2}{\pi m e^2}\)

= \(\frac{8.85 \times 10^{-12} \times 4 \times\left(6.63 \times 10^{-34}\right)^2}{\pi \times 9.11 \times 10^{-31} \times\left(1.6 \times 10^{-19}\right)^2}\)

= \(\frac{1.56 \times 10^{-77}}{7.33 \times 10^{-68}}\)

= 2.13

Question 12. The energy of an excited hydrogen atom is -1.51 eV. Determine the angular momentum of the electron according to Bohr’s hypothesis.
Answer: 

Energy In n = 1 state, E₁ = -13.6 eV

Let the required energy state be n and energy in that state

E_n = -1.51 eV

∴ \(E_n=\frac{E_1}{n^2}\)

Or, -1.51 = \(-\frac{13.6}{n^2}\)

or , n = 3

Now angular momentum according to postulates of Bohr’s theory,

⇒ \(\frac{n h}{2 \pi}=\frac{3 \times 6.63 \times 10^{-34}}{2 \times 3.14}\)

= 3.17 × 10^-34 J.s

Question 13. The voltage applied across the cathode and anode of an X-ray generating machine is 50000V. Determine the shortest wavelength of the X-ray emitted. Given h = 6.62 × 10^-34 J.s 
Answer:

ev = \(h \nu_{\max }=\frac{h c}{\lambda_{\min }}\)

ν_max  and ν_min  are respectively maximum frequency and minimum wavelength of X-ray photon]

∴ \(\frac{h c}{e V}=\frac{\left(6.62 \times 10^{-34}\right) \times\left(3 \times 10^8\right)}{\left(1.6 \times 10^{-19}\right) \times 50000}\)

= 2.48 × 10^-11 m

= 0.248 Å

Question 14. What will be the wavelength of die light emitted due to a transition of election from n = 3 orbit to n = 2 orbit in a hydrogen atom? Given: the Rydberg constant for the hydrogen atom is Rn = 1.09 × 10⁷ m^-1
Answer:

⇒ \(\frac{1}{\lambda}=R\left(\frac{1}{2^2}-\frac{1}{3^2}\right)\)

⇒  \(1.09 \times 10^7 \times \frac{5}{36}\)

λ =  \(\frac{36 \times 10^{-7}}{1.09 \times 5}\)

= 6.606 m × 10⁷ m

= 6606 Å

Question 15. The ground state energy of the hydrogen atom is -13.6 eV. What are the kinetic and potential energies of the electron in this state?
Answer:

If E_k and E_p are the kinetic and potential energies respectively, total energy, E = E_k + E_p

For any state of a hydrogen atom,

E_p = -2E_k and E =  E_k-2E_k = -E_k

or, E_k = -E

∴ For the ground state

E_k = -E = +13.6 eV

E_p = -2E_k

= -2 × 13.6

= 27.2 ev

Question 16. The ground state energy of the hydrogen atom is -13.6 eV. If an electron makes a transition from an energy level -0.85 eV to -3.4 eV, calculate the wavelength of the spectral line emitted. To which series of hydrogen spectrum does this wavelength belong?
Answer:

Given, E₁= -13.6 eV

Now \(\frac{-13.6}{-0.85}\)

And \(\frac{-13.6}{-3.4}\)

= 4

As , \(E \propto \frac{1}{n^2}\) the two given levels correspond to n = 4 and n = 2 , respectively.

The spectral line emitted due to 4 → 2 transition belongs to the Balmer series.

Photon energy due to this transition is,

hf = hc/λ = -0.85 – (-3.4)

= 2.55 eV

λ = \(\frac{h c}{2.55 \mathrm{eV}}=\frac{\left(6.626 \times 10^{-34}\right) \times\left(3 \times 10^8\right)}{2.55 \times 1.6 \times 10^{-9}} \mathrm{n}\)

= \(\frac{\left(6.626 \times 10^{-34}\right) \times\left(3 \times 10^8\right)}{2.55 \times 1.6 \times 10^{-19}} \times 10^{10}\) Å

= 4872 Å

Question 17. Using Bohr’s postulates, derive the expression for the frequency of radiation emitted when an electron in a hydrogen atom undergoes a transition from a higher energy state (quantum number n_i) to a lower state (n_f). When an electron in a hydrogen atom jumps from energy state n_i = 4 to n_f =  3, 2,1, identify the spectral series to which the emission lines belong.
Answer:

4 → 1 transition: The emission line belongs to the Lyman series.

4 → 2 transition: The emission line belongs to the Balmer series.

4 → 3 transition: The emission line belongs to the Paschen series.

Question 18. Using Rutherford’s model of the atom, derive the expression for the total energy of the electron in the hydrogen atom. What is the significance of total negative energy possessed by the
Answer:

Coulomb attractive force between the nucleus (proton) of charge +e and the electron of charge -e is

⇒ \(\frac{1}{4 \pi \epsilon_0} \cdot \frac{e^2}{r^2}=\frac{k}{r^2}\)

We assume the proton to be too heavy compared to the electron. Then the proton remains stationary when the electron revolves in a circular orbit of radius r with velocity v

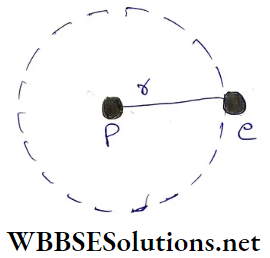
The force F provides the centripetal force \(\frac{m v^2}{r}\) for this revolution (m = electron mass)

∴ \(\frac{m v^2}{r}=\frac{k}{r^2}\)

The kinetic energy

⇒ \(E_k=\frac{1}{2} m v^2=\frac{k}{2 r}\)

The potential energy = work done to bring the electron from an infinite distance, L

⇒  \(E_p=\int_{\infty}^r \frac{k}{r^2} d r=k\left[-\frac{1}{r}\right]_{\infty}^r=-\frac{k}{r}\)

Total Energy

E = E_k + E_p

= \(=\frac{k}{2 r}-\frac{k}{r}=-\frac{k}{2 r}\)

= \(-\frac{1}{4 \pi \epsilon_0} \cdot \frac{e^2}{2 r}\)

This negative value of E suggests that the electron is bound in the hydrogen atom. An equal amount of positive energy is to be supplied from outside to make the total energy of the electron to be zero. Then the electron will be free from the atom.

Question 19. Given the value ofthe groundstate energy of hydrogen atom as -13.6 eV, find out its kinetic and potential energy in the ground and second excited states
Answer:

We know , energy E = \(\frac{k e^2}{2 r}\)

Now , kinetic energy E_k = \(\frac{k e^2}{2 r}\)

And potential energy, \(E_p=-\frac{k e^2}{r}\)

E_k = -E and E_p = 2E

Given that E = -13.6 eV

E_k = -(-13.6) eV = 13.6 eV

E_p = 2(-13.6) eV = -27.2 eV

Question 20. When is H_α line in the emission spectrum of hydrogen atoms obtained? Calculate the frequency of the photon
Answer:

The H_α line in the emission spectrum ofthe hydrogen atom is obtained when the electron transition takes place between n = 2 to n = 1.

We know that the energy in the nth level is

= \(E_n=\frac{-13.6}{n^2}\)

= \(E_2-E_1=\frac{-13.6}{2^2}-\frac{-13.6}{1^2}\)

= \(\frac{-13.6}{2^2}+13.6=10.2 \mathrm{eV}\)

= \(10.2 \times 1.6 \times 10^{-19} \mathrm{~J}=16.32 \times 10^{-19} \mathrm{~J}\)

Now, this difference in energy gives the energy of the photon emitted

∴ E₂ – E₁ = hν

∴ \(\frac{E_2-E_1}{h}=\frac{16.32 \times 10^{-19}}{6.63 \times 10^{-34}}\)

= 2.46 10 15 Hz

Question 21. Calculate the wavelength of radiation emitted when an electron in a hydrogen atom jumps from n = co to n = 1
Answer:

⇒ \(\frac{1}{\lambda}=R\left(\frac{1}{n_1^2}-\frac{1}{n_2^2}\right)\)

Or, \(\frac{1}{\lambda}=1.097 \times 10^7\left(\frac{1}{1}-\frac{1}{\infty}\right)\)

= 1.097 ×10⁷

= \(\lambda=\frac{1}{1.097 \times 10^7}\)

= 911.6 Å

Question 22. Define the distance of the closest approach. An a -particle of kinetic energy K is bombarded on a thin gold foil. The distance of the closest approach is r. What will be the die distance of the closed approach for an a -particle of double the kinetic energy?
Answer:

The distance of the closest approach is the distance from the nucleus where the total kinetic energy of a -particles is completely converted into potential energy.

The distance approach , r = \(\frac{2 Z e^2}{4 \pi \epsilon_0 K}\)

= \(r \propto \frac{1}{K}\)

If the kinetic energy of the α -particle is doubled, then the distance of the closest approach becomes half

Question 23. Find out the wavelength of the electron orbiting in the ground state of the hydrogen atom.
Answer:

The wavelength ofthe electron orbiting in the ground state of the hydrogen atom

= \(\frac{h c}{E}=\frac{6.62 \times 10^{-34} \times 3 \times 10^8}{13.6 \mathrm{eV}}\)

Ground state energy of hydrogen atom = 13.6 eV

= \(=\frac{6.62 \times 10^{-34} \times 3 \times 10^8}{13.6 \times 1.6 \times 10^{-19}}\)

= \(9.126 \times 10^{-8} \mathrm{~m}\)

= 912.6 Å

Question 24. A 12.75 eV electron beam Is used to excite a gaseous hydrogen atom at room temperature. Determine the wavelengths and the corresponding series of the lines emitted.
Answer:

We know that energy in the n-th orbit of the hydrogen atom,

= \(-\frac{13.6}{n^2} \mathrm{eV}\)

The energy of an electron in the excited state after absorbing energy 12.75 eV becomes- 13.6 + 12.75 = -0.85 eV

⇒ \(n^2=-\frac{13.6}{E_n}=\frac{-13.6}{-0.85}\)

= 16

Or, n = 4

Therefore, the electron gets excited to the ti = 4 state.

Total number of wavelengths in the spectrum

= \(\frac{n(n-1)}{2}=\frac{4 \times 3}{2}\)

= 6

During the transition from the fourth Bohr orbit to the ground state, the decrease in energy of the atom may occur in 6 different ways.

The possible emission lines are:

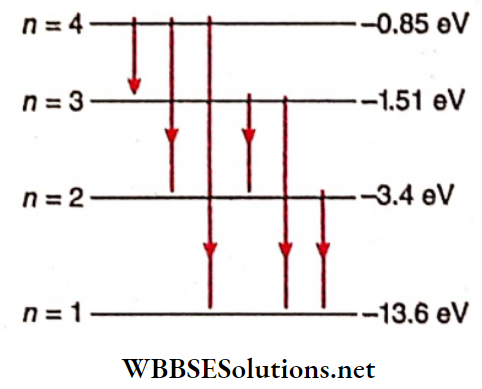
The emitted wavelength, for the jump from initial energy level E_i to final energy level E_f

⇒ \(\lambda_{i f}=\frac{h c}{E_i-E_f}\)

= \(\frac{6.6 \times 10^{-34} \times 3 \times 10^8}{E_i-E_f}\)

= \(\frac{19.8 \times 10^{-26}}{E_i-E_f} \mathrm{~m}\)

= \(\frac{19.8 \times 10^{-26}}{\left(E_i-E_f\right) \times 10^{-10}}\) Å

Wavelength emitted for the transition from n = 3 to n = 2, λ₃₂ = 6547.6 Å

Wavelength emitted for the transition from n = 3 to n = 1 , λ₃₁ = 1023.6 Å

Wavelength emitted for the transition from n = 2 to For energy -3.4 eV,

n = 1, λ₂₁ = 1213.2 Å

Wavelength emitted for the transition from n = 4 to

n = 3, λ₄₃ = 19110 Å

Lyman series → λ₂₁ (1213 A) and A)

Balmer series →  λ₃₂ (6548 A)

Paschen series →   λ₄₃(19110 A)

Question 25. The short wavelength limit for the Lyman series of the hydrogen spectrum is 913.4 A. Calculate the short wavelength limit for the Balmer series of the hydrogen
Answer:

Short wavelength limit for the Lyman series ofthe hydrogen atom,

⇒ \(\frac{1}{\lambda_L}=R\left(\frac{1}{1^2}-\frac{1}{\infty^2}\right)\)

∴ R = \(\frac{1}{\lambda_L}=\frac{1}{913.4 \times 10^{-10}}\)

Now, short wavelength limit for the Balmer series of the hydrogen atom,

⇒ \(\frac{1}{\lambda_B}=R\left(\frac{1}{2^2}-\frac{1}{\infty^2}\right)=\frac{1}{913.4 \times 10^{-10}}\left(\frac{1}{4}\right)\)

= \(\lambda_B=4 \times 913.4 \times 10^{-10}\)

= 3653.6

Question 26. The ground state energy of the hydrogen atom is -13.6 eV. If an electron makes a transition from an energy level of -1.51 eV to -3.4 eV, calculate the wavelength of the spectral line emitted and name the series of hydrogen spectrum to which it belongs. The energy in the n-th level of the hydrogen atom
Answer:

= \(-\frac{13.6}{n^2}\)

For energy -1.51 eV,

⇒ \(-1.51=-\frac{13.6}{n^2}\)

Or, \(n^2=\frac{13.6}{1.51} \approx 9\)

Or, n = 3

For energy -3.4 eV

⇒ \(-3.4=-\frac{13.6}{n^2}\)

Or, \(n^2=-\frac{13.6}{-3.4} \approx 4\)

Or n = 2

Thus, an electron makes a transition from energy level n = 3 to n = 2

⇒ \(\frac{h c}{\lambda_{32}}=\frac{m e^4}{8 \epsilon_0^2 h^2}\left(\frac{1}{n_2^2}-\frac{1}{n_3^2}\right)\)

Or, \(\frac{h c}{\lambda_{32}}=21.76 \times 10^{-19}\left(\frac{1}{2^2}-\frac{1}{3^2}\right)\)

Or, \(\lambda_{32}=\frac{h c}{21.76 \times 10^{-19}\left(\frac{1}{4}-\frac{1}{9}\right)}\)

= \(\frac{6.62 \times 10^{-34} \times 3 \times 10^8}{21.76 \times 10^{-19}} \times \frac{36}{5}\)

= 6.57 m-1

This wavelength belongs to the Balmer series.

Question 27. A hydrogen atom initially in the ground state absorbs a photon which excites it to the n = 4 level. Estimate the frequency ofthe photon
Answer:

Energy of hydrogen atom in n -th state, \(E_n=\frac{-13.6 \mathrm{eV}}{n^2}\)

According to the question, E₄– E₁ = hν

Or, \(-13.6\left(\frac{1}{4^2}-1\right) \mathrm{eV}=13.6 \times \frac{15}{16} \mathrm{eV}\)

Or, \(=13.6 \times \frac{15}{16} \times \frac{1.6 \times 10^{-19}}{6.62 \times 10^{-34}}\)

= 3 × 10¹⁵ Hz