WBCHSE Class 11 Physics Notes For Wave Motion

Wave Motion Introduction

When a small piece of stone is thrown into a pond, the water surface gets disturbed. The ripples formed due to this disturbance do not remain confined to the region where the stone hits the water’s surface, but it spreads in all directions along the surface.

• As the disturbance passes, the water particles start oscillating, i.e., moving up and down about their mean positions but are not displaced along the water surface. The pattern that moves along the pond due to such movement of individual particles of the medium, is called a wave.
• This wave motion transfers energy from one point to another, but no mass transport is associated with this transfer.

Wave: A wave is a disturbance that travels through a medium, whereas the particles constituting the medium do not travel.

• We are familiar with different types of waves; like sound waves, light waves, radio waves, etc. Among these, sound wave is a mechanical wave and the other two are electromagnetic waves.
• In this chapter, we are mainly concerned with mechanical waves. Mechanical waves can propagate only through a material medium, whereas electromagnetic waves do not require any material medium for propagation, i.e., they can propagate through a vacuum.

Wave Motion Mechanical Waves

The origin and propagation of mechanical waves depend on three properties of materials

1. Elasticity
2. Interia and
3. Cohesion
4. Elasticity: If any part of a material or a medium is displaced from its equilibrium position, stress is developed in it due to its elasticity. This stress tries to bring that part back to its equilibrium position.
5. Inertia: When a particle returns to its equilibrium position, it has a motion due to inertia. So the particle cannot come to rest immediately on reaching the equilibrium position. Due to the inertia of motion, it moves to the opposite side crossing the equilibrium position.
   • These two incidents— coming back to the equilibrium position due to elasticity and moving to the opposite side due to inertia of motion—happen alternately in any part of a material or a medium, causing that part to vibrate.
6. Cohesion: The adjacent molecules of a material medium attract each other. This phenomenon is called cohesion. If any part of a material medium begins to vibrate, the adjacent part is then forced to vibrate due to cohesion.
   • In this way, vibration propagates from one layer to the next. This type of propagating vibration is known as a mechanical wave.

Mechanical Waves Definition: The disturbance which travels through a material medium, due to collective vibration of the particles of the medium, is known as a mechanical wave.

Characteristics Of A Mechanical Wave:

1. For the propagation of a mechanical wave, a material medium is necessary. This wave cannot propagate through a vacuum.
2. Each particle of the medium forces the adjacent particles to vibrate, but the particle itself is not carried away from its equilibrium position; rather, the wave advances through the medium.
3. The vibrating particles of any material medium have their own mechanical energies and potential energy. This mechanical energy is forced vibration, i.e., a wave in motion transfers mechanical energy through the medium. So, this type not wave is called a mechanical wave.

Wave Motion Transverse And Longitudinal Waves

Simple Harmonic Wave: If the motion of the particles of a medium is sample harmonic, then the corresponding wave is known as a simple harmonic wave.

There Are Two Types Of Simple Harmonic Waves

1. Transverse wave and
2. Longitudinal waves

Transverse Wave Definition: A wave that propagates in a direction, perpendicular to the direction of motion of the vibrating particles of the medium, is called a transverse wave.

Examples Of Transverse Waves:

1. If a small stone is thrown into a pond, it generates waves that spread out horizontally in all directions. Now, if a small piece of cork is floated on the water surface, it oscillates vertically about its mean position. So the wave motion is directed along the horizontal direction, but the direction of motion of the cork and the vibrating water panicles are vertical. This is an example of a transverse wave.
2. A rubber rope AB is taken. The end B is fixed to a rigid support and the other end A is held in such a way that the rope is in a stretched condition. Now the end A is oscillated vertically A wave is found to be generated along the rope and it propagates towards the end B.

It is evident that the direction of vibration of each particle of the rope is perpendicular to the direction of propagation of the wave through the rope.

• The particle whose position at some instant is P occupies the position Q at another instant. Since PQ and AB are perpendicular to each other, the wave is obviously a transverse wave.
• A transverse wave can be described generally through. The straight line OBDF is the equilibrium position of the rope. Suppose at an instant, the particle at O is passing through its mean position in its course of vibration from an upward to a downward direction.
• The directions of motion of the different particles of the medium at that instant have been shown by arrows. A and E are two points having a maximum displacement in the positive direction.
• These are called crests. Again C is a point having maximum displacement in the negative direction. This is called a trough.

It is evident that at the said instant, points A and E are situated on the same side of and at the same distance from the mean position. Their velocities are also the same. In short, the conditions of motion at points A and E are the same.

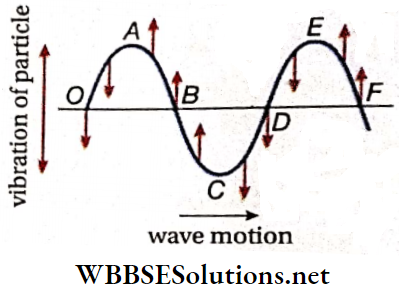
• So, these two points are in the same phase. Similarly, the points O and D, or B and F are in the same phase.
• On the other hand, the conditions of motion at points A and C are opposite. So, these two points are in opposite phases.
• Electromagnetic waves like light waves, radio waves, etc., are transverse waves. The two quantities responsible for the production of electromagnetic waves are the electric field vector and magnetic field vector, which are always perpendicular to the direction of propagation of the wave.
• Electromagnetic waves can propagate through solid, liquid, and gaseous media and even through a vacuum.

Longitudinal Wave Definition: A wave which propagates of motion of the vibrating particles of t a longitudinal wave.

Example Of Longitudinal Wave: A thin and long spring AB is taken whose spring constant is very small. The end B is fixed to a rigid support and the other end A is held in such a way that the spring is in stretched condition.

• Now the end A is made to oscillate back and forth so that a wave moves along its length to end B.
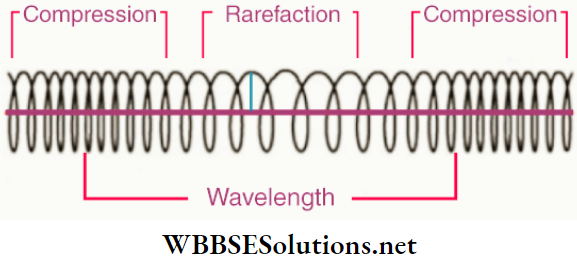
• Some coils of the spring come close to each other creating compression and some other coils move away from each other creating rarefaction.
• Compressions and rarefactions are formed alternately along the length of the spring and they propagate towards the end B.
• Since the wave motion is directed from A to B, and during compressions and rarefactions the coils of the spring oscillate parallel to the length of the spring, this wave is a longitudinal wave.

Actually, a longitudinal wave travels through a medium in the form of periodic compressions and rarefactions.

• Let the point O be the equilibrium position of a particle in a material medium. The medium may be imagined to consist of many layers of equal thickness.
• A few layers on the right side of O have been shown. Now, some energy from outside is supplied to the layer at O so that the layer oscillates along the line AB.
• When the layer moves from A to B due to oscillation, it exerts pressure on the layers in front of it. So, those layers get compressed due to the property of compressibility of solid, liquid and gaseous media.
• Thus compression takes place in the region CD of the medium due to the motion of the layer at point O from A to B.
• The opposite incident happens at the time of motion of the layer from B to A, i.e., the layers of the region CD get rarefied due to the decrease in pressure.
• By that time, the previous compression reaches the region DE by compressing the next layers leaving a rarefaction behind.

So, a complete oscillation (ABA) generates compression and a rarefaction. These compressions and rarefactions are not confined to a region, but move through the medium due to the property of compressibility, thereby producing a wave.

• Again, if we think of any array of layers, it is found that the layers alternately get compressed and rarefied parallel to the direction of wave motion.
• It is evident that density and pressure in the medium increase in the zones of compression and decrease in the zones of rarefaction.
• It is the process of propagation of sound wave in air. It is clear that a sound wave is a longitudinal mechanical wave.

Nature Of The Medium: All longitudinal waves are mechanical waves. This type of waves cannot propagate without any medium. Longitudinal waves can propagate through any solid, liquid or gaseous medium.

• These media revert to their original conditions after the external driving oscillation (like ABA) is withdrawn.
• Till that instant, compression or rarefaction passes to the next parallel layers continuously in the direction of the force applied.
• Transverse elastic waves can be formed only in solids, not in liquids and gases. This is because liquids and gases have negligible compressibility and hence cannot sustain shearing stress as they have no definite shape.
• A solid has a definite shape and it opposes any force exerted to change its shape, i.e., a solid substance can sustain shearing stress.
• So, if one of its layers oscillates, its adjacent layer is also forced to oscillate in the same direction.
• Light waves, radio waves, etc., are not mechanical waves. These are electromagnetic waves. The elasticity of the medium has no relation with the electric and magnetic fields.
• So electromagnetic waves can propagate through vacuum, as well as through solid, liquid, or gas.

Actually, the waves set up on the surface of the water are not elastic waves; they are mechanical waves produced due to Earth’s gravity.

Difference Between Transverse And Longitudinal Waves:

Wave Motion Other Classifications Of Waves

Considering the nature of vibration, we classify waves as mechanical waves, electromagnetic waves, etc. Again, considering the direction of motion of the vibrating particles of the medium and that of the wave, we classify them as transverse waves and longitudinal waves.

In addition, waves can also be categorized on the basis of their different properties. A few of them are discussed below.

On the basis of the direction of energy transmission: The wave that transmits energy in a single direction is called a one-dimensional wave, For example, a transverse wave formed in a stretched string and a longitudinal wave formed In an elastic spring are one-dimensional waves.

• The wave which transmits energy along a plane is called a two-dimensional wave. The wave formed on the surface of water, when a stone is thrown on it, is two-dimensional.
• The wave which transmits energy in all directions is called a three-dimensional wave. Sound waves, light waves, radio waves, etc., are three-dimensional waves.

On the basis of characteristics of particle vibration: If the particles of a medium vibrate simply harmonically, the corresponding wave is called a simple harmonic wave. Practically most waves are produced by complex vibrations. But any complex wave may be described as a superposition of a number of simple harmonic waves.

On the basis of the limit of wave motion: If any wave advances through a medium continuously with a definite velocity, the wave is called a travelling or progressive wave. If the wave is not damped, it can propagate up to infinity. On the other hand, if the wave does not advance, but remains confined in a region, it is called a standing or stationary wave.

Wave Motion Some Physical Terms Related To Waves

Waves Phase: The quantity from which the motion of a wave can be known completely is called the phase of the wave.

Displacement, velocity, acceleration, etc., of a vibrating particle can be obtained from it. The particles at O and D are in the same phase. The particles at A and E, or the particles at C and E, are also in the same phase. Particles at A and C and particles at C and D are in opposite phases.

Complete Wave: The wave in between two consecutive particles, having the same phase at an instant, is known as a complete wave. Show respectively how a complete transverse wave is formed In between two consecutive crests, and how a complete longitudinal wave is formed by the combination of a compression and, a rarefaction.

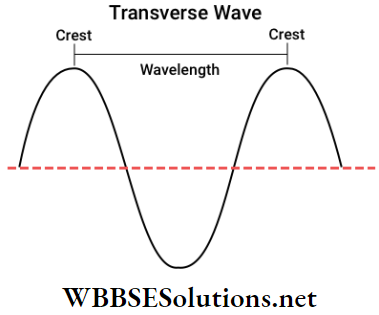
Wavelength: The length of a complete wave, i.e., the distance between two consecutive particles having the same phase at an instant, is called tire wavelength (λ). OD or AE is the wavelength of the transverse wave. Again CE or DF is the wavelength of the longitudinal wave. Generally, it can be said that

The wavelength of a transverse wave = distance between any two consecutive crests or consecutive troughs.

Wavelength of a longitudinal wave = total length of a pair of successive compression and rarefaction.

In general wavelength of a wave is the distance between two consecutive points in the same phase of motion at the same instance of time.

Time period: The time required to form a complete wave, i.e., time taken by a wave to cover a distance between two consecutive particles having the same phase of vibration, is called the time period (T) of the wave.

Frequency: Frequency (n) of a wave is the number of complete waves formed in unit time.

Amplitude: The amplitude of a wave is the maximum displacement of any particle producing the wave, from its mean position. The distance of the points A, C, or E from the straight line OBDF is the amplitude of the wave.

Wave Velocity: The distance traveled by a wave in a unit of time is called its wave velocity (V). Energy is transmitted through the medium by the wave with this velocity. It may be noted that wave velocity is different from particle velocity. Particle velocity is the velocity of the particles of the medium which execute simple harmonic motions about their mean positions.

Wavefront: All the particles on a surface normal to the direction of propagation of a wave have the same phase. A surface of this type is called a wavefront. In other words, the wavefront is the locus of all points having the same phase of motion at the same instance of time.

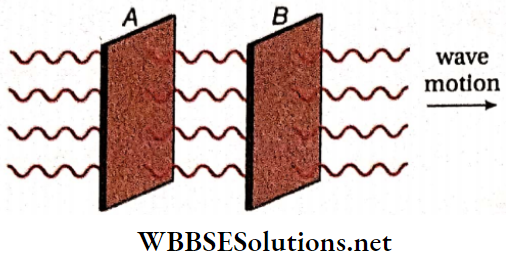
This surface is perpendicular to the direction of propagation of wave at any point. The plane A is a wavefront because the phase of all the particles lying on plane A is the same. Similarly, the plane B is another wavefront. Clearly, planes A and B are parallel.

Wavefront Definition: Any surface, that is normal to the direction of propagation of a wave, is known as a wavefront. The particles lying on a wavefront have the same phase.

Wave Ray: A normal drawn on a wavefront is called a ray. The energy of a wave is transferred from one part of the medium to another along the ray.

Relations Among The Physical Quantities

Relation Between Time Period And Frequency: If T is the time period of a wave, one complete wave is formed in time T. Therefore, the number of complete waves formed in unit time is \(\frac{1}{T}\).

So, according to the definition of frequency,

n = \(\frac{1}{T} \quad \text { or, } T=\frac{1}{n}\)…(1)

Relation Between Wavelength And Wave Number: The number of complete waves in a length λ is 1.

So, the number of complete waves in unit length is \(\frac{1}{\lambda}\). This quantity multiplied by 2π is known as the wave number k, i.e.,

k = \(2 \pi \cdot \frac{1}{\lambda}=\frac{2 \pi}{\lambda} \quad \text { or, } \lambda=\frac{2 \pi}{k}\)…(2)

Relation Among Wave Velocity, Frequency, And Wavelength: Let the frequency of a wave be n, wavelength λ, and wave velocity V. According to the definition of frequency, the number of complete waves formed in unit time is n. Again, the wave covers a distance of V in unit time. So, the length of n number of complete waves is V.

So, the length of one complete wave = \(\frac{V}{n}\);

Then, according to the definition of wavelength, \(\lambda=\frac{V}{n} \quad \text { or, } V=n \lambda\)…(3)

i.e., wave velocity = frequency x wavelength

Again if T is the time period of a wave, n = \(\frac{1}{T}\)

∴ V = \(n \lambda=\frac{\lambda}{T} \quad \text { or, } \lambda=V T\)….(4)

From equation (3), n = \(\frac{V}{\lambda}\). If more than one wave move through a medium with the same velocity, V will be a constant. In that case, \(n \propto \frac{1}{\lambda},\) i.e., frequency and wavelength will be inversely proportional to each other. So, greater the wavelength of a wave, the smaller its frequency, and vice versa.

Wave Motion Numerical Examples

Example 1. The wave generated on a water surface advances 1 m in Is. If the wavelength is 20 cm, how many waves are produced per second?
Solution:

Wavelength, λ = 20 cm;

wave velocity, V = 1 m · s^-1 = 100 cm · s^-1

If n is the frequency, V = nλ

or \(n=\frac{V}{\lambda}=\frac{100}{20}=5 \mathrm{~s}^{-1}\)

So, 5 waves are produced per second.

Example 2. A radio center broadcasts radio waves of length 300 m. What is the frequency of this wave? Given, the velocity of light = 3 x 10⁵ km · s^-1.
Solution:

Wavelength of radio wave, λ = 300 m.

Radio waves are electromagnetic waves like light and both of them have the same velocity.

So, the velocity of radio waves,

V = \(3 \times 10^5 \mathrm{~km} \cdot \mathrm{s}^{-1}=3 \times 10^8 \mathrm{~m} \cdot \mathrm{s}^{-1}\)

∴ Frequency, n = \(\frac{V}{\lambda}=\frac{3 \times 10^8}{300}=10^6 \mathrm{~Hz}=1 \mathrm{MHz}\)

Example 3. The frequency of a tuning fork is 400 Hz and the velocity of sound in air is 320 m · s^-1. Find how far would the sound travel when the fork just completes 30 vibrations.
Solution:

Here V = 320 m · s^-1; n = 400

We know, V = nλ

∴ 320 = 400 x λ

or, \(\lambda=\frac{320}{400}=\frac{4}{5} \mathrm{~m}\)

So, when the fork completes 1 vibration, sound travels \(\frac{4}{5}\)m.

∴ When the fork completes 30 vibrations, sound travels \(\frac{4}{5}\)x30 = 24m

Example 4. A light pointer attached to one arm of a tuning fork touches a plate. The tuning fork is made to vibrate and the plate is allowed to fall freely downwards simultaneously. The tuning fork completes 8 vibrations when the pointer shows a downward displacement of 10 cm of the plate. What is the frequency of the tuning fork?
Solution:

Suppose a time t is taken by the plate to move 10 cm downwards.

So, from the relation h = \(\frac{1}{2}\)gt², we have

10 = \(\frac{1}{2}\) x 980 x t²

or, \(t^2=\frac{1}{49} \quad \text { or, } t=\frac{1}{7} \mathrm{~s}\)

The tuning fork completes 8 vibrations in that time,

So, frequency of the tuning fork = \(\frac{8}{1 / 7}=56 \mathrm{~Hz}\)

Example 5. What is the length of a compression in the sound wave, produced by a tuning fork of frequency 440 Hz? Given, the velocity of sound in air is 330 m · s^-1.
Solution:

Frequency of the sound wave (n) = frequency of the tuning fork = 440 s^-1

Velocity of sound (V) = 330 m · s^-1

∴ Wavelength, \(\lambda=\frac{V}{n}=\frac{330}{440}=\frac{3}{4} \mathrm{~m}\)

∴ Length of a compression = \(\frac{\text { wavelength }}{2}=\frac{3}{4 \times 2}\)

= 0.375 m = 37.5 cm.

Example 6. A rod hanging from a spring is dipped partly in water. The rod vibrates 180 times per minute. As a result, waves are formed on water and have 6 consecutive crests within a distance of 30 cm. Find the velocity of the wave in water.
Solution:

Here, frequency n = \(\frac{180}{60}\) = 3 Hz

There are 5 waves within 6 consecutive crests.

So, the length of these 5 waves = 30 cm

∴ Wavelength, \(\lambda=\frac{30}{5}=6 \mathrm{~cm}\)

∴ Velocity of the wave, V= nλ = 3 x 6 = 10 cm · s^-1.

Example 7. The frequency of a tuning fork is 280 advances 80 m In a medium while the tuning fork executes 70 complete oscillations. Determine the velocity of sound in that medium.
Solution:

Wavelength, \(\lambda=\frac{80}{70}\) = \(\frac{8}{7}\) cm

So, the velocity of sound in the medium,

V = \(n \lambda=280 \times \frac{8}{7}=320 \mathrm{~m} \cdot \mathrm{s}^{-1}\)

Example 8. The frequency of a tuning fork is 512 Hz. When the tuning fork makes 30 vibrations, the emitted sound travels 20 m in the air. Determine the wavelength and the velocity of sound waves in air.
Solution:

Wavelength, \(\lambda=\frac{20}{30}\) = 0.667 m

∴ Velocity of sound in air,

V = nλ = 512 x = 341.33 m · s^-1

Example 9. When two vibrating tuning forks of frequencies 50 Hz and 100 Hz touch the surface of water, they produce waves of wavelengths 0.6 cm and 0.36 cm, respectively. Compare the velocities of the two surface waves.
Solution:

If V₁ and V₂ are die velocities of die two surface waves, then

⇒ \(\frac{V_1}{V_2}=\frac{n_1 \lambda_1}{n_2 \lambda_2}=\frac{50 \times 0.6}{100 \times 0.36}=\frac{5}{6}\)

i.e., \(V_1: V_2=5: 6 .\)

 

Wave Motion Equation Of A Travelling Or Progressive Wave Numerical Examples

Example 1. The equation of a progressive wave is y = 15sin(660πt-0.02πx) cm. Calculate the frequency and the velocity of the wave.
Solution:

The general equation of a progressive wave is,

y = \(A \sin \omega\left(t-\frac{x}{V}\right)\)….(1)

The equation of the given wave is

y = \(15 \sin (660 \pi t-0.02 \pi x)=15 \sin 660 \pi\left(t-\frac{0.02 x}{660}\right)\)

= \(15 \sin 660 \pi\left(t-\frac{x}{660 / 0.02}\right) \mathrm{cm}\)…(2)

Comparing equations (1) and (2) we have ω = 660π

⇔ Frequency, n = \(\frac{\omega}{2 \pi}=\frac{660 \pi}{2 \pi}=330 \mathrm{~s}^{-1}=330 \mathrm{~Hz} \text {; }\)

Wave velocity, V = \(\frac{660}{0.02}=\frac{660 \times 100}{2}=33000 \mathrm{~cm} \cdot \mathrm{s}^{-1}\)

= \(330 \mathrm{~m} \cdot \mathrm{s}^{-1} \text {. }\)

Example 2. A wave vibrating along the y-axis propagates along the negative direction of x-axis. The values of its amplitude/frequency and wavelength are 10cm, 500 Hz, and 100 cm, respectively. Write down the equation of the progressive wave.
Solution:

Amplitude, A = 10 cm; frequency n = 500 Hz and wavelength, λ = 100 cm

∴ Wave velocity, V = nλ = 500 x 100 = 5 x 10⁴ cm · s^-1

Angular frequency, ω = 2πn = 2π x 500 = 1000π Hz

Since the wave propagates along the negative x-direction, the equation of the progressive waves,

y = \(A \sin O\left(t+\frac{x}{V}\right)=10 \sin 1000 x\left(t+\frac{x}{5 \times 10^4}\right) \mathrm{cm}\)

Example 3. The equation of a progressive wave is y = 20sinπ(4t – 0.01x)cm. Determine the amplitude, frequency, wavelength, and velocity of the wave.
Solution:

The general equation of a progressive wave is y = \(A \sin \frac{2 \pi}{d}(V t-x)\)…(1)

The given equation is y = \(20 \sin \pi(4 t-0.01 x)\)

= \(20 \sin \pi\left(4 t-\frac{x}{100}\right)=20 \sin \frac{\pi}{100}(400 t-x)\)

= \(20 \sin \frac{2 \pi}{200}(400 t-x) \mathrm{cm}\)…(2)

Comparing equations (1) and (2) we have,

Amplitude, A = 20 cm; wavelength, λ = 200 cm;

Wave velocity, V = 400 cm · s^-1.

So, frequency, n = \(\frac{V}{\lambda}=\frac{400}{200}=2 \mathrm{~Hz}\)

Example 4. The equation of a progressive wave is y = \(10 \sin 2 \pi\left(\frac{t}{0.005}-\frac{x}{10}\right)\)cm; here t and x are given in CGS units. Determine the amplitude, wavelength, and velocity of the wave.
Solution:

y = \(10 \sin 2 \pi\left(\frac{t}{0.005}-\frac{x}{10}\right)\)

= \(10 \sin \frac{2 \pi}{10}\left(\frac{t}{0.0005}-x\right) \mathrm{cm}\)…(1)

Comparing equation (1) with the general equation of a progressive wave, y = \(A \sin \frac{2 \pi}{\lambda}(V t-x)\), we have,

Amplitude, A = 10 cm; wavelength, λ = 10 cm

Velocity of wave, V = \(\frac{1}{0.0005}=2000 \mathrm{~cm} \cdot \mathrm{s}^{-1}\)

Example 5. A wave traveling along the X-axis is described by the equation y(x, t) = 0.005cos(αx—βt). If the wavelength and the time period of the wave are 0.08 m and 2.0 s respectively, what are the values of α and β?
Solution:

= \(\frac{2 \pi}{\lambda}=\frac{2 \pi}{0.08}=25 \pi \mathrm{m}^{-1} ; \beta=\frac{2 \pi}{T}=\frac{2 \pi}{2}=\pi \mathrm{s}^{-1}\)

[In this question β and α are used instead of the usual symbols ω and k respectively].

Example 6. The equation of a progressive wave is given by y = 10sinπ(t-0.002x) cm. Determine

1. Maximum displacement
2. Maximum velocity and
3. Maximum acceleration of the particles of the medium,

Solution: Given the equation,

y = 10sinπ(t-0.002x) cm….(1)

General equation of a progressive wave,

y = \(A \sin \omega\left(t-\frac{x}{V}\right)\)…(2)

Comparing equations (1) and (2) we have,

A = 10 cm; \(\omega=\pi \mathrm{Hz} ; V=\frac{1}{0.002}=500 \mathrm{~cm} \cdot \mathrm{s}^{-1}\)

1. Maximum displacement of the particles of the medium = amplitude of the wave (A) =10 cm.

2. Velocity of a particle, \(v=\frac{\partial y}{\partial t}=\omega A \cos \omega\left(t-\frac{x}{V}\right) \mathrm{cm} \cdot \mathrm{s}^{-1}\)

∴ Maximum velocity of the particle = ωA = π x 10 = 31.4 cm · s^-1.

3. Acceleration of a particle,

a = \(\frac{\partial v}{\partial t}=-\omega^2 A \sin \omega\left(t-\frac{x}{V}\right) \mathrm{cm} \cdot \mathrm{s}^{-2}\)

∴ Maximum acceleration of the particle = ω²A = π² x 10 = 98.7 cm · s^-2.

Example 7. The equation y = \(y_0 \sin 2 \pi\left(n t-\frac{x}{\lambda}\right)\) expresses a transverse wave. If the maximum particle velocity is equal to four times the wave velocity, show that \(\lambda=\frac{1}{2} \pi y_0\)
Solution:

Given equation,

y = \(y_0 \sin 2 \pi\left(n t-\frac{x}{\lambda}\right)=y_0 \sin \frac{2 \pi}{\lambda}(\lambda n t-x)\)

Comparing this equation with the general equation of a progressive wave, y = \(A \sin \frac{2 \pi}{\lambda}(V t-x)\), we have,

A = y₀; V= λn

Again, particle velocity is given by v = \(\frac{\partial y}{\partial t}=\frac{2 \pi V}{\lambda} A \cos \frac{2 \pi}{\lambda}(V t-x)\)

So, maximum particle velocity, \(v_0=\frac{2 \pi V A}{\lambda}\)

According to the equation \(\frac{v_0}{V}=4 \text { or, } \frac{2 \pi A}{\lambda}=4 \text { or, } \lambda=\frac{2 \pi A}{4}=\frac{1}{2} \pi y_0\)

Example 8. A wave equation that gives a displacement along y direction Is given by y = 10^-4 sin(60t+ 2x) where x and y are in meters and f is time in seconds. Determine the wavelength, frequency, and velocity of the wave.
Solution:

Given equation is y = \(10^{-4} \sin (60 t+2 x)\)

= \(10^{-4} \sin 2(30 t+x)\)

= \(10^{-4} \sin \frac{2 \pi}{\pi}(30 t+x)\)

Comparing the given equation with the general equation of a progressive wave, y = \(A \sin \frac{2 \pi}{\lambda}(V t+x)\), we have,

Wavelength, λ =π= 3.14 m;

Wave velocity, V = 30 m · s^-1; (in the direction of negative x-axis)

Frequecy, n = \(\frac{V}{\lambda}=\frac{30}{3.14}=9.55 \mathrm{~Hz} .\)

Example 9. A wave having a frequency 200 Hz is advancing with a velocity 40 m · s^-1. What is the phase difference of two particles separated by 5 cm in the direction of wave motion?
Solution:

Here n = 200 Hz; V = 40 m · s^-1 = 4000 cm · s^-1

So, \(\lambda=\frac{V}{n}=\frac{4000}{200}=20 \mathrm{~cm}\)

∴ Phase difference = \(\frac{2 \pi}{\lambda}\) x path difference

= \(\frac{2 \pi}{20} \times 5=\frac{\pi}{2} \mathrm{rad}=90^{\circ} .\)

Example 10. \(y_1=0.1 \sin \frac{\pi}{2}(200 t-x) \mathrm{cm}\) and \(y_2=0.2 \sin \frac{\pi}{2}(200 t-x+5) \mathrm{cm}\) are two wave equations. Show that the phase difference of the two waves does not change. What is the value of that phase difference?
Solution:

Comparing the given equations of the two waves with the general equation of a progressive wave,

y = \(A \sin \frac{2 \pi}{\lambda}(V t-x)\),

The wavelength (λ) and the wave velocity (V) of the given two waves are equal. So, the two waves can propagate with any special phase (crest or trough) maintaining equal distance with respect to each other. So, the phase difference of the two waves does not change.

Phase of the first wave \(\left(\theta_1\right)=\frac{\pi}{2}(200 t-x)\)

Phase of the second wave \(\left(\theta_2\right)=\frac{\pi}{2}(200 t-x+5)\)

So, phase difference = θ₂-θ₁

= \(\frac{\pi}{2} \cdot 5=\frac{5 \pi}{2} \mathrm{rad}=\frac{5}{2} \times 180^{\circ}=450^{\circ}\)

Since 450° = 360° + 90°, the two angles 450° and 90° are equivalent, i.e., the required phase difference = 90°.

Example 11. The equation of a progressive wave is y = \(0.1 \sin \frac{\pi}{2}(200 t-x) \mathrm{cm}\)

1. What is the phase of the wave at the point x = 2 at time t =0?
2. What is the phase difference between two points separated by 8 cm?
3. How does the phase at any point change in 0.005 s?

Solution:

The phase of the given progressive wave,

θ = \(\frac{\pi}{2}(200 t-x)\) ….(1)

1. Putting x = 2, t = 0 in equation (1),

θ = \(\frac{\pi}{2}(-2)=-\pi \mathrm{rad}=-180^{\circ}\)

-180° and + 180°—these two angles are equivalent. So, θ = 180°.

2. For two points separated by 8 cm,

⇒ \(\theta_1=\frac{\pi}{2}\left(200 t-x_1\right), \theta_2=\frac{\pi}{2}\left(200 t-x_2\right)\)

∴ Phase difference = θ₂ – θ₁

= \(\frac{\pi}{2}\left(x_2-x_1\right)=\frac{\pi}{2} \times 8=4 \pi \mathrm{rad}\)

But the angle 4π rad is equivalent to 0°.

So, phase difference = 0°.

2. The required phase change

= \(\frac{\pi}{2}(200 \times 0.005)=\frac{\pi}{2} \times 1=\frac{\pi}{2} \mathrm{rad}=90^{\circ}\)

Example 12. The amplitude of a wave propagating in the positive x-direction is given by y = \(\frac{1}{1+x^2}\) at time t = 0 and by y = \(\frac{1}{1+(x-1)^2}\) at t = 2 s where x and y are in meters, The shape of the wave does not change during the propagation. What is the velocity of the wave?
Solution:

Considering the point x = 0 at t = 0, amplitude, y = \(\frac{1}{1+0^2}=1 \mathrm{~m}\)

Now, if the wave propagates up to the point x = x₁ at t = 2s, then

1 = \(\frac{1}{1+\left(x_1-1\right)^2} \quad \text { or } 1+\left(x_1-1\right)^2=1 \)

or, \(\left(x_1-1\right)^2=0 \quad \text { or, } x_1-1=0 \quad \text { or, } x_1=1 \mathrm{~m},\)

i.e., in time 2s tire phase of amplitude 1m has advanced from the point x = 0 to x = 1m.

So, the velocity of the wave = \(\frac{1}{2}\) = 0.52 m · s^-1

Example 13. A plane progressive wave of frequency 25 Has, amplitude 2,5 x 10^-5 m, and initial phase zero propagates along the negative x-direction with a velocity of 300 m · s^-1. At any Instance, what Is the phase difference between the oscillations at two points 6 m apart along the line of propagation of the wave? Also, determine the corresponding amplitude difference.
Solution:

Here, n = 25 Hz, A = 2.5 x 10^-5 m, V= 300 m · s^-1

∴ \(\lambda=\frac{V}{n}=\frac{300}{25}=12 \mathrm{~m}\)

So, two points 6 rn apart have a path difference of \(\frac{\lambda}{2}\).

∴ Phase difference = \(\frac{2 \pi}{\lambda} \cdot \frac{\lambda}{2}=\pi \mathrm{rad}=180^{\circ}\)

So, the two points are in the opposite phase. For example, if a crest is formed at a point, a trough would be formed 6m apart. However, the magnitudes of the amplitude would be the same.

∴ Amplitude difference = 0.

Example 14. The bulk modulus and the density of steel are 80 and 8 times, respectively, of those of water. Calculate the speed of sound in steel. Given, the speed of sound in water = 1493 m · s^-1.
Solution:

The velocity of sound in an elastic medium is

V = \(\sqrt{\frac{E}{\rho}}\)

Where E = modulus of elasticity of the medium ρ = density of the medium

∴ Velocity of sound in water, \(V_w=\sqrt{\frac{E_w}{\rho_w}}\)

Velocity of sound in steel, \(V_w=\sqrt{\frac{E_w}{\rho_w}}\)

∴ \(\frac{V_s}{V_w}=\sqrt{\frac{E_s}{\rho_s} \cdot \frac{\rho_w}{E_w}}=\sqrt{\frac{E_s}{E_w} \times \frac{\rho_w}{\rho_s}}=\sqrt{80 \times \frac{1}{8}}=\sqrt{10}=3.162\)

∴ \(V_s=3.162 V_w=3.162 \times 1493=4721 \mathrm{~m} \cdot \mathrm{s}^{-1} .\)

Example 15. A wave is represented by y = \(20 \cdot \sqrt{3} \sin \left(\frac{2 \pi t}{T}-\frac{2 \pi x}{\lambda}\right) \mathrm{cm}\), Calculate the displacement of a particle at the position x = \(\frac{lambda}{6}\) on the wave at time t = \(\frac{T}{3}\)
Solution:

Given equation, \(y=20 \cdot \sqrt{3} \sin \left(\frac{2 \pi t}{T}-\frac{2 \pi x}{\lambda}\right)\)

Putting x = \(\frac{\lambda}{6}$ and $t=\frac{T}{3}\), we get

Displacement, y = \(20 \cdot \sqrt{3} \sin \left(\frac{2 \pi T}{3 T}-\frac{2 \pi \lambda}{6 \lambda}\right)=20 \cdot \sqrt{3} \sin \left(\frac{2 \pi}{3}-\frac{\pi}{3}\right)\)

= \(20 \cdot \sqrt{3} \sin \frac{\pi}{3}=20 \cdot \sqrt{3} \cdot \frac{\sqrt{3}}{2}=30 \mathrm{~cm}\)

Example 16. The equations of two waves are, \(y_1=0.30 \sin (314 t-1.57 x)\),
\(y_2=0.10 \sin (314 t-1.57 x+1.57)\) Determine the phase difference and the ratio of the intensities of the two waves.
Solution:

Phase of the first wave, θ₁ = 314t – 1.57x

Phase of the second wave, θ₂ = 314t – 1.57x +1.57

∴ The phase difference of the two waves

= \(\theta_2-\theta_1=1.57 \mathrm{rad}=1.57 \times \frac{180}{\pi}=90^{\circ}\)

We know that the intensity of a wave is proportional to the square of its amplitude.

∴ Ratio of the amplitudes of the two waves = \(\frac{A_1}{A_2}=\frac{0.30}{0.10}=\frac{3}{1}\)

∴ Ratio of the intensities of the two waves = \(\frac{I_1}{I_2}=\frac{A_1^2}{A_2^2}=\frac{9}{1}\)

Wave Motion Velocity Of Sound In Solid And Liquid Media

Sound propagates through solids and liquids as longitudinal elastic wave tike in gaseous media. But the velocities of sound in solid and liquid are different. If E is the modulus of elasticity of the medium and ρ is its density, Newton’s formula for the velocity of sound is given by,

c = \(\sqrt{\frac{E}{D}}\)…(1)

• The density of any solid or liquid is greater than that of a gas. Bin the modulus of elasticity of a solid or a liquid is much greater than the Thar of a gas.
• So, the velocity of sound in a solid or B. Liquid is greater than that in a gas. For example, the psVjcities of sound in iron and in water are about 15 times and 4.5 times that in air, respectively.
• The velocity of sound in iron is greater than that in air—it can be easily understood from a simple experiment. If one end of a long iron pipe is struck heavily, the sound of striking is heard twice at the other end of the pipe.
• Sound is heard for the first time due to its propagation through the iron and for the second time due to its propagation through the air in the pipe. An iron pipe nearly 100 m in length should be taken to hear the two sounds distinctly.

There is one important difference between solids and liquids as media of propagation of sound. The modulus of elasticity for the solid is, Young’s modulus (Y) while it is the bulk modulus (k) in the case of the liquid. So the equation (1)

For solid, c = \(\sqrt{\frac{Y}{\rho}}\)….(2)

and for liquid, c = \(\sqrt{\frac{k}{\rho}}\) …(3)

In case of steel, Y = 2 x 10¹¹ N · m^-2; ρ = 7850 kg · m^-3;

From equation (2) we get,

c = \(\sqrt{\frac{2 \times 10^{11}}{7850}} \approx 5048 \mathrm{~m} \cdot \mathrm{s}^{-1}\)

In case of water, k = 2.1 x 10⁹ N · m^-2; ρ = 1000 kg · m^-3

From equation (3) we get, c = \(\sqrt{\frac{2.1 \times 10^9}{1000}}=1449 \mathrm{~m} \cdot \mathrm{s}^{-1}\)

Velocity Of Sound In Solid And Liquid Media Numerical Examples

Example 1. Two explosions are made simultaneously from a ship, one above the surface of the water and another just below it. Sound is heard in a hydrophone placed below water from another ship 5 km apart 11s earlier than the sound reaching the deck of the ship. What is the velocity of sound in water? Given that the velocity of sound in air = 348 m · s^-1.
Solution:

Distance between the two ships = 5 km = 5000 m

So time taken by the sound to come through air = \(\frac{5000}{348} \mathrm{~s}\)

According to the question, time is taken by the sound to come through water = \(\frac{5000}{348}-11=\frac{5000-3828}{348}=\frac{1172}{348} \mathrm{~s}\)

Therefore, the velocity of sound in water

= \(\frac{5000}{\frac{1172}{348}}=1485 \mathrm{~m} \cdot \mathrm{s}^{-1}\) (approx.)

Example 2. If one end of a long steel pipe is struck, the sound is heard twice at an interval of 3 s at the other end. What is the length of the pipe? (The velocities of sound in air = 350m · s^-1, in steel = 5000 m · s^-1)
Solution:

Let the length of the pipe be l m.

So, time is taken by the sound to come through the air in the pipe = \(\frac{l}{350}\)s

Again, time taken by die sound to come through steel =\(\frac{l}{5000}\)s

According to the question, \(\frac{l}{350}-\frac{l}{5000}=3 \quad \text { or, } l\left(\frac{1}{350}-\frac{1}{5000}\right)=3\)

or, \(l\left(\frac{100-7}{35000}\right)=3\)

or, \(l=\frac{35000 \times 3}{93}=1129 \mathrm{~m}=1.129 \mathrm{~km} .\)

Example 3. Sound moves through a liquid with a velocity 1340 m · s^-1. If the density of the liquid is 0.8 g · cm^-3, determine the compressibility of the liquid.
Solution:

We know, c = \(\sqrt{\frac{k}{\rho}}\); k = bulk modulus of the liquid

∴ k = c²ρ [c = 1340 m · s^-1 = 134 x 10³ cm · s^-1]

Again, compressibility = \(\frac{1}{k}=\frac{1}{c^2 \rho}\)

= \(\frac{1}{\left(134 \times 10^3\right)^2 \times 0.8}=\frac{1}{143648} \times 10^{-5}\)

= \(6.96 \times 10^{-11} \mathrm{~cm}^2 \cdot \mathrm{dyn}^{-1}\)

Wave Motion Musical Sound And Noise

Musical sound: The sound produced due to regular and periodic vibrations, that is pleasing to hear, is called musical sound. While singing, the vocal cords of a singer vibrate with regular periodic motion.

• Similarly when die strings of a sitar are excited to produce a certain note, diey vibrate with regular periodic motion. The sound of clapping is usually unpleasant, but rhythmic clapping of hands may produce a pleasant sound.
• This is due to the regular vibration of the source. Similarly pattern of raindrops on tin roofs or the clickers’ sound of train wheels may be sonorous.

Noise: The sound produced by an irregular or short-lived vibration is called noise. The explosion of a cracker, the firing of a gun, the horn of a car, etc., are noises.

Tone: A musical sound of a single frequency is called a tone. When the source of sound vibrates in SHM, it produces a tone. The two prongs of a tuning fork vibrate in SHM and produce the sound of a single frequency. Thus, the sound of a tuning fork is a pure tone.

Tone note: A musical sound due to a mixture of more than one frequency is called a note. The sound produced by different musical instruments consists, in general, of more than one tone. If a tone is compared with monochromatic light such as red light, a note can be compared with compound light, i.e., white light. The tones within a note are classified on the basis of their frequencies.

• Fundamental Tone: The tone with the lowest frequency present in a note is called the fundamental tone.
• Overtones: The tones in a note, other than the fundamental tone, are called overtones.
• Harmonics: Harmonics are tones having frequencies that are integral multiples of that of the fundamental tone. The fundamental tone is also a harmonic.
• Octave: A tone whose frequency is twice that of the fundamental tone is said to be the octave of the fundamental tone.

Suppose, a musical note consists of tones of frequencies 200 Hz, 300 Hz, 400 Hz, 500 Hz, and 600 Hz. So it can be said:

1. Each of these five different frequencies corresponds to a tone,
2. The combination of these five tones is a note,
3. The tone of 200 Hz is die fundamental tone,
4. The tones having frequencies 300 Hz, 400 Hz, 500 Hz, and 600 Hz are overtones,
5. Both of the tones of frequencies 400 Hz and 600 Hz are harmonics, as the frequencies are 2 and 3 umes that of the fundamental tone respectively. The tone of 400 Hz is called the second harmonic as its frequency is 2 times of the fundamental tone. Similarly, the tone of 600 Hz is called the third harmonic. The fundamental tone is called the first harmonic.
6. The tone of 400 Hz is the octave because this frequency is the mice die frequency of the fundamental tone.

Characteristics Of Musical Sound Or Note: There are three characteristics that differentiate musical notes. These are loudness, pitch, and quality.

1. Loudness: Loudness Is related to the sound energy reaching our ears per unit time. If the sound energy reaching our ears in 1 second goes up, we perceive a corresponding increase in the loudness.
2. Pitch: It is that characteristic that differentiates a sharp or shrill sound from a grave one. It increases with the Increase in frequency of the source.
3. Quality Or Timbre: It is that characteristic of a musical note that enables us to distinguish between a note emitted by one musical instrument from a note of the same loudness and pitch emitted by another instrument. It depends on the number of overtones present in a note and their relation with the fundamental tone.

Wave Motion Conclusion

A wave is a disturbance that travels through a medium, but the particles constituting the medium do not travel.

• The disturbance that travels through a material medium due to a collective vibration of the particles of the medium is known as a mechanical wave.
• It is an elastic wave if the particles vibrate due to the elasticity of the medium. If the motion of the particles of a medium is simple harmonic, the corresponding wave is known as a simple harmonic wave.
• A wave, that propagates in a direction perpendicular to the direction of motion of the vibrating particles of the medium, is called a transverse wave.
• A wave, that propagates along the direction of motion of the vibrating particles of the medium, is called a longitudinal wave.
• All longitudinal waves are mechanical waves. Longitudinal waves can be transmitted through any solid, liquid, or gaseous medium. This type of wave cannot propagate without any medium.
• Transverse elastic waves cannot be produced in liquids or gases but can be created in solids.

Some physical quantities related to waves:

1. Phase: The quantity from which the motion of a wave, except the amplitude, can be known completely is called the phase of the wave.

2. Complete Wave: The wave in between two consecutive particles having the same phase at an instant is known as a complete wave.

3. Wavelength: The length of a complete wave, i.e., the distance between two consecutive particles having the same phase at an instant is called the wavelength of the wave.

4. Time period: The time required to create a complete wave, i.e., the time taken by a wave to cover the distance between two consecutive particles having the same phase of vibration is called the time period of the wave.

5. Frequency: The frequency of a wave is the number of complete waves produced in unit time.

6. Amplitude: The amplitude of a wave is the maximum displacement from the mean position of a particle-producing tire wave.

7. Wave velocity: The distance traversed by a wave in unit time is called the wave velocity.

8. Wavefront: A surface normal to the direction of propagation of a wave is known as a wavefront. Particles lying on a wavefront have the same phase.

9. Ray: A normal drawn on a wavefront is called a ray.

The velocity with which sound wave propagates in a material medium depends on two properties of the medium density, and elasticity.

• Newton assumed that the propagation of sound through a gaseous medium is an isothermal process.
• According to Laplace, the propagation of sound through a gaseous medium is an adiabatic process.
• The pressure of a gas has no effect on the velocity of sound.
• The velocity of sound in a gas is directly proportional to the square root of its absolute temperature.
• The velocity of sound in air increases by 0.61 m · s^-1 or 61 cm · s^-1 for 1°C rise in temperature.
• The velocity of sound in moist air is greater than that in dry air.
• The velocity of sound in a gas is inversely proportional to the square root of its density.
• To obtain regular reflection of sound, the reflector must be large but the surface of the reflector need not be very smooth.
• If the reflected sound is heard separately from an original sound, it is called an echo of the original sound.
• The sensation of an inarticulate sound (sound produced by gunshot, clapping, etc.) persists in our ear for about \(\frac{1}{10}\)th of a second. This is known as the persistence of hearing.
• The velocity of sound in air at 0°C is about 330 m · s^-1.

Wave Motion Useful Relations for Solving Numerical Problems

Relation between time period (T) and frequency (n): T = \(\frac{1}{n}\)

Relation between wavelength (λ) and wave number (k): \(\lambda=\frac{2 \pi}{k}\)

Relation between frequency (n) and angular frequency ω:ω = 2πn

Wave velocity (V) = frequency (n) x wavelength (λ)

Equation of a progressive wave moving along the positive and negative direction of the x-axis:

y = \(A \sin \omega\left(t \mp \frac{x}{V}\right)=A \sin (\omega t \mp k x)\)

= \(A \sin \frac{2 \pi}{\lambda}(V t \mp x)=A \sin 2 \pi\left(\frac{t}{T} \mp \frac{x}{\lambda}\right)\)

If The equation of a progressive wave can be expressed using cosine function also.

Physical quantities related to the motion of a particle in a progressive wave:

\(\begin{array}{|l|l|}
\hline \text { Displacement } & y=A \sin (\omega t-k x \pm \phi) \\
\hline \text { Velocity } & \nu=\frac{d y}{d t}=\omega A \cos (\omega t-k x \pm \phi) \\
\hline \text { Max. velocity } & \pm \omega A \\
\hline \text { Acceleration } & a=\frac{d^2 y}{d t^2}=-\omega^2 A \sin (\omega t-k x \pm \phi) \\
\hline \text { Max, acceleration } & \pm \omega^2 A \\
\hline
\end{array}\)

 

If the particle velocity is v and the wave velocity is V, then \(\nu=-V \frac{\partial y}{\partial x}\)

Velocity of Progressive waves in different media:

\(\begin{array}{|l|l|l|}
\hline \begin{array}{l}
\text { Velocity of a long- } \\
\text { tidal wave in a } \\
\text { solid medium }
\end{array} & V=\sqrt{\frac{Y}{\rho}} & \begin{array}{l}
Y=\text { Young’s modulus of } \\
\text { the medium and } \rho= \\
\text { density of the medium }
\end{array} \\
\hline \begin{array}{l}
\text { Velocity of a long- } \\
\text { tidal wave in a } \\
\text { liquid or a gaseous } \\
\text { medium }
\end{array} & V=\sqrt{\frac{E}{\rho}} & \begin{array}{l}
E=\text { bulk modulus of the } \\
\text { medium and } \rho=\text { density } \\
\text { of the medium }
\end{array} \\
\hline
\end{array}\) \(\begin{array}{|c|c|c|}
\hline \begin{array}{l}
\text { Velocity of sound } \\
\text { wave in a gaseous } \\
\text { medium }
\end{array} & V=\sqrt{\frac{\gamma p}{\rho}} & \begin{array}{l}
p=\text { pressure of the gas, } \\
\gamma=c_p / c_\nu=\text { ratio of the } \\
\text { two specific heats of the } \\
\text { gas and } \rho=\text { density of } \\
\text { the gas }
\end{array} \\
\hline \begin{array}{l}
\text { Velocity of a trans- } \\
\text { verse wave in a } \\
\text { stretched string }
\end{array} & V=\sqrt{\frac{T}{m}} & \begin{array}{l}
T=\text { tension in the string } \\
\text { and } m=\text { mass per unit } \\
\text { length of the string }
\end{array} \\
\hline
\end{array}\)

 

If M is the mass of 1 mol of a gas, then c = \(\sqrt{\frac{\gamma R T}{M}}\)

where T = absolute temperature of the gas,

R = universal gas constant

If c₀ and c are the velocities of sound at 0°C and t °C respectively, then c = c₀(1+ 0.001830 t)

If the densities of two different gases at the same temperature and pressure are ρ₁ and ρ₂ and the velocities of sound in the two gases are c₁ and c₂ respectively, then \(\frac{c_1}{c_2}=\sqrt{\frac{\rho_2}{\rho_1}}\)

If the distance of a reflector from the source of sound is D, the time required to hear the echo is t, and the velocity of sound is V, then, 2D = Vt

Wave Motion Very Short Answer Type Questions

Question 1. What kind of energy is transmitted through an elastic wave?
Answer: Mechanical energy

Question 2. Give an example of an elastic wave.
Answer: Soundwave

Question 3. Is It possible for a transverse wave to propagate in a liquid?
Answer: No

Question 4. What kind of wave is an X-ray?
Answer: Electromagnetic

Question 5. If the direction of motion of a wave and the direction of vibration of the particles of a medium are parallel to each other, the wave is called a transverse wave.
Answer: False

Question 6. If the direction of motion of a wave and the direction of vibration of the particles of a medium are perpendicular to each other, the wave is called a ______ wave.
Answer: Transverse

Question 7. During propagation of sound, compressions and rarefactions occur so rapidly that the _______ of the gas cannot remain constant.
Answer: Temperature

Question 8. If λ is the wavelength of a transverse wave, what will be the distance between two consecutive crests?
Answer: λ

Question 9. If λ is the wavelength of a longitudinal wave, what will be the length of a compression?
Answer: \(\frac{\lambda}{2}\)

Question 10. If the velocity of sound is 330 m · s^-1, what will be the wavelength of the sound wave emitted from a tuning fork of frequency 220 Hz?
Answer: 1.5 m

Question 11. The time required to form a complete wave is called the time period of the wave. Is the statement true or false?
Answer: True

Question 12. What is the number of complete waves formed in unit time called?
Answer: Frequency

Question 13. The maximum displacement of a particle on the wave from its mean position is called the _______ of the wave.
Answer: Amplitude

Question 14. The surface of vibration of the particles of a medium having the same phase during the propagation of a wave is called the ________ of the wave.
Answer: Wavefront

Question 15. The frequency of a progressive wave x = velocity of the wave.
Answer: Wavelength

Question 16. The equation of a progressive wave is given by y = Acos\(\frac{2 \pi}{\lambda}\)(Vt-x). Find the frequency of the wave.
Answer: \(\frac{V}{\lambda}\)

Question 17. The equation of a progressive wave is given by y = \(4 \sin 20 \pi\left(t-\frac{x}{100}\right) \mathrm{cm}\). Find the amplitude of the wave.
Answer: 4cm

Question 18. The equation of a progressive wave is given by y = \(4 \sin 20 \pi\left(t-\frac{x}{100}\right) \mathrm{cm}\). Find the frequency of the wave.
Answer: 10 Hz

Question 19. The equation of a progressive wave is given by \(y=4 \sin 20 \pi\left(t-\frac{x}{100}\right) \mathrm{cm}\). Find the velocity of the wave.
Answer: 100 cm · s^-1

Question 20. Why is the sound heard in CO₂ more intense in comparison to the sound heard in the air?
Answer: Density more

Question 21. By what process does the propagation of sound through a gaseous medium take place?
Answer: Adiabatic

Question 22. What is the ratio of the velocities of sound through hydrogen and oxygen at STP?
Answer: 4: 1

Question 23. If the temperature remains constant, what will be the bulk modulus of a gas?
Answer: Equal to the pressure of the gas

Question 24. Why is the velocity of sound greater in deep water than that on its surface?
Answer: Pressure increases with the depth of water, velocity of sound increases with the increase of pressure

Question 25. Why does a violinist return his instrument on entering a warm room?
Answer: Due to an increase in temperature velocity increases

Question 26. If temperature is kept constant, the velocity of sound in a gas is independent of which of the following properties? Pressure, density, or wind flow.
Answer: Pressure

Question 27. Is the velocity of sound in dry air at constant temperature, less or greater than that in moist air?
Answer: Less

Question 28. The equation of a progressive wave is given by y = \(A \cos \omega\left(t-\frac{x}{V}\right)\). What will be the frequency and amplitude of the wave?
Answer: \(\left[\frac{\omega}{2 \pi}, A\right]\)

Question 29. What should be the minimum time interval between an original sound and its echo, so that the echo is heard separately?
Answer: \(\frac{1}{10}\)s

Question 30. The velocity of sound in air is 330 m • s^-1. What should be the minimum distance of a reflector so that a listener can hear the echo distinctly?
Answer: 16.5 m

Question 31. In a big hall, sound persists even after the original sound is stopped. What is the phenomenon called?
Answer: Reverberation

Question 32. If a sound wave enters water from the air, is the angle of refraction less or greater than the angle of incidence?
Answer: Greater

Wave Motion Assertion Reason Type Questions And Answers

Direction: These questions have statement 1 and statement 2. Of the four choices given below, choose the one that best describes the two statements.

1. Statement 1 is true, statement 2 is true; statement 2 is a correct explanation for statement 1.
2. Statement 1 is true, and statement 2 is true; statement 2 is not a correct explanation for statement 1.
3. Statement 1 is true, statement 2 is false.
4. Statement 1 is false, and statement 2 is true.

Question 1.

Statement 1: A sound wave is regarded as a pressure wave.

Statement 2: Energy in this type of wave is transported due to the formation of compression and rarefaction in the medium in which pressure difference is created.

Answer: 1. Statement 1 is true, statement 2 is true; statement 2 is a correct explanation for statement 1.

Question 2.

Statement 1: Any function of space and time that satisfies the following equation represents a wave \(\frac{d^2 y}{d x^2}=\frac{1}{V^2} \frac{d^2 y}{d t^2}\)

Statement 2: y s Asin ωt, y = Acosωt do not satisfy the above equations and hence do not represent waves.

Answer: 2. Statement 1 is true, and statement 2 is true; statement 2 is not a correct explanation for statement 1

Wave Motion Match The Columns

Question 1. A wave is transmitted from a denser to a rarer medium.

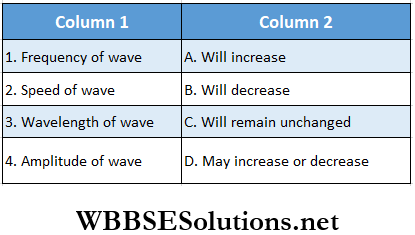
Answer: 1. C, 2. A, 3. A, 4. A

Question 2. Three traveling sinusoidal waves on identical strings have the same tension. The mathematical form of the waves are \(y_1=A \sin (3 x-6 t), y_2=A \sin (4 x-8 t) and y_3=A \sin (6 x-12 t)\).

Answer: 1. D, 2. A, 3. B, 4. C

Wave Motion Comprehension Type Questions And Answers

Read the following passages carefully and answer the questions at the end of them.

Question 1. Represents two snaps of a traveling wave on a string of mass per unit length μ = 0.25 kg · m^-1. The first snap is taken at t = 0 and the second is taken at t = 0.053 s.

1. The speed of the wave is

1. \(\frac{20}{3}\) m · s^-1
2. \(\frac{10}{3}\) m · s^-1
3. 20 m · s^-1
4. 10 m · s^-1

Answer: 2. \(\frac{10}{3}\) m · s^-1

2. The frequency of the wave is

1. \(\frac{5}{3}\) Hz
2. \(\frac{10}{3}\) Hz
3. 5 Hz
4. 10 Hz

Answer: 1. \(\frac{5}{3}\) Hz

3. The maximum speed (in m · s^-1) of the particle is

1. \(\frac{5 \pi}{13}\)
2. \(\frac{5 \pi}{13}\)
3. \(\frac{\pi}{30}\)
4. \(\frac{7 \pi}{20}\)

Answer: 3. \(\frac{\pi}{30}\)

Question 2. A sinusoidal wave is propagating in a negative x -x-direction in a string stretched along the x-axis. A particle of string at x = 2 cm is found at its mean position and it is moving in a positive y-direction at t = 1s. The amplitude of the wave, the wavelength, and the angular frequency of the wave are 0.1 m, \(\frac{\pi}{4}\) m and 4π rad • s^-1 respectively,

1. The equation of the wave is

1. y = 0.1sin[47π(t- 1) + 8(x-2)]
2. y = 0.1 sin [(t- 1) – (x-2)]
3. y = 0.1sin [4π(t- 1)-8(x-2)]
4. None of these

Answer: 1. y = 0.1sin[47π(t- 1) + 8(x-2)]

2. The speed of particle at x = 2m and t = 1 s is

1. 0.2π m · s^-1
2. 0.67π m · s^-1
3. 0.4π m · s^-1
4. 0

Answer: 3. 0.4π m · s^-1

3. The instantaneous power (in J · s^-1) transfer through x = 2 m and t = 1.125 s is

1. 10
2. \(\frac{4 \pi}{3}\)
3. \(\frac{2 \pi}{3}\)
4. Zero

Answer: 4. Zero

Question 3. Suppose a musical note consists of tones of frequencies 100 Hz, 200 Hz, 300 Hz, 400 Hz, 500 Hz, and 600 Hz.

1. What should be the frequency of the fundamental tone?

1. 200 Hz
2. 100 Hz
3. 400 Hz
4. 600 Hz

Answer: 2. 100 Hz

2. Among the frequencies the third and fourth harmonics are respectively

1. 300 Hz, 500 Hz
2. 400 Hz, 500 Hz
3. 300 Hz, 400 Hz
4. None of these

Answer: 3. 300 Hz, 400 Hz

3. Among the tones the octave is

1. 200 Hz
2. 300 Hz
3. 400 Hz
4. None

Answer: 1. 200 Hz

Wave Motion Integer Answer Type Questions

In this type, the answer to each of the questions Is a single-digit integer ranging from 0 to 9.

Question 1. A transverse wave propagating along x -axis is represented by (x, t) = 8.0sin (0.5π x – 4πt – \(\frac{\pi}{4}\)) where x is in meters and t is in seconds. Calculate the speed in m µ s^-1 of the wave.
Answer: 8

Question 2. The water waves are traveling along the surface of an ocean at a speed of 2.5 m · s^-1 and splashing up periodically against a pole. Each adjacent crest is 5 m apart. The crest splashes upon reaching the foot of the pole. How much time (in seconds) passes between each successive splashing?

Answer: 2