WBCHSE Class 11 Physics Notes For Vectors

Kinematics Vector

Definition Of Scalar And Vector Quantities

Physical quantities, used in science and technology are broadly classified into two groups, scalar quantities and vector quantities.

Scalar Quantities Definition: A scalar quantity is a physical quantity having only magnitude but no direction.

Physical quantities like length, mass, time, relative density, energy, temperature, etc. are fully described by their magnitudes. These quantities do not have any direction. These are examples of scalar quantities.

Scalar Definition: Any number, that has a real magnitude only but no direction, is called a scalar.

Essentially, any real number—like 8, -2, √3, etc. is a scalar. Naturally, if an appropriate unit is added to a scalar, it becomes a scalar quantity.

Scalar Example:

1. The distance of a railway station is 10 km from my residence.
2. It takes me 30 minutes (time) to reach school from my home.

In all the above examples we can see that a scalar quantity is completely expressed by a real number and a unit and thus have complete information about the quantities.

Mathematical operations of scalars follow simple algebraic rules.

Read and Learn More: Class 11 Physics Notes

Vector Quantities Definition A vector quantity is defined as a physical quantity, having both magnitude and direction.

• For physical quantities like displacement, velocity, acceleration, force, etc. the magnitude does not define the quantity fully. If we express the position of our school by saying that it is 4 km away from my residence then this statement is incomplete.
• The school cannot be located until we say that it is 4 km west of my residence. Thus position is a vector quantity. A statement such as ‘the bus stop is 200 m from where one is standing’ may not be useful until a direction is specified, like 200 m east.

Vector Definition: Any number, that has a real magnitude as well as a direction, is called a vector.

A vector quantity is expressed by a real number, a unit, and a specific direction.

Vector Example: Velocity of a particle = 10 m · s^-1 towards the east. If the unit is omitted, we get a vector. In this example, the vector is ‘10 towards east’.

5 towards the south, -8 downwards, \(\frac{1}{2}\) along the north-west, 2√2 from the south-west, etc. are examples of vectors. Obviously, the vector ‘-8 downwards’ is identical to the vector ‘8 upwards’. If an appropriate unit is added to a vector, it becomes a vector quantity.

Differences Between Scalar And Vector Quantities:

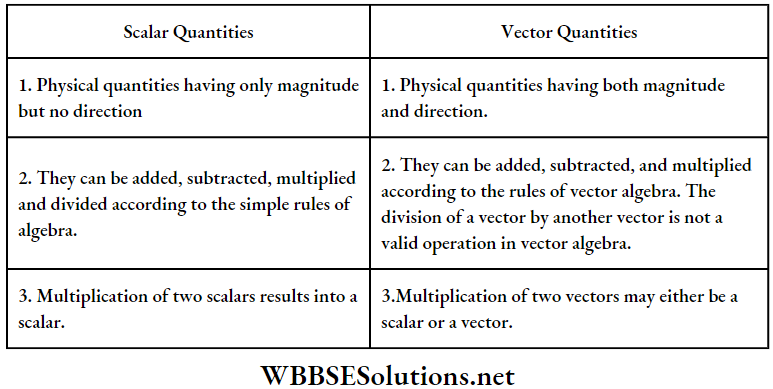
It is to be noted that, scalars and vectors are mathematical elements only. After all mathematical operations, a proper unit must be added to the final result to obtain a meaningful physical quantity.

Geometrical Representation Of A Vector

A vector is represented by a line segment with an arrowhead. The length of the line segment (in a predetermined scale), is the magnitude of the vector and the arrowhead denotes the direction. The front end (carrying the arrow) is called the head and the rear end is called the tail.

The velocity of a particle 6 cm • s^-1 towards the east is represented by the line segment AB. If the scale is chosen such that CD =0.5 in. represents 2cm · s^-1, then the length of the segment AB should be 1.5 in.

So, the 1.5 in. long line segment AB with the arrowhead pointing towards the east represents both the magnitude and direction (towards the east) of the velocity of the particle. The velocity vector is represented as \(\overrightarrow{A B}\), where A is the initial point and B is the terminal point.

Generally, a vector is expressed as an algebraic quantity by using a letter with an arrowhead like \(\vec{a}, \vec{b}, \vec{c},\), etc. The magnitude or absolute value of a vector is a scalar and is always positive. It is called the modulus of the vector. It is expressed as a, b, c (without an arrow) or \(|\vec{a}|,|\vec{b}|,|\vec{c}|\).

Modulus of the velocity vector \(\overrightarrow{A B}\), as shown.

Therefore, \(|\stackrel{\rightharpoonup}{\nu}|=|\overrightarrow{A B}|=A B \text { (length) }=6 \mathrm{~cm} \cdot \mathrm{s}^{-1}\)

Some Facts About Vectors

Equal Vectors: Two vectors, equal in magnitude as well as in direction, are called equal vectors. \(\overrightarrow{A B}\) and \(\overrightarrow{C D}\) are equal vectors as both have the same magnitude (length) and direction.

If \(\overrightarrow{A B}\) represents 20 towards the north, then \(\overrightarrow{C D}\) will also represent 20 towards the north. If \(\overrightarrow{A B}\) = \(\vec{a}\), then \(\overrightarrow{C D}\) is also = \(\vec{a}\), i.e., \(\overrightarrow{A B}\) = \(\overrightarrow{C D}\).

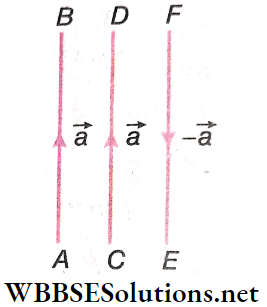
Two vectors may be equal even when their initial and final points are not the same. This means that we can translate a vector keeping its magnitude and direction unchanged.

Opposite Vectors: Two vectors having the same absolute value but opposite directions are called opposite vectors. \(\overrightarrow{F E}\) and \(\overrightarrow{A B}\) or \(\overrightarrow{C D}\) are opposite vectors.

∴ \(\overrightarrow{F E}\) represents a vector with a magnitude same as that of \(\overrightarrow{A B}\) or \(\overrightarrow{C D}\) = a (say), but the direction of \(\overrightarrow{F E}\) is opposite to that of \(\overrightarrow{A B}\) or \(\overrightarrow{C D}\).

Hence, if \(\overrightarrow{A B}\) = \(\overrightarrow{C D}\) = \(\vec{a}\), then \(\overrightarrow{F E}\) = –\(\vec{a}\). It may also be written as \(\overrightarrow{A B}\) = \(\overrightarrow{C D}\) = –\(\overrightarrow{F E}\).

As magnitudes or moduli of two opposite vectors are the same, we have, \(|\vec{a}|=a,|-\vec{a}|=a\).

Collinear Vectors: Vectors that are of the same or different magnitudes, but jure parallel or anti-parallel to one another, are known as collinear vectors. \(\vec{d}, \vec{e}, \vec{f}\) are collinear vectors. Also \(\vec{x}, \vec{y}, \vec{z}\) acting along the same line, represent a set of collinear vectors.

Coplanar Vectors: Vectors lying on the same plane are coplanar vectors. \(\vec{a}\) and –\(\vec{a}\) and all vectors lie on the plane of the paper and hence are coplanar.

Unit Vector: A vector in the direction of a given vector with unit magnitude is called a unit vector. A unit vector is often denoted by a lowercase letter with circumflex or ‘hat’ (^).

The absolute value of any vector is a scalar. This scalar, multiplied by the unit vector in that direction, gives the corresponding vector.

Unit Vector Example: \(|\vec{A}|\) = A and \(A \hat{n}=\vec{A}\) where \(\hat{n}\) is the unit vector in the direction of \(\vec{A}\). This means \(\frac{\vec{A}}{A}=\hat{n}\).

Therefore a vector divided by its magnitude gives the unit vector in the direction of that vector. In the cartesian coordinate system, unit vectors along x, y, and z axes are conventionally represented as \(\hat{i}, \hat{j} \text { and } \hat{k}\) respectively.

Composition Of Scalars And Vectors

Scalars have only magnitudes. So addition or subtraction of scalars means the addition or subtraction of their magnitudes only. Accordingly, scalar addition follows simple algebraic rules.

Vectors have both magnitude and direction. Therefore, during addition or subtraction of vectors, their directions should be taken into account as well. Thus, they cannot be added or subtracted by using simple algebraic rules. Hence, the geometrical method or analytical method of vector algebra is used for vector addition.

Addition Or Two Vectors

Suppose a particle starting from its initial position O undergoes \(\vec{a}\) displacement a and reaches point A. Hence, \(\vec{a}\) = \(\overrightarrow{O A}\). After a further displacement of \(\vec{b}\) in a  different direction, the particle reaches point B.

Hence, \(\vec{a}\) = \(\overrightarrow{A B}\). Starting from point O, the net displacement of the particle is thus \(\overrightarrow{O B}\) = \(\vec{c}\) (say).

Vector \(\vec{c}\) is called the sum or the resultant of \(\vec{a}\) and \(\vec{b}\), expressed as \(\vec{c}\) = \(\vec{a}\) + \(\vec{b}\).

This sum will obviously be a vector sum, because by adding only the magnitudes of \(\vec{a}\) and \(\vec{b}\), \(\vec{c}\) cannot be found. The magnitudes of \(\vec{a}\), \(\vec{b}\) and \(\vec{c}\) can be determined from the lengths of the sides OA, AB and OB respectively.

This method of finding the resultant of two displacements also holds good for finding the sum of any two vectors. Illustrates one such case where forces \(\vec{P}\) and \(\vec{Q}\) pull a boat simultaneously. As a result, the boat moves along the resultant \(\vec{R}\), and \(\vec{P}\) + \(\vec{Q}\) = \(\vec{R}\).

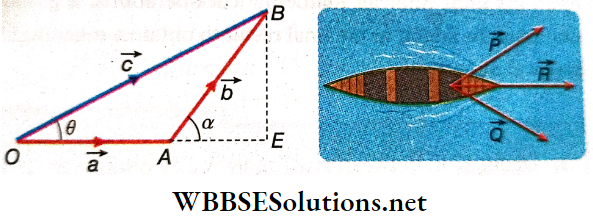
Addition Of Two Vectors Resultant: A single vector, which represents the result of the summation of a number of vectors both in magnitude and in direction, is called the resultant of those vectors.

∴ \(\overrightarrow{O B}\) is the resultant.

To find the sum of \(\vec{P}\) and \(\vec{Q}\), draw the vectors \(\vec{P}\) as shown and name it as AB i.e., \(\overrightarrow{A B}\) = \(\vec{P}\).

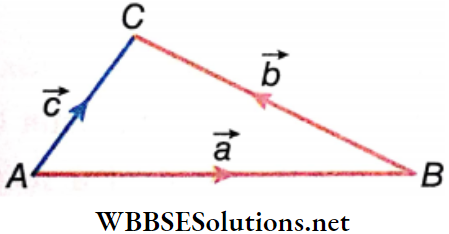
Now move the vector \(\vec{Q}\) parallel to itself such that its tail coincides with the tip B of the vector \(\vec{P}\).

Mark the tip of the vector \(\vec{Q}\) as C i.e., \(\overrightarrow{B C}\) = \(\vec{Q}\). Join the tail of the vector \(\vec{P}\) to the tip of the vector \(\vec{Q}\) i.e., \(\overrightarrow{A C}\) = \(\vec{R}\) where \(\vec{Q}\) represents the sum of the vectors \(\vec{P}\) and \(\vec{Q}\). Thus, \(\vec{R}\) = \(\vec{P}\) + \(\vec{Q}\).

Vector addition follows either of the following equivalent laws:

1. Law of triangle of vectors,
2. Law of parallelogram of vectors and
3. Polygon law of vectors.

Magnitude In vector Addition: Let us consider two vectors a and b whose resultant is found to be \(\vec{c}\) i.e., \(\vec{a}\) + \(\vec{b}\) = \(\vec{c}\). Now to think, that the magnitude of \(\vec{c}\) is actually the sum of the magnitude of \(\vec{a}\) and magnitude of \(\vec{b}\) is incorrect. This is because we can see that \(|\vec{c}|<|\vec{a}|+|\vec{b}|\).

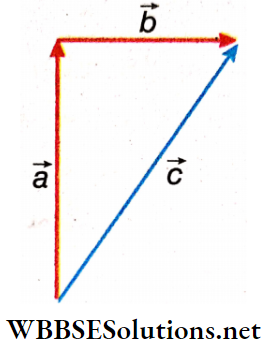
It means that the magnitude of resultant vector \(\vec{c}\) not only depends on the magnitude of \(\vec{c}\) and \(\vec{c}\) but also depends on the angle between the two vectors \(\vec{a}\) and \(\vec{b}\).

To find out the relationship between \(\vec{a}\), \(\vec{b}\), \(\vec{c}\) and the angle between the vectors, let us study the following law of vector addition.

Law Of Triangle Of Vectors

Law Of Triangle Of Vectors Geometrical Method: \(\vec{a}\) + \(\vec{b}\) = \(\vec{c}\) or \(\overrightarrow{O A}+\overrightarrow{A B}=\overrightarrow{O B}\). Vectors \(\overrightarrow{O A}, \overrightarrow{A B} \) and their resultant \(\overrightarrow{O B}\), therefore represent the three sides of the A OAB. The following triangle law of vector addition can be obtained by considering the magnitudes and directions of the vectors.

Law Of Triangle Of Vectors Geometrical Method Statement: When the magnitudes and directions of two vectors, are represented by two adjacent sides of a triangle taken in order, the third side taken in the opposite order, represents the magnitude and direction of the resultant of the two vectors.

Two adjacent sides taken in order and the third side taken in the opposite order—means that if the former is in a clockwise direction in the triangle, the third side, representing the resultant, should be in an anticlockwise direction.

Law Of Triangle Of Vectors Geometrical Method Corollary: With reference to the A OAB, \(\overrightarrow{O A}+\overrightarrow{A B}=\overrightarrow{O B}\) or, \(\overrightarrow{O A}+\overrightarrow{A B}-\overrightarrow{O B}=0\)

or, \(\overrightarrow{O A}+\overrightarrow{A B}+\overrightarrow{B O}=0\)

Hence, if three vectors are completely represented by the three sides of a triangle taken in order (all clockwise or all anticlockwise), then the resultant of the vectors will be zero.

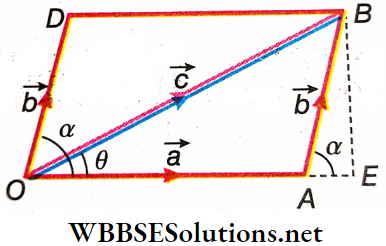
Law Of Triangle Of Vectors Analytical Method: Let the two vectors \(\vec{a}\) and \(\vec{b}\) be represented both in magnitude and direction by the sides \(\overrightarrow{O A}\) and \(\overrightarrow{A B}\) of ΔOAB is taken in the same order. Then according to the triangle law of vector addition, the resultant \(\vec{c}\) is given by the side OB taken in the reverse order, as shown.

Law Of Triangle Of Vectors Analytical Method Magnitude Of The Resultant \(\vec{c}\): BE is the perpendicular drawn from B on the extension of OA. The angle between the vectors \(\vec{a}\) and \(\vec{b}\), ∠BAE = α. It is to be noted that to measure the angle between two vectors, their initial points are superimposed on each other without changing the directions of the vectors.

Now from right-angled ΔAEB we have \(BE \frac{B E}{A B}=\sin \alpha\)

or, \(B E=A B \sin \alpha\)

or, \(B E=b \sin \alpha\) and \(\frac{A E}{A B}=\cos \alpha\) or, \(A E=A B \cos \alpha\)

or, \(A E=b \cos \alpha\)

Applying Pythagoras theorem in right-angled ΔOEB we get, \((O B)^2=(O E)^2+(E B)^2=(O A+A E)^2+(E B)^2\)

or, \(c^2=(a+b \cos \alpha)^2+(b \sin \alpha)^2\)

= \(a^2+2 a b \cos \alpha+b^2 \cos ^2 \alpha+b^2 \sin ^2 \alpha\)

or, \(c^2=a^2+2 a b \cos \alpha+b^2\left(\cos ^2 \alpha+\sin ^2 \alpha\right)\)

or, \(c^2=a^2+b^2+2 a b \cos \alpha\) (because \(\cos ^2 \alpha+\sin ^2 \alpha=1\))

or \(c=\sqrt{a^2+b^2+2 a b \cos \alpha}\)….(1)

Law Of Triangle Of Vectors Analytical Method Direction Of The Resultant \(\vec{c}\): Let the resultant vector (\(\vec{c}\)) make an angle α with the first vector \(\vec{a}\). Then from right-angled ΔOEB, we get, \(\tan \theta=\frac{B E}{O E}=\frac{B E}{O A+A E}\)

or, \(\tan \theta=\frac{b \sin \alpha}{a+b \cos \alpha}\)….(2)

Therefore, if the magnitudes a and b, and the value of the angle α are known, then from equations (1) and (2), the magnitude of the resultant vector and its direction can be determined.

Law Of Parallelogram Of Vectors

Law Of Parallelogram Of Vectors Geometrical Method: The law of parallelogram of vector addition is just an alternative form of the law of triangle of vector addition. let \(\overrightarrow{O A}=\vec{a}\) and \(\overrightarrow{A B}=\vec{b}\).

Therefore, from the triangle law of vector addition, the vector \(\overrightarrow{O B}\) represents the magnitude and direction of the resultant \(\vec{c}\). Hence, \(\vec{a}+\vec{b}=\vec{c}\) or, \(\overrightarrow{O A}+\overrightarrow{A B}=\overrightarrow{O B}.\)

Now the parallelogram OABD is completed. AB and OD being the opposite sides of the parallelogram, are equal and parallel.

Hence, \(\overrightarrow{A B}=\overrightarrow{O D}=\vec{b}\).

So, \(\overrightarrow{O A}+\overrightarrow{O D}=\overrightarrow{O B}\)

Law Of Parallelogram Of Vectors Geometrical Method Statement: If the magnitudes and directions of two vectors are represented by two adjacent sides of a parallelogram, the diagonal drawn from the point of origin of the two vectors represents the magnitude and direction of the resultant.

Law Of Parallelogram Of Vectors Analytical Method:

Law Of Parallelogram Of Vectors Analytical Method Magnitude Of Resultant \(\vec{c}\): Let the point of origin of the vectors \(\vec{a}\) and \(\vec{b}\) be O. The two vectors are represented by the line segments OA and OD.

The parallelogram OABD is completed by drawing DB parallel to OA and AB parallel to OD. Then the diagonal OB from point 0 represents the magnitude and direction is the resultant of the vectors \(\vec{a}\) and \(\vec{b}\).

Here, \(\vec{a}=\overrightarrow{O A}, \vec{b}=\overrightarrow{O D}=\overrightarrow{A B} \text { and } \vec{c}=\overrightarrow{O B}\)

Perpendicular BE is drawn from B on the extension of OA. Then ∠BAE = ∠DOA = α

Here, the length of the side OA = \(|\vec{a}|\) = a

length of the side OD = length of the side AB = \(|\vec{b}|\) = b and length of the side OB = \(|\vec{c}|\) = c

From right angled ΔBAE we have, \(\frac{B E}{A B}=\sin \alpha\) or, BE = \(A B \sin \alpha\)

∴ BE = \(b \sin \alpha\)

Again, \(\frac{A E}{A B}=\cos \alpha\) or, \(A E=A B \cos \alpha\)

∴ AE = \(b \cos \alpha\)

Using Pythagoras theorem in right-angled ΔOEB, we get, \((O B)^2=(O E)^2+(B E)^2=(O A+A E)^2+(B E)^2\)

or, \(c^2=(a+b \cos \alpha)^2+(b \sin \alpha)^2\)

or, \(c^2=a^2+b^2 \cos ^2 \alpha+2 a b \cos \alpha+b^2 \sin ^2 \alpha\)

= \(a^2+b^2\left(\cos ^2 \alpha+\sin ^2 \alpha\right)+2 a b \cos \alpha\)

∴ \(c^2=a^2+b^2+2 a b \cos \alpha\) (because \(\cos ^2 \alpha+\sin ^2 \alpha=1\))

or, c = \(\sqrt{a^2+b^2+2 a b \cos \alpha}\)…….(1)

Law Of Parallelogram Of Vectors Analytical Method Direction Of Resultant \(\vec{c}\): Let the resultant \(\vec{c}\) make an angle θ with the direction of \(\vec{a}\). Then from right-angled ΔOEB, we get,

tanθ= \(\frac{B E}{O E}=\frac{B E}{O A+A E} \text { or, } \tan \theta=\frac{b \sin \alpha}{a+b \cos \alpha}\)…..(2)

Equations (1) and (2) are identical to those in the previous section.

It may be noted that two vectors and their resultant are always confined on a plane. This is not the case for three or more vectors and their resultant. The vectors and their resultant are, in general, distributed in 3-dimensional space.

Some Special Cases Of Addition Of Two Vectors

1. Two Parallel Vectors (α = 0): In this case, sinα = 0 and cosα = 1. Thus from equation (1), we can write,

c = \(\sqrt{a^2+b^2+2 a b}=\sqrt{(a+b)^2}=(a+b)\)

From equation (2), we can conclude that tanθ = 0, or, θ = 0°.

Hence, the magnitude of the resultant is the sum of the magnitudes of the vectors and it is directed along the vectors. It is simply a scalar addition.

2. Two anti-parallel vectors (α = 180°): In this case, sinα = 0 and cosα = -1.

Therefore, from equation (1), we get, c = \(\sqrt{a^2+b^2-2 a b}=\sqrt{(a-b)^2}\) or, \(c=|a-b|\) and from equation (2), tanθ = 0 or, θ = 0° when a > b or θ= 180° when b> a.

Hence, the magnitude of the resultant is the difference in the magnitudes of the vectors and its direction is along the larger vector. It is also a simple scalar operation.

3. Two Equal And Anti-Parallel Vectors (a = b and α = 180°): In this case sinα = 0 and cosα = -1.

Hence, using equation (1), c = \(\sqrt{a^2+a^2+2 a^2 \cos 180^{\circ}}=\sqrt{2 a^2-2 a^2}=0\)

Therefore, the magnitude of the resultant is zero. It is essentially a null vector.

4. Two Orthogonal (Perpendicular To Each Other) Vectors (α = 90°): In this case, sinα = 1 and cosα= 0

∴ From equation (1) and (2), c = \(\sqrt{a^2+b^2+2 a b \cos 90^{\circ}}=\sqrt{a^2+b^2}\) and \(\tan \theta=\frac{b}{a}\)

Therefore, we can conclude that,

1. The maximum possible value of the resultant = sum of magnitudes of the vectors i.e., c(max) = a + b
2. The minimum possible value of the resultant = difference of magnitudes of the vectors i.e., c (min) = |a- b|
3. If the vectors are equal in magnitude and anti-parallel, then the resultant will be 0.

Properties Of Vector Addition (Commutative And Associative Rules)

Properties Of Vector Addition Commutative Rule: Vector addition is commutative. If \(\vec{a}\) and \(\vec{b}\) are two vectors, then by this rule, \(\vec{a}+\vec{b}=\vec{b}+\vec{a}\). This means that vectors can be added in any order.

Properties Of Vector Addition Commutative Rule Proof: OA and OC representing two vectors are two adjacent arms of the parallelogram OABC.

Here, \(\vec{a}=\overrightarrow{O A}=\overrightarrow{C B}\) and \(\vec{b}=\overrightarrow{O C}=\overrightarrow{A B}\)

So, \(\vec{a}+\vec{b}=\overrightarrow{O A}+\overrightarrow{A B}=\overrightarrow{O B}=\vec{c}\), (as per the triangle law of addition of vectors).

Again, \(\vec{b}+\vec{a}=\overrightarrow{O C}+\overrightarrow{C B}=\overrightarrow{O B}=\vec{c}\)

Hence, \(\vec{a}+\vec{b}=\vec{b}+\vec{a}\) or in other words, vector sum is commutative.

Properties Of Vector Addition Associative Rule: Vector addition is associative. To add any three vectors, the addition may be initiated with any two of the vectors. Mathematically, \((\vec{a}+\vec{b})+\vec{c}=\vec{a}+(\vec{b}+\vec{c})\), where \(\vec{a}\), \(\vec{b}\) and \(\vec{c}\) are the given vectors. This rule holds good for the addition of more than three vectors as well.

Properties Of Vector Addition Associative Rule Associative Rule Proof: Let \(\vec{a}\), \(\vec{b}\) and \(\vec{c}\) be represented by the three arms OA, AB and BC of the quadrilateral OABC.

As shown, \(\vec{a}+\vec{b}=\overrightarrow{O A}+\overrightarrow{A B}=\overrightarrow{O B}\) and \(\overrightarrow{O B}+\overrightarrow{B C}=(\vec{a}+\vec{b})+\vec{c}=\overrightarrow{O C}\)

Again, as per figure, \(\vec{b}+\vec{c}=\overrightarrow{A B}+\overrightarrow{B C}=\overrightarrow{A C}\)

∴ \(\vec{a}+(\vec{b}+\vec{c})=\overrightarrow{O A}+\overrightarrow{A C}=\overrightarrow{O C}\)

Hence, \(\vec{a}+(\vec{b}+\vec{c})=(\vec{a}+\vec{b})+\vec{c}\)

Itis noted that scalar addition also follows these two rules.

Law Of Polygon Of Vectors: Addition Of Three Or More Vectors: The sum (resultant) of two vectors can be determined by the law of triangle for vector addition. If the number of vectors is three or more, then by applying the law of triangle successively the law of polygon of vectors is derived. By the application of the law of polygon of vectors, the addition of any number of vectors is possible.

Suppose the resultant of four vectors \(\vec{a}, \vec{b}, \vec{c} \text { and } \vec{d}\) is to be determined. For this, vector \(\overrightarrow{O A}\) equal to \(\vec{a}\) is drawn from any point O.

Now taking A as the initial point, \(\overrightarrow{A B}\) equal to \(\vec{b}\) is drawn. Similarly, \(\overrightarrow{B C}\) equal to \(\vec{c}\) and \(\overrightarrow{C D}\) equal to \(\vec{d}\) are drawn one after another.

Then, the vector \(\overrightarrow{O D}\) drawn by joining the initial point O of the first vector and the terminal point D of the last vector represents the resultant of the vectors \(\vec{a}, \vec{b}, \vec{c} \text { and } \vec{d}\).

∴ \(\overrightarrow{O D}=\vec{R}=\vec{a}+\vec{b}+\vec{c}+\vec{d}=\overrightarrow{O A}+\overrightarrow{A B}+\overrightarrow{B C}+\overrightarrow{C D}\)

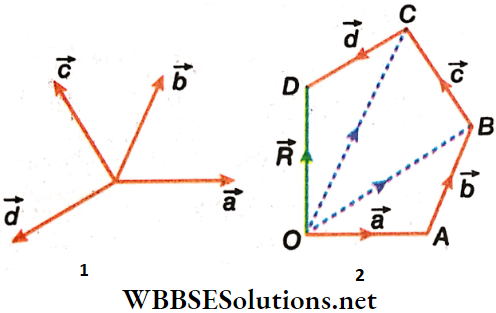
This is called the law of polygon of vectors and with this law, the resultant of any number of vectors can be determined.

Laws Of Polygon Of Vectors Statement: If the magnitudes and directions of a number of vectors are represented by the sides of a closed polygon, taken in order, then the last side of the polygon, taken in the opposite order, represents the magnitude and direction of the resultant of the vectors.

The coplanarity of the vectors is not necessary for the validity of polygon law. Vectors can be added by using the polygon law irrespective of their number and sequence.

Proof Of The Law Of Polygon Of Vectors: Let the magnitudes and directions of the vectors \(\vec{a}, \vec{b}, \vec{c}, \vec{d}\) be represented, by the arms of the polygon OABCD, taken in order: \(\overrightarrow{O A}, \overrightarrow{A B}, \overrightarrow{B C}, \overrightarrow{C D}\).

From Triangle Law,

In triangle OAB, \(\overrightarrow{O B}=\overrightarrow{O A}+\overrightarrow{A B}\),

In triangle OBC, \(\overrightarrow{O C}=\overrightarrow{O B}+\overrightarrow{B C}=\overrightarrow{O A}+\overrightarrow{A B}+\overrightarrow{B C}\) and

In triangle OCD, \(\overrightarrow{O D}=\overrightarrow{O C}+\overrightarrow{C D}=\overrightarrow{O A}+\overrightarrow{A B}+\overrightarrow{B C}+\overrightarrow{C D}\)

∴ \(\overrightarrow{O D}\)=\(\vec{R}=\overrightarrow{O A}+\overrightarrow{A B}+\overrightarrow{B C}+\overrightarrow{C D}\)=\(\vec{a}+\vec{b}+\vec{c}+\vec{d}\)

Hence, \(\overrightarrow{O D}\) is the resultant of the vectors \(\vec{a}, \vec{b}, \vec{c}\) and \(\vec{d}\). The arm \(\overrightarrow{O D}\) is the remaining arm of the polygon taken in the opposite order. This proves the law and also shows that it is nothing but an extension of the law of triangle of vector addition.

Polygon Of Vector Corollary: In the pentagon OABCD, \(\overrightarrow{O A}+\overrightarrow{A B}+\overrightarrow{B C}+\overrightarrow{C D}=\overrightarrow{O D}\)

or, \(\overrightarrow{O A}+\overrightarrow{A B}+\overrightarrow{B C}+\overrightarrow{C D}-\overrightarrow{O D}=0\)

or, \(\overrightarrow{O A}+\overrightarrow{A B}+\overrightarrow{B C}+\overrightarrow{C D}+\overrightarrow{D O}=0\)

In other words, if three or more vectors can be represented by the sides of a closed polygon, taken in order, the resultant of the vectors must be zero.

The physical quantities that have both magnitude and direction and obey all the laws of vector addition such as

1. Triangle law of vectors,
2. Parallelogram law of vectors and
3. The Polygon law of vectors are called vector quantities.

Unit 2 Chapter 2 Vector

Polygon Of Vectors Numerical Examples

Example 1. If \(\vec{a}+\vec{b}=\vec{c}\) and a + b = c, find the angle between \(\vec{a}\) and \(\vec{b}\).
Solution:

Let the angle between \(\vec{a}\) and \(\vec{b}\) be

Here, \(\vec{a}+\vec{b}=\vec{c}\) = \(\vec{c}\)

∴ c² = a² + b² + 2 ab cosα……..(1)

Again, a+b=c or, a² + b²+ 2ab = c²……(2)

Hence from (1) and (2), a² + b² + 2ab = a² + b² + 2ab cosα

or, 2ab = 2ab cosα or, cosα = 1 = cos0°

∴ α = 0°

Example 2. Can the magnitude of the resultant of two equal vectors be equal to the magnitude of each of the vectors? Explain.
Solution:

Let the magnitude of each vector and of the resultant be a and the angle between the vectors be α.

Hence, a² = a² + a² + 2a · a cosα

or, a² = 2a²(1+ cosα) or, 2(1 + cosα) = 1

or, cosα = \(\frac{1}{2}\) – 1 = –\(\frac{1}{2}\) = cos 120°

∴ α = 120°

Hence, the magnitude of the resultant of two equal vectors is equal to that of each of the given vectors when they are inclined at an angle of 120° with each other.

Example 3. 2P and P are two vectors inclined to each other at such an angle that if the 1st vector is doubled, the value of the resultant becomes three times. What is the angle between the two vectors?
Solution:

Let the initial resultant be R and the angle between the two vectors 2 P and P be α.

∴ \(R^2=(2 P)^2+P^2+2 \cdot 2 P \cdot P \cos \alpha\)

or, \(R^2=5 P^2+4 P^2 \cos \alpha\)…..(1)

In the second case, 1st vector =2 P x 2 = 4P and resultant = 3R

or, \((3 R)^2=(4 P)^2+P^2+2.4 P \cdot P \cos \alpha\)

or, \(9 R^2=17 P^2+8 P^2 \cos \alpha\)

or, \(R^2=\frac{17}{9} P^2+\frac{8}{9} P^2 \cos \alpha\)…..(2)

From (1) and (2), \(5 P^2+4 P^2 \cos \alpha=\frac{17}{9} P^2+\frac{8}{9} P^2 \cos \alpha\)

or, \(\left(4-\frac{8}{9}\right) \cos \alpha=\frac{17}{9}-5\)

or, \(\frac{28}{9} \cos \alpha=-\frac{28}{9}\)

or, \(\cos \alpha=-1=\cos 180^{\circ}\)

∴ \(\alpha=180^{\circ}\)

Example 4. Using vectors, prove that the line joining the midpoints of two sides of a triangle is parallel to the base and half its length.
Solution:

From the triangle law of vector addition in ΔABC, \(\overrightarrow{A B}+\overrightarrow{B C}=\overrightarrow{A C}\)

or, \(\frac{1}{2} \overrightarrow{A B}+\frac{1}{2} \overrightarrow{B C}=\frac{1}{2} \overrightarrow{A C}\)…..(1)

As D and E are the mid-points of sides AB and AC, \(\overrightarrow{A D}\)=\(\frac{1}{2} \overrightarrow{A B}\) and \(\overrightarrow{A E}=\frac{1}{2} \overrightarrow{A C}\)

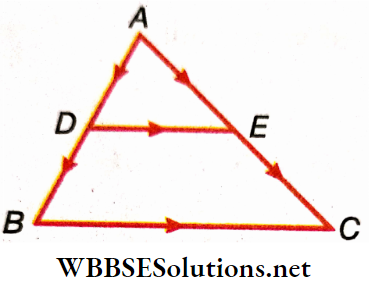
From the triangle law of vector addition in ΔADE \(\overrightarrow{A D}+\overrightarrow{D E}\)=\(\overrightarrow{A E}\)

or, \(\frac{1}{2} \overrightarrow{A B}+\overrightarrow{D E}=\frac{1}{2} \overrightarrow{A C}\)……(2)

From (1) and (2), \(\overrightarrow{D E}=\frac{1}{2} \overrightarrow{B C}\)

Hence, \(\overrightarrow{D E}\) and \(\overrightarrow{B C}\) are parallel and DE = \(\frac{1}{2}\)BC.

Example 5. When will the magnitude of the resultant of two equal vectors be

1. √2 times and
2. √3 times the magnitude of each of them?

Solution:

Let the value of each vector be a and the angle between the two vectors be α.

1. In this case, the magnitude of the resultant =√2a

∴ (√2a)² = a² + a² + 2a · acosα

or, 2a² cosα = 0 or, cosα = 0 = cos 90°

∴ α = 90°

Hence, the angle between the two vectors will be 90°.

2. In this case, the magnitude of the resultant = √3a

∴ (√3a)² = a² + a²+ 2a · acosα

or, 2a² cosα = a² or, cosα = 1/2 = cos 60°

∴ α = 60°

The angle between the two vectors will be 60°.

Example 6. The maximum and the minimum values of the resul¬tant of two forces are 15 N and 7 N respectively. If the magnitude of each force is increased by 1 N and these new forces act at an angle of 90° to each other, find the magnitude and direction of their resultant.
Solution:

Let the magnitudes of the two forces be P and Q.

As per given condition, P + Q = 15 N…..(1)

and P-Q = 7N……(2)

By solving (1) and (2), we get, P = 11 N and Q = 4 N.

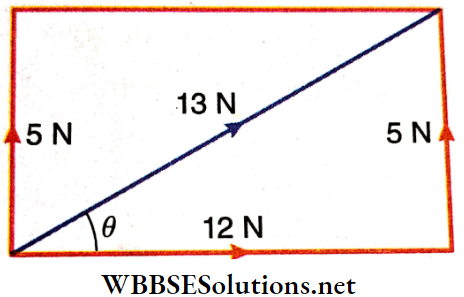
When each of the two forces is increased by 1 N in magnitude, the new magnitudes are P= (11 + 1) = 12 N and Q = (4 + 1) = 5 N and since the angle between these new forces is 90°, new resultant = \(\sqrt{12^2+5^2}=13 \mathrm{~N}\)

Let the angle of inclination of the resultant with the force 12 N be d.

Then, tanθ = \(\frac{5}{12}\) or, d = tan-1 \(\frac{5}{12}\)

∴ The magnitude of the resultant is 13 N and it is inclined with the force 12 N at an angle tan \(\frac{5}{12}\).

Example 7. The resultant \(\vec{R}\) of two vectors has a magnitude equal to one of the vectors and is at a right angle to it. Find the value of the other vector.
Solution:

Let Q be the value of the other vector.

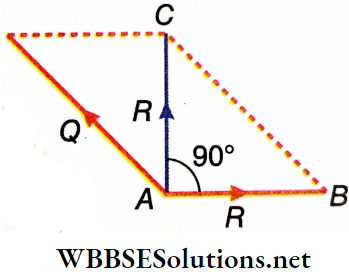
R² + R² = Q² or, Q² = 2 R²

∴ Q = √2R

Example 8. The maximum magnitude of the resultant of two vectors, \(\vec{P}\) and \(\vec{Q}\)(where P> Q) is x times the minimum magnitude of the resultant When the angle between \(\vec{P}\) and \(\vec{Q}\) is θ, the magnitude of the resultant Is equal to half the sum of the magnitudes of the two vectors. Prove that, \(\cos \theta=\frac{x^2+2}{2\left(1-x^2\right)}\).
Solution:

P+Q=x(P-Q) (given) or, Q = \(\frac{x-1}{x+1} \cdot P\)

If R is the resultant of the two vectors when the angle between them is θ, then \(R^2=P^2+Q^2+2 P Q \cos \theta\)…..(1)

Given, \(R=\frac{P+Q}{2}=\frac{P+\frac{x-1}{x+1} \cdot P}{2}=\frac{x P}{x+1}\)

Putting in (1),

∴ \(\frac{x^2 P^2}{(x+1)^2}=P^2+\frac{(x-1)^2}{(x+1)^2} P^2+2 P^2\left(\frac{x-1}{x+1}\right) \cos \theta\)

or, \(\frac{x^2}{(x+1)^2}=1+\frac{(x-1)^2}{(x+1)^2}+\frac{2(x-1)}{x+1} \cos \theta\)

or, \(\frac{-\left(x^2+2\right)}{(x+1)^2}=\frac{2(x-1)}{(x+1)} \cos \theta\)

or, \(\cos \theta=\frac{-\left(x^2+2\right)}{2\left(x^2-1\right)}=\frac{x^2+2}{2\left(1-x^2\right)}\)

Example 9. Out of two vectors, the larger one is √2 times the smaller one. Show that the resultant cannot make an angle greater than π/4 with the larger one.
Solution:

Let the two vectors be √2Q and Q and the resultant be R. Angle between R and √2 Q is θ and ∠ADB = α.

From ΔABD, \(\frac{A B}{\sin \angle A D B}=\frac{B D}{\sin \angle D A B} \text { or, } \frac{\sqrt{2} Q}{\sin \alpha}=\frac{Q}{\sin \theta}\)

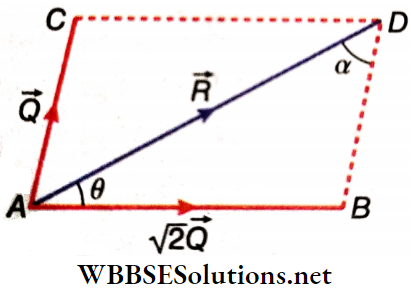
or, \(\sin \theta=\frac{1}{\sqrt{2}} \sin \alpha\)

∴ sinα ≯ 1, sinθ ≯  \(\frac{1}{\sqrt{2}}\)

Hence, θ ≯ \(\frac{\pi}{4}\)

Example 10. Show that If three forces are represented by the three medians of a triangle, they will be In equilibrium.
Solution:

Let the medians of the A ABC be \(\overrightarrow{A D}, \overrightarrow{B E} \text { and } \overrightarrow{C F}\). Vectors AD, BE and CF represent the three forces.

We have to show: \(\overrightarrow{A D}+\overrightarrow{B E}+\overrightarrow{C F}=\overrightarrow{0} \text {. }\)

As per triangle law of vector addition, \(\overrightarrow{A D}=\overrightarrow{A C}+\overrightarrow{C D}=\overrightarrow{A C}+\frac{1}{2} \overrightarrow{C B}\)

⇒ \(\overrightarrow{B E}=\overrightarrow{B A}+\overrightarrow{A E}=\overrightarrow{B A}+\frac{1}{2} \overrightarrow{A C}\)

⇒ \(\overrightarrow{C F}=\overrightarrow{C B}+\overrightarrow{B F}=\overrightarrow{C B}+\frac{1}{2} \overrightarrow{B A}\)

Hence, \(\overrightarrow{A D}+\overrightarrow{B E}+\overrightarrow{C F}=\frac{3}{2}(\overrightarrow{A C}+\overrightarrow{C B}+\overrightarrow{B A})=\overrightarrow{0}\)

[as the sum of 3 vectors, represented by the 3 sides of a triangle taken in order, is zero].

Example 11. The magnitude of the resultant of two forces P and Q acting at a point is (2m+1) \(\sqrt{P^2+Q^2}\) when the angle between them is a, and is (2m- 1)\(\sqrt{P^2+Q^2}\) when the angle is \(\left(\frac{\pi}{2}-\alpha\right)\) Prove that, \(\tan \alpha=\frac{m-1}{m+1}\).
Solution:

When the angle is, the magnitude of the resultant is, R = \(\sqrt{P^2+Q^2+2 P Q \cos \alpha}\)

Given, \(R=(2 m+1) \sqrt{P^2+Q^2}\)

On comparison, \(P^2+Q^2+2 P Q \cos \alpha=(2 m+1)^2\left(P^2+Q^2\right)\)

or, \(2 P Q \cos \alpha=\left(P^2+Q^2\right)\left\{(2 m+1)^2-1\right\}\)

= \(\left(P^2+Q^2\right)\left(4 m^2+4 m+1-1\right)\)

or, \(P Q \cos \alpha=2\left(P^2+Q^2\right) m(m+1)\)

= \(2 m(m+1)\left(P^2+Q^2\right)\)….(1)

Again, when the angle is \(\left(\frac{\pi}{2}-\alpha\right)\), the magnitude of the resultant is,

R’ = \(=\sqrt{P^2+Q^2+2 P Q \cos \left(\frac{\pi}{2}-\alpha\right)}=\sqrt{P^2+Q^2+2 P Q \sin \alpha}\)

Given, \(R^{\prime}=(2 m-1) \sqrt{P^2+Q^2}\).

On comparison, \(P^2+Q^2+2 P Q \sin \alpha=(2 m-1)^2\left(P^2+Q^2\right)\)

or, \(2PQ \sin \alpha=\left(P^2+Q^2\right)\left\{(2 m-1)^2-1\right\}\)

= \(\left(P^2+Q^2\right)\left(4 m^2-4 m+1-1\right)\)

or, \(P Q \sin \alpha=2\left(P^2+Q^2\right) m(m-1)\)

= \(2 m(m-1)\left(P^2+Q^2\right)\)….(2)

Dividing (2) by (1), we have, \(\tan \alpha=\frac{m-1}{m+1}\).

Example 12. Two forces P and Q have a resultant R. This resultant is doubled, either when Q Is doubled, or when Q is reversed. Show that, P:Q:P = √2:√3:√2.
Solution:

Let the angle between P and Q be \(\alpha\).

So, \(R^2=P^2+Q^2+2 P Q\) cosα

or, \(2 P Q \cos \alpha=R^2-P^2-Q^2\)…..(1)

When Q is doubled, \((2 R)^2=P^2+(2 Q)^2+2 P \cdot 2 Q \cos \alpha\)

or, \(2 P Q \cos \alpha=2 R^2-\frac{1}{2} P^2-2 Q^2\)…..(2)

Again, when Q is reversed, \((2 R)^2=P^2+Q^2+2 P Q \cos \left(180^{\circ}-\alpha\right)\)

or, \(2 P Q \cos \alpha=-4 R^2+P^2+Q^2\)…..(3)

From (1) and (3), \(R^2-P^2-Q^2=-4 R^2+P^2+Q^2\)

or, \(2 P^2+2 Q^2-5 R^2=0\)…..(4)

From (1) and (2), \(R^2-P^2-Q^2=2 R^2-\frac{1}{2} P^2-2 Q^2\)

or, \(P^2-2 Q^2+2 R^2=0\)…….(5)

Adding (4) and (5), \(3 P^2-3 R^2=0\) or, \(P=R\)

Putting this in (5), \(P^2-2 Q^2+2 P^2=0\) or, \(3 P^2=2 Q^2\) or, \(Q=\frac{\sqrt{3}}{\sqrt{2}} P\)

∴ \(P: Q: R=P: \frac{\sqrt{3}}{\sqrt{2}} P: P=1: \frac{\sqrt{3}}{\sqrt{2}}: 1=\sqrt{2}: \sqrt{3}: \sqrt{2}\)

Example 13. The resultant of two forces P and Q, inclined at a fixed angle, is it which makes an angle θ with P. If P is replaced by (P+ Q) keeping the direction unchanged, show that the resultant of (P+ R) and Q would be inclined at \(\frac{\theta}{2}\) with P+ R.
Solution:

Let a = angle between P and Q.

Then, R² = P² + Q² + 2PQcosα….(1)

and \(\tan \theta=\frac{Q \sin \alpha}{P+Q \cos \alpha}\)…..(2)

In the second case, if the resultant makes an angle θ₁ with P+R, then \(\tan \theta_1=\frac{Q \sin \alpha}{(P+R)+Q \cos \alpha}\)….(3)

Now, \(\tan \left(\theta-\theta_1\right)=\frac{\tan \theta-\tan \theta_1}{1+\tan \theta \tan \theta_1}\)

= \(\frac{\frac{Q \sin \alpha}{P+Q \cos \alpha}-\frac{Q \sin \alpha}{(P+R)+Q \cos \alpha}}{1+\frac{Q^2 \sin ^2 \alpha}{[P+Q \cos \alpha][(P+R)+Q \cos \alpha]}}\)

= \(\frac{R Q \sin \alpha}{[P+Q \cos \alpha][(P+R)+Q \cos \alpha]+Q^2 \sin ^2 \alpha}\)

= \(\frac{R Q \sin \alpha}{P^2+Q^2+2 P Q \cos \alpha+P R+R Q \cos \alpha}\)

= \(\frac{R Q \sin \alpha}{R^2+R(P+Q \cos \alpha)}=\frac{Q \sin \alpha}{(P+R)+Q \cos \alpha}\)

= \(\tan \theta_1 \text { [using equation (1) and (3)] }\)

∴ \(\theta-\theta_1=\theta_1 \quad \text { or, } \theta_1=\frac{\theta}{2}\)

Example 14. Four forces 2 P, P, P, and 2P acton a point towards NE, NW, SW, and SE directions respectively. Find the resultant of the forces.
Solution:

The forces 2P along NE, P along NW, P along SW, and 2P along SE are represented by \(\overrightarrow{O A}, \overrightarrow{A B}, \overrightarrow{B C} \text { and } \overrightarrow{C D}\) respectively.

The initial point O and the terminal point D are joined. Hence, the resultant of the four given vectors is \(\overrightarrow{O D}\).

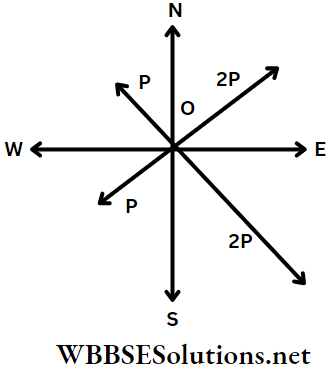
Let ∠EOD = θ.

The quadrilateral EABC is a square. So, from ΔODE, DE = OE =P and ∠OED = 90°

∴ OD = \(\sqrt{P^2+P^2}=\sqrt{2 P^2}=\sqrt{2 P}\)

and \(\tan \theta=\frac{D E}{O E}=\frac{P}{P}=1 \quad \text { or, } \theta=45^{\circ}\)

Since, \(\overrightarrow{O A}\) is along NE, \(\overrightarrow{O D}\) is along east.

Alternative solution: The forces 2 P and P along NE and SW directions respectively are opposite to each other. Hence, their resultant = (2P-P) is equal to a force P directed towards NE.

Similarly, the resultant of P directed towards NW and 2P towards SE is equal to a force P along SE.

Now there two forces, P along NE and P along SE, are perpendicular to each other. So, the resultant is, F = \(\sqrt{P^2+P^2}=\sqrt{2} P\)

If the resultant F makes an angle 6 with P along NE, then tan9 = \(\frac{P}{P}\) = 1 or, 6 = 45°

Thus, F is in the eastward direction.

Hence, the resultant of the four forces is 72 P along the x-axis, i.e., along the east.

 

Resolutions Of Vectors

A vector can be resolved into many components just as many vectors can be added to give a single resultant vector.

Resolutions Of vectors Definition: When a vector is split into two or more vectors in such a manner that, the original vector becomes the resultant of the resolved parts or components of the vector, this splitting is called the resolution of vectors.

• Apparently, it may seem that the resolution of vectors is just the opposite of the addition of vectors. But it may be noted that, when two vectors are added, it gives only one resultant vector. On the other hand, in the resolution of vectors, different sets of components can be formed.
• The resolution of vectors into two or three components is an elegant technique to solve most of the problems related to vectors. Splitting into more than three components is very rare in practice.

Resolution In Two Dimensions

Resolution Of Vectors Into Two Components: Let the magnitude and direction of a given vector \(\vec{R}\) be represented by \(\overrightarrow{O A}\). OM and ON are inclined to OA by angles α and β respectively, in such a way that OA, OM, and ON lie on the same plane. The vector \(\vec{R}\) is to be resolved into components along OM and ON.

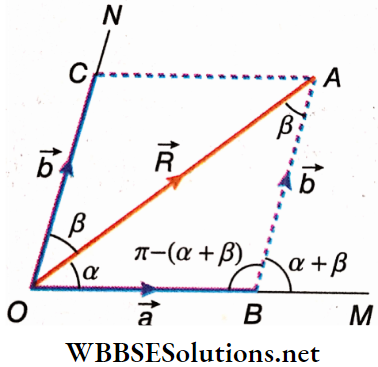
AC parallel to OM and AB parallel to ON are drawn to complete the parallelogram. Now, from the law of parallelogram of vectors, \(\overrightarrow{O B}+\overrightarrow{O C}=\overrightarrow{O A}\)

Thus, the two components of \(\overrightarrow{O A}\) are \(\overrightarrow{O B}\) and \(\overrightarrow{O C}\)  and their magnitudes are a and b respectively.

∴ OB = a and OC = BA = b

Applying trigonometric rules to ΔOAB, we get, \(\frac{O B}{\sin \angle O A B}=\frac{B A}{\sin \angle A O B}=\frac{O A}{\sin \angle A B O}\)

or, \(\frac{a}{\sin \beta}=\frac{b}{\sin \alpha}=\frac{R}{\sin [\pi-\{\alpha+\beta\}]}\)

or, \(\frac{a}{\sin \beta}=\frac{b}{\sin \alpha}=\frac{R}{\sin (\alpha+\beta)}\)

Hence, \(a=\frac{R \sin \beta}{\sin (\alpha+\beta)}\) and \(b=\frac{R \sin \alpha}{\sin (\alpha+\beta)}\)…..(1)

Since α and β can have many sets of values, a and b can also have many values. Hence, a vector can be resolved into different pairs of components.

The two most useful components of a vector are the two mutually perpendicular components when α + β = 90°. Then from equation (1), we get

a = \(\frac{R \sin \left(90^{\circ}-\alpha\right)}{\sin 90^{\circ}}=R \cos \alpha\)

and b = \(\frac{R \sin \alpha}{\sin 90^{\circ}}=R \sin \alpha\)…..(2)

Again, depending on α, different pairs of orthogonal components are possible.

Resolution Of Vectors Into Rectangular Or Orthogonal Components: \(\vec{R}\) has been resolved into two components along two mutually perpendicular axes OX and OY.

Let \(\overrightarrow{O B}=\vec{a}, \overrightarrow{O C}=\vec{b} \text { and } \angle A O B=\alpha\); i.e., the component \(\vec{a}\) is inclined to the vector R at an angle α.

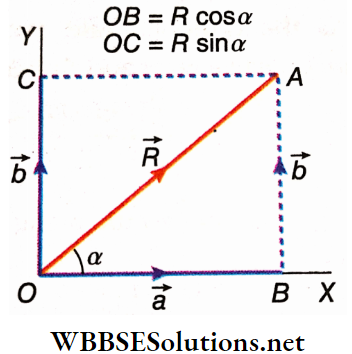
According to \(\cos \alpha=\frac{O B}{O A}=\frac{a}{R}\)

or, a = Rcosα…..(1)

and \(\sin \alpha=\frac{A B}{O A}=\frac{b}{R} \text { or, } b=R \sin \alpha\)….(2)

Hence, the component of \(\vec{R}\) along a direction that makes an angle α with \(\vec{R}\) is Rcosα, and the other component is Rsinα.

Resolution Of Vectors Into Rectangular Or Orthogonal Components Special Cases: To determine the component of a vector \(\vec{R}\) along its own direction, we put α = 0° in equation (1) and get a = Rcos0° = R.

Again, by putting α = 0° in equation (2) [or, α = 90° in equation (1)], we get the other component, i.e., the component in the direction perpendicular to \(\vec{R}\), as, b = Rsin 0° = 0.

Hence, we may conclude that,

1. The component (or resolved part) of a vector along its own direction is the vector itself.
2. There is no component in a direction perpendicular to the vector.

Practical Example—Pull Or Push: A body can be set into motion along a horizontal plane by pushing it from the back or by pulling it towards the front. When the body is pushed, the applied force F₁ usually acts downwards at an angle with the horizontal.

• Horizontal motion of the body is due to the horizontal component F_x of the applied force F₁. The vertical component F_y, acting downwards, adds to the weight of the body, and hence, pushing becomes difficult.
• On the other hand, while pulling, the applied force F₂ acts upwards at an angle with the horizontal. In this case, also, the horizontal component F’_x of F₂ produces the horizontal motion of the body. The vertical component F’_y acting upwards, effectively reduces the weight of the body. Hence pulling becomes easier.

Thus, it is easier to pull a body than to push it.

Resolutions In Two Dimensional Numerical Examples

Example 1. A force of 30 dyn Is inclined to the y-axis at an angle of 60°. Find the components of the force along the x and y axes respectively.
Solution:

Given, F = 30 dyn and θ = 60°

Hence, a component of F along the y-axis, F_y = F cosθ = F cos60° = 30 x 1/2 = 15 dyn

and a component of F along x-axis \(F_x=F \sin 60^{\circ}=30 \times \frac{\sqrt{3}}{2}=15 \sqrt{3} \text { dyn }.\)

Example 2. The value of the resultant of two mutually perpendicular forces is 80 Dyn. The resultant makes an angle 60° with one of the forces. Find the magnitudes of the forces.
Solution:

Let the forces be \(\vec{P}\), \(\vec{R}\) and the resultant that makes an angle 60° with \(\vec{P}\) be  \(\vec{R}.\)

Here, R = 80 dyn

∴ P = R cosθ – 80 cos60° = 80 x 1/2 = 40 dyn

and Q = R sin60° =80 sin60° = \(80 \times \frac{\sqrt{3}}{2}\) = 40√3 dyn .

 

Motion Of A Projectile

Motion Of A Projectile Definition: A body thrown upwards in any direction from the earth’s surface or a point close to it, is called a projectile. Common examples of projectiles are

1. A javelin thrown by an athlete,
2. A bullet fired from a rifle,
3. An object dropped from an airplane,
4. A jet of water coming out from the side hole of a vessel,
5. A stone thrown from the top of a hill or a tower,
6. A rocket after its fuel is exhausted.

The path traced out by a projectile is called its trajectory. The motion of a projectile is two-dimensional as it is always confined to a vertical plane.

• To study the projectile motion the horizontal direction is taken along the x-axis and the vertical direction is taken along the y-axis. The only force acting on the projectile is the gravitational force.
• So the projectile has acceleration in the y direction which is equal to the acceleration due to gravity. The force acting on the projectile is zero along the x-axis. So the horizontal acceleration is zero. This means, that projectile motion is a combination of horizontal motion with constant velocity and vertical motion with uniform acceleration.
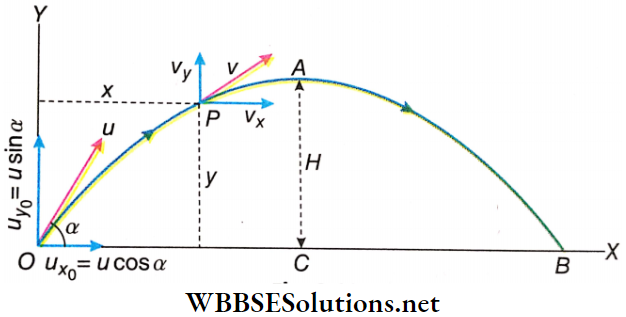
• Let a body be projected from a point O with velocity u making an angle α with the horizontal. The body reaches B following the path OAB through the highest point A. Points B and O lie on the same horizontal plane.

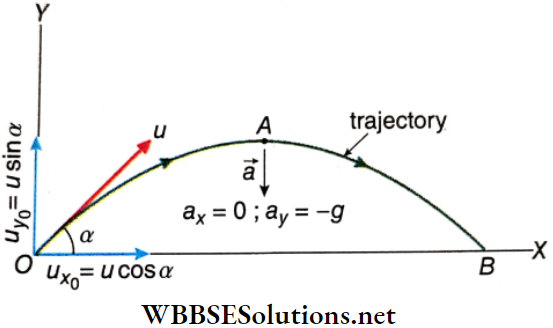
O is the point of projection, u is the velocity of projection, the angle of projection, and OB is the horizontal range; the time taken for traveling the path OAB is called the time of flight. Velocity of projection u has a horizontal component = \(u_{x_0}=u \cos \alpha\) and a vertical component = \(u_{y_0}=u \sin \alpha\).

• As acceleration due to gravity (g) acts vertically downward, the velocity component usina gradually changes. Hence, the vertical motion of the body is a motion under gravity. But since g has no component along the horizontal direction, the acceleration or deceleration of the horizontal component of motion is zero.
• Hence, the motion of the body along the horizontal direction is uniform, i.e., horizontal velocity \(u_{x_0}=u \cos \alpha\) = constant. It is convenient to use the vertical motion and the horizontal motion separately in discussions related to projectile motion.

Principle Of Physical Independence Of Motions: In the absence of air resistance the motion of a projectile is considered as the combination of the following two independent motions.

1. Motion along the horizontal direction with uniform velocity.
2. Motion along vertical direction under gravity i.e., uniform acceleration equal to g.

The two motions of a projectile along horizontal and vertical directions are independent of each other. This is called the principle of physical independence of motion.

Principle Of Physical Independence Of Motions Key Points:

1. A projectile returns to the ground at the same angle and with the same velocity with which it is projected.
2. When a projectile is at the highest point of its trajectory
   1. It possesses velocity only along horizontal,
   2. The velocity and acceleration of the projectile are perpendicular to each other.

Equations For Projectile Motion: Let the time taken by the projectile to reach the point P be t.

For the horizontal motion of the projectile we have, \(v_x=u_{x_0}+a_x t\)

or, \(v_x=u \cos \alpha\) (because \(a_x=0\))….(1)

where v_x is the horizontal component of the velocity of the projectile after time t.

Again, \(x=u_{x_0} t+\frac{1}{2} a_x t^2\)

or, \(x=u_{x_0} t=u \cos \alpha t\)….(2)

From this equation, we get the horizontal position of the projectile after time t.

For the vertical motion of the projectile, a_y=-g

∴ \(v_y=u_{y_0}+a_y t\)

[v_y is the vertical component of velocity of the projectile after time t]

or, \(v_y=u_{y_0}-g t\) (because \(a_y=-g\))……(3)

Again, \(v_y^2=u_{y_0}^2-2 g y\)

and \(y=u_{y_0} t+\frac{1}{2} a_y t^2=u_{y_0} t-\frac{1}{2} g t^2\)

From equation (4), we get the vertical position of the projectile after time t.

Equations For Projectile Motion Maximum Height: The maximum height of a projectile is the maximum vertical distance attained by the projectile above the horizontal plane of projection. It is denoted by H. To calculate the height of the projectile, consider only its vertical motion. The vertical component of the velocity of projection = u sinα and velocity at the highest point = 0.

If H = maximum height, \(0=(u \sin \alpha)^2-2 g H \text { or, } H=\frac{u^2 \sin ^2 \alpha}{2 g}\)….(5)

It should be noted that the body attains maximum height by reaching a point that is not exactly above point O. Because of the horizontal component of motion, the body has a horizontal displacement as well. the highest point on the trajectory of the projectile is point A and maximum height (H) = AC.

Time Of Flight: It is the time taken by the projectile from the instant it is projected till it reaches a point in the horizontal plane of its projection. As shown the total time taken to reach point B from point O is the time of flight.

Let the time taken to reach the maximum height be T₁

Hence, \(0=u \sin \alpha-g T_1 \text { or, } T_1=\frac{u \sin \alpha}{g}\)….(6)

On reaching the highest point, the body starts descending again. At the same time, the body covers a horizontal path due to its horizontal component of motion. Finally, the body reaches B. Thus, the body follows the trajectory OAB.

The height of B with respect to the point of projection, O is zero, i.e., the vertical displacement of the object is zero. Hence, if T is the time required to cover the path OAB,

0 = \(u \sin \alpha \cdot T-\frac{1}{2} g T^2\) [from equation (4)]

∴ \(2 u \sin \alpha=g T or, T=\frac{2 u \sin \alpha}{g}\)…..(7)

Comparing equations (6) and (7), \(2 T_1=\frac{2 u \sin \alpha}{g}=T \text { or, } T_1=\frac{T}{2}\)

So, the time required to reach the maximum height is equal to half the time of flight.

Horizontal Range: Let the horizontal range be OB = R. The horizontal distance traversed by the body in time T is R. Since the body crosses the distance R horizontally with a uniform velocity ucosα,

R = \(u \cos \alpha \cdot T=u \cos \alpha \cdot \frac{2 u \sin \alpha}{g}\)

= \(\frac{2 u^2 \sin \alpha \cos \alpha}{g}=\frac{u^2}{g} \sin 2 \alpha\)…..(8)

The value of R is maximum when sin 2α is maximum. Therefore, the condition for covering the maximum horizontal distance for a particular initial velocity u, is sin 2α = 1 = sin90° or, α = 45°.

∴ \(R_{\max }=\text { Maximum range }=\frac{u^2}{g}\)…..(9)

To send a projectile to the maximum possible distance, it has to be thrown at an angle of 45° with the horizontal. This is why sportsmen try to throw the javelin or discus at 45°.

It should be noted that if the angle of projection is (\(\frac{\pi}{2}-\alpha\)) instead of a, the horizontal range remains the same for a particular velocity of projection, since, \(R^{\prime}=\frac{u^2}{g} \sin \left\{2\left(\frac{\pi}{2}-\alpha\right)\right\}\) = \(\frac{u^2}{g} \sin (\pi-2 \alpha)=\frac{u^2}{g} \sin 2 \alpha=R\)

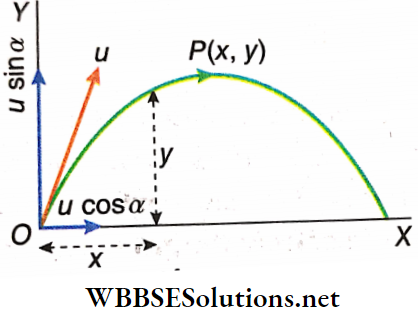
Locus Of A Projectile: Let us take a reference frame such that, its positive y-axis extends vertically upwards, and the positive x-axis extends horizontally in the direction of the horizontal component of the velocity of the projectile. The origin is taken as the point of projection

Let the point reached by the body from O in time f be P and the coordinates of P be (x, y).

y = \(u \sin \alpha \cdot t-\frac{1}{2} g t^2\)…………(10)

Again, as the horizontal velocity =ucosa = constant, x = ucosα · t

or, \(t=\frac{x}{u \cos \alpha}\)

From (10) and (11), \(y=u \sin \alpha \frac{x}{u \cos \alpha}-\frac{1}{2} g \frac{x^2}{u^2 \cos ^2 \alpha}\)

or, y = \(x \tan \alpha-\frac{g}{2 u^2 \cos ^2 \alpha} x^2\)

This equation is the locus of the projectile.

The equation is of the type y=ax+bx² which is the equation of a parabola. Hence, the trajectory of a projectile is parabolic.

Motion Of A Projectile Numerical Examples

Example 1. A body is projected with a velocity of 20 m s^-1, making an angle of 45° with the horizontal. Calculate—

1. The time taken to reach the ground [g = 10 m s^-2],
2. The maximum height it can attain and
3. Horizontal range.

Solution:

The vertical and horizontal components of the velocity of 20 m s^-1 are \(u_H=20 \cos 45^{\circ}=20 \times \frac{1}{\sqrt{2}}=10 \sqrt{2} \mathrm{~m} \cdot \mathrm{s}^{-1}\)

and \(u_V=20 \sin 45^{\circ}=20 \times \frac{1}{\sqrt{2}}=10 \sqrt{2} \mathrm{~m} \cdot \mathrm{s}^{-1}\).

1. Let the total time of flight of the body be t. Considering the vertical motion of the body, we get from the equation h = ut-\(\frac{1}{2}\)gt²,

0 = \(10 \sqrt{2} t-\frac{1}{2} \cdot 10 \cdot t^2 \quad[\text { as } h=0]\)

or, \(5 t^2=10 \sqrt{2} t\)

∴ Total time of flight, \(t=\frac{10 \sqrt{2}}{5}=2 \sqrt{2}=2 \times 1.414=2.828 \mathrm{~s}\)

2. Let the maximum height attained be h. Vertical velocity at the maximum height = 0.

Considering the vertical motion of the body, we get from the equation v² = u²- 2gh,

0 = (10√2)² -2 ·10 · h

∴ h = 10m

3. Let the distance from the point of projection to the point at the ground where the body touches be x. By considering the horizontal motion of the body, we get,

x = u_H x t = 10√2 x 2√2 = 40 m.

∴ The horizontal range = 40 m.

Example 2.  A plane is flying horizontally at a height of 196 m at 600 km · h^-1 with respect to the ground. On reaching a point directly above A, the plane drops an object that reaches the ground at B. Find the distance AB.
Solution:

Let the point directly above A from where the object is dropped be O

Therefore, OA = 1960 m. Let the time taken by the object to hit the ground at B be t.

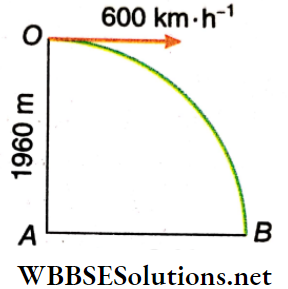
Since the plane is flying horizontally, the initial vertical velocity of the object = 0 and vertical (downward) A displacement = 1960 m.

Considering the vertical motion of the object, we get from the equation h = ut + \(\frac{1}{2}\) gt²,

1960 = \(0 \times t+\frac{1}{2} \times 9.8 \times t^2\)

or, \(t^2=\frac{2 \times 1960}{9.8}=400\) or, \(t=20 \mathrm{~s}\)

The initial horizontal velocity of the body = \(600 \mathrm{~km} \cdot \mathrm{h}^{-1}=\frac{600 \times 1000}{60 \times 60} \mathrm{~m} \cdot \mathrm{s}^{-1}=\frac{500}{3} \mathrm{~m} \cdot \mathrm{s}^{-1}\)

So, the object moves at a uniform velocity of \(\frac{500}{3} \mathrm{~m} \cdot \mathrm{s}^{-1}\) in the horizontal direction. Hence,

AB = \(\frac{500}{3} \times 20 \mathrm{~m}=\frac{500 \times 20}{3 \times 1000} \mathrm{~km}=\frac{10}{3}=3.33 \mathrm{~km}\)

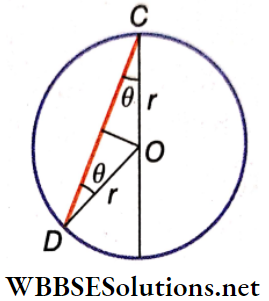
Example 3.  A particle falls from rest from the highest point of a vertical circle of radius r, along a chord without any friction. Show that the time taken by the particle to come down is independent of the chord’s length. Find the time in terms of r and g.
Solution:

Let the chord along which the particle falls be CD. The CD makes an angle θ with the vertical diameter as shown. CD = 2 r cosθ. The component of acceleration due to gravity along CD = g cosθ.

Let the time taken by this particle to fall from rest along CD be t.

Hence, from the equation s = ut + \(\frac{1}{2}\) at²,

2r \(\cos \theta\) = \(0+\frac{1}{2} g \cos \theta \cdot t^2\)

or, \(t^2=\frac{4 r}{g} \text { or, } t=2 \sqrt{\frac{r}{g}}\)

The time is independent of θ, and hence on the length of the chord CD.

Example 4. At what angle with respect to the horizontal, should a projectile be thrown with a velocity of 19.6 m · s^-1, to just clear a wall 14.7 m high, at a distance of 19.6 m?
Solution:

Let the angle of projection be θ. Hence horizontal component of velocity = 19.6 cosθ m · s^-1 and its vertical component = 19.6 sinθ m · s^-1.

Let the time after which the projectile crosses the wall be t.

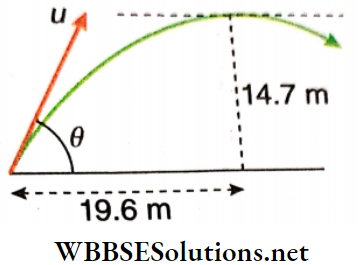
Considering horizontal motion, 19.6 = 19.6 cosθ x t or, t = secθ

For the vertical motion, 14.7 =19.6 sinθ x t \(\frac{1}{2}\) x 9.8 x t²

or, 14.7 = 19.6 sinθ x secθ-4.9 sec²θ

or, 3 = 4 tanθ – (1 + tan²θ) or, tan²θ-4tanθ + 4 = 0

or, (tanθ – 2)³ = 0 or, tanθ = 2

∴ θ = tan^-1 2 = 63.4°

Example 5. A block of ice is sliding down the sloping roof of a house and the angle of inclination of the roof with the horizontal is 30°. The maximum and minimum heights of the roof from the ground are 8.1 m and 5.6 m. How far from the starting point, measured horizontally, does the block land? [ignore friction].
Solution:

Let the highest point of the roof be A and the lowest point be P as shown.

∴ AC= 8.1 m; PD = 5.6 m

∴ AB =AC-BC =AC-PD= 8.1 -5.6 = 2.5 m

AP = \(\frac{A B}{\sin 30^{\circ}}=\frac{2.5}{\frac{1}{2}}=5 \mathrm{~m}\)

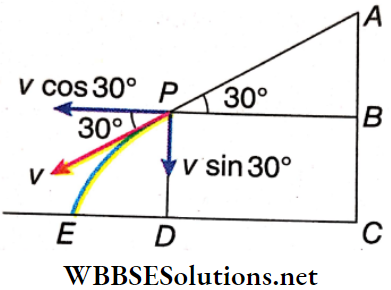
Let the velocity of the block at P be v.

Considering the motion of the block from A to P,

v² = 2g sin30° xAP = 2 x 9.8 x \(\frac{1}{2}\) x 5 = 49 or, v = 7 m · s^-1.

The horizontal and vertical components of the velocity at P are \(v \cos 30^{\circ}=\frac{7 \sqrt{3}}{2} \mathrm{~m} \cdot \mathrm{s}^{-1} \quad \text { and } \quad v \sin 30^{\circ}=\frac{7}{2} \mathrm{~m} \cdot \mathrm{s}^{-1}\) respectively.

Let the total time taken by the block to come from P to E be t. Considering the vertical motion of the block,

5.6 = \(\frac{7}{2} t+\frac{1}{2} \times 9.8 \times t^2 \quad \text { or, } 7 t^2+5 t-8=0\)

∴ t = \(\frac{-5 \pm \sqrt{25+224}}{14}=0.77 \mathrm{~s}\) [taking the positive value of t]

Now, DE =  \(\nu \cos 30^{\circ} \times t=\frac{7 \sqrt{3}}{2} \times 0.77=2.7 \sqrt{3} \mathrm{~m}\)

∴ CE = \(C D+D E=2.5 \sqrt{3}+2.7 \sqrt{3}\)

(\(\tan 30^{\circ}=\frac{A B}{P B} \text { or, } P B=2.5 \sqrt{3} \mathrm{~m}\) and, DC = \(P B=2.5 \sqrt{3} \mathrm{~m}]\))

= \(5.2 \sqrt{3}=9 \mathrm{~m}\)

Example 6. The equation of the trajectory of a projectile on s vertical plane is y = ax- bx², where a and b are constants, and x and y respectively are the horizontal distances of the projectile from the point of projection. Find out the maximum height attained by the projectile, and also the angle of projection with respect to the horizontal.
Solution:

Let, u = velocity of projection; α = angle of projection

The velocity ucosα in the horizontal direction is uniform.

So, in time t, x = \(u \cos \alpha \cdot t \quad \text { or, } t=\frac{x}{u \cos \alpha}\)

The velocity u sin α in the vertically upward direction is under a uniform retardation -g, where g is the acceleration due to gravity.

Then, in time t, y = \(u \sin \alpha \cdot t-\frac{1}{2} g t^2=u \sin \alpha \cdot \frac{x}{u \cos \alpha}-\frac{1}{2} g \frac{x^2}{u^2 \cos ^2 \alpha}\)

or, \(y=x \tan \alpha-\frac{g}{2 u^2 \cos ^2 \alpha} x^2\)

Comparing with the given equation y=a x-bx², we get

1. a =θ, or angle of projection, \(\theta=\tan ^{-1} a\).
2. b = \(\frac{g}{2 u^2 \cos ^2 \alpha} \quad or, u^2=\frac{g}{2 b \cos ^2 \alpha}\)

At maximum height H, the velocity of the projectile is zero.

Considering vertical motion, we have

0 = \((u \sin \alpha)^2-2 g H\)

or, H = \(\frac{u^2 \sin ^2 \alpha}{2 g}=\frac{g}{2 b \cos ^2 \alpha} \cdot \frac{\sin ^2 \alpha}{2 g}\)

= \(\frac{\tan ^2 \alpha}{4 b}=\frac{a^2}{4 b}\)

Example 7. A gun fires at an angle 30° with the horizontal and hits a target at a distance of 3 km. Can another target at a distance of 5 km be hit by changing the angle of projection but keeping the velocity of projection unchanged?
Solution:

Horizontal range, \(R=\frac{u^2 \sin 2 \alpha}{g}\)

In the first case, 3 = \(\frac{u^2 \sin \left(2 \times 30^{\circ}\right)}{g}=\frac{u^2}{g} \frac{\sqrt{3}}{2}\)

or, \(\frac{u^2}{g}=2 \sqrt{3}\)

If the velocity of projection is unchanged, the maximum horizontal range for \(\alpha=45^{\circ}\), is \(R_{\max }=\frac{u^2 \sin \left(2 \times 45^{\circ}\right)}{g}=\frac{u^2}{g}=2 \sqrt{3}\)

= \(2 \times 1.732=3.464 \mathrm{~km}\)

So, a target at a distance of 5 km cannot be hit.

Example 8. A gun is kept on a horizontal road and is used to hit a running car. The uniform speed of the car is 72 km/h. At the instant of firing at an angle of 45° with the horizontal, the car is at a distance of 500 m from the gun. Find out the distance between the gun and the car at the instant of hitting. Given, 10 m/s².
Solution:

Velocity of the car, v = 72 km/h = 20 m/s;

if it is hit after a time s, then its displacement = 201 m

∴ Distance between the gun and the car at that instant,

D =  500 + 20tm.

If u be the initial velocity of the bullet, then its horizontal range,

R = \(\frac{u^2 \sin \left(2 \times 45^{\circ}\right)}{g}=\frac{u^2}{g}\)

∴ \(\frac{u^2}{g}=500+20 t\)…..(1)

Considering the vertical motion of the bullet in time t, we have

0 = \(u \sin 45^{\circ} \cdot t-\frac{1}{2} g t^2 \quad \text { or, } \frac{u t}{\sqrt{2}}=\frac{1}{2} g t^2\)

or, u = \(\frac{1}{\sqrt{2}} g t\)

∴ \(u^2=\frac{1}{2} g^2 t^2 \quad \text { or, } \frac{u^2}{g}=\frac{1}{2} g t^2=\frac{1}{2} \times 10 \times t^2=5 t^2\)

Putting in (1), we get \(5 t^2=500+20 t \text { or, } t^2-4 t-100=0\)

∴ t = \(\frac{4 \pm \sqrt{16-4 \times 1 \times(-100)}}{2 \times 1}=2 \pm \sqrt{104}\)

Keeping only the positive value of t, we have \(t=2 \pm \sqrt{104}=12.2 \mathrm{~s}\)

So, we get, \(D=500+20 t=500+20 \times 12.2=744 \mathrm{~m} \text {. }\)

Example 9. The initial velocity of a projectile is (\(\hat{i}+2 \hat{j}\)) m/s, where i and j are unit vectors along the horizontal and vertical directions respectively. Find out the locus of the projectile, taking g = 10 m/s².
Solution:

Horizontal and vertical components of initial velocity are, respectively,

u_x = 1 m/s and u_y = 2 m/s

Let, at t = 0, the initial coordinates of the projectile are (0, 0); at time t, these are (x, y).

So, \(x=u_x t=t \mathrm{~m}\)

y = \(u_y t-\frac{1}{2} g t^2=\left(2 t-5 t^2\right)=2 x-5 x^2\)

∴ \(y=2 x-5 x^2\) is the locus of the projectile.

Example 10. Two objects are thrown simultaneously from the same point with the same initial velocity at angles of projection α and β respectively. If they reach the top and the bottom of a tower simultaneously, then prove that tanα – tanβ = tanθ where θ = angle of elevation of the tower from the point of projection.
Solution:

Horizontal range of the 2nd projectile

OB = \(x=\frac{u^2 \sin 2 \beta}{g}=\frac{2 u^2}{g} \sin \beta \cos \beta\)…..(1)

Time of flight of the 2nd projectile, t = \(\frac{2 u \sin \beta}{g}\)

= time taken by the 1st projectile from O to A

For the horizontal motion of the 1st projectile,

OB = \(x=u \cos \alpha \cdot t=u \cos \alpha \cdot \frac{2 u \sin \beta}{g}\)

= \(\frac{2 u^2}{g} \sin \beta \cos \alpha\)……….(2)

Comparing (1) and (2), we have \(\cos \alpha=\cos \beta\)…….(3)

For the vertical motion of the 1st projectile, AB = \(y=u \sin \alpha \cdot t-\frac{1}{2} g t^2\)

= \(u \sin \alpha \frac{2 u \sin \beta}{g}-\frac{1}{2} g\left(\frac{2 u \sin \beta}{g}\right)^2\)

= \(\frac{2 u^2}{g}\left(\sin \alpha \sin \beta-\sin ^2 \beta\right)\)…….(4)

Diving (4) by (1), we have \(\tan \theta=\frac{A B}{O B}=\frac{y}{x}=\frac{\sin \alpha \sin \beta-\sin ^2 \beta}{\sin \beta \cos \beta}\)

= \(\frac{\sin \alpha}{\cos \beta}-\frac{\sin \beta}{\cos \beta}=\frac{\sin \alpha}{\cos \alpha}-\tan \beta\)

= \(tan \alpha-\tan \beta\) (using (3))

Example 11. A truck starts from rest and accelerates uniformly. at 2 m · s^-2 At t = 10 s, a stone is dropped by a person standing on the top of the truck (6 m high from the ground). What are the

1. Velocity and
2. Acceleration of the stone at t = 11 s? (Neglect air resistance).

Solution:

The velocity of the truck after 10s = at = 2 x 10 = 20 m · s^-1

So, at the time of release, the stone has a horizontal velocity of 20 m • s^-1, but no vertical velocity. Its horizontal acceleration = 0 and vertical acceleration, g = 9.8 m • s^-2.

1. At 11 s, i.e., 1 s after release, the horizontal velocity of the stone = 20 m • s^-1;

vertical velocity = gt = 9.8 x 1 = 9.8 m • s^-1

So, the resultant velocity = \(\sqrt{(20)^2+(9.8)^2}=22.27 \mathrm{~m} \cdot \mathrm{s}^{-1}\)

2. The acceleration of the stone = downward acceleration due to gravity = 9.8 m • s^-2

 

Vector Synopsis

Geometrical Representation Of A Vector:

1. A vector is represented by a line segment with an arrowhead,
2. The magnitude of the vector is the length of the line segment and
3. The direction of the vector is shown by the arrowhead.

Triangle Law Of Vector Addition: If two sides of a triangle taken in order, represent the magnitudes and directions of two vectors, the third side, taken in the opposite order, represents the magnitude and direction of the resultant of the two vectors.

If three vectors can be represented by the three sides of a triangle, taken in order, the resultant of the vectors is a zero vector.

Parallelogram Law Of Vector Addition: If two adjacent sides of a parallelogram represent the magnitudes and directions of two vectors, then the diagonal, drawn through the intersection of the two sides of the parallelogram, represents the magnitude and direction of the resultant of the two vectors. In this case the point of intersection is the initial point of the two vectors and their resultant.

Polygon Law Of Vector Addition: If the magnitudes and directions of a number of vectors are represented by the sides of a polygon, taken in order, then the last side, taken in the opposite order, represents the magnitude and direction of the resultant of the vectors.

• If any vector is split into two or more vectors such that the original vector becomes the resultant of the split parts or components of the vector, then this splitting is called the resolution of vectors.
• Component of a vector in the same direction as the vector has the same magnitude as the vector itself.
• No vector has a component at right angles to itself.
• When the position of a point with respect to the origin is represented by a vector, then that vector is called the position vector.
• The apparent velocity of a body, with respect to another body at rest or in motion, is called its relative velocity.
• The apparent acceleration of a body, with respect to another body moving with or without, is called its relative acceleration.
• The scalar product or dot product of two vectors is a scalar, whereas the vector product or cross product of two vectors is another vector.
• A body thrown obliquely from the earth’s surface or from a point close to it is called a projectile.
• The path of a projectile is parabolic except for those projected along the vertical direction. In that case, it is a straight line.

Resultant of \(\vec{a}\) and \(\vec{b}\), when the angle between them is \(\alpha\), is \(\vec{c}\) such that \(c=\sqrt{a^2+b^2+2 a b \cos \alpha}\). [equation giving the magnitude of the resultant]

If the angle between \(\vec{a}\) and \(\vec{c}\) is \(\theta\), then \(\tan \theta=\frac{b \sin \alpha}{a+b \cos \alpha}\) [equation giving the direction of the resultant]

1. When \(\alpha=0\), \(c=a+b=c_{\max }\) (maximum possible value of the resultant) and \(\theta=0\).
2. For \(\alpha=\pi\), \(c=|a-b|=c_{\text {min }}\) (minimum possible value of c) and \(\theta=0\), (when a>b) or \(\theta=\pi\) (when a<b)
   • Note: α or θ is usually measured from \(\vec{a}\).
3. When \(\alpha=\frac{\pi}{2}\) then \(c=\sqrt{a^2+b^2}\) and \(\theta=\tan ^{-1} \frac{b}{a}\).

Characteristics Of Vector Addition:

1. \(\vec{A}+\vec{B}=\vec{B}+\vec{A}\) [Commutative rule]
2. \(\vec{A}+(\vec{B}+\vec{C})=(\vec{A}+\vec{B})+\vec{C}\)[Associative rule]
3. \(\vec{A}-\vec{B}=\vec{A}+(-\vec{B})\)
4. \((-n) \vec{A}=n(-\vec{A})=-n \vec{A}\)

If two components of \(\vec{R}\) are \(\vec{a}\) and \(\vec{b}\), angle between \(\vec{R}\) and \(\vec{a}\) is \(\alpha\), and angle between \(\vec{R}\) and \(\vec{b}\) is \(\beta\), then

a = \(\frac{R \sin \beta}{\sin (\alpha+\beta)} \text { and } b=\frac{R \sin \alpha}{\sin (\alpha+\beta)}\)

When \(\alpha+\beta=\frac{\pi}{2}\) then \(a=R \cos \alpha, b=R \sin \alpha\)

If coplanar vectors \(\vec{P}, \vec{Q}\) and \(\vec{R}\) are at angles \(\alpha, \beta\) and \(\gamma\) respectively with respect to the positive x-axis, then the component of their resultant \(\vec{F}\) along the positive x-axis, \(F_x=P \cos \alpha+Q \cos \beta+R \cos \gamma\)

and component of \(\vec{F}\)

along the positive y-axis \(F_y=P \sin \alpha+Q \sin \beta+R \sin \gamma\)

and hence, \(F=\sqrt{F_x^2+F_y^2}\)

When \(\vec{F}\) makes an angle \(\theta\) with the positive x-axis \(\theta=\tan ^{-1} \frac{F_y}{F_x}\)

Taking O as the origin of the three-dimensional cartesian coordinate system, we get the position vector of A(x, y, z) as, \(\vec{r}=\overrightarrow{O A}=x \hat{i}+y \hat{j}+z \hat{k}\)

∴ r = \(\sqrt{x^2+y^2+z^2}\)

If \(\vec{r}\) makes angles \(\alpha, \beta, \gamma\), with x, y, z axes respectively, then the direction cosines of \(\vec{r}\) are \(\cos \alpha=\frac{x}{r}, \cos \beta=\frac{y}{r}\) and \(\cos \gamma=\frac{z}{r}\) where, \(\cos ^2 \alpha+\cos ^2 \beta+\cos ^2 \gamma=1\)

The resultant of \(\vec{r}_1=x_1 \hat{i}+y_1 \hat{j}+z_1 \hat{k}\) and \(\vec{r}_2=x_2 \hat{i}+y_2 \hat{j}+z_2 \hat{k}\) is \(\vec{r}_1+\vec{r}_2=\left(x_1+x_2\right) \hat{i}+\left(y_1+y_2\right) \hat{j}+\left(z_1+z_2\right) \hat{k}\)

If \(\theta\) is the angle between \(\vec{A}\) and \(\vec{B}\), vector and scalar products of the vectors \(\vec{A}\) and \(\vec{B}\) are respectively \(\vec{A} \times \vec{B}=A B \sin \theta \hat{n} \text { and } \vec{A} \cdot \vec{B}=A B \cos \theta\)

(where \(\hat{n}\) is the unit vector perpendicular to both \(\vec{A}\) and \(\vec{B}\))

If the velocities of two particles are \(\vec{v}_1\) and \(\vec{v}_2\), then the relative velocity of the second particle with respect to the first is, \(\vec{v}=\vec{v}_2-\vec{v}_1\)

If velocity and angle of projection of a projectile are u and \(\alpha\) respectively, then

1. Maximum height, \(H=\frac{u^2 \sin ^2 \alpha}{2 g}\)
2. Time of flight, \(T=\frac{2 u \sin \alpha}{g}\)
3. Range of the projectile, \(R=\frac{u^2 \sin 2 \alpha}{g}\)
4. Equation of the locus of the projectile, y = \(x \tan \alpha-\frac{g}{2 u^2 \cos ^2 \alpha} x^2 \text { (a parabola). }\)

 

Vector Match The Columns

Question 1. \(\vec{A}=(3 \hat{i}+4 \hat{j}-5 \hat{k})\)

Answer: 1-B, 2-C, 3-D, 4-D

Question 2. \(|\vec{A}|=2 \text { and }|\vec{B}|=4\). \(\theta\) is the angle between \(\vec{A} \text { and } \vec{B} \text {. }\)

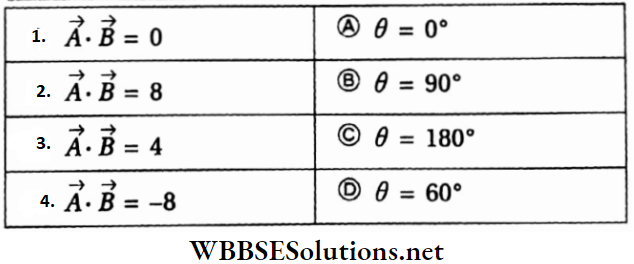
Answer: 1-B, 2-A, 3-D, 4-C

Question 3. \(|\vec{A}|=2 \text { and }|\vec{B}|=4\). \(\theta\) is the angle between A and B

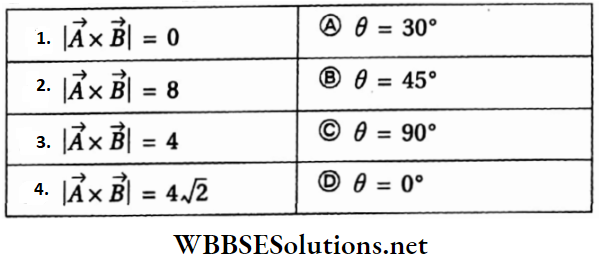
Answer: 1-D, 2-C, 3-A, 4-B

Question 4. If θ is the angle between two vectors A and B, then match the following columns.

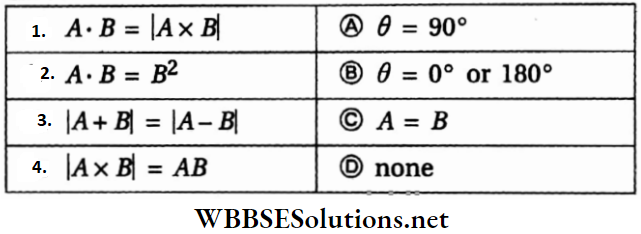
Answer: 1-D, 2-B, C, 3-A, 4-A

Question 5. Vector A is pointing eastwards and vector B is northwards. Then match the following two columns.

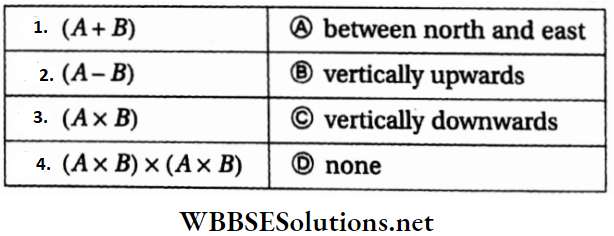
Answer: 1-A, 2-D, 3-B, 4-D

Question 6. Given below in Column A is the relations between vectors \(\vec{a}\), \(\vec{b}\) and \(\vec{c}\) and in Column B are the orientations of \(\vec{a}\), \(\vec{b}\) and \(\vec{c}\) in the XY plane.

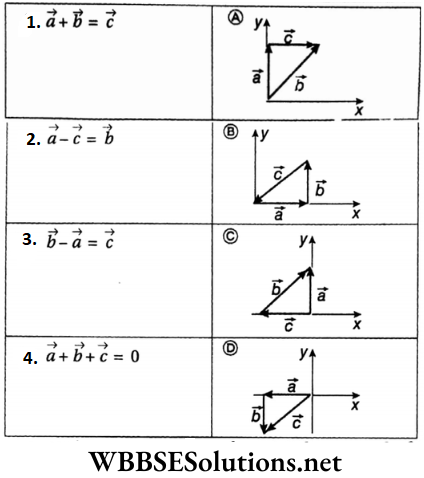
Answer: 1-D, 2-C, 3-A, 4-B

Question 7. For a projectile thrown from the ground at an angle with the horizontal

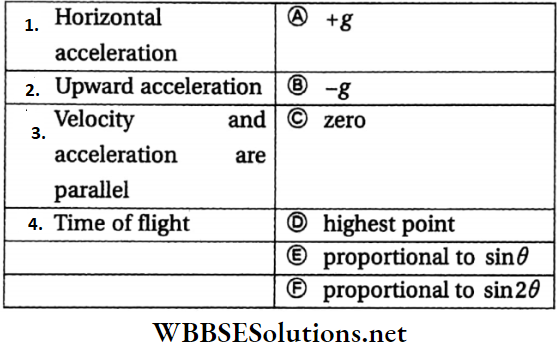
Answer: 1-C, 2-B, 3-D, 4-E

Vector Comprehension Type Questions And Answers

Read the following passages carefully and answer the questions at the end of them.

Question 1. A farmer goes 500 m due north, 400 m due east and 200 m due south to reach his field. He takes 20 min to reach the field.

1. How much distance does he to walk to reach the field?

1. 900 m
2. 1100 m
3. 1300 m
4. 700 m

Answer: 2. 1100 m

2. What is the displacement from his house to the field?

1. 550 m
2. 700 m
3. 500 m
4. 714 m

Answer: 3. 500 m

3. What is the average speed of the farmer during the walk?

1. 35 m • min^-1
2. 63 m • min^-1
3. 55m · min^-1
4. 65 m · min^-1

Answer: 3. 55m · min^-1

4. What is the average velocity of the farmer during the walk?

1. 27 m · min^-1
2. 30 m · min^-1
3. 35 m · min^-1
4. 25 m · min^-1

Answer: 4. 25 m · min^-1

Question 2. A man crosses a river in a boat. If he crosses the river in minimum time he takes 10 min with a drift 120 m. If he crosses the river taking the shortest path, he takes 12.5 min.

1. What is the width of the river?

1. 250 m
2. 200 m
3. 300 m
4. 230 m

Answer: 2. 200 m

2. What is the velocity of the boat in still water?

1. 21 m · min^-1
2. 24 m · min^-1
3. 20 m · min^-1
4. 18 m · min^-1

Answer: 3. 20 m · min^-1

3. What is the speed of the current?

1. 13 m · min^-1
2. 12 m · min^-1
3. 14 m · min^-1
4. 15 m · min^-1

Answer: 2. 12 m · min^-1

Question 3. A particle is projected from the surface of the earth with a speed of 20 m · s^-1 at an angle 30° with the horizontal.

1. The time of flight of that particle is

1. 3 s
2. 4s
3. 2s
4. 1s

Answer: 3. 2s

2. The range of that particle is

1. 10 m
2. 12√2 m
3. 20√3 m
4. 30 m

Answer: 3. 20√3 m

3. The maximum height the particle can reach is

1. 3 m
2. 7 m
3. 5m
4. 12 m

Answer: 3. 5m

Vector Integer Answer Type Questions

In this type, the answer to each of the questions is a single-digit Integer between 0 and 9.

Question 1. A projectile is launched from the ground and it returns to the ground level. The horizontal range of the projectile is R = 175 m. If the horizontal component of the projectile’s velocity at any instant is 25 m · s^-1, then determine the time of flight of the projectile.
Answer: 7

Question 2. A food packet is to be dropped by a helicopter on a flood relief mission. The helicopter is moving horizontally with a constant speed of y = 50 m · s^-1. At the time of dropping the food packet, it is at a height of 2 km from the ground level. For the person shown to receive the packet, what should be the value of x in km? [Given, g = 10 m · s^-2]

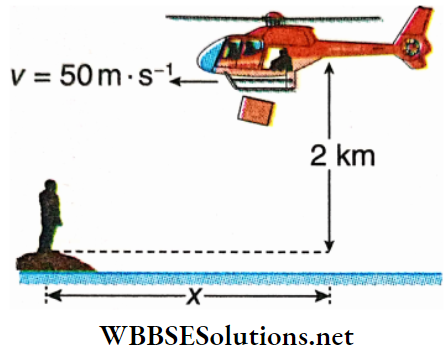
Answer: 1

Question 3. Two men are running on a straight north-south track. Person A moves north with a speed of 5 m · s^-1 while B moves south with a speed of 2 m · s^-1. Determine the velocity (magnitude only) of

1. A with respect to B.
2. The ground with respect to A.
3. B with respect to A.

Answer: 7, 5, 7