WBCHSE Class 11 Physics Notes For Statics

Statics – Rigid Bodies

Rigid Bodies: Statics is a branch of mechanics where the equilibrium of bodies under the action of a number of forces and the conditions for equilibrium are studied.

The concept of rigid bodies has to be introduced in the discussions of rotation, in particular. The particle description of material bodies is not sufficient in that context.

Rigid Bodies Definition: A body that does not change its shape or size under the action of forces is called a rigid body.

No substance is perfectly rigid. However, most material bodies may be assumed to be rigid when the forces acting on them do not exceed a certain limit.

Read and Learn More: Class 11 Physics Notes

Statics – Resultant Of Parallel Forces

Forces acting in the same direction are called parallel forces. \(\vec{F}_1, \vec{F}_2 \text { and } \vec{F}_3\) are examples of like parallel forces. When two forces act in opposite directions, they constitute a pair of, unlike parallel forces. \(\vec{F}_4\) and \(\vec{F}_5\) are two unlike parallel forces.

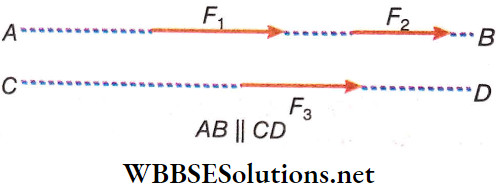
The straight line along which a force acts is called the line of action of that force. For example, AB is the line of action of forces \(\vec{F}_1\) and \(\vec{F}_2\), CD is the line of action of force \(\vec{F}_3\).

Resultant Of Two Like Parallel Forces: Let \(\vec{F}_1\) and \(\vec{F}_2\) be a pair of like parallel forces acting at points A and B. Their resultant has to be calculated. O is a point on the plane of the forces.

Line OX is drawn perpendicular to the lines of action of \(\vec{F}_1\) and \(\vec{F}_2\). OX is taken as the x-axis. OY is the y-axis on the same plane.

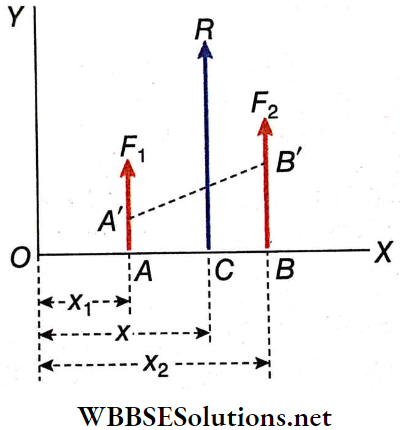
Since \(\vec{F}_1\) and \(\vec{F}_2\) do not have any components along the x-axis, the resultant \(\vec{R}\)(say) will also have no component along the x-axis and its line of action will be perpendicular to the line OX, i.e., parallel to the lines of action of \(\vec{F}_1\) and \(\vec{F}_2\). Hence, component of \(\vec{R}\) along y-axis is, R = F₁ + F₂….(1)

Hence, the magnitude of the resultant is the sum of the magnitudes of the forces and its direction will be the same as the direction of the parallel forces.

To find the line of action of R, let us assume that \(\vec{R}\) acts at point C. \(\vec{R}\) is the resultant of \(\vec{F}_1\) and \(\vec{F}_2\). It implies that the effect of forces \(\vec{F}_1\) and \(\vec{F}_2\) together, should be the same as that of R acting alone.

Hence, the algebraic sum of moments due to \(\vec{F}_1\) and \(\vec{F}_2\) about the point O should be equal to the moment of \(\vec{R}\) about O,

or, F₁ x OA + F₂ x OB = R x OC

or, F₁(OC-AC) + F₂(OC+CB) = (F₁ + F₂) x OC

or, F₁ x AC = F₂ x BC

or, \(\frac{F_1}{F_2}=\frac{B C}{A C}\)….(2)

Hence, the line of action of the resultant divides the distance AB into two parts in the inverse ratio of the magnitudes of the forces.

Suppose the line A’B’ is obtained by joining any two points on the lines of action of F₁ and F₂. Obviously, the line of action of the resultant divides it into two parts in the inverse ratio of the magnitude of the forces \(\vec{F}_1\) and \(\vec{F}_2\).

Position of the point C can also be determined in terms of points A and B. If OA = x₁, OB = x₂ and OC = x, then, taking moments about the point O of the forces \(\vec{F}_1\), \(\vec{F}_2\) and resultant \(\vec{R}\) we get,

∴ \(F_1 x_1+F_2 x_2=R \times x=\left(F_1+F_2\right) x\)

∴ x = \(\frac{F_1 x_1+F_2 x_2}{F_1+F_2}\)…(1)

Resultant of Two Unlike Parallel Forces: Let \(\vec{F}_1\) and \(\vec{F}_2\) be two unlike parallel forces acting at points A and B as shown. Let \(F_1\) > \(F_2\). Suppose \(\vec{R}\) is the resultant of these two forces. Then, R = \(F_1\) – \(F_2\)

The resultant acts along the direction of the greater force.

Taking moments about point O and from the definition of resultant we get, F₁ x OA – F₂ x OB = R x OC

or, F₁(OC+ CA) – F₂(OC+ CB) = (F₁ – F₂) x OC

or, \(\frac{F_1}{F_2}=\frac{B C}{A C}\)

and x = \(\frac{F_1 x_1-F_2 x_2}{F_1-F_2}\)…(2)

Hence, the line of action of the resultant divides externally the distance of separation of the two forces in the inverse ratio of their magnitudes.

Resultant Of Three Or More Parallel Forces: When a body is acted upon by the parallel forces \(\vec{F}_1\), \(\vec{F}_2\), \(\vec{F}_3\),…… simultaneously, the resultant force \(\vec{R}\) can be obtained by framing an equation similar to equation (1).

R = \(F_1+F_2+F_3+\cdots=\sum_i F_i\)….(5)

Any one of the forces may be taken as positive. Correspondingly, forces in the same direction as this force are positive and those in the opposite direction are negative. If the forces are coplanar. the distance of the line of action of the resultant from any point O on that plane is,

x = \(\frac{F_1 x_1+F_2 x_2+F_3 x_3 \cdots}{R} \text { or, } x=\frac{\sum_i F_i x_i}{\sum_i F_i}\)…(6)

where x₁, x₂, x₃,…are the perpendicular distances of the point O from the lines of action of the forces, \(\vec{F}_1\), \(\vec{F}_2\), \(\vec{F}_3\)… etc.

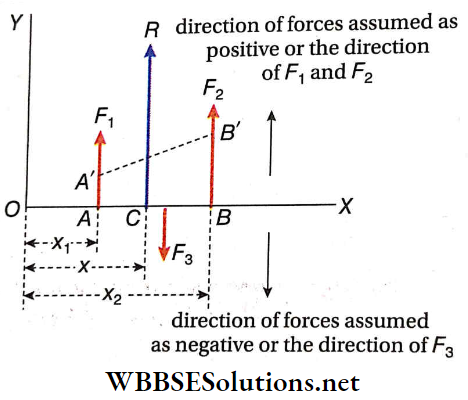
The moment of the resultant about the point O can be positive or negative. The direction of rotation caused by the resultant about the point O is determined by this positive or negative sign.

 

 Statics- Centre Of Gravity

It is known that an extended object is an aggregation of a large number of particles. If the masses of these constituents are m₁, m₂, m₃, …., the earth pulls these particles with respective forces m₁g, m₂g, m₃g,…. towards its centre.

• The resultant of these parallel forces acting on the particles is the weight W of the object. This weight W, acts vertically downward through a definite point.
• This point is called the centre of gravity (G) of the body. Like the centre of mass, the centre of gravity may be located inside or outside the body.
• The respective distances between the particles remain fixed. Hence, the resultant of the weights also remains the same both in magnitude and in direction and acts through the same point.
• Thus, it can be stated that the position of the centre of gravity of a body does not depend on the orientation of the body.

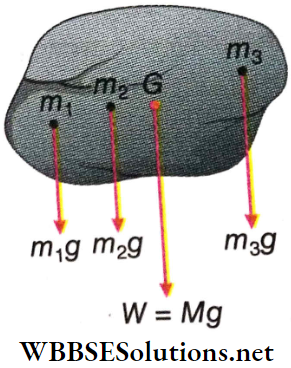
Centre Of Gravity Definition: The force of gravity that is exerted on an extended object always acts through a unique point. This point; is the centre of gravity of the object.

To Find The Position Of The Centre Of Gravity: The position of the centre of gravity (G) can be determined using the rules for finding the resultant of parallel forces. The magnitude of the resultant is equal to the weight of the body:

W = m₁g + m₂g + m₃g + …. = Mg

[Here, M=m₁ + m₂ + m₃ + = total mass of the object]

Let the coordinates of \(m_1, \quad m_2, \ldots\) be \(\left(x_1, y_1, z_1\right)\), \(\left(x_2, y_2, z_2\right)\), …respectively and the point of action of the resultant W, i.e., the coordinates of the centre of gravity be (x, y,z).

∴ \(\left.\begin{array}{l}
\begin{array}{rl}
therefore \quad x & =\frac{m_1 g x_1+m_2 g x_2+\cdots}{m_1 g+m_2 g+\cdots} \\
& =\frac{m_1 x_1+m_2 x_2+\cdots}{m_1+m_2+\cdots} \\
& =\frac{\sum_i m_i x_i}{\sum_i m_i}
\end{array} \\
\text { Similarly, } y=\frac{\sum_i m_i y_i}{\sum_i m_i} \text { and } z=\frac{\sum_i m_i z_i}{\sum_i m_i}
\end{array}\right\}\)

Some Important Information About The Centre Of Gravity: To establish equation (1), the magnitude of g is assumed to be the same for all particles constituting the body. This assumption is acceptable for small objects only, where the variation of g is negligible. But if the body is very large, the magnitude of g varies from point to point and equation (1) is not applicable.

Equation (1) of this section and equation (2) are identical. Hence, if the magnitudes of acceleration due to gravity at all points within a body are the same, the centre of mass and the centre of gravity become identical.

Uniqueness Of Centre Of Gravity: A body cannot have more than one centre of gravity. This is called the uniqueness of the centre of gravity. Let us assume a body has two centres of gravity G and G’. From the definition of centre of gravity, irrespective of the orientation of the body, its weight should act through G and G’.

But the weight of a body always acts vertically downwards. Therefore, GG’ must always be a vertical line. But for aflPoftlntations of the body, GG’ cannot be vertical. Hence a body having two centres of gravity is not a possibility.

Statics – Difference Between Centre Of Mass And Centre Of Gravity

The Centre of mass of a body is such a point that a force applied at this point produces linear motion only and does not produce any rotational motion.

1. The Centre of gravity is the point through which the weight of a body acts vertically downwards.
2. As the mass of a body remains constant anywhere in the universe, a body always has a definite centre of mass. But at places where the weight of the body becomes zero, there is no centre of gravity, for example, a body inside an artificial satellite rotating around the earth or a free-falling body is weightless. So in these cases, the body has no centre of gravity.
3. If the magnitude of acceleration due to gravity remains the same at all points of a body, the centre of mass and the centre of gravity will be identical.
   • But if the magnitude of gravitational acceleration is different at different points of a body, then the centre of mass will not coincide with the centre of gravity, For example, for very large mountains like the Himalayas acceleration due to gravity is greater at the bottom of the mountain, that’s why even for an object of uniform density earth’s attraction is greater at the bottom.
   • Again position of the centre of mass depends only on the density and not on the weight of the body. That is why for massive and tall objects like these centre of gravity remains below the centre of mass.

Statics – Centre Of Mass And Centre Of Gravity Numerical Examples

Example 1. A rod of weight W is kept horizontally on two knife edges with a distance d between them. The Centre of gravity of the rod is at a distance x from A. Find the normal reactions at points A and B.

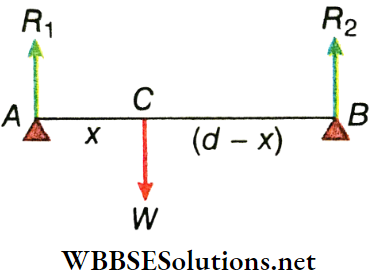
Solution:

Let the centre of gravity of the rod be C. Normal reactions at A and B are R₁ and R₂. Weight W through C acting downwards, and R₁ and R₂ are the three coplanar forces that keep the rod in equilibrium.

Hence, W- R₁ – R₂ = 0 or, W = R₁ + R₂

Taking the moment of the forces about C, \(-R_1 \cdot x+R_2(d-x)=0 \text { or, } R_1 x=R_2(d-x)\)

or, \(x\left(R_1+R_2\right)=R_2 d \text { or, } W x=R_2 d\)

∴ \(R_2=\frac{x}{d} W\)

and \(R_1=W-R_2=W-\frac{W x}{d}=W\left(1-\frac{x}{d}\right)\)

Example 2. Show that the centre of gravity of three equal weights suspended from three vertices of a triangle coincides with the centre of mass of the triangle.
Solution:

Let the median of the triangle ABC be AD. Identical weights W are hung from each of the vertices A, B and C. Resultant of the weights suspended from B and C = 2W and it acts from the point D.

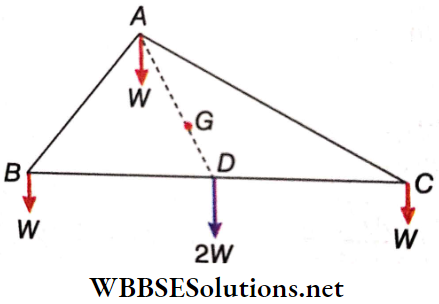
The resultant of the forces 2 W at D and W at A is 3 W. Suppose this resultant acts at G.

Hence, W x AG = 2 W x DG

or, AG = 2 DG

Thus, G is the point of intersection of the medians of the triangle, which is the centre of mass.

Example 3. A person of mass 80 kg is standing on the top of an 18 kg ladder of length 6 m. The upper end of the ladder rests on a smooth vertical wall and the lower end is on the ground 3 m away from the vertical wall. What should be the minimum coefficient of friction between the floor and the ladder so that the system remains in equilibrium?
Solution:

Given that, for the ladder weight W acts through the centre of gravity, C (mid-point of AB) of the ladder.

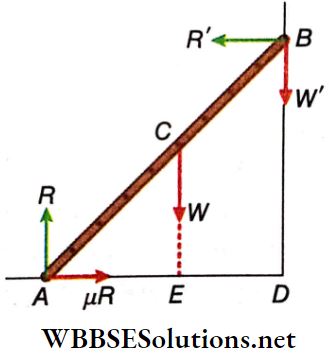
Weight W’ of the man acts at B downwards.

Normal reaction R’ by the wall acts at B.

Normal reaction R by the ground acts at A.

Limiting friction that acts along the floor at A, f = μR where, n is the coefficient of friction, required for equilibrium.

At equilibrium, the sum of all horizontal forces and the sum of all vertical forces will be zero separately.

⇒ \(\mu R-R^{\prime}=0 \text { or, } \mu R=R^{\prime}\)…(1)

⇒ \(R-W-W^{\prime}=0 \text { or, } R=W+W^{\prime}\)…(2)

Again the sum of the moments of all forces taken about A will be zero.

∴ \(R^{\prime} \times B D-W \times A E-W^{\prime} \times A D=0\)

or, \(\mu\left(W+W^{\prime}\right) \times B D=W \times A E+W^{\prime} \times A D\)

∴ \(\mu=\frac{W \times A E+W^{\prime} \times A D}{\left(W+W^{\prime}\right) \times B D}\)

Here, AB = \(6 \mathrm{~m}, A D=3 \mathrm{~m}\).

∴ AE = \(\frac{A D}{2}=\frac{3}{2} \mathrm{~m} ; B D=\sqrt{A B^2-A D^2}=5.2 \mathrm{~m}\)

W = \(18 \mathrm{~kg} \times g ; W^{\prime}=80 \mathrm{~kg} \times \mathrm{g}\)

∴ \(\mu=\frac{18 \times \frac{3}{2}+80 \times 3}{(18+80) \times 5.2}=0.52\).

Example 4. A uniform cylinder of diameter 8 cm is kept on a rough inclined plane, whose angle of inclination with the ground is 30°. What should be the maximum height of the cylinder so that it does not topple?
Solution:

The cylinder ABCD is kept on the plane of inclination 30°. Suppose the cylinder is on the verge of toppling over when its height is 2h. At this stage, the line of action of gravity must pass vertically through the endpoint A at the base of the cylinder.

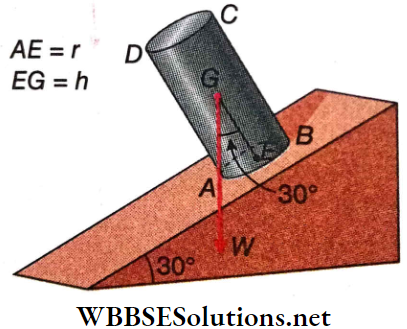
From \(tan 30^{\circ}=\frac{A E}{E G}\)

or, \(\frac{1}{\sqrt{3}}=\frac{r}{h} or, h=\sqrt{3} r=4 \sqrt{3} \mathrm{~cm}\)

Hence, maximum permissible height = 2h = x 4√3 = 8√3 cm.

Example 5. A hollow cylinder of height 100 cm and diameter 8 cm has its top end open. It contains water up to a height of 50 cm. The mass per square centimetre of the hollow cylinder is 9 g. Find the height of the centre of gravity of the water cylinder system from the base of the cylinder.
Solution:

The Centre of gravity of the curved surface of the empty cylinder is 50 cm above its base at P.

The weight of the base of the empty cylinder

= π(4)² x 9 x g dyn [g = acceleration due to gravity]

Weight of curved surface of the cylinder = 2π x 4 x 100 x 9 x g dyn. Its centre of gravity is at G₁, where PG₁ = 50 cm.

Weight of water in the cylinder =π(4)² x 50 x 1 x g dyn and the centre of gravity of this water is at G₂ which is 25 cm above the base of the cylinder.

Suppose the centre of mass of the cylinder filled with water is at G, which is h cm above its base. The centre of gravity coincides with this centre of mass.

Taking moments of all the weights about P, \(2 \pi \times 4 \times 100 \times 9 \times G_1 P+\pi \times 16 \times 50 \times 1 \times G_2 P\)

= \((\pi \times 16 \times 9+2 \pi \times 4 \times 100 \times 9+\pi \times 16 \times 50 \times 1) \times G P\)

or, \(2 \pi \times 4 \times 100 \times 9 \times 50+\pi \times 16 \times 50 \times 1 \times 25\)

= \((\pi \times 16 \times 9+2 \pi \times 4 \times 100 \times 9 +\pi \times 16 \times 50 \times 1) \times h\)

or, h = 46.7 cm.

Example 6. A uniform narrow rod of mass M and length 2 L is kept vertically, along the y-axis, on a smooth horizontal plane. The lower end of the rod coincides with the origin (0,0). Due to a slight disturbance at time t = 0, the rod slides along the positive x-axis and begins to fall. Determine

1. The shift in the centre of gravity during the fall,
2. The equation of the locus of a point at a distance r from the lower end of the rod also mentions the shape of the locus.

Solution:

Initially (0, L) was the coordinates of the centre of gravity of the rod. Let B be the point at a distance r, from point O (0, 0), on the rod. So, (0, r) denotes the point B.

At t = 0, the rod is disturbed and it falls. During this fall there is no other force acting on it except the downward gravitational force.

1. We know that the centre of gravity is influenced only by an external force which is gravity in this case. So the centre of gravity will be shifted vertically downwards from (0, L) to (0, 0).
2. Let (x, y) be the position of B’ at any moment dining the fall of the rod, where B’ is the point at a distance r, from the lower end O’ of the rod.

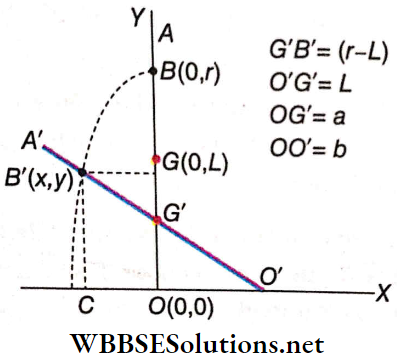
From, \(\frac{O G^{\prime}}{O^{\prime} G^{\prime}}=\frac{C B^{\prime}}{O^{\prime} B^{\prime}}\)

or, \(\frac{a}{L}=\frac{y}{r}\)

Again, \(\frac{O^{\prime} O}{O C}=\frac{O^{\prime} G^{\prime}}{G^{\prime} B^{\prime}}\)

or, \(\frac{O C}{G^{\prime} B^{\prime}}=\frac{O^{\prime} O}{O^{\prime} G^{\prime}} or, \frac{x}{r-L}=\frac{b}{L}\)

∴ \(\frac{x^2}{(r-L)^2}=\frac{b^2}{L^2}=\frac{L^2-a^2}{L^2}=1-\frac{a^2}{L^2}=1-\frac{y^2}{r^2}\)

or, \(\frac{x^2}{(r-L)^2}+\frac{y^2}{r^2}=1\)

This is the equation of the locus of a point (x, y) and it is elliptical with its centre at the origin.

Example 7. A circular metal plate of uniform thickness has a radius of 10 cm. A hole of radius 4 cm is punched on the plate a little away from its centre. The centres of the plate and of the hole are 5 cm apart. Find the centre of gravity of the plate with the hole.
Solution:

The Centre of gravity of the plate before the hole was punched was at its centre G. The centre of gravity of the circular part before the punching was at its centre G₁. Suppose the centre of gravity of the plate with the hole is at G₂, on the line GG₁.

Let W₁ = weight of the plate with a hole, W₂ = weight of the disc that is cut off

∴ \(W_1 \times G G_2=W_2 \times G G_1 \text { or, } G G_2=\frac{W_2}{W_1} \times G G_1\)

But \(G G_1=5 \mathrm{~cm}, W_1=\pi\left(10^2-4^2\right) \times \rho\)(ρ= weight per unit area) and \(W_2=\pi \times 4^2 \times \rho\)

∴ \(G G_2=\frac{4^2}{10^2-4^2} \times 5=\frac{20}{21} \mathrm{~cm}\)

Hence, the centre of gravity of the system will be \(\frac{20}{21}\) cm to the left of the centre of the plate.

Example 8. A mass of 10 kg is suspended using two strings. One string makes an angle of 60° with the vertical. What should be the angle made by the other string so that the tension in the string will be minimum? Find the tension in each wire.
Solution:

As the mass is in equilibrium, from the force diagram we get \(T_1 \cos \theta_1+T_2 \cos \theta_2=m g\)

or, \(T_1 \cos \theta_1=m g-T_2 \cos \theta_2\)….(1)

Also \(T_1 \sin \theta_1=T_2 \sin \theta_2\)…(2)

From (2) and (1) we get, \(\frac{\sin \theta_1}{\cos \theta_1}=\frac{T_2 \sin \theta_2}{m g-T_2 \cos \theta_2}\)

or, \( m g \sin \theta_1=T_2\left(\sin \theta_1 \cos \theta_2+\cos \theta_1 \sin \theta_2\right)\)

∴ \(T_2=\frac{m g \sin \theta_1}{\sin \left(\theta_1+\theta_2\right)}\)….(3)

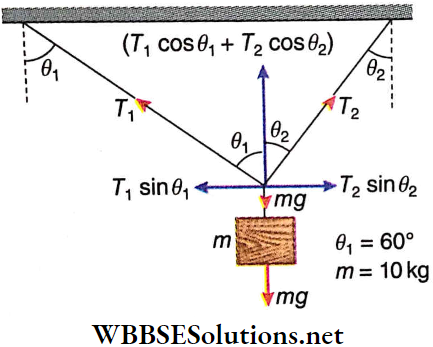
As m, g and θ are constants, the minimum value of T₂ corresponds to the maximum value of sin(θ₁ + θ₂) i.e., sin(θ₁ + θ₂) = 1 = sin90°

∴ θ₁+ θ₂ = 90° or, θ₂ = 90° – θ₁ = 90° -60° = 30°

From equation (3) we get, \(T_2=\frac{10 \times g \sin 60^{\circ}}{1}=84.87 \mathrm{~N}\)

From equation (2) we get, \(T_1=\frac{T_2 \sin \theta_2}{\sin \theta_1}=\frac{5 \sqrt{3} \times 9.8 \times \sin 30^{\circ}}{\sin 60^{\circ}}=49 \mathrm{~N} \text {. }\)

Example 9. A chain of mass m and length l is kept on a horizontal frictionless table, such that \(\frac{1}{4}\) th of the length of chain is the hanging part of the chain onto the table.
Solution:

Mass per unit length of the chain = \(\frac{m}{l}\)

∴ Mass of hanging part of the chain = \(\frac{m}{l}\) x  \(\frac{l}{4}\) =  \(\frac{m}{4}\)

∴ The Centre of gravity of this portion is at \(\frac{l}{4 \times 2}=\frac{l}{8}\) below the top of the table.

∴ Work to be done to lift the hanging part of the chain = \(\frac{m}{4} \times g \times \frac{l}{8}=\frac{m g l}{32}\)

Statics – Equilibrium Of A Body Under Gravity

Suppose a chair is at rest with its legs resting on the floor. This stationary state of the chair is its equilibrium position. If the chair is tilted a little, it has to be held in that position to prevent it from falling.

• This shows that this position is not the equilibrium position of the chair. On tilting it further the chair falls to the ground on its side and comes to rest again.
• This is another equilibrium position of the chair. In general, under the influence of gravity, a body may have one or more equilibrium states.

Under The Influence Of Gravity, A Body May Stay In Three Types Of Equilibrium States:

1. Stable Equilibrium: If a substance is disturbed from its equilibrium state, and if on release it gets back to the same equilibrium state, then the body is initially in a stable equilibrium.
   • If a book, placed on a table with its largest surface resting on the table, is lifted a little by any one of the edges and then released, it falls back and returns to its initial state of equilibrium.
2. Unstable Equilibrium: If a body, when displaced a little from its equilibrium position, tends to move away further, then the body was in an unstable equilibrium.
   • Unstable Equilibrium Examples:
     • A pencil made to balance on its pointed end
     • An egg is made to stand on its narrow end (it is almost impossible to keep a pencil or an egg in this position by hand). If a slight push is given these fall over.
3. Neutral Equilibrium: Let a body be displaced a little from its state of equilibrium and then released. If it still remains in equilibrium in its new position, then it is said to be in a neutral equilibrium. Let a sphere be placed on a smooth horizontal plane.
   • On being pushed, it rolls a bit and comes to rest in a new position. Hence the sphere is said to be in a neutral equilibrium state. Different equilibrium states of a body can be ascertained by either of the following two methods.

Extension Of The Base: A chair on a floor rests with all four legs in contact with the floor. The quadrilateral obtained by joining the contact points of its legs with the floor is called the base of the chair. A body that has a larger base area compared to the area of its upper regions is always in stable equilibrium if the vertical line through its centre of gravity passes through its base.

From everyday experience, we know that, for an object of a given height, stability increases with the extent of the base area. For a stable object, the line of action of its weight always lies within its base.

Extension Of The Base Stable Equilibrium: Consider two possible ways of placing a brick on a horizontal surface. In the brick is placed on its largest face AB. This is a position of stable equilibrium and the lines of action of the weight W and the normal reaction R coincide. If we tilt the brick to stand it on the edge through point A, as shown, the lines of action of W and R do not lie on the same straight line.

• Consequently, when we release the brick, the couple created by W and R brings it back on the original base AB. Note that the line of action of the weight W still passes through the base AB when the brick is tilted. So the initial state of the brick is a state of stable equilibrium.
• To topple the brick, it needs to be tilted further about the edge through A so that the line of action of W goes outside the base AB. The brick is on the verge of toppling.
• The same brick is shown to be standing on one of its smaller faces like BC. In this case, it will be easier to topple the brick because the line of action of W goes outside the base BC on turning the brick through a smellier angle. But this initial state is also a stable equilibrium state. Thus, we can say that stability increases with the extent of the base area.

Unstable Equilibrium: A cone is shown balanced on its tip. Since the lines of action of W and R coincide, we can say that the cone is in equilibrium.

• However, a minute displacement from this position is enough to make it lose its balance and topple over. Thus, the cone standing on its tip is an example of unstable equilibrium.
• In this state, if the object is disturbed and then released, it does not return to its initial position but seeks a position of stable equilibrium.
• Note that when the cone is displaced slightly, the lines of action of W and R do not lie on the same straight line and the line of action of W does not pass through the base A. The bricks are at state of unstable equilibrium.

Neutral Equilibrium: Consider a ball resting on a horizontal surface as shown. In this situation, the lines of action of W and R coincide. We conclude that the ball is in equilibrium. If we roll the ball to a new position, we find that it is in a similar kind of equilibrium as before.

• As a matter of fact, the lines of action of W and R coincide in any orientation. Such an equilibrium is called a neutral equilibrium. When a body is displaced from a position of neutral equilibrium does not tend to return to its initial position; rather it settles into a new position of neutral equilibrium.
• Thus, if the line of action of its weight does not pass through the base of the body, it cannot remain in equilibrium. Once displaced from its equilibrium position, a body comes to a new equilibrium either by returning to the old base or on a new base.
• A chair is not stable when it stands on two legs, as the line of action of its weight does not pass through the baseline defined by the two legs. Quadrupeds are more stable than animals who walk on two legs.
• Hence, in the latter case, the technique of standing on two legs has to be learned. When a bucket full of water is carried in the right hand, the body is leaned towards the left in order to keep the line of action of the weight of the body and the bucket together within our feet.

Position Of Centre Of Gravity: Objects tend to move towards the centre of the earth due to the gravitational pull of the earth. Since the point of action of this attractive force (i.e., weight) is the centre of gravity of the body, the natural tendency of the centre of gravity is to attain the least height. Therefore, the lower the position of the centre of gravity of a body, the more stable its equilibrium.

If on displacing a body, it’s centre of gravity

1. Goes up, then the body was at a stable equilibrium initially,
2. Goes down, the body attains a more stable equilibrium than before,
3. Remains at the same height, and then the body is said to be in neutral equilibrium.

Position Of Centre Of Gravity Example:

1. A person standing with his feet apart is more stable than when his feet are together. By placing his feet apart, the person increases the base area so he can stand more comfortably.
   • When a person lies down, along with the increase in the base area, there is a considerable lowering of the centre of gravity. Hence, the lying-down position is the most stable equilibrium for a person.
2. Boxes and books, when placed on the floor, are usually kept with their largest surfaces in contact with the ground. This lowers the centre of gravity and the equilibrium becomes most stable.
3. Passengers are not allowed to stand on the upper deck of a double-decker bus in order to keep the centre of gravity low.
4. Hydrometers, lactometers, special types of toys, etc. are loaded with lead in their lower part (base) so that the centre of gravity of the system remains very low. Hence, the vertical position is the most stable equilibrium position in these cases.
   • The toys can be tilted about their bases through large angles, but they will come back to their vertical positions as soon as they are released. Hence, each of these toys has only one state of equilibrium.

Relationship Of The Centre Of Gravity And Gravitational Potential With Different Types Of Equilibrium: Gravitational potential energy is related to the position of the centre of gravity of a body. Potential energy depends on the height of the centre of gravity from the ground.

• Lower the position of the centre of gravity, lower the potential energy and higher the stability of the body.
• Thus, when the centre of gravity of a body or a system attains the lowest possible height and hence has the lowest possible gravitational potential energy, the body achieves stable equilibrium. When the centre of gravity is at its maximum height, potential energy is also the maximum and therefore, the equilibrium becomes unstable.
• The body tends to deviate from that position to a state of lower potential energy.
• For a body in neutral equilibrium, the height of the centre of gravity does not change when it is displaced and the potential energy remains the same. Hence, the body remains in equilibrium even in the displaced state.

Statics Conclusion

Centre Of Mass is a unique point for an extended object or a system of particles such that, any force applied through that point produces only translational motion of the body, but no rotational motion.

1. No object can have more than one centre of mass,
2. The centre of mass of an object can be outside the material of the object.
3. The position of the centre of mass changes as the shape of an object changes.

The vector quantity which tends to rotate a body by the combination of the force acting on the body and the perpendicular distance of the line of action of that force from the axis of rotation is called the moment of the applied force with respect to that axis of rotation.

The magnitude of the moment of force is expressed by the product of the magnitude of the force and the perpendicular distance of the force from the axis of rotation.

The vector form of the relation between the moment of force and the applied force is \(\vec{G}=\vec{r} \times \vec{F}\).

• A number of forces acting in the same direction are called parallel forces. Two forces acting in mutually opposite directions are called unlike parallel forces.
• The straight line along which a force acts is called the line of action of that force.
• The line of action of the resultant of two like parallel forces acting at two different points on a body divides the distance between the like parallel forces in inverse ratio of the magnitudes of the two forces.
• The line of action of the resultant of two unlike parallel forces acting at two different points on a body divides externally the distance between the unlike parallel forces in inverse ratio of the magnitudes of the forces.
• A body is said to be in equilibrium when it is at rest moving with uniform linear velocity moving with a uniform angular speed or moving with both uniform linear and angular velocities.

Conditions of equilibrium of a body under the action of a number of coplanar forces:

1. 1st Condition: The resultant of all coplanar forces acting on the body should be zero. If this condition is fulfilled, the linear acceleration of the body will be zero.
2. 2nd Condition: The algebraic sum of the moments of coplanar forces acting on the body, taken about any point on the plane, should be zero. If this condition is fulfilled, the angular acceleration of the body will be zero.

Both conditions, satisfied simultaneously, keep a body in equilibrium.

• Conditions of equilibrium under the action of two forces: Two forces acting on a body should be equal, collinear and opposite to each other.
• Conditions of equilibrium under the action of three non-parallel forces:
• Three forces must be on the same plane and the resultant of any two should be equal and opposite to the third force and the lines of action of all three forces must pass through the same point.
• The single force that cancels the action of all other forces acting on a body is called an equilibrant.

Centre of gravity is a fixed point of an object through which the weight of the body acts. Its position is independent of the orientation of an object of fixed shape and volume.

1. No object can have more than one centre of gravity.
2. When acceleration due to gravity at all points of an object becomes the same, the centre of mass coincides with the centre of gravity.

At zero gravity, the centre of mass exists but the centre of gravity has no significance.

Under the action of gravity, objects may have three types of equilibrium:

1. Stable,
2. Unstable and
3. Neutral.

A body with a wider base compared to its upper regions, becomes stable. Also if the line drawn vertically through the centre of gravity of the object passes through its base, the object remains in a stable equilibrium.

Statics Useful Relations For Solving Numerical Problems

Let \(\vec{F}_1\) and \(\vec{F}_2\) be two like parallel forces. They act on two different points on x-axis with coordinates x₁ and x₂ respectively. The resultant (\(\vec{R}\)) of the above forces act through a point with coordinate x. Here,

R = \(F_1+F_2 \text { and } x=\frac{F_1 x_1+F_2 x_2}{F_1+F_2}\)

If \(\vec{F}_1\) and \(\vec{F}_2\) are unlike parallel forces then,

R = \(\left|F_1-F_2\right| \text { and } x=\frac{F_1 x_1-F_2 x_2}{F_1-F_2}\)

For n number of parallel forces

R = \(\sum_{i=1}^n F_i \text { and } x=\frac{\sum_{i=1}^n F_i x_i}{\sum_{i=1}^n F_i}\)

For n number of parallel forces \(R=\sum_{i=1}^n F_i \text { and } x=\frac{\sum_{i=1}^n F_i x_i}{\sum_{i=1}^n F_i}\)

For equilibrium og n number of coplanar forces, according to the 1st condition, \(R_x=\sum_{i=1}^n F_{i x}=0 \text { and } R_y=\sum_{i=1}^n F_{i y}=0\)

and according to the 2nd condition, \(\tau=\sum_{i=1}^n F_i x_i=0\)

Mathematical expressions for Lami’s theorem, \(\frac{P}{\sin (Q, R)}=\frac{Q}{\sin (R, P)}=\frac{R}{\sin (P, Q)}\)

Suppose particles of masses m₁, m₂,…..m_n constitute an extended object or a system of particles. The positional coordinates of the particles are (x₁, y₁, z₁, (x₂, y₂, z₂),…..(x_n, y_n, z_n) and that of the centre of mass are (x_cm, y_cm, z_cm), Then

∴ \(x_{\mathrm{cm}}=\frac{\sum_{i=1}^n m_i x_i}{\sum_{i=1}^n m_i}, y_{\mathrm{cm}}=\frac{\sum_{i=1}^n m_i y_i}{\sum_{i=1}^n m_i}, z_{\mathrm{cm}}=\frac{\sum_{i=1}^n m_i z_i}{\sum_{i=1}^n m_i}\)

Statics Assertion Reason Type Questions And Answers

Direction: These questions have statement 1 and statement 2. Of the four choices given below, choose the one that best describes the two statements.

1. Statement 1 is true, statement 2 is true; statement 2 is a correct explanation for statement 1.
2. Statement 1 is true, and statement 2 is true; statement 1 is not a correct explanation for statement 1.
3. Statement 1 is true, statement 2 is false.
4. Statement 1 is false, and statement 2 is true.

Question 1.

Statement 1: The centre of mass of the system will not alter in any direction if the external force is not exerted on it.

Statement 2: If net external force is zero then the linear momentum of the system remains constant.

Answer: 4. Statement 1 is false, statement 2 is true.

Question 2.

Statement 1: The position of the centre of mass of a body is independent of the shape and size of the body.

Statement 2: The centre of mass of a body may be in a position where there is no mass.

Answer: 4. Statement 1 is false, statement 2 is true.

Question 3.

Statement 1: Centre of mass of a rigid body always lies inside the body.

Statement 2: Centre of mass and centre of gravity coincide if gravity is uniform.

Answer: 4. Statement 1 is false, statement 2 is true.

Question 4.

Statement 1: The centre of mass of an electron-proton system, when released, moves faster towards the proton.

Statement 2: Proton is heavier than an electron.

Answer: 4. Statement 1 is false, statement 2 is true.

Question 5.

Statement 1: When a body dropped from a height explodes in mid-air, its centre of mass keeps moving in a vertically downward direction.

Statement 2: Explosion occurs under internal forces only External force is zero.

Answer: 3. Statement 1 is true, statement 2 is false.

Statics Match The Columns

Question 1.

Answer: 1. A, 2. C, 3. D, 4. B

Question 2. The two-block systems shown, match the following.

Answer: 1. C, D, 2. C, D, 3. B, 4. B

Question 3.  If the net force on a system of particles is zero, then match the following columns

Answer: 1. B, 2. C, 3. C, 4. D

Question 4. The arrangement is shown to match the following.

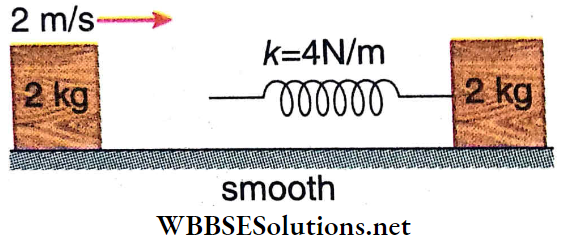
Answer: 1. B, 2. B, 3. B, 4. A

Statics Comprehension Type Questions And Answers

Read the following passages carefully and answer the questions at the end of them.

Question 1. A solid cylinder rolls up an inclined plane with an angle of inclination of 30°. At the bottom of the inclined plane, the centre of mass of the cylinder has a speed of 5 m · s^-1.

1. The distance up to which the cylinder goes up the plane is

1. 4m
2. 3.8m
3. 3.6m
4. 3m

Answer: 2. 3.8m

2. The time taken for the cylinder to return to the bottom is

1. 3.5 s
2. 3.7 s
3. 3.0 s
4. 3.8 s

Answer: 3. 3.0 s

Question 2. Four particles of masses 1 kg, 2 kg, 3 kg and 4 kg are placed at the four vertices A, B, C and D of a square of side 1 m.

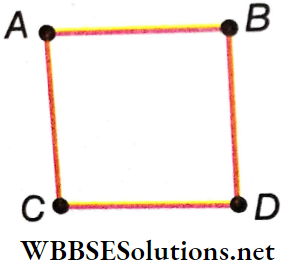
1. Taking D as origin, x -coordinates of centre of mass is

1. 0.7 m
2. 0.8 m
3. 0.5 m
4. 0.6 m

Answer: 3. 0.5 m

2. Taking D as origin, y -coordinates of the centre of mass is

1. 0.5 m
2. 0.6 m
3. 0.3 m
4. 0.4 m

Answer: 3. 0.3 m

3. Taking C as the origin, the position of the centre of mass of the particles is

1. (0.7 m, 0.6 m)
2. (-0.5 m, 0.3 m)
3. (0.6 m, 0.4 m)
4. (-0.5 m, 0.6 m)

Answer: 2. (-0.5 m, 0.3 m)

Question 3. Two balls having masses m₁ = 4 kg and m₂ = 5 kg have initial velocities of 5m • s^-1 in the directions shown. They collide at the origin. (Take all integer values)

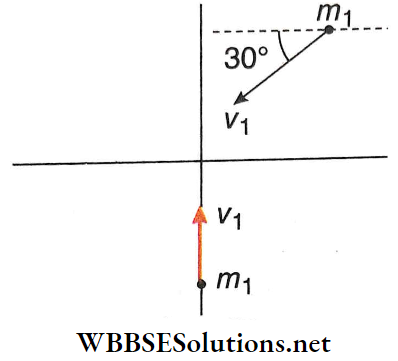
1. Velocity of the centre of mass 3 s before the collision is

1. \((-2 \hat{i}+1 \hat{j}) \mathrm{m} \cdot \mathrm{s}^{-1}\)
2. \((-2 \hat{i}-1 \hat{j}) \mathrm{m} \cdot \mathrm{s}^{-1}\)
3. \((2 \hat{i}+1 \hat{j}) \mathrm{m} \cdot \mathrm{s}^{-1}\)
4. \((2 \hat{i}+1 \hat{j}) \mathrm{m} \cdot \mathrm{s}^{-1}\)

Answer: 1. \((-2 \hat{i}+1 \hat{j}) \mathrm{m} \cdot \mathrm{s}^{-1}\)

2. The position of the centre of mass 2 s after the collision is

1. \(-4 \hat{i}-2 \hat{j}\)
2. \(4 \hat{i}-2 \hat{j}\)
3. \(-4 \hat{i}+2 \hat{j}\)
4. \(4 \hat{i}+2 \hat{j}\)

Answer: 3. \(-4 \hat{i}+2 \hat{j}\)

Question 4. A particle of mass 1 kg has velocity \(\overrightarrow{v_1}=(2 t) \hat{i}\) and another particle of mass 2 kg has velocity \(\overrightarrow{v_2}=\left(t^2\right) \hat{j}\)

1. Net force on the centre of mass at 2 s

1. \(\frac{20}{9}\) unit
2. \(\sqrt{68}\) unit
3. \(\frac{\sqrt{80}}{3}\) unit
4. None of these

Answer: 2. \(\sqrt{68}\) unit

2. Velocity of the centre of mass at 2 s

1. \(\frac{20}{9}\) unit
2. \(\sqrt{68}\) unit
3. \(\frac{\sqrt{80}}{3}\) unit
4. None of these

Answer: 3. \(\frac{\sqrt{80}}{3}\) unit

3. Displacement of the centre of mass in 2 s

1. \(\frac{20}{9}\) unit
2. \(\sqrt{68}\) unit
3. \(\frac{\sqrt{80}}{3}\) unit
4. None of these

Answer: 1. \(\frac{20}{9}\) unit

Statics Integer Answer Type Questions And Answers

In this type, the answer to each of the questions is a single-digit integer ranging from 0 to 9.

Question 1. Two ice skaters A and B approach each other at right angles. Skater A has a mass of 30 kg and a velocity 1 m · s^-1, and skater B has a mass 20 kg and a velocity 2m · s^-1. They meet and cling together. Find the final velocity (in m · s^-1) of the couple.
Answer: 1

Question 2. Both the blocks as shown in the given arrangement are given a horizontal velocity towards the right together. If a_cm is the subsequent acceleration of the centre of mass of the system of blocks then find the value of a_cm (in m · s^-2).
Answer: 2

Question 3. Blocks A and B shown have equal masses m. The system is released from rest with the spring unstretched. The string between A and the ground is cut when there is a maximum extension in the spring. Find the acceleration of the centre of mass (in terms of how many times of g) of the two blocks.
Answer: 2