WBCHSE Class 11 Physics Newtons Law Of Motion Notes

Newton Law Of Motion

Statements Of The Laws Of Motion

In 1687, Sir Isaac Newton, in his book Principia, published three laws of motion related to all the moving objects in the universe. These are known as Newton’s laws of motion. These laws cannot be proved using any old concept of physics. Yet, their validity has been confirmed through successful explanations and predictions on numerous events in nature. Hence, the laws founded a new branch of physics called kinetics.

The Laws Are:

1. 1st Law: Everybody continues to be in its state of rest or of uniform motion in a straight line unless the body is compelled to change its state by a net external force.
2. 2nd Law: The rate of change of momentum of a body is proportional to the impressed force and takes place in the direction in which the force acts.
3. 3rd Law: To every action, there is an equal and opposite reaction.

These laws involve the new concepts of

1. Inertia,
2. Force and
3. Momentum.

Read and Learn More: Class 11 Physics Notes

The study of these laws is the subject of this chapter and is called Newtonian mechanics. It should be mentioned, however, that Newtonian mechanics does not apply to all situations.

1. If the interacting bodies are moving with the speed of light (or an appreciable fraction of the speed of light), Newtonian mechanics should be replaced by Einstein’s special theory of relativity.
2. If the interacting bodies are of atomic dimensions (for example, electrons in an atom), Newtonian mechanics should be replaced by quantum mechanics.

In short, Newtonian mechanics may be treated as a special case of these two more comprehensive theories.

Weight Of A Body Gravitational Unit Of Force

Gravitational Unit Of Force Definition: The weight of a body is the force with which the earth attracts the body.

• The earth’s attraction is the force of gravity, the acceleration produced by it is called the acceleration due to gravity, g, and it is always directed towards the center of the earth.
• Hence, using the relation \(\vec{F}=m \vec{a}\), the weight of a body of mass m is formulated as \(\vec{W}=m \vec{g}\)
• So, weight = mass x acceleration due to gravity at the place. The value of g varies at different places and hence the weight also changes. For example, a bowling ball of mass 7.2 kg weighs 71N on Earth, but only 12N on the Moon.
• The mass of the ball is the same on both earth and the moon but the free fall acceleration on the moon is only 1.6 m/s².

Gravitational unit Of force: The force with which the earth pulls a body of unit mass, is the unit of gravitational force.

Gravitational units are no longer accepted for use with the SI units by BIPM.

From the above definitions, we see that gravitational units depend on acceleration due to gravity which varies at different places. So we cannot use these units as standard.

But accepting a value of g as a standard, a standard gravitational unit of force can be defined. Kilogram-force or kgf is the most commonly used standard unit.

1 kgf is the weight of a mass of 1 kg at a place where the acceleration due to gravity, g = 9.80665 m · s^-2, and

therefore 1 kgf = 1 kg x 9.80665 m · s^-2 = 9.80665 N .

However, this unit has become almost obsolete at present.

Inertial Mass: Suppose, two small pieces, one of wood and the other of iron, are at rest on the floor. From experience, we know that, on applying the same amount of force, the piece of wood will have an acceleration greater than that of the iron piece.

• Conversely, to produce the same acceleration on both pieces, the force applied on the iron piece will have to be greater. So, the inertia of rest for the iron piece is more than that for the piece of wood. Thus, the mass of a body is a measure of inertia of rest.
• This is also true for the inertia of motion. For instance, to stop a bicycle and a truck, moving with the same velocity within the same distance, i.e., to produce the same retardation, the force applied on the truck has to be greater. So, in this case, too, the masses of the vehicles give a measure of their inertia of motion.

Therefore, to produce the same acceleration in any two different objects, at rest or in motion, force required will be different if their masses are unequal. Thus, the mass of an object is the measure of its inertia. So mass is also called Inertial mass. The mass m in the equation \(\vec{F}=m \vec{a}\), is the inertial mass.

Newton Law Of Motion Weigt Of Body Numerical Examples

Example 1. A paratrooper of mass 75 kg falls with a constant velocity. Find the air resistance acting on him.
Solution:

As the acceleration is zero, there is no resultant force acting on the paratrooper. The weight acting downwards = 75 x 9.8 = 735 N.

Therefore, the air resistance, acting upwards, is R = 735 N.

 Newton Law Of Motion – Analysis Of Different Motions

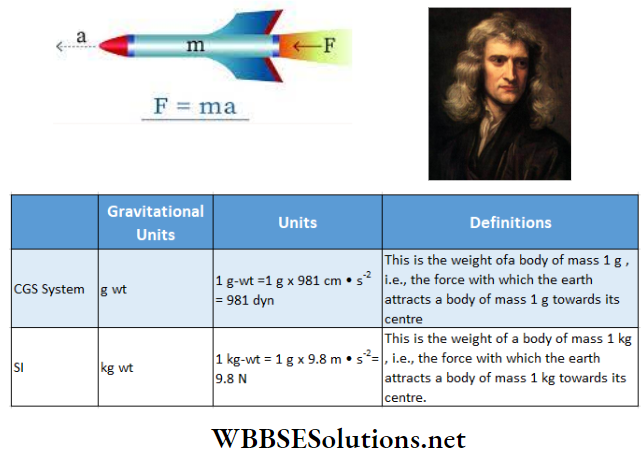
Walking On A Horizontal Plane: AB is a horizontal plane. A man wants to walk on the plane towards B. He applies an oblique force F on the plane. The plane too, exerts a reaction force R on the man.

• The vertical component of R acts opposite to the weight of the man and the horizontal component H provides the force for the forward movement. So, the motion of the man is not directly due to the force F that he exerts, but due to the opposite reaction.
• In fact, this force decides the type of the motion. As the frictional force is low on a smooth surface, it is not possible to exert a large force. If the horizontal component of F becomes much larger than the frictional force, the man may slip.
• Hence one cannot walk fast on a very smooth surface as there is a high chance of slipping.

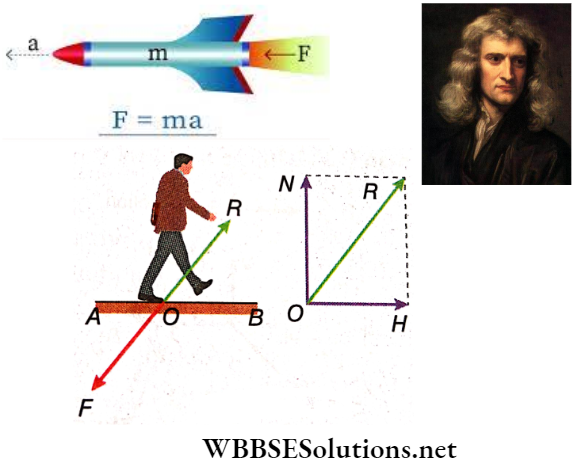
Flight Of Birds: If a bird intends to fly along OC, it flaps its wings along OA and OB, thus exerting some force on the air. At the same time, the reaction forces on the bird are OE and OD.

• If both the wings of the bird exert equal force on air, the resultant reaction force will be along OC. The bird moves forward due to this reaction force.
• By adjusting the force applied by the wings, the resultant may be shifted to the left or right of the direction of OC, and the bird can thus change its course.
• In the absence of air, i.e., in a vacuum, the reaction forces OD and OE cannot be generated, and so birds cannot fly.

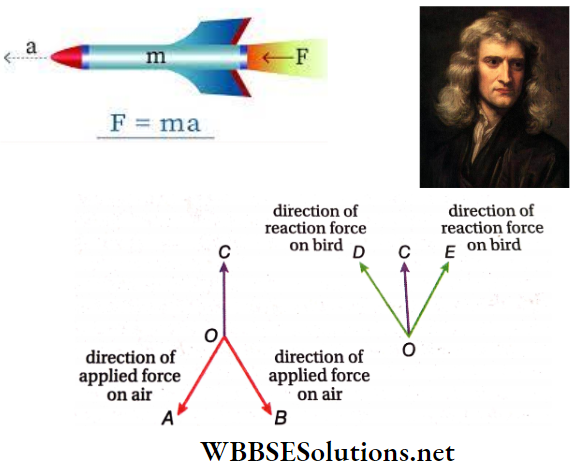
Motion Of A Hand-Pulled Rickshaw: A man is trying to pull a rickshaw on a horizontal plane, towards point B. He exerts an oblique force P on the plane. The reaction R of the plane has a vertical component N and a horizontal component F. Force N is balanced by the weight of the man. Horizontal component F sets up the motion of the man.

• Let the force exerted by the man on the handles of the rickshaw be T. The rickshaw also pulls the man backward with the same force T.
• Due to the motion of the wheel on the plane, frictional force F’ acts at the point of contact of the wheel and the plane in a direction opposing the motion.
• Hence, the resultant of forces acting on the man = F- T in the forward direction and that on the rickshaw in the same direction = T-F’
• Let the masses of the man and the rickshaw be m₁ and m₂ respectively and common acceleration in the forward direction be a.

For the man, F- T = m₁a or, T = F-m₁a

For the rickshaw, \(T-F^{\prime}=m_2 a \text { or, } T=F^{\prime}+m_2 a\)

∴ \(F-m_1 a=F^{\prime}+m_2 a \text { or, }\left(m_1+m_2\right) a=F-F^{\prime}\)

or, \(a=\frac{F-F^{\prime}}{\left(m_1+m_2\right)}\)

The expression for the acceleration shows that,

1. Individual masses m₁ or m₂ are not significant but the sum of the masses (m₁ + m₂) matters,
2. The rickshaw will start moving, i.e., Accelerating when F> T>F’,
3. During the early stages of motion, F > T > F’. It attains a uniform speed when F =T= F’ and then of course a = 0, and
4. If F< F’, the rickshaw cannot be set into motion at all.

There are many similar examples in nature, where a force (action) is applied backward, and the reaction force generated is actually responsible for the intended forward motion.

Newton Law Of Motion – Equation Of Motion And Its Solution

The body, whose motion is to be analyzed, should be identified at first.

1. This ‘‘body” may consist of a single body a combination of bodies (often called a system of bodies’) or even a part of a single body.
2. Then we have to consider the surroundings (or environment). Every other body having a direct influence on our chosen body is a part of the surroundings. In general, action-reaction-type forces act between the body and its surroundings.
3. Now we search for every force acting on our body. Each of the forces is either a force of action on the body or a force of reaction on it due to some other body in the surroundings.
4. Once the forces are identified, we regard them on the same footing, i.e., we no longer differentiate between actions and reactions. In this sense, the body under consideration acts as a free body, subject to a system of forces acting on it.
5. If the active forces are \(\vec{F}_1, \vec{F}_2, \vec{F}_3, \cdots\), then the resultant or net force is \(\vec{F}=\vec{F}_1+\vec{F}_2+\vec{F}_3+\cdots\)

For a body of constant mass m, we get from Newton’s 2nd law, m \(\vec{a}=\vec{F}=\vec{F}_1+\vec{F}_2+\vec{F}_3+\cdots\)…(1)

This equation (1) is to be solved to find out the acceleration \(\vec{a}\) of the body.

As \(\vec{a}\) is related to the velocity \(\vec{v}\) as \(\vec{a}\) = \(\frac{d \vec{v}}{d t}\), equation (1) may be written as \(m \frac{d \vec{v}}{d t}=\vec{F}\)…(2)

Clearly, this is the differential equation to be solved if the velocity of the body is to be found. Similarly, as \(\vec{v}=\frac{d \vec{r}}{d t}\)(\(\vec{r}\) = position vector of the body), we may write m \(\frac{d^2 \vec{r}}{d t^2}=\vec{F}\)…(3)

This 2nd-order differential equation is to be solved to obtain \(\vec{r}\) at any instant.

Any of the equations (1) to (3) is then called the equation of motion of a body.

It is to be noted that the equation of motion is a vector equation. Each vector has three independent components. So the solution of an equation of motion involves, in general, a solution of three independent equations corresponding to three mutually orthogonal coordinate axes.

However, this is not always the case we come across plenty of examples of one or two-dimensional motions where one or two equations, respectively, are relevant.

Newton Law Of Motion Problems On Tension

Horizontal Motion With The Help Of A Smooth Pulley: A body A of mass m is resting on a frictionless horizontal plane. To set body A into motion along the plane, it is tied, using a massless, inextensible string passing over a massless, frictionless pulley, with another m body B of mass M as shown.

Both the bodies, A and B, get the same constant acceleration and the tension in the string remains constant (as the pulley is frictionless).

Let the tension in the string = T; acceleration produced = a

∴ For the motion of A, T = ma …(1)

For the downward motion of B, Mg- T = Ma ….(2)

From (1) and (2), (M+ m)a = Mg

or, a = \(\frac{M}{M+m^g} g\)…(3)

As, \(\frac{M}{M+m}<1, a<g\), though the surface and the pulley are frictionless.

Also, inserting the value of an in equation (1) we get, T = \(\frac{M m}{M+m^2} g=\frac{M}{1+\frac{M}{m}^g}\)

Here, \(\frac{M}{1+\frac{M}{m}}<M\), and hence T < mg, i.e., the tension in the string is less than the weight of the body B.

Vertical Motion With The Help Of A Smooth Pulley; To raise a body A of mass m vertically, a heavy body B of mass M is connected to A using a massless inextensible string passing over a massless smooth frictionless pulley as shown.

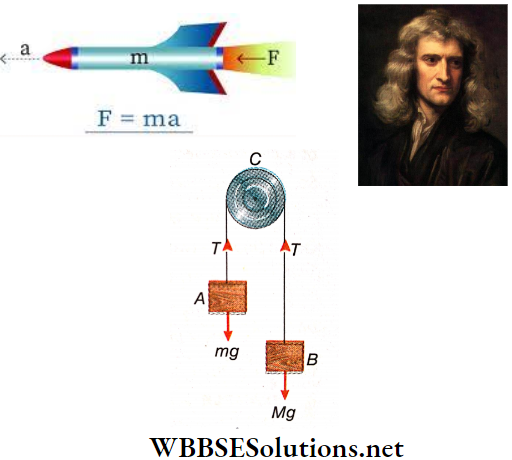
Let the tension developed in the string be T and the common acceleration of A and B be a. Mass of B is more than that of A. Hence B will move downwards, A will move upwards.

Considering the motion of B, Mg – T = Ma …(1)

and the motion of A, T – mg = ma …(2)

Adding equations (1) and (2), (M- m)g = (M+ m)a

or, a = \(\frac{M-m}{M+m} g\)…(3)

From (3), a < g though the pulley is smooth.

Again, inserting the value of an in (1),

T = \(M g-\frac{M(M-m)}{M+m} g=M g\left(1-\frac{M-m}{M+m}\right)\)

= \(\frac{2 M m}{M+m^2} g\)…(4)

The value of a in equation (3) is positive (i.e., acceleration of A is upwards), if M> m, i.e., if the mass of B is more than that of A. As M > m, we get from equation (4), T> mg and T< Mg, i.e., the tension in the string is more than the weight of A (mg), but less than the weight of B. The net force on the pulley,

2T = \(\frac{4 M m}{M+m} g\)…(5)

 Newton Law Of Motion Problems On Tension Numerical Examples

Example 1. A block of mass m₂ = 100 g is suspended from one end of an inextensible string. The other end of the string is tied to another block of mass m₁ = 200 g, kept on a frictionless table surface. The string passes over a pulley. Find the tension in the string and the acceleration of the blocks.

Solution:

Let the tension in the string = T, and the acceleration of each block be a.

So, the equations of motion of the two blocks are, T = m₁a and m₂g – T = m₂a

On adding the two equations, we get, m₂g = m₁a + m₂a

or, \(a=\frac{m_2}{m_1+m_2} \cdot g=\frac{100}{200+100} \times 980=326.7 \mathrm{~cm} \cdot \mathrm{s}^{-2}\)

and T = m₁a  = 200 x 326.7 = 65340 dyn.

Example 2. Two blocks of masses 2.9 kg and 1.9 kg are suspended from a rigid support, using two inextensible wires, each of length 1 m, as shown. The mass of the upper string is negligible and that of the lower string is 0.2 kg • m^-1. If the whole system is moving up with an acceleration of 0.2 ms^-2, find

1. The tension at the midpoint of the lower string and
2. The tension at the midpoint of the upper string.Acceleration due to gravity = 9.8 m · s^-2.

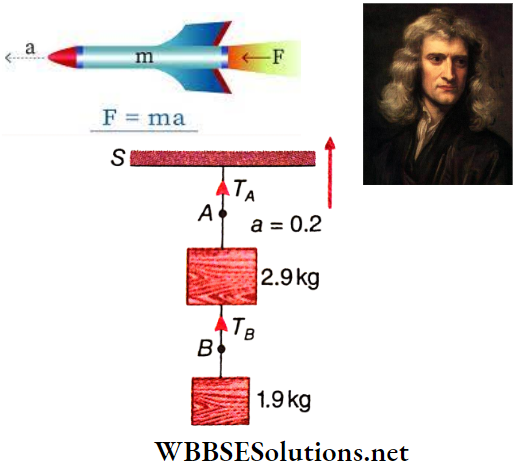
Solution:

1. Mass of the lower string =0.2 x 1 = 0.2 kg. Its midpoint is B, and so the total mass hanging from B, \( m_B=\left (1.9+\frac{0.2}{2}\right)=2 \mathrm{~kg} \text {. }\)

Hence, downward force at B = m_Bg

Upward force at B = T_B

So applying Newton’s 2nd law of motion for the mass below B, m_Ba = T_B– m_Bg

or, T_B = m_Ba + m_Bg = m_B(a + g)

= 2(0.2 + 9.8) = 20 N

2. Midpoint of the upper string is A; total mass hanging below A, m_A = 2.9 + 0.2 + 1.9 =5.0 kg.

Hence, downward force at A = m_Ag

Upward force at A = T_A

Hence applying Newton’s 2nd law of motion for the whole system, m_Aa = T_A– m_Ag

or, T_A = m_A(g+ a) = 5(9.8 + 0.2) = 50 N

Example 3. Two bodies of mass 7 kg and 5 kg 200 N are joined with a rope of mass 4 kg. An upward force of 200 N is applied on the upper body. Find

1. The acceleration of the system,
2. Tension at the top end of the rope and
3. Tension at the midpoint of the rope.

Solution:

Let the acceleration be a.

1. From Newton’s 2nd law, 16a = 200- 16g or, 16a = 200- 16 x 9.8

∴ a = 2.7 m · s^-2.

2. The upper end of the rope carries the masses of the second body and the rope. If the tension is T, then T- (4 + 5)g = (4 + 5)a

or, T =9(g+ a) =9(9.8 + 2.7) =112.5 N.

3. The mass carried by the midpoint of the rope is half the mass of the rope and the whole mass of the lower body. Hence, if the tension is T’ at the midpoint, the equation of motion is T’-(2 + 5)g= (2 + 5)a

or, T’ = 7(g+ a) = 7(9.8 + 2.7) = 87.5 N.

Example 4. A double inclined— plane A is placed on a horizontal table and two blocks of masses m₁ and m₂ are placed on the two frictionless inclined planes of A. Two ends of a rope wound over a pulley are tied to the two blocks. Find out the horizontal acceleration to be imparted to the system so that the blocks are at rest relative to A. In this condition, what will be the tension in the rope?

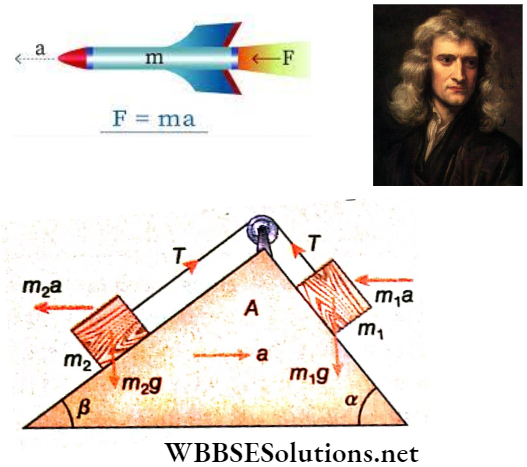
Solution:

Let the tension in the rope be T and the horizontal acceleration be a .

The forces acting on the two blocks have been shown. They are at rest relative to A, if

∴ \(T-m_2 g \sin \beta-m_2 a \cos \beta=0\)…(1)

∴ \(T-m_1 g \sin \alpha+m_1 a \cos \alpha=0\)…(2)

Subtracting (2) from (1), we get, \(g\left(m_1 \sin \alpha-m_2 \sin \beta\right)=a\left(m_1 \cos \alpha+m_2 \cos \beta\right)\)

or, \(a=\frac{m_1 \sin \alpha-m_2 \sin \beta}{m_1 \cos \alpha+m_2 \cos \beta} \times g\)

∴ T = \(m_2 a \cos \beta+m_2 g \sin \beta\)

= \(m_2 g\left[\frac{m_1 \sin \alpha-m_2 \sin \beta}{m_1 \cos \alpha+m_2 \cos \beta} \cdot \cos \beta+\sin \beta\right]\)

= \(\frac{m_1 m_2 \sin (\alpha+\beta)}{m_1 \cos \alpha+m_2 \cos \beta} g .\)

Newton Law Of Motion Reaction In A Moving Lift Apparent Weight

When a person of mass m stands on a floor, a downward force mg acts on the floor due to his weight. At the same time, the floor exerts an upward reaction force or normal force R on the man.

• As R acts on the person, the person feels his weight. From the third law of motion, R = mg.
• So, in this case, the actual weight mg, and the normal reaction, R, is the same. If the normal force R is absent, the person feels weightless.
• For example, while jumping from a height, one feels weightless before touching the earth’s surface, because the normal force R is absent.
• The normal force R varies inside a lift due to its vertical motion. The floor of the lift, in this case, provides the reaction. A person feels lighter or heavier than his actual weight depending on the reaction force R. This is called apparent weight.

Let the mass of a man in a lift be m.

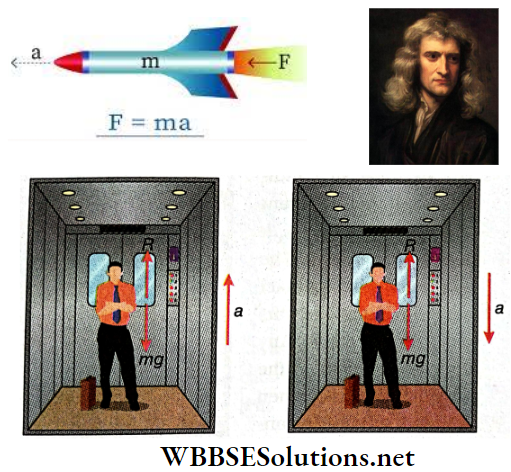
The downward attractive force acting on him = real weight = mg

The upward normal force of the floor – apparent weight = R

∴ The net upward force F = R- mg.

We can determine the apparent weight R using F = ma for different types of motion of the lift.

1. The Lift Is Moving Up With An Acceleration a(Or, Moving Down With A Retardation a): Here the upward acceleration is a.

∴ R- mg = ma

or, R = mg+ ma = m(g+ a)….(1)

∴  As R> mg, the man feels heavier.

2. The Lift Is Moving Down With An Acceleration a(Or, Moving Up With A Retardation a): Here the downward acceleration = a or upward acceleration =-a (here a < g).

∴ R- mg = -ma

or, R = m(g- a)…(2)

He feels lighter as m(g- a) < mg.

3. The Cable Of The Lift Snaps And The Lift With The Man, Falls Freely Due To Gravitational Pull: Here, the acceleration, a = g. Hence from (2),

R = m(g- g) = 0…(3)

As no normal force acts on the man, he feels weightless.

This apparent weightlessness is true for all freely falling bodies.

4. The Lift Is At Rest Or In Uniform Motion: The value of a is zero and so the reaction force, R = mg. Hence, the apparent weight is the same as the real or true weight.

5. The Lift Falls With A Downward Acceleration Greater Than g: Here, a > g. From equation (2), R = m(g-a) or, R = -m(a- g).

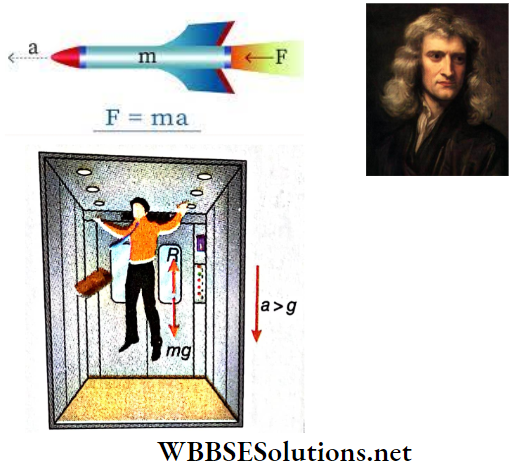
The negative sign indicates that the apparent weight is negative, i.e., it is directed upwards. The man thus loses contact with the floor and hits the ceiling of the lift. Any item on the floor of the lift will hit the roof when exposed to similar conditions. This is termed as super-weightlessness.

Newton Law Of Motion – Reaction In A Moving Lift Apparent Weight Numerical Examples

Example 1. A body of mass 1 kg is suspended from a spring balance calibrated for acceleration due to gravity of 10 m · s^-2. What is the reading on the spring balance when the system

1. Is ascending with an acceleration of 5 m · s^-2 and
2. Is descending with the same acceleration? [g = 10 m · s^-2]

Solution:

1. Reaction force on the balance when it ascends, R = m(g+ a) = 1(10 + 5) = 15 N.

∴ In this case, the reading of the spring balance = 15/100 = 1.5 kg

Reaction force when the system descends, R = m(g – a) =1(10-5) = 5N.

∴ In this case, the reading of the spring balance = 5/10 = 0.5 kg

Example 2. A man of 50 kg is standing on a weighing machine in a lift. As the lift moves with a constant acceleration, the weighing machine registers the man’s weight as 45 kg. State whether the lift is ascending or descending. Give reasons for your answer. What is the acceleration of the lift? [g = 9.8 m · s^-2]
Solution:

The weighing machine shows a reading lower than the real weight of the man. So, the lift is descending with an acceleration, because in such cases, apparent weight R = m(g- a) < mg.

The downward acceleration, a = \(g-\frac{R}{m}=9.8-\frac{45 \times 9.8}{50}=0.98 \mathrm{~m} \cdot \mathrm{s}^{-2}\)

Example 3. A man of mass 70 kg is sitting in a motor car. The car is moving with an acceleration of 5 m · s^-2. What is the gravitational force on the man? [g = 9.8 m s^-2]

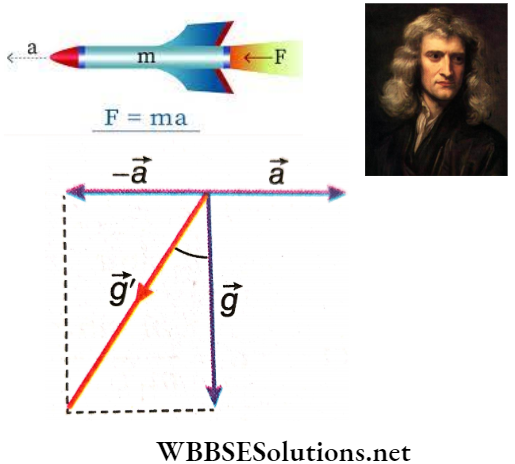
Solution:

Let the horizontal acceleration of the car be \(\vec{a}\).

The apparent acceleration due to gravity with respect to the car, \(\vec{g}^{\prime}=\vec{g}-\vec{a}=[\vec{g}+(-\vec{a})]\)

which is the resultant of the vectors \(\vec{g}\) and –\(\vec{a}\).

From, \(g^{\prime}=\sqrt{g^2+a^2}=\sqrt{(9.8)^2+5^2}\)

= \(11 \mathrm{~m} \cdot \mathrm{s}^{-2}\)

Force of gravitation on the man =70 x 11 = 770 N,

which acts in the direction of g’.

[The apparent weight of the man is, W’ = \(\frac{770}{9.8}\) = 78.6 kg]

The force of gravity, acting on the man, remains constant but, because of acceleration, the man feels a greater pull of gravity.

Example 4. A man of mass 60 kg is standing in a lift at rest. What will be the reaction force on the man when the lift is

1. Stationary,
2. Moving up with an acceleration of 4.9 m · s^-2,
3. Moving up at a constant speed, and
4. Moving up with a retardation of 4.9 m · s^-2? [G = 9.8 m · s^-2]

Solution:

1. The reaction of the lift floor when the lift is stationary R = mg = 60 x 9.8 N = 588 N.

2. The lift is moving up with an acceleration, of a = 4.9 m · s^-2.

Reaction, R=m(g+a) =60(9.8 + 4.9) N =882N,

3. When the lift is moving up at a constant speed, a = 0.

∴ R = mg =60 x 9.8 N = 588 N.

4. When the  lift moves up with a retardation of 4.9 m · s^-2,

R = m[g+ (-a)] = m(g- a) = 60(9.8- 4.9) N = 294 N

Example 5. A man of mass 98 kg, is standing on a weighing machine in a lift. What will be the readings of the weighing machine in the following cases:

1. The lift ascends at 100 cm per second,
2. The lift descends with an acceleration of 30 cm · s^-2. [g= 980 cm · s^-2]

Solution:

1. When the lift ascends with uniform velocity (acceleration a = 0 ) then, R = mg = 98 x 9.8 N.

Reading of the weighing machine = \(\frac{98 \times 9.8}{9.8} \mathrm{~kg}=98 \mathrm{~kg} .\)

2. When the lift descends, R = m(g- a)

= 98(9.8-0.3) [30 cm · s^-2 = 0.3 m · s^-2]

= 98 x 9.5 N

∴ Reading of the weighing machine = \(\frac{98 \times 9.5}{9.8} \mathrm{~kg}=95 \mathrm{~kg} .\)

Example 6. A man weighing 60 kg is in a lift that descends with an acceleration of 4 cm · s^-2. What force will the man exert on the floor of the lift? If the lift begins to ascend with the same acceleration, what reaction force will act on the man? For what acceleration of the lift, while descending, will the man experience weightlessness? [g = 980 cm · s^-2]
Solution:

If the lift descends with an acceleration a, and if the reaction force exerted by the lift on the man in the upward direction is R, then mg- R = ma …(1)

The force exerted by the man on the lift’s floor, R = mg – ma [4cm · s^-2 = 0.04m · s^-2] = 60(9.8-0.04) = 585.6 N

If the lift ascends with the same acceleration, then the reaction force on the man is, R’ = mg+ ma [4 cm · s^-2 = 0.04 m · s^-2]

= 60(9.8 + 0.04) = 590.4 N

When the acceleration of the lift, while descending, is a = g, then from equation (1), we get, R = mg- mg = 0 . For this zero reaction force, the man feels weightless.

So, for a lift falling freely with an acceleration g, the man inside it will feel no weight.

Example 7. A lift of mass 200 kg is moving up with an acceleration of 4 m · s^-2. What is the tension in the lift cable? If the lift moves down with the same acceleration, what will be the tension in that case? [g = 9.8 m · s^-2]
Solution:

Let the tension in the cable be T when the lift is moving with an acceleration a.

The equation of motion for the lift is, T- mg = ma

or, T = m(g+a)…(1)

Substituting the values for m, g, and a in equation (1), for upward motion,

T =200(9.8 + 4) = 200×13.8 = 2760 N

For the lift moving downwards, a = -4m · s^-2,

T= 200(9.8-4) = 200×5.8 = 1160 N

Example 8. A lift of mass 2000 kg is supported by thick steel ropes. If the maximum upward acceleration of the lift be 1.2 m/s², and the breaking stress for the ropes be 2.8 x 10⁸ N/m², what should be the minimum diameter of the rope?
Solution:

Here, m = 2000 kg, a = 1.2 m/s²

Breaking stress =2.8 x 10⁸ N/m²

Let the diameter of the rope be D.

When the lift moves upwards, the tension in the rope is T= m(g+ a) = 2000(9.8 + 1.2) = 22000 N.

Now, breaking stress = \(\frac{\text { force }}{\text { area }}=\frac{T}{\pi D^2 / 4}=\frac{4 T}{\pi D^2}\)

or, 2.8 x \(10^8=\frac{4 \times 22000 \times 7}{22 \times D^2}\)

or, \(D^2=\frac{4 \times 22000 \times 7}{22 \times 2.8 \times 10^8}=10^{-4} \quad \text { or, } D=1 \mathrm{~cm}\)

Newton Law Of Motion – Law Of Conservation Of Linear Momentum

Newton’s second law of motion states that the rate of change of momentum of a body is directly proportional to the applied force. Hence, in the absence of an external force, there is no change in the momentum of a body.

This law is also applicable for a system consisting of a number of bodies. The members in a many-body system may have interactions among themselves due to collisions, attractions, repulsions, etc. These forces are to be treated as internal forces, not external forces.

Law Of Conservation Of Linear Momentum Statement: In the absence of any external force acting on a system of bodies, even if interactions exist among the bodies, the total linear momentum of the system remains constant.

• This statement is the law of conservation of linear momentum. For a system of bodies, we can calculate the components of linear momentum, of all the bodies present, in any chosen direction. The stun of these individual components in this direction will be a constant.
• We shall illustrate the law for a one-dimensional collision. Suppose two particles of mass m₁ and m₂, moving with velocities u₁ and u₂ respectively in a straight line, collide with each other. After the collision, the particles move with velocities v₁ and v₂ respectively in the same direction.

Hence, total momentum before collision =m₁u₁ + m₂u₂, and total momentum after collision =m₁v₁ + m₂v₂.

If there is no external force, as per the conservation law of linear momentum, m₁u₁ + m₂u₂ = m₁v₁ + m₂v₂…….(1)

Law Of Conservation Of Linear Momentum From Newton’s Third Law Of Motion: In the absence of any external force, two bodies, during a collision, exert an impulsive force on each other. From Newton’s third law of motion, impulsive force on the first body is equal and opposite to that exerted on the second body.

Forces F₁₂ and F₂₁ are shown: F₂₁ = -F₁₂

If the collision lasts for a time t, the impulse of F₂₁ on the first body = F₂₁ • t = change of momentum of the first body = m₁u₁– m₂u₂

Similarly, impulse of F₁₂ on the second body = F₁₂ x t = change of momentum of the second body = \(m_2 v_2-m_2 u_2\)

As \(F_{21}=-F_{12}, \quad m_1 v_1-m_1 u_1=-\left(m_2 v_2-m_2 u_2\right)\)

or, \(m_1 u_1+m_2 u_2=m_1 v_1+m_2 v_2\)

As total momentum before collision = total momentum after collision, the total linear momentum remains conserved.

Alternative Method: Suppose two bodies of masses m₁ and m₂, moving along the same straight line with velocities v₁ and v₂ respectively, collide with each other.

Since there is no external force present, let the force on the first body exerted by the second body be F₂₁, and that on the second body exerted by the first body be F₁₂.

∴ \(a_1=\frac{d v_1}{d t} \text { and } a_2=\frac{d v_2}{d t}\) = are the respective accelerations of the bodies.

From Newton’s third law of motion, \(F_{21}=-F_{12}\)

or, \(m_1 \frac{d v_1}{d t}=-m_2 \frac{d v_2}{d t}\)

or, \(\frac{d}{d t}\left(m_1 v_1+m_2 v_2\right)=0 or, \quad m_1 v_1+m_2 v_2\)= constant

Hence, the total linear momentum remains conserved.

Newton’s Third Law Of Motion From The Law Of Conservation Of Linear Momentum: Let the initial momentum of two bodies be p₁ and p₂. They come in contact for a time t and their momenta change to p₁‘ and p₂‘ respectively.

In the absence of any external force, as per law of conservation of linear momentum, \(p_1+p_2=p_1^{\prime}+p_2^{\prime}\)

or, \(p_1^{\prime}-p_1=-p_2^{\prime}+p_2 \quad \text { or, } \frac{p_1^{\prime}-p_1}{t}=-\frac{p_2^{\prime}-p_2}{t}\)

The left-hand side of the equation is the rate of change of the momentum of the first body = force on the first body = F₂₁; similarly, the right-hand side is -F₁₂.

Hence F₂₁ = -F₂₁ or, action = – reaction.

So action and reaction between two bodies are equal and opposite. This is nothing but Newton’s third law of motion.

Practical Applications Of The Principle Of Conservation Of Linear Momentum:

1. Recoil Of A Gun: When a bullet is fired from a gun, the gun recoils or gives a kick in the backward direction. It can be explained as follows:

• let m₁ be the mass of the bullet and m₂ be the mass of the gun. Initially, both the gun and the bullet are at rest. On firing the gun, let the bullet move with a velocity \(\vec{v}_1\), and the gun move with a velocity \(\vec{v}_2\).
• According to the principle of conservation of linear momentum, the total momentum of gun and bullet before firing = total momentum of gun and bullet after firing.

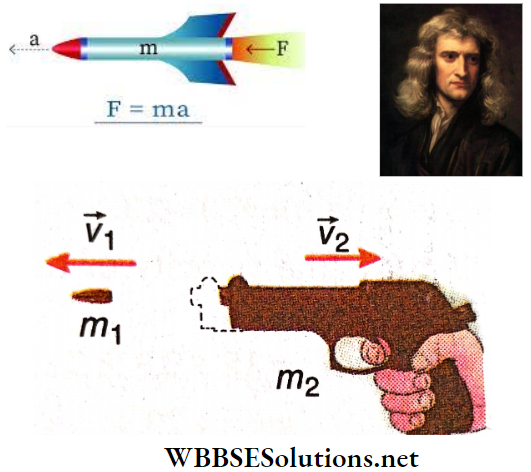
i.e., 0 = \(m_1 \vec{\nu}_1+m_2 \vec{\nu}_2 \quad \text { or, } \vec{\nu}_2=-\frac{m_1}{m_2} \vec{v}_1\)

• The negative sign shows that \(\vec{v}_2\) and \(\vec{v}_2\) are in opposite directions, i.e., as the bullet moves forward, the gun will move in backward direction. This backward motion of the gun is called the recoil of the gun.
• Hence, while firing a bullet, the gun must be held tight to the shoulder otherwise, because of the recoil velocity of the gun, the shoulder of the man who fires the gun, may get hurt.
• If the gun is held tight to the shoulder then the gun and the body of the man recoil as a single system. As the mass is quite large, the recoil velocity will be very small and the shoulder of the man will not get hurt.

2. Explosion Of A Bomb: Let a bomb of mass M be initially at rest. So, the initial momentum = 0.

After the explosion, suppose that the bomb is split into a few fragments of masses m₁, m₂, m₃ ……; the fragments fly away from the center of the explosion with velocities \(\vec{v}_1\), \(\vec{v}_2\), \(\vec{v}_3\)…

Thus, the final momentum is,\(\vec{p}=\vec{p}_1+\vec{p}_2+\vec{p}_3+\cdots=m_1 \vec{v}_1+m_2 \vec{v}_2+m_3 \vec{v}_3+\cdots\)

As no external force acts on the bomb, the momentum must be conserved, i.e., final momentum = initial momentum, or, \(\vec{p}=\vec{p}_1+\vec{p}_2+\vec{p}_3\)+….. = 0

As a special case, if the bomb explodes into two fragments only, then \(\vec{p}_1+\vec{p}_2=0 \text {, or } m_1 \vec{v}_1+m_2 \vec{v}_2=0 \text {, or } m_1 \vec{v}_1=-m_2 \vec{v}_2\)

Again, if the two fragments are of equal mass, \(m_1=m_2 \text { then } \vec{v}_1=-\vec{v}_2\)

Hence, the two fragments would acquire equal and opposite velocities due to the explosion.

Newton Law Of Motion – Law Of Conservation Of Linear Momentum Numerical Examples

Example 1. A bullet of mass 6 g is fired with a velocity of 500 m s-1 from a gun of mass 4 kg. Find the recoil velocity of the gun.
Solution:

A bullet of mass 6 g is fired with a velocity of 500 m s-1 from a gun of mass 4 kg.

As the bullet and the gun were at rest before the firing, the initial momentum of the system was zero. Let the speed of the bullet after the firing be v, and that of the gun be V.

From the law of conservation of linear momentum, 0 = MV+ mv

[mass of the bullet = m , mass of the gun = M]

or, \(V=-\frac{m}{M} \nu=-\frac{6 \times 10^{-3}}{4} \times 500=-0.75 \mathrm{~m} \cdot \mathrm{s}^{-1}\)

Hence, the recoil velocity of the gun = 0.75 m · s^-1.

Example 2. While firing a bullet of mass 8 g, the recoil velocity of the gun of mass 5 kg becomes 64 cm · s^-1. The bullet penetrates 50 cm through a target and then stops. Express the average resistance on the bullet in Newton.
Solution:

While firing a bullet of mass 8 g, the recoil velocity of the gun of mass 5 kg becomes 64 cm · s^-1. The bullet penetrates 50 cm through a target and then stops.

The initial momenta of the gun and the bullet were zero, as both were at rest. Let the masses of the gun and the bullet be M and m respectively and the respective velocities after firing be V and v.

From the law of conservation of Linear momentum, 0 = MV+ mu

∴ \(\nu=-\frac{M}{m} V=-\frac{5}{8 \times 10^{-3}} \times 0.64=-400 \mathrm{~m} \cdot \mathrm{s}^{-1}\)

The bullet comes to rest after penetrating a distance of 50 cm or 0.5 m. If the retardation is due to the resistance of the material of the target, 0 = (400)² – 2a · 0.5 or, a = 16 x 10⁴ m · s^-2

Hence, average resistance, F = ma = 0.008 x 16 x 10⁴ = 1280 N.

Example 3. A body of mass m moving with velocity V along the X-axis collides with another mass M moving with velocity v along the Y-axis. The masses coalesce after the collision. Find the velocity and the direction of motion of the combined mass.
Solution:

A body of mass m moving with velocity V along the X-axis collides with another mass M moving with velocity v along the Y-axis. The masses coalesce after the collision.

Let the velocity of the combined mass be u which makes an angle θ with the X- axis.

Applying the law of conservation of linear momentum along the X-axis, mV+ 0 = (m + M)u cosθ…(1)

Similarly, along Y-axis, 0 + Mv = (m + M)u sinθ…(2)

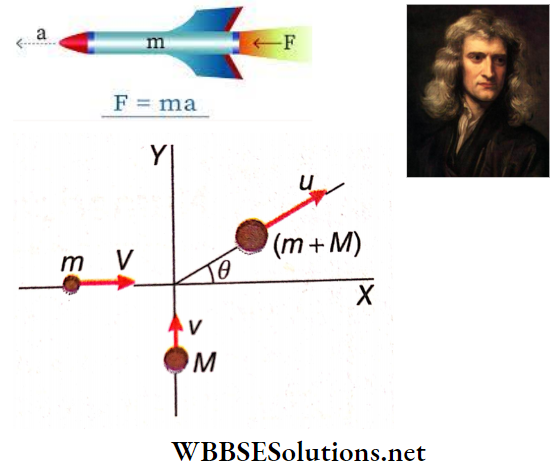
Squaring and adding (1) and (2), \(m^2 V^2+M^2 v^2=(m+M)^2 u^2\)

or, \(u^2=\frac{m^2 V^2+M^2 v^2}{(m+M)^2}\)

Hence, u = \(\frac{\sqrt{m^2 V^2+M^2 v^2}}{M+m}\)

Also, (2) ÷ (1) gives, \(\tan \theta=\frac{M v}{m V} \text { or, } \theta=\tan ^{-1} \frac{M v}{m V} .\)

Example 4. A body of mass 50 kg is projected vertically upwards with a velocity of 100 m s^-1. After 5 s it splits up into two parts due to an explosion. One part of mass 20 kg moves vertically upwards with a velocity of 150 m s^-1. Find the velocity of the second body. Find the sum of the momenta of the two parts 3 s after the explosion and show that if there was no explosion, the momentum of the body would have been constant.
Solution:

Let the velocity of the projectile 5 s after the projection and just before the explosion be v.

∴ v = 100- 9.8 x 5 = 51 m · s^-1

Let the velocity of the second part after the explosion be v₁.

Applying the law of conservation of linear momentum along the direction of projection, 50 x 51 = 20 x 150 + 30 x v₁.

or, \(v_1=\frac{50 \times 51-3000}{30}=-15 \mathrm{~m} \cdot \mathrm{s}^{-1} \text { (downwards) }\)

Let the velocity of the 20 kg mass, produced due to the explosion, 3 seconds after the explosion be v’.

∴ v’ = 150- 9.8 x 3

= 150-29.4 = 120.6 m · s^-1 (upwards)

If v” is the velocity of the 30 kg mass 3 seconds after the explosion, then v” =15 + 9.8×3

= 15 + 29.4 = 44.4 m · s^-1 (downwards)

Thus the total momentum 3 seconds after the explosion = 20 x 120.6- 30 x 44.4 = 1080 kg · m · s^-1

In the case of no explosion, the velocity after 8 seconds of projection would have been v₂ = 100-9.8×8 = 100-78.4 = 21.6 m · s^-1

Hence, its momentum after 8 s would have been 50 x 21.6 = 1080 kg · m ·  s^-1

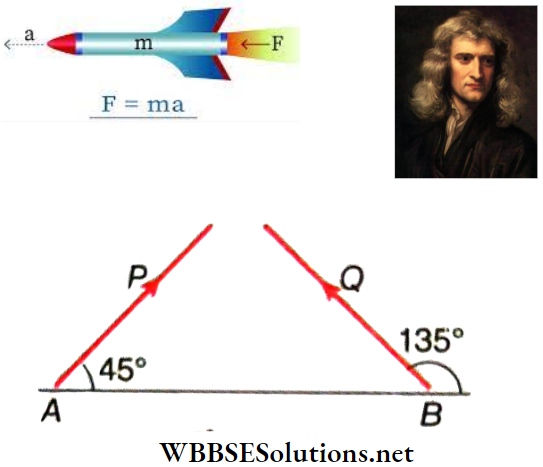
Example 5. A body P of mass 20 g and another body Q of mass 40 g are projected at the same time from points A A and B on the earth’s surface. The velocity of projection for each was 49 m · s^-1 and it was directed at an angle of 45° with the horizontal. Distance AB = 245 m. P and Q collide on the same vertical plane. After the collision, P retraces its path to the ground. Find the position where Q touches the ground. How long will Q take to reach the ground after the collision? [g = 9.8 m · s^-2]

Solution:

A body P of mass 20 g and another body Q of mass 40 g are projected at the same time from points A A and B on the earth’s surface. The velocity of projection for each was 49 m · s^-1 and it was directed at an angle of 45° with the horizontal. Distance AB = 245 m. P and Q collide on the same vertical plane. After the collision, P retraces its path to the ground.

As the two bodies P and Q are projected at the same time with the same velocity u = 49 m · s^-1 and angle = 45°, the horizontal range for both P and Q is the same an equal to, \(\frac{u^2 \sin 2 \alpha}{g}=\frac{(49)^2 \times \sin 90^{\circ}}{9.8}=245 \mathrm{~m} .\)

Hence the two bodies P and Q will meet at a point on the perpendicular bisector of AB.

Let the time when P and Q meet after projection be t. Hence horizontal distance moved by each x = \(49 \cos 45^{\circ} \times t=\frac{245}{2}, \quad \text { or, } t=\frac{5}{\sqrt{2}} \mathrm{~s}\)

During the collision, the vertical velocity of P and Q \(u_y=u \sin 45^{\circ}-g t=\frac{49}{\sqrt{2}}-\frac{9.8 \times 5}{\sqrt{2}}=0\)

Horizontal velocity of P and Q \(u_x=u \cos 45^{\circ}=\frac{49}{\sqrt{2}} \mathrm{~m} \cdot \mathrm{s}^{-1}\)

Hence horizontal component of the total momentum before collision p_x = (0.02 u_x – 0.04 u_x) kg · m · s^-1

P retraces its path after the collision. Hence horizontal velocity of P after collision =-u_x and for Q it is = v (say).

Hence horizontal component of the total momentum of the system after the collision = (- 0.02 u_x + 0.04 v) kg · m · s^-1

From the law of conservation of momentum, 0.02 u_x– 0.04 u_x = (- 0.02 u_x + 0.04 V)

or, v = 0

Thus Q does not have any horizontal component of velocity and hence it falls down vertically at the midpoint of AB, at a distance 245/2 = 122.5 m from both A and B

Let t = time required by Q to reach the ground after the collision.

t = \(\frac{u \sin 45^{\circ}}{g}=\frac{49 \times 1}{\sqrt{2} \times 9.8}=3.53 \mathrm{~s} .\)

Example 6. A car of mass 2000 kg collides with a truck of mass 10⁴ kg moving at 48 km · h^-1. After the collision, the car rides up the truck, and the truck-car combination moves at 15 km · h^-1. What was the velocity of the car before the collision?
Solution:

A car of mass 2000 kg collides with a truck of mass 10⁴ kg moving at 48 km · h^-1. After the collision, the car rides up the truck, and the truck-car combination moves at 15 km · h^-1.

Suppose a body of mass m moving with velocity u collides along a straight line with a body of mass M and velocity v. After collision the two masses combine and move with velocity V. Applying the law of conservation of equal to, momentum, mu + mv = (m + M)V

or, mu = (m + M)V- Mv

or, \(u=\frac{m+M}{m} V-\frac{M}{m} v=\left(1+\frac{M}{m}\right) V-\frac{M}{m} v\).

Here \(m=2000 \mathrm{~kg}, v=48 \mathrm{~km} \cdot \mathrm{h}^{-1}, M=10000 \mathrm{~kg}\),

V = \(15 \mathrm{~km} \cdot \mathrm{h}^{-1}\)

∴ \(\frac{M}{m}=\frac{10000}{2000}=5\)

u = \((1+5) \times 15-5 \times 48=90-240=-150 \mathrm{~km} \cdot \mathrm{h}^{-1}\)

The negative sign indicates that before collision the car was moving in the direction opposite to that of the truck.

Example 7. A ball weighing 100 g was thrown vertically upwards with a velocity 49 m · s^-1. At the same moment, another identical ball was dropped from a height of 98 m vertically above the first ball. After some time the two balls collided and got stuck together. This combined mass reached the ground finally. Determine how long the balls were in motion.
Solution:

A ball weighing 100 g was thrown vertically upwards with a velocity 49 m · s^-1. At the same moment, another identical ball was dropped from a height of 98 m vertically above the first ball. After some time the two balls collided and got stuck together. This combined mass reached the ground finally.

Let the height attained by the balls above the ground in time t₁ be h when they collide with each other.

Analyzing the upward motion of the first ball, we get \(h=49 t_1-9.8 t_1^2 / 2\)…(1)

Analyzing the downward motion of the second ball, we get \(98-h=9.8 t_1^2 / 2\)…(2)

From equations (1) and (2), 98 = 49 t₁ or, t₁ = 2 s

At the time of collision, the velocity of the ball thrown in the upward direction is v₁ and that of the ball thrown in the downward direction is v₂.

∴ v₁ = 49 -9.8 x 2 = 29.4 m · s^-1(upward)

and v₂ = 9.8 x 2 = 19.6 m · s^-1 (downward)

After collision, the velocity of the combined mass is V. Then according to the law of conservation of momentum, 0.1 x 29.4- 0.1 x 19.6 = 2 x 0.1 x V

or, V = 4.9m · s^-1 (upward)

From equation (1), we get h = 49 x 2- 9.8 x (2)²/2 = 78.4 m

Let the time taken by the combined mass to reach the ground be t₂.

Then \(78.4=-4.9 t_2+9.8 \times t_2^2 / 2 \quad \text { or, } t_2^2-t_2-16=0\)

or, \(t_2=(1 \pm \sqrt{1+64}) / 2=4.53 \mathrm{~s}\) (because t 0)

∴ Total time = \(t_1+t_2=2+4.53=6.53 \mathrm{~s} .\)

Example 8. A body of mass m is at rest on a smooth horizontal plane. A force F = kt is applied on the body making an angle α with the horizontal. In the equation of force, t is time and k is a constant. At the instant the object loses contact with the plane, how far will it move along the plane and what will be its velocity?
Solution:

A body of mass m is at rest on a smooth horizontal plane. A force F = kt is applied on the body making an angle α with the horizontal. In the equation of force, t is time and k is a constant. At the instant the object loses contact with the plane

As F= kt, the force increases with time. The vertical component of the force = Fsinα.

The body loses contact with the plane when the vertical force equals the weight of the body, i.e., Fsinα = mg. Let the time after which the body loses contact be t₀.

∴ Fsinα = kt₀sinα = mg

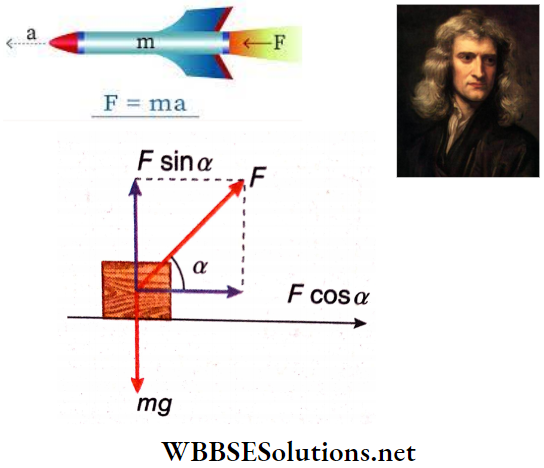
∴ \(t_0=\frac{m g}{k \sin \alpha}\)

The horizontal component of the force F = Fcosα = ktcosα

Hence horizontal acceleration = \(\frac{k t \cos \alpha}{m}=\frac{d v_x}{d t}\)

∴ \(d v_x=\frac{k t \cos \alpha}{m} \cdot d t\)

Integrating, \(v_x=\frac{k \cos \alpha}{m} \frac{t^2}{2}+A\)…(1)

[where A = integration constant]

As the body starts from rest, at t = 0, v = 0.

Inserting this in equation (1), A = 0

∴ \(v_x=\frac{k \cos \alpha}{2 m} t^2\)…(2)

If the displacement along the plane is s, then \(v_x=\frac{d s}{d t}=\frac{k \cos \alpha}{2 m} t^2 \quad \text { or, } d s=\frac{k \cos \alpha}{2 m} t^2 d t\)

Integrating again, \(s=\frac{k \cos \alpha}{6 m} t^3+B\)…(3)

[where B = integration constant]

As at the initial moment, the displacement is zero, i.e., at t = 0, s = 0.

From equation (3), we get B = 0

Inserting the value of B in equation (3), \(s=\frac{k \cos \alpha}{6 m} r^3\)

Hence, displacement along the plane in time t0, \(s_0=\frac{k \cos \alpha}{6 m} t_0^3=\frac{k \cos \alpha}{6 m}\left(\frac{m g}{k \sin \alpha}\right)^3\)

or, \(s_0=\frac{m^2 g^3 \cos \alpha}{6 k^2 \sin ^3 \alpha}\) and corresponding velocity, \(v_0=\frac{k \cos \alpha}{2 m}\left(\frac{m g}{k \sin \alpha}\right)^2\)

∴ \(v_0=\frac{m g^2 \cos \alpha}{2 k \sin ^2 \alpha} .\)

Example 9. A cannonball of mass 50 kg is fired with a velocity of 40 m s^-1 from a cannon of mass 1000 kg. What will be the recoil velocity of the cannon? If the force of friction between the surface and the wheels of the cannon is 1/10th of the weight of the cannon, how far will the cannon move before coming to rest? [Given, g = 10 m s^-2].
Solution:

A cannonball of mass 50 kg is fired with a velocity of 40 m s^-1 from a cannon of mass 1000 kg. What will be the recoil velocity of the cannon? If the force of friction between the surface and the wheels of the cannon is 1/10th of the weight of the cannon

Let the velocity of the recoil of the cannon be V.

Hence, from the law of conservation of momentum \(0=m v+M V \quad \text { or, } V=-\frac{m v}{M}\)

Here, m = mass of the cannonball = 50 kg, v = its velocity = 40 m · s^-1, and M = mass of the cannon = 1000 kg.

From given data, V \(=-\frac{50 \times 40}{1000}=-2 \mathrm{~m} \cdot \mathrm{s}^{-1}\)

Hence, the recoil velocity of the cannon = 2 m · s^-1 (backward).

Frictional force between the wheels and the land surface = 1/10 x 1000 x 10 = 1000N

∴ Retardation of the cannon = \(\frac{1000 \mathrm{~N}}{1000 \mathrm{~kg}}=1 \mathrm{~m} \cdot \mathrm{s}^{-2}\)

∴ Distance traveled by the cannon before coming to rest is, \(s=\frac{V^2}{2 a}=\frac{2^2}{2 \times 1}=2 \mathrm{~m}\)

Example 10. Four identical blocks, each of mass m, are connected as shown and are kept on a horizontal table. A force F Is applied on the first block. Find the tension in each string, neglecting friction.
Solution:

Four identical blocks, each of mass m, are connected as shown and are kept on a horizontal table. A force F Is applied on the first block.

Let the acceleration of the system, on applying force F on the first block, be a. Let tension in the string connecting the 1st and the 2nd block be T₁, 2nd, and that for the 3rd be T₂ and for the 3rd and the 4th be T₃.

Equations of motion for the

1st block, F- T₁ = ma…(1)

2nd block, T₁-T₂ = ma……(2)

3rd block, T₂-T₃ = ma….(3)

and 4th block, T₃ = ma …(4)

Since T₃ = ma, T₂ = ma+ T₃ = 2ma and T₁ = 3ma.

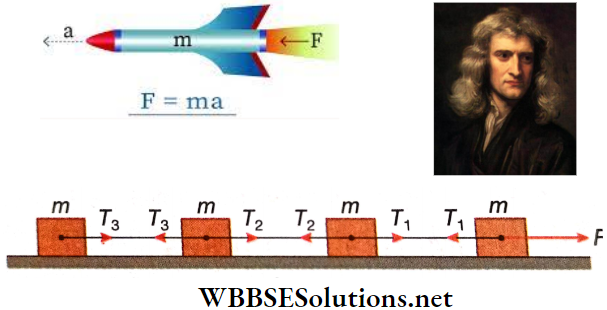
Hence, applied force, F = ma+ T₁ = 4 ma

Expressing the tensions in terms of the applied force F, we get \(T_1=\frac{3}{4} F, T_2=\frac{F}{2} \text { and } T_3=\frac{F}{4} \text {. }\)

Newton Law Of Motion – Motion Of An Object Or A System Of Objects With Variable Mass

The mass of an object or a system of objects may change with time. Newton’s second law of motion can be applied in this case only after taking into account the time-variation of mass.

• Suppose you are cycling along a road with a uniform velocity, carrying your friend in the backseat. You ask your friend to get down by jumping from the running cycle.
• Then you can realize that suddenly your velocity has increased a bit. The change of velocity would depend on the momentum with which your friend got down from the cycle.

Many examples of this type can be cited which are part of our day-to-day experience.

Equation Of Motion Of An Object Or A System Of Objects With Variable Mass: Let us suppose, at any moment t, the mass and velocity of an object (moving in a straight line) be m and v respectively and the external fixed net force acting on the object be F.

At that moment, another object of an infinitesimal mass dm moving in the same straight line with velocity u is added with the first one. As a result, after an infinitesimal interval of time, i.e., at the moment (f + dt), the mass of the system of objects becomes (m + dm), and let us assume that the velocity becomes (v + dv).

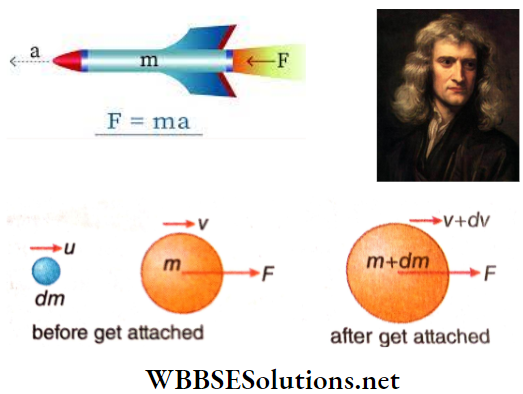
Now the initial and final momentum of the system of the objects would be respectively, p = mv+ udm….(1)

and p+dp = (m+ dm)(v+ dv)

= mv+ mdv+ vdm…….(2)

As the quantity dmdv is very very small, it is ignored.

So, change in momentum of the system of objects in time dt (p+ dp) – p = mv+ mdv+ vdm – mv- udm

or, dp = mdv-(u-v)dm

Therefore, according to Newton’s second law of motion, F = \(\frac{d p}{d t}=m \frac{d v}{d t}-(u-v) \frac{d m}{d t}\)

It is to be noted that, (u-v) is the relative velocity of dm with respect to m. Putting u_rel in place of (u – v) in equation (3) we get,

F = \(m \frac{d v}{d t}-u_{\text {rel }} \frac{d m}{d t} \quad \text { or, } F+u_{\text {rel }} \frac{d m}{d t}=m \frac{d v}{d t}\)…(4)

The resulting acceleration of the system is \(\frac{dv}{dt}\) = a.

So from equation (4), \(m a=F+u_{\mathrm{rel}} \frac{d m}{d t}\)….(5)

In the case of time-varying mass, equation (4) or (5) is the effective form of Newton’s second law of motion. Note that, due to time-variation of mass the last term in equation (5) is added to the familiar equation ma = F.

 Newton Law Of Motion – Rocket And Jet Plane

Rocket And Jet Plane Working Principle: A rocket or a jet plane works on the principle of conservation of momentum. In a rocket or a jet engine, there is a combustion chamber with a small aperture (exhaust) H at its rear end.

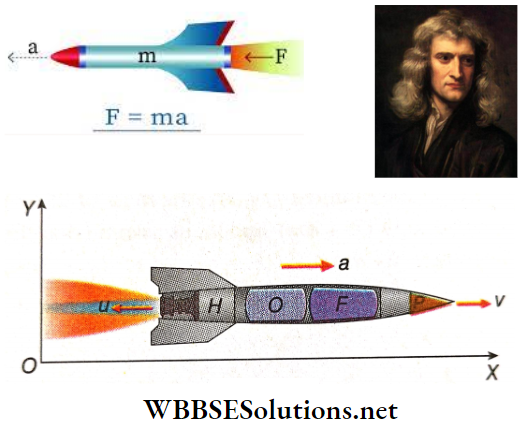
Solid or liquid fuel is ignited in the chamber. As a result of combustion, a large amount of spent fuel, in gaseous form, escapes at a high velocity through the exhaust. This provides a forward thrust to the rocket.

Force On The Rocket And Acceleration: Suppose, at time t, m= mass of a rocket, and v= its velocity in an inertial frame of reference. So, initial momentum of the system, p = mv.

When fuel is burnt in the combustion chamber, a gas is formed. High pressure within the chamber forces the gas out of a nozzle at the back of the rocket. Let the gas eject at a constant speed u relative to the rocket (u is assumed to be constant); the actual speed with respect to the same inertial frame = (u + v).

Let, dm = mass of the gas ejected in time dt

m – dm = residual mass of the rocket

and, v+ dv = velocity of the rocket after time dt

Then, total momentum after time dt, p+ dp = (u+ v)dm + (m- dm)(v+ dv) = mv+ udm + mdv [ignoring the product dmdv]

So, the change of momentum in time dt, dp = (p+ dp) – p = udm + mdv

And, external force = rate of change of momentum =\(\frac{d p}{d t}=u \frac{d m}{d t}+m \frac{d v}{d t}=u \frac{d m}{d t}+m a\)

where, a = \(\frac{dv}{dt}\) = acceleration of the rocket.

As no external force is acting on the rocket, we have 0 = \(u \frac{d m}{d t}+m a, \quad \text { or, } a=-\frac{u}{m} \frac{d m}{d t}\)…(1)

The negative sign shows that the rocket is accelerated in a direction opposite to that of u. Therefore, the rocket increases its speed in the forward direction as a result of the backward exhaust of the burnt fuel.

A jet plane cannot fly where there is no air, because oxygen from air is used for combustion. Otherwise, its motion follows the same working principle as that of a rocket.

Newton Law Of Motion – Rocket And Jet Plane Numerical Examples

Example 1. A wagon is moving along a straight railway track with a velocity of 3.2 m · s^-1. The wagon is being loaded with coal in moving condition at a rate of 540 kg · min^-1. How much force is to be applied to move the wagon at a constant velocity? Mention the direction of force. Assume, the initial velocity of the coal in the horizontal direction is zero.
Solution:

A wagon is moving along a straight railway track with a velocity of 3.2 m · s^-1. The wagon is being loaded with coal in moving condition at a rate of 540 kg · min^-1.

The velocity of the wagon, v = 3.2 m · s^-1,

and the rate of change of mass of the wagon, \(\frac{d m}{d t}=540 \mathrm{~kg} \cdot \mathrm{min}^{-1}=\frac{540}{60} \mathrm{~kg} \cdot \mathrm{s}^{-1}=9 \mathrm{~kg} \cdot \mathrm{s}^{-1}\)

Hence, force to be applied on the wagon to maintain its constant velocity (acceleration, a = \(\frac{dv}{dt}\) = 0),

F = \(v \frac{d m}{d t}+m \frac{d v}{d t}=3.2 \times 9+0=28.8 \mathrm{~N}\)

In this case, the direction of applied force and the direction of velocity of the wagon is identical.

Example 2. A rocket loses 1/40th of its mass in one second, during its upward motion. The speed of ejection of gas is 4000 m · s^-1. Find the acceleration gained.
Solution:

A rocket loses 1/40th of its mass in one second, during its upward motion. The speed of ejection of gas is 4000 m · s^-1.

In this case, \(\frac{dm}{m}\) = \(\frac{1}{40}\), dt = 1s,

u = – 4000 m ·  s^-1 (since u is in a downward direction)

Hence, acceleration, a = \(-\frac{u}{m} \frac{d m}{d t}=-\frac{u}{d t} \frac{d m}{m} \)

= \(4000 \times \frac{1}{40}=100 \mathrm{~m} \cdot \mathrm{s}^{-2} .\)

The positive value shows that the acceleration is upwards.

Example 3. A rocket is using 200 kg of fuel per second for its flight. Gas produced during combustion is ejected at a velocity of 6000 m • s^-1. What is the force acting on the rocket?
Solution:

A rocket is using 200 kg of fuel per second for its flight. Gas produced during combustion is ejected at a velocity of 6000 m • s^-1.

Force acting on the rocket, F = \(\frac{dm}{dt}\)u.

Given, rate of combustion of fuel, \(\frac{dm}{dt}\) = 200 kg · s^-1, the velocity of ejection of gas, u = 6000 m · s^-1.

∴ F = -200 x 6000 kg · m- s^-2 = -1.2 x 10⁶ N

The negative sign indicates that the force acts in the direction opposite to that of the ejected gas.

Example 4. A machine gun fires bullets at the rate of 180 shots per minute. Each bullet is of mass 20 g and moves with a velocity of 1 km · s^-1. After colliding perpendicularly with a steel plate, the bullets rebound at half the incident speed. What will be the force required to keep the steel plate in position?
Solution:

A machine gun fires bullets at the rate of 180 shots per minute. Each bullet is of mass 20 g and moves with a velocity of 1 km · s^-1. After colliding perpendicularly with a steel plate, the bullets rebound at half the incident speed.

Velocity of each bullet before impact = 1 km · s^-1 = 1000 m · s^-1

Velocity of each bullet after impact

= -1/2 km · s^-1 = -1/2 x 1000 m · s^-1 = -500 m · s^-1

The negative sign indicates this velocity is oppositely directed.

∴ Change in velocity of each bullet after impact = 1000 – (-500) = 1500 m · s^-1

Number of bullets incident per second on the steel plate = \(\frac{180}{60}\) = 3

∴ Rate of change of momentum of the three bullets = 3 x \(\frac{2}{1000}\) x 1500 = 90 N = force exerted on the steel plate.

Hence, the force required to hold the steel plate in position = 90 N.

Example 5. Just before take-off, the mass of a rocket is 4000 kg and the velocity of ejection of the burnt fuel is 400 m · s^-1. What should be the rate of combustion of the fuel so that the rocket can take off vertically?
Solution:

Just before take-off, the mass of a rocket is 4000 kg and the velocity of ejection of the burnt fuel is 400 m · s^-1.

Let the mass of gas ejected per second be m.

∴ Change of momentum of the ejected gas per second = mv = 400m N.

∴ Force on the ejected gas =400mN = upward vertical reaction on the rocket.

Weight of the rocket = 4000 x 9.8 N.

For taking off, the vertical reaction force on the rocket must be slightly greater than the weight of the rocket.

As the limiting case, the vertical reaction force = weight of the rocket.

∴ 400m = 4000 x 9.8 or, m = 98 kg · s^-1.

Newton Law Of Motion Synopsis

Newton’s First Law: Everybody continues to be in its state of rest or of uniform motion in a straight line unless the body is compelled to change its state by a net external force.

Newton’s Second Law: The rate of change of momen¬tum of a body is proportional to the impressed force and takes place in the direction in which the force acts.

Newton’s Third Law: To every action, there is an equal and opposite reaction.

• Property by the virtue of which a body tries to retain its state of rest or of uniform motion is called inertia of the body. There are two types of inertia—inertia of rest and inertia of motion.
• The tendency of a stationary body to remain at rest forever is called its inertia of rest.
• The tendency of a moving body to maintain its motion in a straight line at a constant velocity is called its inertia of motion.

The external influence that changes or tends to change the state of rest or state of uniform motion of a body is called force. Force is a vector quantity.

• The dynamic property arising from the combined effect of mass and velocity of a moving body is called its momentum.
• A frame of reference, at rest or in uniform motion, is called an inertial frame of reference.
• All three of Newton’s laws of motion are applicable in an inertial frame of reference.

An accelerating frame of reference is called a non-inertial frame of reference. None of Newton’s laws of motion holds good in a non-inertial frame of reference.

• Mass of a body determines its inertia. Hence this mass is called inertial mass.
• For a force acting on a body for an interval of time, the product of this force and the time is called the impulse of that force.
• If a large force acts on a body for a very short interval of time, it is called an impulsive force.
• Law of conservation of linear momentum: In the absence of any external force acting on a system of bodies—even if action-reaction forces exist among them, the total linear momentum of the system remains conserved.
• A rocket’s or a jet plane’s motion is based on the law of conservation of linear momentum.

The oxidant (oxygen) required for the combustion of rocket fuel is carried in the rocket itself so the rocket can travel in outer space where there is no air. A jet plane requires atmospheric oxygen to bum its fuel. Therefore, a jet plane cannot fly in space.

Newton Law Of Motion Useful Relations For Solving Numerical Problems

Momentum, p = mv

Applied force, F = \(m a=m\left(\frac{v-u}{t}\right)=\frac{m v-m u}{t}\)

Impulse of a force, Ft = mv- mu.

If the reaction force or normal force of a moving lift is R, its value when the lift

Ascends with an acceleration a is, R = m(g+ a)

Descends with an acceleration a is, R = m(g- a)

Ascends with a retardation a is, R = m(g- a)

Descends with a retardation a is, R = m(g+ a)

When the lift is at rest or ascending or descending with uniform velocity, R – mg

During free fall R = 0.

Law of conservation of linear momentum between two bodies: m₁u₁ + m₂u₂ = m₁v₁+ m₂v₂

The form of Newton’s second law of motion for time-varying mass, \(F+u_{\mathrm{rel}} \frac{d m}{d t}=m a\)

 

Newton Law Of Motion Very Short Answer Type Questions

Question 1. As per Newton’s laws of motion, only an external force can change the inertia of a body. When a car is brought to rest by the application of brakes, which external force stops the car?
Answer: Frictional force applied by road

Question 2. A man, in a train in uniform motion, throws a ball vertically upwards. Will the ball return to his hand?
Answer: Yes

Question 3. From the roof of a train, a metal ball is suspended by a string. When the train moves with uniform velocity, will the string remain vertical?
Answer: Yes

Question 4. A body in motion is acted upon by a force. Will the body stop at the moment of withdrawal of the force?
Answer: No

Question 5. A force of 200 dyn acts on a mass of 10 g for 5 s. Initially the body was at rest. What will be the final velocity of the mass?
Answer: 100cm · s^-1

Question 6. A man is coming down a hanging rope. The rope can bear up to 2/3 of his weight. The minimum acceleration with which the man can come down is _________
Answer: g/ 3

Question 7. Write the name of the physical quantity whose unit is the same as that of the impulse of a force.
Answer: Momentum

Question 8. A body of weight W₁ is suspended from the ceiling of a room by a rope of weight W₂. What is the force exerted by the ceiling on the rope?
Answer: W₁ + W₂

Question 9. Arrangements of two identical pulleys are shown in Fig. 1.49. The strings have negligible masses. In (1) mass m is pulled up by attaching a mass 2 m at the other end of the string and (2) mass m is pulled up by applying a downward force of 2 mg at the other end of the string. What are the accelerations in the two cases?

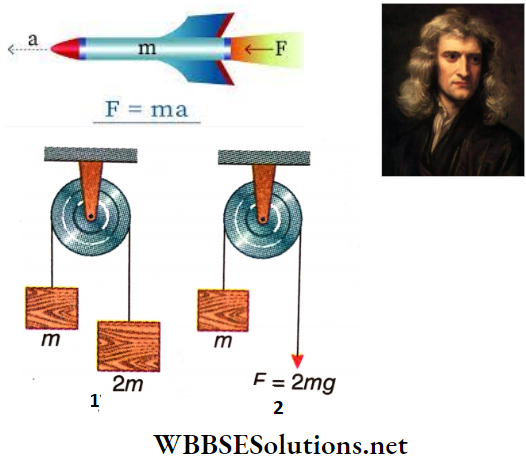
Answer: g/3,g

Question 10. A spring balance is set in a stationary lift. A person of mass 50 kg is standing on that balance. What will be the change in the reading of the balance if the lift moves upwards with constant velocity?
Answer: No change

Question 11. A spring balance is set in a stationary lift. A person of mass 50 kg is standing on the balance. What will be the change in the balance reading when the lift moves upwards with a constant acceleration?
Answer: An increase

Question 12. A man of mass 50 kg is descending at a constant velocity using a parachute. What is the air resistance on the man?
Answer: 490 N

Question 13. Two bodies of equal masses are kept on the scale pans of a beam balance in a lift. If the lift starts moving up with an acceleration, will the beam balance be in equilibrium?
Answer: Yes

Question 14. Can a rocket operate in free space?
Answer: Yes

Question 15. What is the principle of Rocket propulsion?
Answer: Conservation of linear momentum

Question 16. A bomb explodes in mid-air into two equal fragments. What is the direction of motion of the two fragments?
Answer: Opposite to each other

Newton Law Of Motion Assertion Reason Type Question And Answers

Direction: These questions have statement 1 and statement 2. Of the four choices given below, choose the one that best describes the two statements.

1. Statement 1 is true, statement 2 is true; statement 2 is a correct explanation for statement 1.
2. Statement 1 is true, and statement 2 is true; statement 2 is not a correct explanation for statement 1.
3. Statement 1 is true, statement 2 is false.
4. Statement 1 is false, and statement 2 is true.

Question 1.

Statement 1: A cloth covers a table. Some dishes are kept on it. The cloth can be pulled out without dislodg¬ing the dishes from the table.

Statement 2: For every action, there is an equal and opposite reaction.

Answer: 2. Statement 1 is true, statement 2 is true; statement 2 is not a correct explanation for statement 1.

Question 2.

Statement 1: A reference frame attached to the earth is an inertial frame of reference.

Statement 2: The reference frame which has zero acceleration is called an inertial frame of reference.

Answer: 4. Statement 1 is false, statement 2 is true.

Question 3.

Statement 1: A concept of pseudo forces is valid both for inertial as well as non-inertial frames of reference.

Statement 2: A frame accelerated with respect to an inertial frame is a non-inertial frame.

Answer: 4. Statement 1 is false, statement 2 is true.

Question 4.

Statement 1: Block A is moving on a horizontal surface towards right under the action of force F. All surfaces are smooth. At the instant shown, the force exerted by block A on block B is equal to the net force on block B.

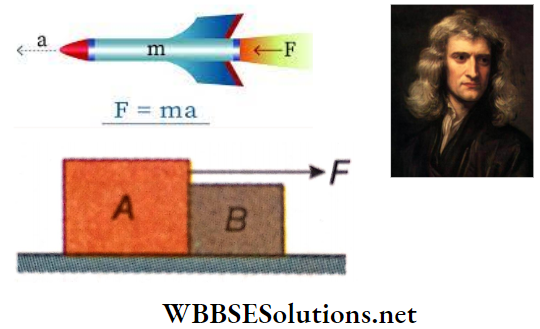
Statement 2: From Newton’s third law of motion, the force exerted by block A on B is equal in magnitude to the force exerted by block B on A.

Answer: 4. Statement 1 is false, statement 2 is true.

Question 5.

Statement 1: During free fall of a person one feels weightlessness because his weight becomes zero.

Statement 2: He falls with an acceleration of g.

Answer: 1. Statement 1 is true, statement 2 is true; statement 2 is a correct explanation for statement 1.

Question 6.

Statement 1: When a person walks on a rough surface, the net force exerted by the surface on the person in the direction of his motion.

Statement 2: It is the force exerted by the road on the person that causes the motion.

Answer: 4. Statement 1 is false, statement 2 is true.

Newton Law Of Motion Match Column 1 With Column 2

Question 1. A block of mass m is released from rest when the spring was in its natural length. The pulley also has mass m but it is frictionless. Suppose the value of m is such that finally it is able to just lift the block M.

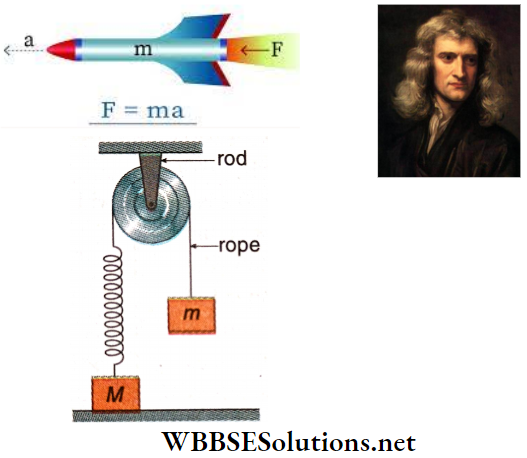
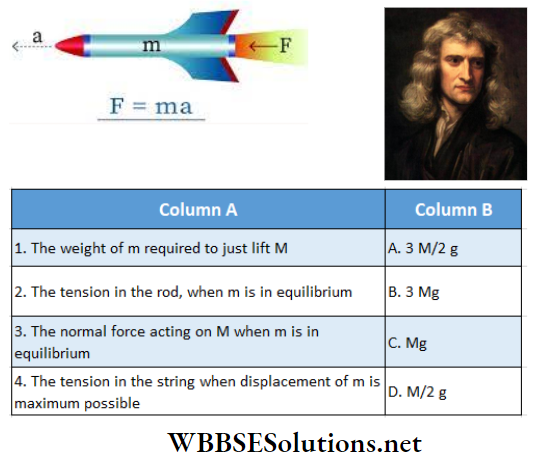
Answer: 1. C, 2. C,3. C, 4. B, D

Question 2. In the diagram shown, match the following column, (g = 10 m/s²)

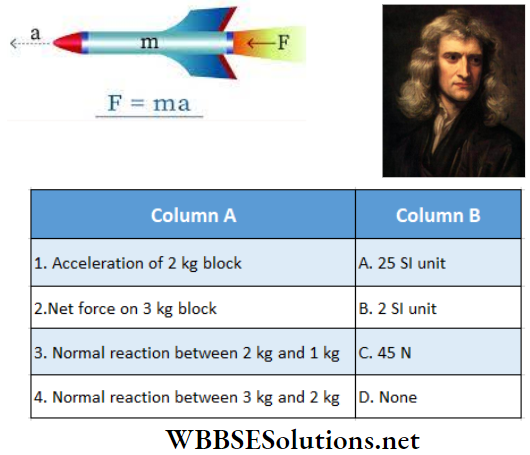
Answer: 1. B, 2. D, 3. A, 4. D

Newton Law Of Motion Comprehension Type Question And Answers

Read the following passages carefully and answer the questions at the end of them.

Question 1. A ball of mass 200 g is thrown with a speed 20 m · s^-1. The ball strikes a bat and rebounds along the same line at a speed of 40 m · s^-1. Variation in the interaction force, as long as the ball remains in contact with the bat, is shown.

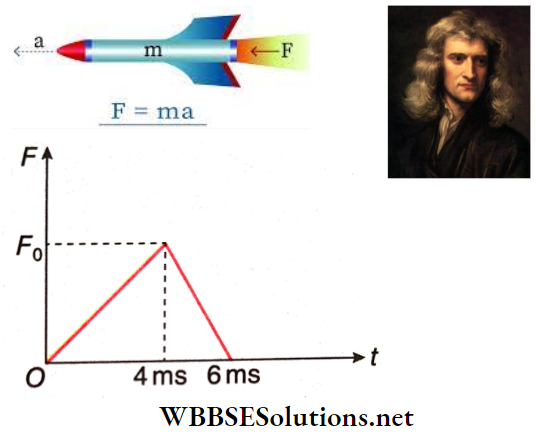
1. Maximum force F₀ exerted by the bat on the ball is

1. 4000 N
2. 5000 N
3. 3000 N
4. 2500 N

Answer: 1. 4000 N

2. Average force exerted by the bat on the ball is

1. 5000 N
2. 2000 N
3. 2500 N
4. 6000 N

Answer: 2. 2000 N

3. What is the speed of the ball at the instant the force acting on it is maximum?

1. 40 m · s^-1
2. 30 m · s^-1
3. 20 m · s^-1
4. 10 m · s^-1

Answer: 3. 20 m · s^-1

Question 2. Three blocks m₁ = 10 kg, m₂ = 20 kg, and m₃ = 30 kg are on a smooth horizontal table, connected to the adjacent blocks by light horizontal strings. A horizontal force F = 60 N is applied to m₃, towards the right.

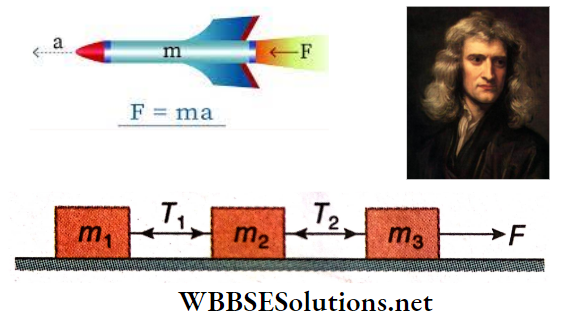
1. The tension (T₁) acting between m₁ and m₂ is

1. 10N
2. 15N
3. 20N
4. 25N

Answer: 1. 10N

2. Tension (T₂) acting between m₂ and m₃ is

1. 25N
2. 30N
3. 24N
4. 15N

Answer: 2. 30N

3. The tension (T₂), if all of a sudden the string between m₁ and m₂ snaps, is

1. 30N
2. 24N
3. 25N
4. 15N

Answer: 2. 24N

Question 3. A stone of mass 0.05 kg is thrown in vertically by upward direction (take g = 10 m · s^-2). Neglect air friction,

1. The net force acting on the stone during its upward motion is

1. 0.5 N, upward
2. 0.5 N, downward
3. 5 N, upward
4. Zero

Answer: 2. 0.5 N, downward

2. The net force acting on the stone during its downward motion is

1. 0.5 N, upward
2. 0.5 N, downward
3. 5 N, upward
4. Zero

Answer: 2. 0.5 N, downward

Newton Law Of Motion Integer Answer Type Questions

In this type, the answer to each of the questions is a single-digit integer ranging from 0 to 9.

Question 1. On planet X, a man throws a 500 g mass with a speed of 20 m · s^-1 and catches it as it comes down 20 seconds later. Find the weight of the mass (in N units).
Answer: 1

Question 2. The elevator shows it is descending with an acceleration of 2 m · s^-1. The mass of the block A = 0.5 kg. What should be the force (in N) exerted by the block A on the block B? Given g = 10 m • s^-2.

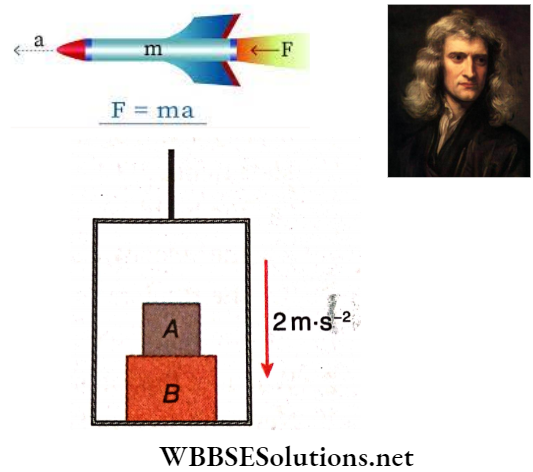
Answer: 4

Question 3. A monkey of mass 30 kg climbs a rope that can withstand a maximum tension of 360 N. Find the maximum acceleration (in m · s^-2) of the climbing monkey which this rope can tolerate, (g = 10m · s^-2)
Answer: 2

Question 4. In the arrangement shown the mass M is very heavy compared to m (M»m). The tension in the string is nmg. Find the value of n.

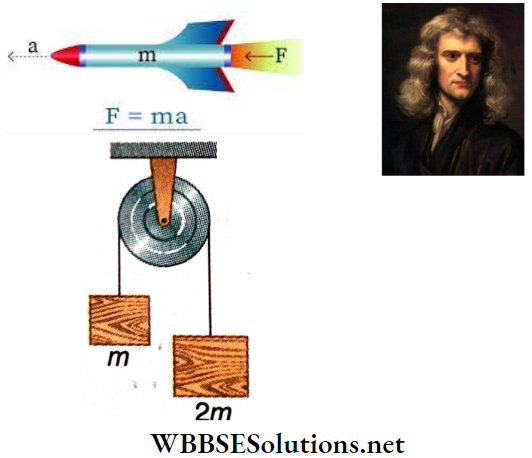
Answer: 4

Question 5. Find the acceleration of three blocks (in m • s^-2) as shown. Each surface of the system is smooth.

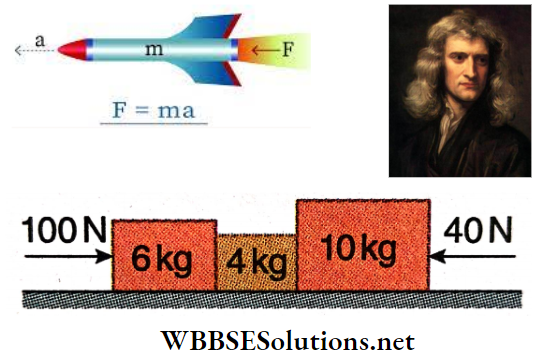
Answer: 3