WBBSE Solutions For Class 9 Maths Modern Algebra Chapter 1 Set Theory

Modern Algebra Chapter 1 Set Theory

Chapter 1 Set Theory Introduction 

1. Set theory is a key basis of mathematics.
2. Without the concept of set theory, any subject of mathematics like calculus, algebra, etc. can never be completely discussed.
3. George Bull, an English mathematician at first discussed set theory.
4. Later on, George L.P. Cantor, a German mathematician developed it.
5. He is called the father of modern set theory.

Read and Learn More WBBSE Solutions For Class 9 Maths

Chapter 1 Set Theory Concepts Of Sets

1. If any definition of any subject is to be made, then we have to depend on some other related subjects.
2. Because the definition is such a statement that expresses the new concepts depending on its old concepts.
3. That is why, the definition of any subject is at all impossible.
4. It is very difficult to define set theory.
5. According to their benefit of works, different persons have defined set theory differently.
6. According to Cantor-Set is a collection of certain different objects.
7. In general, a set is a collection of some well-defined different objects.
8. Here, the term ‘well-defined’ is of special significance.
9. By this term, it means whether an element x will be included in a certain set A. or not.
10. For example, it is possible to construct a set of students who have secured 60 or more than 60 in the madhyamik exam.
11. Here the student who has secured 61, is a member of that set, but who has secured 59 is not a member or element of that set.
12. But it is quite impossible to define a set by taking the intelligent students of class-9.
13. Because here the term ‘intelligent’ is not well-defined, that is, it is not possible to detect whether a certain student is intelligent or not and whether he or she will be an element of that set or not.
14. In the definition, it is noticed that the objects should be different, i.e., a certain object can not be a member of a set more than once.
15. For example, let set A consists of the roots of the equation x²- 12x +36= 0.
16. Now, the roots of x² – 12x+36= 0 are 6, 6, i.e., the roots are equal.
17. Then, A consists of only one element 6.
18. Since repeat is not allowed in the definition.

Chapter 1 Set Theory Different Symbols Relating To Set And Their Meanings

Chapter 1 Set Theory Definition Of Set

A collection of different well-defined and independent-of-order objects is called a set, i.e., a collection of objects will be said a set, if

1. The collection is well-defined.
2. Any two objects included in the collection are different from each other.
3. The objects included in the collection are independent of order.
   What is meant by a collection of well-defined objects has already been discussed earlier.
   Now, we shall explain the term independent of order.
   Order of a set means that among the objects of the set which one is at the first, which one at the second, which one at the third, …… etc.
   The objects of the set are independent of order means that the objects may take any place in the set.
   Thus the sets A = (a, e, i, o, u-) or A= (a, i, e, o, u} denote the same set.

Chapter 1 Set Use Of Symbol

Any set is usually denoted by the capital letters A, B, C, ……………X, Y, Z, etc. of English alphabets, and the elements or members of the sets are usually denoted by the small letters a, b, c, x, y, z, etc. 

Thus, if a is any element of the set A, then we write a € A and is read by a belongs to A. 

Again, if a is not an element of A, then we write A and we read it by a does not belong to A.

Chapter 1 Set Presentation Of Sets

Sets:-

There are two methods of describing sets.

1. Roster Method or Tabular Method.
2. Set-builder method or property method or statement method.

1. Roster method: The method in which all the elements of a set are expressed tabularly in a second bracket is known as the Roster method.

For examples:

1. If the vowels of English alphabets be a set V, then in the Roster method V = {a, e, i, o, u). 
2. If P denotes the set of first four prime numbers, then P= (2, 3, 5, 7).
3.  If C is the set of cube roots of 1, then C = {1, ω, ω²).
4. If N₁ denotes the set of first 6 natural numbers, then in the Roster method, N₁ = {1, 2, 3, 4, 5, 6). 

2. Set-builder method:

The method in which the characteristic or property, the elements of the set obey is expressed by a statement is known as the set-builder method.

Here no individual element is expressed in the second bracket.

Thus if each and every element of a set A obey the common property or characteristic P(x), then in the set-builder method, A is expressed as follows:

A = {x | P(x)} or, A = {x: P (x)}.

For examples:

1. If N₁ is a set of the first 6 natural numbers, then in the set builder method, N₁ is expressed as follows:

N = {x1≤x≤6 and x is an integer).

2. If V denotes the vowel of English alphabets, then V = {x: x is a vowel of English alphabets}

Chapter 1 Set Sets Of Different Types

1. Finite and Infinite Set:

The set in which the number of elements can be determined by counting them, i.e., the set in which the number of elements is finite is called a finite set.

If the number of elements of a set A is n, then n is called the degree or order of A and it is denoted by | A | or, n (A).

For examples:

1. The set A = {1, 2, 3, 4, 5, 6) is a finite set, and n (A) = 6.
2. The set V = (a, e, i, o, u) is a finite set, and n (V) = 5.

Infinite Set:

The set in which the number of elements is not countable, i.e., the number of elements is infinite, is known as an infinite set.

For examples:

1. Set of natural numbers, N = {1, 2, 3 … … ) is an infinite set.
2. A set of integers Z = {….. 3, 2, 1, 0, 1, 2, 3 ….) is an infinite set.

 

2. Singleton Set:

The set in which the number of elements is one is called a singleton set.

For examples:

1. A (2) is a singleton set,
2. B= {x: x is a positive integer and x is the root of the equation x²+2x-8=0} is a singleton set.

3. Null Set:

If the number of elements of a set is zero, then it is called a null set.

Null sets are usually denoted by o.

For examples:

1. Φ = (x: x is a whole number and 3 < x < 4) is a null set.
2. Φ = {x: x is real and roots of the equation x2+1= 0) is a null set.

4. Subset:

If two sets A and B be such that each and every element of B is an element of A, then B is called a subset of A.

It is usually denoted by B⊆ A or A ⊇ B.

For examples:

1. Let A = {1, 2, 3, 4, 5, 6, 7, 8) and B = {1, 2, 3, 4}, then B is a subset of A since each and every element of B is an element of A.
2. Let A = (a, b, c) and B = (a, b, c, d, e ), then A is a subset of B.

5. Equal Sets: 

If two sets A and B be such that every element of A is an element of B and every element of B is an element of A, i.e., if A ⊆  B and B ⊆ A, then A = B.

For examples:

1. Let A = {2, 3, 4, 5) and B = {2, 4, 3, 5), then A = B.
2. Let X = {2, 3} and Y = {x: x is the roots of x²-5x+6=0), then X = Y.

6. Proper subset and superset: 

A set A is called the proper subset of B if

1. A is a subset of B and

2. there exists at least one element in B which is not in A, i.e., at least one x ∈ B, x ∉ A.

Proper subsets are denoted by A ⊂ B.

The set B is called the superset of A and is written by B ⊃ A.

For examples :

Let N = {1, 2, 3……………} and Z = {-3, -2, -1, 0, 1, 2, 3, ……… }.

Then N ⊂ Z and N ≠ Z.

Let A = (1, 3, 5, 7) and B = {x: 1 ≤ x ≤ 8 and x = N}

A ⊂ B and A ≠ B,

∴ A ⊂ B [ ∵ 8 ∈ B, but 8 ∉ A]

7. Equivalence Sets:

Two sets A and B are said to be equivalence sets if both of them have the same number of elements.

For examples:

Let A= {1, 2, 3, 4) and B (a, b, c, d).

Here, n (A) = 4 and n (B) = 4.

∴ A and B are equivalence sets.

8. Universal Set: 

In the mathematical problems of sets, sometimes it is needed to find such a set which is the superset of all the sets consisting of the problem, i.e., all the sets of the problem are a subset of that set.

This super set is called the universal set with respect to all the sets in the specific problem.

Universal sets are usually denoted by U.

For examples:

The set of all real numbers R is a universal set with respect to all the sets of natural numbers, sets of integers, sets of rational numbers, set of irrational numbers, etc.

Also, let in a mathematical problem, A, B, and C are three sets such that A= {1, 2, 3, 4), B = (3, 4, 5}, and C = {1, 3, 5, 6, 7, 8), then with respect to A, B, C the set U = {1, 2, 3, 4, 5, 6, 7, 8} is a universal set.

1. Power Set: 

The set consisting of all the subsets of a given set A is called the power set of A and is usually denoted by P (A).

Symbolically, P (A) = (X: X ⊂ A).

For examples:

Let A = (a, b, c). P(A) = (Φ, (a), (b), (c), (a, b), (b, c), (c, a), (a, b, c}} 

Let A = {1,2}

∴ P(A)= {Φ, (1), (2), (1, 2)}

Chapter 1 Set Sets Operations On Sets

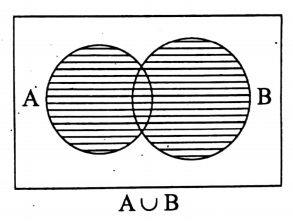
1. Union of two sets :

Let A and B be two given sets.

Then, the union of the sets A and B, denoted by \(A \cup B\), is defined by \(A \cup B\) = {x:x ∈ A or x ∈ B) i.e., \(A \cup B\) consists of all the elements of both A and B, but not repeated.

For examples:

1. Let A = (a, b, c, d) and B = (b, c, d, e)

∴ \(A \cup B\)

2. Let A = {1, 3, 5, 7……….} and B (2, 4, 6, 8……..}

∴ \(A \cup B\)

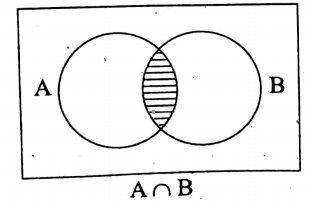
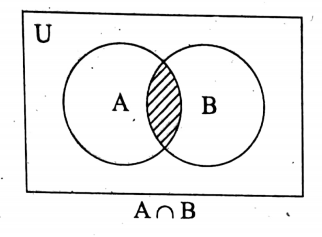
2. The intersection of two sets :

Let A and B be two given sets. 

Then the intersection of A and B, usually denoted by \(\), is defined by \(A \cap B\) = {x:x ∈ A and x ∈ B), i.e., \(A \cap B\) consists of only the common elements of both A and B.

For examples:

1. Let A = {1, 2, 3, 4, 5) and B = (2, 3, 4}

∴ \(A \cap B\) = (2, 3, 4)

2. Let A = {x:1≤x≤3, x ∈ N) and B = (x: 0≤x≤2, x ∈ N}

∴ \(A \cap B\) = (1, 2)

 

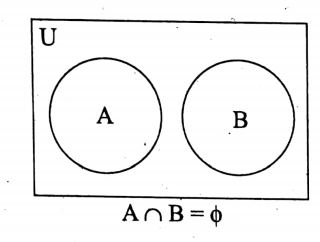
3. Disjoint sets:

If two given sets A and B be such that there is no common element in between them, then the sets are called disjoint sets.

Here, \(A \cup B\) = Φ and Φ = null set.

For examples:

Let A= {x: x is a rational number}

and B= {x: x is an irrational number}

Then clearly, \(A \cap B\) ≠ Φ

∴ A and B are disjoint sets.

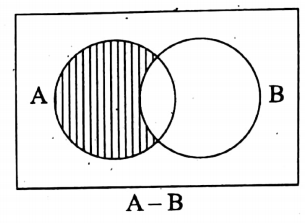
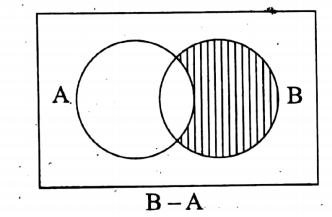
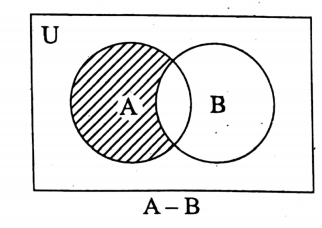
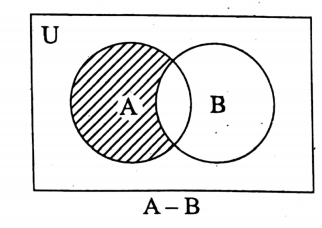
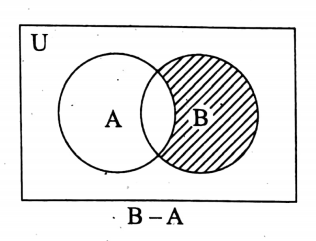
4. Difference between two sets:

Let A and B be two given sets. 

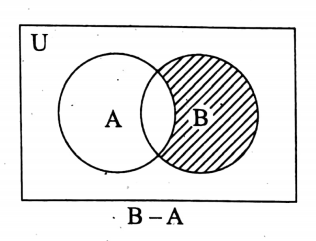
Then the difference between A and B, usually denoted by A – B or B – A is defined by A-B = {x:x ∈ A and x ∉ B). 

Similarly, B A = {x: x ∈ B and x ∉ A}

i.e., (A-B) consists of those elements which are not an element of B

For examples:

Let A= (a, b, c, d, e) and B = (c, d, e, f)

∴ A – B (a, b) and B – A = {f}

 

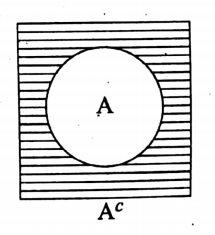
5. Complement of a set :

With respect to a universal set U, the complement of a set A, usually denoted by A’ or A^c, is defined by

Ac = U – A = (x: x ∈ U and x ∈ A).

For examples :

Let R= (x: x is real), Q= {x: x is rational}

∴ with respect to the universal set R,

Q^c = R – Q = {x: x is irrational).

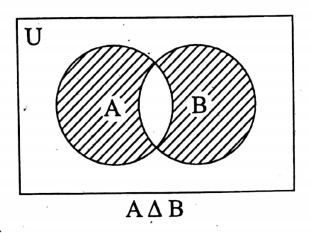
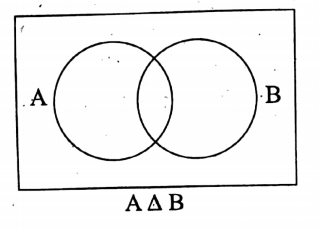
5. Symmetric Difference :

The symmetric difference of two sets A and B is usually denoted by AΔB and is defined by

\(\mathrm{A} \Delta \mathrm{B}=(\mathrm{A}-\mathrm{B}) \cup(\mathrm{B}-\mathrm{A})\)

Chapter 1 Set Sets Venn Diagram

The by which the operations on sets are presented in a befitting manner is known as Venn diagrams.

Method:

In Venn diagrams, the universal set is usually expressed by a rectangle and the subsets of the universal set are expressed by some circles which are generally shaded.

Set Operations by Venn diagrams:

1. Union of two sets:

2. Intersection of two sets:

3. disjoint Sets:

4. Difference of the two sets:

5. Complement of a set:

6. Symmetric Difference: