WBBSE Solutions For Class 9 Maths Mensuration Chapter 3 Area Of Circles

Mensuration Chapter 3 Area Of Circles

Chapter 3 Area Of Circles Area of a circle

Area of circles circumscribed in Determination of in-radius and circum-radius

Area of a circle = x (radius)² = πr² [ r = radius of circle]

The area of the region closed by two concentric circles= \(\pi\left(\mathrm{R}^2-r^2\right)=\pi(\mathrm{R}+r)(\mathrm{R}-r)\)   [R and r are the radii of the circles ]

Let the sides AB, BC, CD, ………… of a regular polygon touch the circle with the center at O and the number of sides of it be n. 

Let us draw the radii OP, OQ, OR,………………contacts.

∴ these are perpendiculars to AB, BC, CD,… etc. respectively.

Read and Learn More WBBSE Solutions For Class 9 Maths

Let the radius = r, 

∴ r = OP = OQ = OR = ………..

Now, the area of the polygon ABCD ………….

= ΔOAB + ΔOBC + ΔOCD + ………..

= \(\frac{1}{2} \mathrm{AB} \cdot \mathrm{OP}+\frac{1}{2} \mathrm{BC} \cdot \mathrm{OQ}+\frac{1}{2} \mathrm{CD} \cdot \mathrm{OR}+\cdots \cdots\)

= \(\frac{1}{2} \mathrm{AB} \cdot r+\frac{1}{2} \mathrm{BC} \cdot r+\frac{1}{2} \mathrm{CD} \cdot r+\cdots \cdots\)

= \(\frac{1}{2} r(\mathrm{AB}+\mathrm{BC}+\mathrm{CD}+\cdots \cdots)\)

= \(\frac{1}{2} r \times \text { (Perimeter of the polygon) }\)

Now, if the number of sides is a large number, i.e., if n tends to infinity, then AB, BC, CD,….. etc. reduces smaller and smaller and ultimately at the limiting position they coincide with the circumference of the circle.

∴ Area of the circle = \(\frac{1}{2} r \times(\text { circumference of the circle) }\)

= \(\frac{1}{2} r \times 2 \pi r=\pi r^2\)

∴ Area of a circle = πr² (r= radius). (Proved)

 

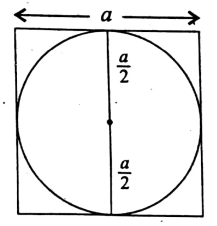
Chapter 3 Area Of Circles  Area Of Circles Inscribed In A Square

Area Of Circles Inscribed In A Square:-

If a circle is inscribed in a square, then the diameter of the circle is equal to the equal sides of the square.

∴ Radius of the circle = \(\frac{\text { side of the square }}{2}\)

Now, if the equal sides of the square be units, then the radius of the circle = \(\frac{a}{2}\) units, 

∴ Area of the circle = \(\pi\left(\frac{a}{2}\right)^2 \text { sq-units }=\frac{\pi a^2}{4} \text { sq-units }\)

 

 

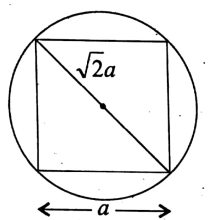
Chapter 3 Area Of Circles Area Of Circles Circumscribing A Square

Area Of Circles Circumscribing A Square:-

If a circle is circumscribed in a square, then the diameter of the circle is equal to the diagonal of the square.

Now, if the equal sides of the square be a unit, then its diagonal is √2a units.

∴ Diameter = √2a units

∴ Radius = \(\frac{\sqrt{ } 2 a}{2} \text { units }=\frac{a}{\sqrt{ } 2} \text { units. }\)

∴ Area of the circle = \(\pi \times\left(\frac{a}{\sqrt{ } 2}\right)^2 \text { sq-units }=\frac{\pi a^2}{2} \text { sq-units. }\)

 

 

Chapter 3 Area Of Circles Area Of Circles Circumscribing A Rectangle

Area Of Circles Circumscribing A Rectangle:-

If a circle is circumscribed in a rectangle, then the diameter of the circle is equal to the diagonal of the rectangle.

Now, if the length and breadth of the rectangle be a units and b units respectively,

then the diagonal of the rectangle is \(\sqrt{a^2+b^2}\) units.

∴ Diameter of the circle = \(\sqrt{a^2+b^2}\)

∴ Radius of the circle = \(\frac{\sqrt{a^2+b^2}}{2}\)

∴ Area of the circle = \(=\pi\left(\frac{\sqrt{a^2+b^2}}{2}\right)^2 \text { sq-units }=\frac{\pi}{4}\left(a^2+b^2\right) \text { sq-units }\)

 

 

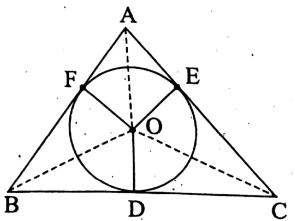
Chapter 3 Area Of Circles To Find The In-Radius Of A Triangle

The In-Radius Of A Triangle:-

Let O be the in-radius of the ΔABC.

Let us draw perpendiculars OD, OE, and OF to the sides BC, CA, and AB respectively.

Let the in-radius of ΔABC be r.

∴ OD = OE = OF = r units

Now, the area of ΔABC = area ofΔOBC + area of ΔOAC + area of ΔOAB.

⇒ \(\frac{1}{2} \times \mathrm{BC} \times \mathrm{OD}+\frac{1}{2} \times \mathrm{AC} \times \mathrm{OE}+\frac{1}{2} \times \mathrm{AB} \times \mathrm{OF}\)

= \(\frac{1}{2} \times \mathrm{BC} \times r+\frac{1}{2} \times \mathrm{AC} \times r+\frac{1}{2} \times \mathrm{AB} \times r\)

= \(\frac{1}{2}(\mathrm{BC}+\mathrm{AC}+\mathrm{AB}) \times r\)

∴ Area of the triangle = \(\frac{1}{2}\) x Perimeter of the triangle x in-radius

 

 

Chapter 3 Area Of Circles To Find The In Radius And Circum Radius Of An Equilateral Triangle

 

The In Radius And Circum Radius Of An Equilateral Triangle:-

Let the equal sides of the equilateral triangle be a unit.

∴ Height of the triangle = \(\frac{\sqrt{3}}{2} a \text { units }\)

Since, the height of an equilateral triangle is also a median of it, the median 

= \(\frac{\sqrt{3}}{2} a \text { units }\)

Now, the median is divided internally at the centroid of the triangle into the ratio 2: 1,

∴  in-radius = \(\frac{1}{3} \times\left(\frac{\sqrt{3}}{2} a\right) \text { units }=\frac{a}{2 \sqrt{3}} \text { units }\)

and circum-radius = \(\frac{2}{3} \times \frac{\sqrt{3} a}{2} \text { units }=\frac{a}{\sqrt{3}} \text { units }\)

If an arc of a circle of radius r units produces an angle e at the center, then the length of that arc = \(\frac{\theta}{360} \times \text { circumference of the circle }=\frac{2 \pi r \theta}{360} \text { units. }\)

and the area of the circle = \(\frac{\theta}{360} \times \text { area of the circle }=\frac{\pi r^2 \theta}{360} \text { sq-units }\)

 

Chapter 3 Area Of Circles Select The Correct Answer (MCQ)

 

Question 1. The area of a circle is x sq-units. If the circumference is y-units and the diameter be z-units, then the value of \(\frac{x}{yz}\)is

1. \(\frac{1}{2}\)
2. \(\frac{1}{4}\)
3. 1
4. \(\frac{1}{8}\)

Solution :

The area of the circle = \(\pi\left(\frac{z}{2}\right)^2 \text { sq-units }\)

and circumference = \(y=2 \pi\left(\frac{z}{2}\right) \text { units }\)

∴ \(\frac{x}{y z}=\frac{\frac{\pi z^2}{4}}{\pi z \cdot z}=\frac{1}{4}\)

 

 

Question 2. The ratio of the areas of two squares circumscribed and inscribed in a circle is.

1. 4:1
2. 1:4
3. 2:1
4. 1:2

Solution:

Let the radius of the circle = r units.

∴ The side of the circumscribed square = diameter of the circle = 2r units and the diagonal of the inscribed square = diameter of the circle = 2r units.

The side of the square inscribed in the circle= \(\frac{\text { diagonal }}{\sqrt{2}}=\frac{2 r}{\sqrt{2}} \text { units }=\sqrt{2} r \text { units }\)

The required ratio = (2r units)²: (√2r units)² = 4r² sq-units: 2r² sq-units = 2: 1.

 

Question 3. The numerical value of the circumference and area of a circle are equal. The length of the diagonal of the circumscribed circle is

1. 4 units
2. 2 units
3. 4√2 units
4. 2√2 units

Solution: 

Let the radius of the circle is r units, 

∴ \(2 \pi r=\pi r^2 \Rightarrow r=2\)

∴ The side of the square circumscribing the circle = the diameter = 2r units 2 x 2 units = 4 units

∴ Diagonal = side x √2 = 4√2 units.

 

Question 4. The ratio of the areas of the circles circumscribing and inscribed in an equilateral triangle is

1. 4:1
2. 1:4
3. 2:1
4. 1:2

Solution: 

The circum-radius: in-radius of the equilateral triangle = \(\frac{2}{3} \times \text { height : } \frac{1}{3} \times \text { height }\)

= 2: 1

The ratio of the areas is 2²: 1² = 4: 1.

 

Chapter 3 Area Of Circles Short Answer Type Questions

 

Question 1. If the radius of a circle is increased by 10%, then what percentage of its area will be increased?

Solution:

Given

The radius of a circle is increased by 10%

Let the initial radius of the circle be 100r units.

‍ initial area = \(\pi \times(100 r \text { units })^2=10000 \pi r^2 \text { sq-units }\)

Radius when increased by 10% = \(\left[100 r \times\left(1+\frac{10}{100}\right)\right] \text { units }=110 r \text { units }\)

∴ Area after increment = \(\left(12100 \pi r^2-10000 \pi r^2\right) \text { sq-units }=2100 \pi r^2 \text { sq-units }\)

∴ Increased area = \(\left(12100 \pi r^2-10000 \pi r^2\right) \text { sq-units }=2100 \pi r^2 \text { sq-units }\)

∴ Percentage of increment = \(\left(\frac{2100 \pi r^2}{10,000 \pi r^2} \times 100\right) \%=21 \%\)

 

Question 2. If the circumference of a circle is decreased by 50%, then what percentage of its area will be decreased?

Solution: 

Given

The circumference of a circle is decreased by 50%

Let the initial radius of the circle = r units.

∴ initial circumference = 2πr units and area = πr² units.

Now, circumference after discriminant = \(\frac{2πr}{2}\) = units = πr unit [ \(50 \%=\frac{50}{100}=\frac{1}{2}\) ]

∴ Then the radius = \(\frac{\text { circumference }}{2 \pi}=\frac{\pi r}{2 \pi} \text { units }=\frac{r}{2} \text { units }\)

∴ Area after discriminant = \(\pi \times\left(\frac{r}{2}\right)^2 \text { sq-units }=\frac{\pi r^2}{4} \text { sq-units }\)

∴ Decreased area = \(\left(\pi r^2-\frac{\pi r^2}{4}\right) \text { sq-units } ₹ \frac{3 \pi r^2}{4} \text { sq-units }\)

∴ Required percentage = \(\left(\frac{\frac{3 \pi r^2}{4}}{\pi r^2} \times 100\right) \%=\left(\frac{3 \pi r^2}{4} \times \frac{1}{\pi r^2} \times 100\right) \%=75 \%\)

 

Question 3. Jaya inscribed a circle in a square. This circle is also the circumcircle of an equilateral triangle, each of whose sides are 4√3 cm. Find the diagonal of the square.

Solution:

Given

Jaya inscribed a circle in a square.

This circle is also the circumcircle of an equilateral triangle, each of whose sides are 4√3 cm.

The side of the equilateral triangle is 4√3 cm

∴ Height = \(\frac{\sqrt{3}}{2} \times 4 \sqrt{3} \mathrm{~cm}=6 \mathrm{~cm} .\)

∴ Radius of the circumcircle= \(\frac{2}{3}\) x 6 cm = 4 cm.

∴ Diameter = 2 x 4 cm = 8 cm

Since the circle is inscribed in the square, the side of the square is equal to the diameter of the circle.

∴ Side of the square = 8 cm.

The diagonal of the square = 8√2 cm.

 

 

Question 4. Sumit cut a wire into two equal parts and bent one of them into a square and the other into a circle. If the area of the square be 33 sq-cm more than that of the circle, find the length of the wire.

Solution:

Given

Sumit cut a wire into two equal parts and bent one of them into a square and the other into a circle.

The area of the square be 33 sq-cm more than that of the circle.

Let the length of the wire be 2a cm.

∴ length of each part =\(\frac{2a}{2}\) cm = a cm 

Also, let the side of the square be x cm, 

∴ Perimeter = 4x cm.

∴ 4x = a ⇒ x = \(\frac{a}{4}\)

Again, let the radius of the circle be r cm. 

∴ Circumference = 2πr cm

∴ \(2 \pi r=a \Rightarrow r=\frac{a}{2 \pi}\)

As per the question, \(\frac{14 a^2-11 a^2}{176}=33 \Rightarrow 3 a^2=33 \times 176 \Rightarrow a^2=\frac{33 \times 176}{3}\)

⇒ \(\frac{14 a^2-11 a^2}{176}=33 \Rightarrow 3 a^2=33 \times 176 \Rightarrow a^2=\frac{33 \times 176}{3}\)

⇒ \(a=\sqrt{11 \times 11 \times 16}=11 \times 4=44\)

∴ The length of the wire = 2 x 44 cm = 88 cm.

 

Chapter 3 Area Of Circles Long Answer Type Questions

 

Question 1. Palas and Piyali have drawn two circles, the radius of which is 4: 5; then find the ratio of their areas.

Solution:

Given

Palas and Piyali have drawn two circles, the radius of which is 4: 5.

Let the radii of the circles be 4r units and 5r units respectively.

The ratio of the areas of the circles = \(\pi(4 r)^2: \pi(5 r)^2=16 \pi r^2: 25 \pi r^2=16: 25\)

The required ratio = 16: 25.

 

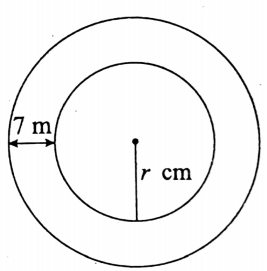
Question 2. There is a road of the breadth of 7 m of a circular park along its outer circumference. If the circumference of the circle is 352 m, then find the area of the road. To concrete the road at a rate of Rs. 20 per sq-m, what will be the required expenditure?

Solution:

Given

There is a road of the breadth of 7 m of a circular park along its outer circumference.

The circumference of the circle is 352 m.

To concrete the road at a rate of Rs. 20 per sq-m

Let the radius of the circular park be r m.

∴ its circumference = 2πr m

As per question, 2лr = 352 ⇒ \(2 \times \frac{22}{7} \times r=352\)

⇒ \(r=\frac{352 \times 7}{2 \times 22}=56\)

The radius of the park = 56 m.

∴ Including the road the radius of the park = (56+7) m = 63 m 

∴ Including the road the area of the circle = π x 63² sq-cm

Again, the area of the circle = π x 56²

∴ The area of the road only = \(\left(\pi \times 63^2-\pi \times 56^2\right) \mathrm{sq}-\mathrm{m}=\pi\left(63^2-56^2\right) \mathrm{sq}-\mathrm{m}\)

= π x (63 + 56) x (63 – 56) sqm

= \(\frac{22}{7} \times 119 \times 7 \mathrm{sq}-\mathrm{m}=2618 \mathrm{sq}-\mathrm{m}\)

Now the expenditure to concrete the road= is Rs. 20 x 2618 

= Rs. 52360.

∴ The required area is 2618 sqm and the required expenditure = is Rs. 52360.

 

 

Question 3. There is a road of equal breadth around the circular field of Bakultala. The outer circumference of the road is 132 m more than its inner circumference. If the area of the road is 14190 sqm, then find the area of the field.

Solution:

Given

There is a road of equal breadth around the circular field of Bakultala.

The outer circumference of the road is 132 m more than its inner circumference.

the area of the road is 14190 sqm,

Let the inner radius and outer radius of the circular road be \(r_1 \mathrm{~m} \text { and } r_2\) respectively.

∴ inner circumference = \(2 \pi r_1 \mathrm{~m} \text { and outer circumference }=2 \pi r_2 \mathrm{~m} \text {. }\)

As per the question, \(2 \pi r_2-2 \pi r_1=132 \Rightarrow 2 \times \frac{22}{7}\left(r_2-r_1\right)=132\)

\(r_2-r_1=\frac{132 \times 7}{2 \times 22} \Rightarrow\left(r_2-r_1\right)=21\) ……………(1)

Given that the area of the road = 14190 sqm

∴ \(\pi\left(r_2^2-r_1^2\right)=14190 \Rightarrow\left(r_2+r_1\right)\left(r_2-r_1\right)=14190 \times \frac{7}{22} \Rightarrow\left(r_2+r_1\right) \times 21=14190 \times \frac{7}{22}\) ………………(2)

Adding (1) + (2) we get, \(r_2-r_1+r_2+r_1=21+215 \Rightarrow 2 r_2=236 \Rightarrow r_2=118\)

\(r_1=215-118=97\)    ∴ the radius of the field = 97 m

∴ The area of the circle = \(\pi r^2 \mathrm{sq}-\mathrm{m}=\frac{22}{7} \times(97)^2 \mathrm{sq}-\mathrm{m}=\frac{206998}{7} \mathrm{sq}-\mathrm{m}=29571 \frac{1}{7} \mathrm{sq}-\mathrm{m} .\)

 

Question 4. The area of a circular field is 154 sq-cm. Find the perimeter of the square circumscribing the circle and also find the area of the square. If the square would be inscribed in the circle, then what would be the perimeter and area of the square?

Solution: 

Given

The area of a circular field is 154 sq-cm.

The square would be inscribed in the circle.

Let the radius of the field = r cm,

∴ Area of the field = r² sq-cm.

As per the question, \(\pi r^2=154 \Rightarrow \frac{22}{7} \times r^2=154 \Rightarrow r^2=\frac{154 \times 7}{22} \Rightarrow r^2=49 \Rightarrow r=7\)

The radius of the field = 7 cm.

∴ Diameter of the field 2r= 2 x 7 cm = 14 cm.

The side of the square circumscribing the circular field is equal to the diameter of the field. 

∴ The perimeter of the square = 4 x side = 4 x 14 cm = 56 cm.

∴ Area of the square = (side)² = (14)² sq-cm = 196 sq-cm.

Again, when the square is inscribed in the circular field, the diagonal of the square is equal to the diameter of the field.

∴ Now, if the side of the square be x cm, then \(\sqrt{2} x=14 \Rightarrow x=\frac{14}{\sqrt{2}}=7 \sqrt{2}\)

∴ Then the perimeter of the square = 4 x side = 4 x 7√2 cm = 28 √2 cm. 

and the area of it (side)²= (7√2)² sq-cm = 98 sq-cm

The perimeter and area of the square 28 √2 cm and 98 sq-cm.

 

Question 5. Lina bought a bracelet from a fair. There is 269-5 sq-cm metal in the bracelet. If the outer diameter of the bracelet is 28 cm, find its inner radius.

Solution:

Given 

Lina bought a bracelet from a fair. There is 269-5 sq-cm metal in the bracelet.

The outer diameter of the bracelet is 28 cm.

Let the inner radius be r cm

Given that the outer-diameter = 28 cm, 

∴ outer-radius = 14 cm

There is 269.5 sq-cm metal in the bracelet.

∴ π (14² – r²) = 269.5

(196 – r²) = 269.5 x \(\frac{7}{22}\)

∴ Inner-radius = 2 x \(\frac{21}{2}\) cm = 21 cm.

 

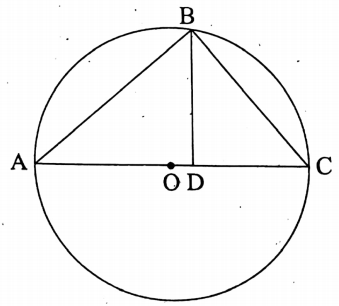
Question 6. The radius of the circumcircle of a right-angled triangle is 5 cm and the perpendicular distance of the hypotenuse from its opposite vertex is 4 cm. Find the area of the triangle. 

Solution: 

Given 

The radius of the circumcircle of a right-angled triangle is 5 cm and the perpendicular distance of the hypotenuse from its opposite vertex is 4 cm.

We know that the circumcentre of the circumcircle of a right-angled triangle is the midpoint of the hypotenuse and its circum-radius is half of the hypotenuse.

In the ∠B is the right angle of the ΔABC.

The midpoint of the hypotenuse AC is O and BD is the perpendicular drawn from B to AC.

Now, AC = 2 x OA = 2 x 5 cm = 10 cm and BD = 4 cm.

∴ Area of ΔABC = \(\frac{1}{2}\) × AC x BD sq-cm

 = \(\frac{1}{2}\) x 10 x 4 sq-cm 20 sq-cm

∴ The required area = 20 sq-cm.

 

 

Question 7. In the given figure, OPQR is a rhombus three of whose vertices are on the circle with the center at O. If the area of the rhombus is 32√3 sq-cm, find the radius of the circle.

Solution:

Given

OPQR is a rhombus three of whose vertices are on the circle with the center at O.

The area of the rhombus is 32√3 sq-cm.

Let the radius of the circle = r cm.

OPQR is a rhombus,

∴ OP = PQ = QR = RO

∴ OP = OR radius.

OP = OQ = PQ = r cm 

∴ ΔOPQ is an equilateral triangle.

Now area of the rhombus = 2 x (area of ΔOPQ)

= \(2 \times \frac{\sqrt{3}}{4} \times r^2 \mathrm{sq}-\mathrm{cm}=\frac{\sqrt{3}}{2} r^2 \mathrm{sq}-\mathrm{cm}\)

As per the question, \(\frac{\sqrt{3}}{2} r^2=32 \sqrt{3} \Rightarrow r^2=64 \Rightarrow r=8\)

∴ The required radius = 8 cm.

 

 

Question 8. The circle inscribed in an equilateral triangle of sides 24 cm just touches the sides of the triangle. Find the area of the rest part of the triangle (let √3 = 1-732)

Solution: 

Given 

The circle inscribed in an equilateral triangle of sides 24 cm just touches the sides of the triangle.

The ΔABC is an equilateral triangle, each side of which is 24 cm. 

AD is the perpendicular drawn from A to BC. 

Since the triangle is equilateral,

∴ D bisects BC.

∴ BD = CD = \(\frac{24}{2}\) cm = 12 cm. 

The incentre and centroid of the ΔABC are the same points O.

∴ OD = \(\frac{1}{3}\) AD…………(1)

Height of the triangle ABC= AD = \(\mathrm{ABC}=\mathrm{AD}=\frac{\sqrt{3}}{2} \times 24 \mathrm{~cm}=12 \sqrt{3} \mathrm{~cm}\)

∴ from (1) we get, OD = \(\mathrm{OD}=\frac{1}{3} \times \mathrm{AD}=\frac{1}{3} \times 12 \sqrt{3} \mathrm{~cm}=4 \sqrt{3} \mathrm{~cm}\)

∴ Area of the circle = \(\pi \times(\mathrm{OD})^2=\left\{\frac{22}{7} \times(4 \sqrt{3})^2\right\} \mathrm{sq}-\mathrm{cm}\)

= \(\left(\frac{22}{7} \times 48\right) \mathrm{sq}-\mathrm{cm}=150 \cdot 86 \mathrm{sq}-\mathrm{cm}\)

Area of the ΔABC = \(\frac{\sqrt{3}}{4} \times(\text { side })^2=\frac{\sqrt{3}}{4} \times(24)^2 \mathrm{sq}-\mathrm{cm}=144 \sqrt{3} \mathrm{sq}-\mathrm{cm}\)

= 144 x 1.732 sq. cm

= 249.40 sq. cm

∴ Area of the ΔABC = (249.40 – 150.86) sq. cm

= 98.54 sq. cm

 

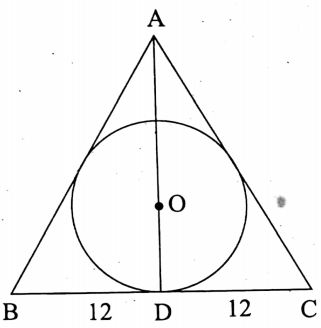
The area of the rest part of the triangle = 98.54 sq. cm

 

 

Question 9. ABCD is a square of side 12 cm. Four arcs, each of radius 6 cm have been drawn with centers at A, B, C, and D respectively, and in the remaining part, some sketches are also drawn. Find the perimeter and area of the sketched region.

Solution: 

Given

ABCD is a square of side 12 cm.

Four arcs, each of radius 6 cm have been drawn with centers at A, B, C, and D respectively, and in the remaining part, some sketches are also drawn.

Let the four circles drawn with centers A, B, C, and D respectively, and a radius of 6 cm intersect each other at P, Q, R, and S.

We have to find the perimeter and area of the curved region PQRS

Now, area of the square ABCD = (12)² sq-cm = 144 sq.cm

 

 

 

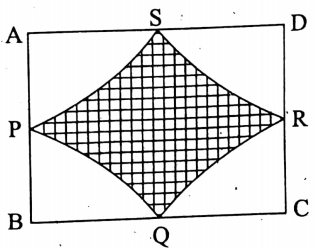
Each of the arcs PS, PQ, QR, and RS have produced an angle of 90° at each center. 

Since each of the radii of the circles is equal. 

∴ arc PS arc PQ = arc QR arc arc RS

= \(\frac{\text { angle produced at the centre }}{360^{\circ}} \times(\text { circumference of circle })\)

= \(\left(\frac{90^{\circ}}{360^{\circ}} \times 2 \pi \times 6\right) \mathrm{cm}=3 \pi \mathrm{cm}=3 \times \frac{22}{7} \mathrm{~cm}=\frac{66}{7} \mathrm{~cm}\)

∴ Perimeter of the curved region 

PQRS = 4 x arc Ps = (4 x \(\frac{66}{7}\)) cm

= 37 \(\frac{5}{7}\)

Again, the region APS = region BPQ = region CQR = region DRS

= \(\frac{\text { angle produced at the centre }}{360^{\circ}} \times \text { area of the circle }\)

= \(\left[\frac{90^{\circ}}{360^{\circ}} \times \pi \times(6)^2\right] \mathrm{sq}-\mathrm{cm}=\frac{198}{7} \mathrm{sq}-\mathrm{cm}\)

∴ Area of the 4 regions = \(\left(4 \times \frac{198}{7}\right) \mathrm{sq}-\mathrm{cm}=\frac{792}{7} \mathrm{sq}-\mathrm{cm}\)

Area of the curved region PQRS = (Area of the square) (Area of 4 regions)

= \(\left(144-\frac{792}{7}\right) \mathrm{sq}-\mathrm{cm}=30 \frac{6}{7} \mathrm{sq}-\mathrm{cm}\)

 

 

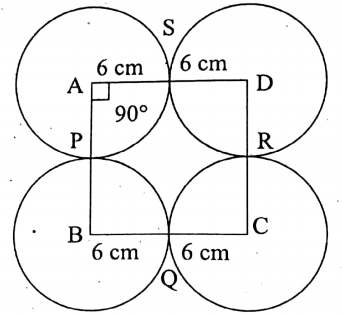
Alternative Method:

 The radius of each of the circles with centers at A, B, C, and D is 6 cm and the length of the arc cut by each of the four circles is part \(\frac{1}{4}\) of the circumference of the circles, and the area of each part is also \(\frac{1}{4}\) part of the area of the circle.

∴ The required perimeter = \(4 \times \frac{1}{4} \times 2 \times \frac{22}{7} \times 6 \mathrm{~cm}=37 \frac{5}{7} \mathrm{~cm}\)

and required area = \(\left\{(6+6)^2-4 \times \frac{1}{4} \times \pi \times 6^2\right\} \mathrm{sq}-\mathrm{cm}=30 \frac{6}{7} \mathrm{sq}-\mathrm{cm}\)

 

Question 10. In the given find the area of the lined region.

Solution

Area of each circle = \(\pi \times(r)^2[r=\text { radius }]=\left[\frac{22}{7} \times(3 \cdot 5)^2\right] \mathrm{sq}-\mathrm{cm}=38 \cdot 5 \mathrm{sq}-\mathrm{cm}\)

The arc PS has produced an angle of 90° at the center A.   [ABCD is a square]

∴ Area of the region APS

= \(=\frac{\text { angle produced at the centre }}{360^{\circ}} \times \text { area of circle }\)

= \(\left[\frac{90^{\circ}}{360^{\circ}} \times 38 \cdot 5\right] \mathrm{sq}-\mathrm{cm}=9.625 \mathrm{sq}-\mathrm{cm}\)

∴ The area of the lined region of the circle with the center at A

= (38.5 – 9.625) sq-cm 

= 28.875 sq-cm 

∴ The required area = (4 x 28.875) sq-cm

= 115.5 sq-cm

 

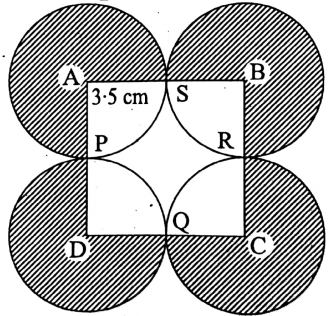
The area of the lined region = 115.5 sq-cm

 

Alternative Method:

The radius of each of the circles with centers at A, B, C, and D is 3.5 cm.

Now in the lined region, \(\frac{1}{4}\) part of the area of each of the circles must be subtracted and \(\frac{3}{4}\) part of the area of each of the circles must be added.

The area of the lined region = \(4 \times \frac{3}{4} \times \frac{22}{7} \times(3 \cdot 5)^2 \mathrm{sq}-\mathrm{cm}=115 \cdot 5 \mathrm{sq}-\mathrm{cm}\)

 

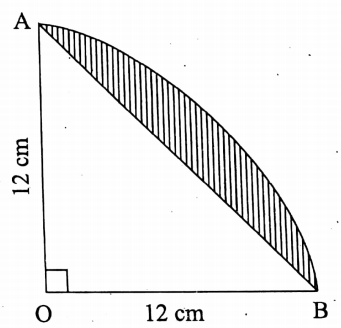
Question 11. In the given find the area and perimeter of the shaded region. 

Solution:

The perimeter of the shaded region = line segment AB + arc AB.

= \(\sqrt{\mathrm{OA}^2+\mathrm{OB}^2}\left [ \angle \mathrm{AOB}=90^{\circ}\right]+\frac{1}{4} \times 2 \times \frac{22}{7} \times 12 \mathrm{~cm}\)(radius 12 cm)

= \(\sqrt{(12)^2+(12)^2} \mathrm{~cm}+\frac{132}{7} \mathrm{~cm}\)

= \(\left(12 \sqrt{2}+\frac{132}{7}\right) \mathrm{cm}\)

= 35.83 cm

∴ Area of the shaded region

= \(\left\{\frac{1}{4} \times \frac{22}{7} \times(12)^2-\frac{1}{2} \times 12 \times 12\right\} \text { sq-cm }\)

= \(\frac{288}{7} \mathrm{sq}-\mathrm{cm}=41 \frac{1}{7} \mathrm{sq}-\mathrm{cm} .\)

 

 

Question 12. Protul has drawn an equilateral triangle ABC the side of which is 10 cm. Sumita has drawn three arcs each of length 5 cm with centers at A, B, and C, and have colored some region in the middle position. Find the area of the colored region. (Take √3 = 1.732)

Solution:

Given

Protul has drawn an equilateral triangle ABC the side of which is 10 cm.

Sumita has drawn three arcs each of length 5 cm with centers at A, B, and C, and have colored some region in the middle position.

The area of the equilateral ΔABC = \(\frac{\sqrt{3}}{4} \times(\text { side })^2=\left[\frac{\sqrt{3}}{4} \times(10)^2\right] \text { sq-cm }\)

= \(25 \sqrt{3} \mathrm{sq}-\mathrm{cm}=25 \times 1.732 \mathrm{sq}-\mathrm{cm}\)

= 43.3 sq-cm (approx.)

Since the arc PR has produced an angle of 60°. at A,

∴ Area of the region APR = \(=\frac{\text { angle produced at the centre }}{360^{\circ}}\) x (area of the circle)

= \(\left[\frac{60^{\circ}}{360^{\circ}} \times \pi \times(5)^2\right] \mathrm{sq}-\mathrm{cm}\)

= \(\left[\frac{1}{6} \times \frac{22}{7} \times 25\right] \mathrm{sq}-\mathrm{cm}=\frac{275}{21} \mathrm{sq}-\mathrm{cm}\)

Similarly, the areas of the regions BPQ and CQR are \(\frac{275}{21}\)sq-cm.

. The area of the colored region= (area of the triangle) – (area of the three equal regions)

= \(\left(43 \cdot 3-3 \times \frac{275}{21}\right) \mathrm{sq}-\mathrm{cm}=(43 \cdot 3-39 \cdot 29) \mathrm{sq}-\mathrm{cm}\)

= 4.01 sq-cm (approx.)

 

 

Question 13. Rabeya has drawn an equilateral triangle of side 21 cm on a large piece of paper. Find the area of the incircle of the triangle.

Solution:

Given

Rabeya has drawn an equilateral triangle of side 21 cm on a large piece of paper.

The side of the equilateral triangle is 21 cm.

∴ height = \(\frac{\sqrt{3}}{2} \times 21 \mathrm{~cm}=\frac{21}{2} \sqrt{3} \mathrm{~cm}\)

The median of the equilateral triangle = its height.

Now, inradius of the incircle = \(\frac{1}{3}\) x median

= \(\frac{1}{3} \times \frac{21 \sqrt{3}}{2} \mathrm{~cm}=\frac{7 \sqrt{3}}{2} \mathrm{~cm}\)

∴ Area of the incircle = \(\frac{22}{7} \times\left(\frac{7 \sqrt{3}}{2}\right)^2 \mathrm{sq}-\mathrm{cm}\)

= \(\frac{22}{7} \times \frac{7 \times \sqrt{3}}{2} \times \frac{7 \times \sqrt{3}}{2} \mathrm{sq}-\mathrm{cm}\)

= 115.5 sq. cm

The area of the incircle of the triangle = 115.5 sq. cm

 

Question 14. The perimeter of a triangle is 32 cm and the area of its incircle is 38-5 sq-cm. Find the area of the triangle.

Solution:

Given 

The perimeter of a triangle is 32 cm and the area of its incircle is 38-5 sq-cm.

Let the radius of the incircle be r cm.

∴ Area of the incle = πr² sq. cm

∴ As per the question, \(\pi r^2=\frac{385}{10} \Rightarrow \frac{22}{7} r^2=\frac{385}{10} \Rightarrow r^2=\frac{385 \times 7}{10 \times 22}=\frac{49}{4} \Rightarrow r=\frac{7}{2}=3 \cdot 5\)

∴ The radius of the incircle = 3.5 cm.

Now, area of the triangle = \(\frac{1}{2} \times \text { perimeter } \times \text { radius }=\frac{1}{2} \times 32 \times 3.5 \mathrm{sq}-\mathrm{cm}=56 \mathrm{sq}-\mathrm{cm}\)

 

Question 15. Find the radii of the incircle and circumcircle of a triangle of sides 20 cm, 15 cm and 25 cm. Also, find their areas.

Solution:

Given

A triangle of sides 20 cm, 15 cm, and 25 cm.

 20² + 15² = 400+ 225 = 625 = 25²

∴ The given triangle is a right-angled triangle, the hypotenuse of which is 25 cm.

Now, the radius of the circumcircle = \(\frac{\text { hypotenuse }}{2}=\frac{25}{2} \mathrm{~cm}=12 \frac{1}{2} \mathrm{~cm}\)

∴ Area of the circumcircle = \(\frac{22}{7} \times\left(\frac{25}{2}\right)^2 \mathrm{sq}-\mathrm{cm}=\frac{22}{7} \times \frac{625}{4} \mathrm{sq}-\mathrm{cm}\)

Again, area of the triangle = \(\frac{6875}{14} \mathrm{sq}-\mathrm{cm}=491 \frac{1}{14} \mathrm{sq}-\mathrm{cm}\)

Let the radius of the incircle be r cm,

∴ \(\frac{1}{2}\) perimeter of the triangle x r = 150

\(\frac{1}{2} \times(15+20+25) \times r=150 \Rightarrow \frac{1}{2} \times 60 \times r=150 \Rightarrow r^{\prime}=\frac{150}{30}=5\)

∴ The radius of the incircle = 5 cm

∴ Area of the incircle = \(\frac{22}{7} \times 5^2 \mathrm{sq}-\mathrm{cm}=\frac{550}{7} \mathrm{sq}-\mathrm{cm}=78 \frac{4}{7} \mathrm{sq}-\mathrm{cm}\)