WBBSE Solutions For Class 9 Maths Algebra Chapter 1 Polynomials

Algebra Chapter 1 Polynomials

Algebra Chapter 1 Polynomials:

Definition Of A Polynomial :

If x is variable, n is a whole number, and a₀, a₁ a₂, and a_n (a_n ≠ 0) be all constant real numbers, then the expression.

\(a_n x^n+a_{n-1} x^{n-1}+a_{n-2} x^{n-2}+\cdots \cdots+a_1 x+a_0\) is called a polynomial of the variable x.

Similarly, if x, and y be two variables, and n be a whole number, then the expression

\({ }^n \mathrm{C}_0 x^n+{ }^n \mathrm{C}_1 x^{n-1} y+{ }^n \mathrm{C}_2 x^{n-2} y^2+\cdots \cdots+{ }^n \mathrm{C}_{n-1} x y^{n-1}+{ }^n \mathrm{C}_n y^n\) is called a polynomial of the variables x and y.

For example, each of the expressions 2, 10, 6x, 8x + 1, x² + 4x + 2, x³ + 3x + 2x + 2, x⁴ + 3x³ + 2x² + 8x + 4, etc are polynomials.

Because the index of the variables of each term of these expressions is a whole number.

Similarly, x + y + 2xy, x + y + z + 3xyz, etc are also polynomials, because here also the indices of variables of each term are whole numbers.

Read and Learn More WBBSE Solutions For Class 9 Maths

Algebra Chapter 1 Term Of Polynomials

Let p (x)= \(a_n x^n+a_{n-1} x^{n-1}+\cdots \cdots \cdots+a_1 x+a_0 \quad\left(a_n \neq 0\right)\) be a polynomial. 

Here the expressions \(a_n x^n, a_{n-1} x^{n-1}, \cdots \cdots \cdots a_1 x, a_0\) are called terms of the polynomial. Clearly, there are (n + 1) number of

terms in this polynomial, of which

the first term = \(a_n x^n\)

second term = \(a_{n-1} x^{n-1}\)

………………………………

……………………………..

(n + 1)-th term = a0.

For example, there are 4, terms in the polynomial x³+3x²+2x+ √2 which are x³, 3x², 2x, and 2.

Similarly, the polynomial

4 consists of only one term 4,

x + 1 consists of two terms x and 1,

x²+√2x+√3 consists of three terms x², √2x and √3.

Also, x + y + z consists of 3 terms,

x+y+z+2xyz consists of 4 terms, etc.

Algebra Chapter 1 Coefficients Of Polynomials

Let p (x) = \(a_n x^n, a_{n-1} x^{n-1}, \cdots \cdots \cdots a_1 x, a_0\)

The terms of this polynomial are

\(a_n x^n, a_{n-1} x^{n-1}, \cdots \cdots \cdots a_1 x, a_0\)

Each of these terms contains variables (with their indices).

Such as, in the term \(a_n x^n\), the variable is xⁿ (with index n)

in the term \(a_{n-1} x^{n-1}\), the variable is x^n-1 (with index n – 1)

…………………………………………………………………………………………………………………………………………

…………………………………………………………………………………………………………………………………………

in the term ax, the variable is x (with index 1)

in the term, a the variable is x° (with index 0).

Now, the constant part except this variable part of each term of the polynomial is called the coefficient of that term.

For examples,

The coefficient of the term x° of the polynomial 10=10, since 10 = 10x⁰

or, 10 = 10.y°

or, …………..etc.

Similarly, the coefficient of the term x in \(\left(x+\frac{1}{2}\right)\) = 1. and of x° = \(\frac{1}{2}\)

the coefficient of x² of (x²+√3x+√7)-1, of x=√3 and of x-√7.

Also, the co-efficient of the term 2xy in (x²+y+z+ 2xy +2yz + zx) = 2, of x² = 1,….. etc. 

Again, in the expansion of (x + 2)², the co-efficient

of x° = 4

of x¹ = 4

of x² = 1, since (x + 2)² = x² + 4x + 4.

In the expansion of (x + 3)³, the co-efficient

of x³ = 1,

of x² = 9,

of x¹ = 27, and

of x° = 27, since (x + 3)³ = x³ + 9X² + 27 x + 27.

Algebra Chapter 1 Degree Of Polynomials

The highest power of the variable, (if there is only one variable) present in any term of a polynomial or the highest sum of the powers of the variables (if there is more than one variable), present in any term of a polynomial is called the degree of the polynomial.

For examples,

The highest power of x of the polynomial q (x) = x + 1 is 2. The degree of q (x) is 2.

The highest power of x of the polynomial g(x) = x⁴+3x³+2x²+x + √7 is 4, and

∴ The degree of g (x) is 4.

Similarly, the highest sum of the powers of the variables of the term 3xy of the polynomial f (x, y) = x + y + 3xy is 2. (Power of x = 1, power of y = 1, the power of xy = 1 + 1 = 2), the degee of f (x, y) is 2.

Again, the highest sum of the powers of the variables of the term 3xyz of the polynomial/ (x, y, z) = x + y + z + 3xyz is 3 (power of x = 1, power of v = 1, power of z = 1, power of xyz = 1 + 1 + = 3),

∴ The degree of f (x, y, z) is 3.

Thus, the degree of the polynomial

p(x) = a_nxⁿ +a_n-1x^n-1 + +a₁x + a₀ is n, since highest power of x is n.

Types of Polynomials:

Polynomials can be classified into two different types

1. on the basis of the number of terms:

In any polynomial, there exists one or more than one term. On the basis of these terms, the polynomials are called

1 Monomial

2 Binomials

3 Trinomials

4 Tetranomials

………………

n Polynomials.

1. Monomials:

The polynomials which have only one term are called monomials. Such as–

 \(q(x)=6=6 x^0, g(x)=3 x, f(x)=10 x, h(x)=x^2\) ,….. etc.

 

2. Binomials:

The polynomials consisting of only two terms are called binomials. Such as-

1 + x, x² + 1, y² + 2, x³ + 1,….. etc.

 

3. Trinomials:

The polynomials having three terms are called trinomials. Such as- 

x² + x + 1, y² + 2y²+1, z²+2x+√3,……… etc.

 

4. Tetranomials :

The polynomials having four terms are called trinomials. Such as—

x³ + 3x² + x + √5, y³ + 2y² + 4y + 1,.….. etc.

 

2. On the basis of degree: Polynomials can be classified on the basis of their degrees as follow:

1. Constant Polynomials

2. Linear Polynomials

3. Quadratic Polynomials

4. Cubic Polynomials

5. Biquadratic Polynomials

………………………………

n. n-Polynomials etc.

 

1. Constant Polynomials :

Polynomials of degree 0 are called constant polynomials. Such as-

-3, -1, 0, 2, 4, 6, 8, √2, √3,……… etc.

 

2. Linear Polynomials :

The polynomials which have the degree 1, are called linear polynomials. Such as- 2x+1, 3y, 5z+2, 6u+ √2,……… etc.

The standard form of linear Polynomials:

The standard form of linear polynomials is ax + b where a 0, a, and b are constants and x variable.

For examples, x + 1, here a = 1, b = 1,

4x + 3, here a= 4, b = 3,

10y+2, here a= 10, b = 2,…. etc.

The graph of any linear polynomial is always a straight line. To prove this, let us draw the graph of the linear polynomial f (x) = x + 4.

Let f (x)=y,

y = x+4 = the following chart:

 

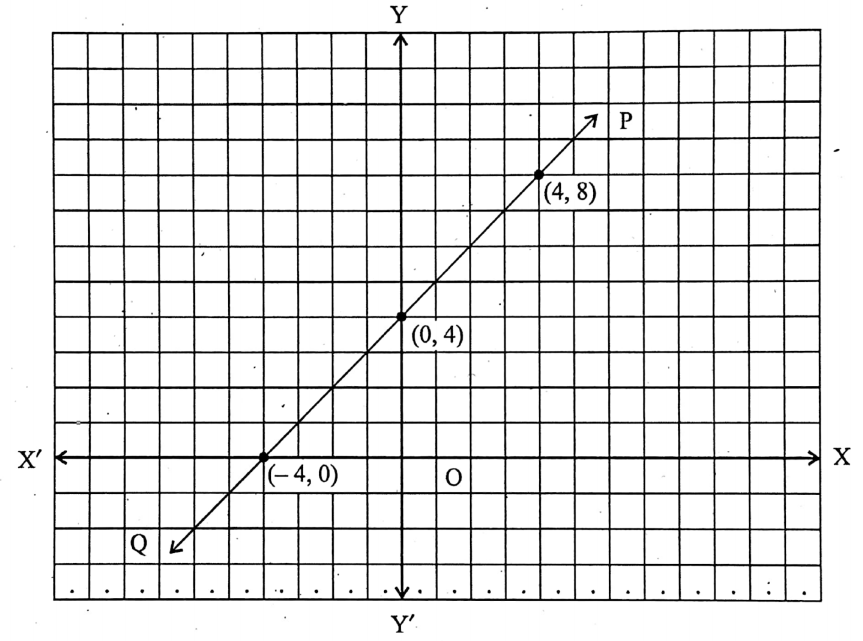
Clearly, we see from the above graph that, the graph of the linear polynomial f (x) = x + 4 is a straight-line PQ.

Similarly, we can show that the graph of any linear polynomial is a straight line.

If the linear polynomial is of the form of f (x)= mx, where m is a constant, or, of the form of y = mx (y = f (x)), then the straight line always passes through the origin.

Thus, the graph of ƒ (x) = 2x is a straight line passing through the origin (Draw the graph yourself).

 

3. Quadratic Polynomials:

The polynomials of degree 2 are called quadratic polynomials. Such as

x²+1, 2x² −3x+2, y² + √2y + √7,…….. etc.

Standard form :

The standard form of quadratic polynomials is ax2+ bx + c, a 0, a, b, c are constants.

For examples x²+ 4x + 4, here a = 1, b = 4, c=4

2y²+ 3y+1, here a = 2, b = 3, c = 1.

3z² + 2 = 3z² + 0z + 2, here a= 3, b = 0, c = 2.

 

4. Cubic Polynomials:

The polynomials of degree 3 are called cubic polynomials. Such as

x³+1, x³ – 1, y³ + 3y² + 3y + 1, ……………etc.

Standard form:

The standard form of cubic polynomials is ax³ + bx² + cx+d, where a 0 and a, b, c, d are constants. 

For examples, x³ + x² + 2x + 1; here a = 1, b = 1, c = 2, d = 1.

y³ + 2y² – y + √2; here a = 1, b = 2, c=-1, d= √2

If a = 0 of any cubic polynomial, then it reduces to a quadratic polynomial.

5. Biquadratic Polynomials:

Polynomials having degree 4 are called biquadratic polynomials. Such as-

√2x² +1, x² +3x² +2, y⁴ + √7y³+ y + 1, ….. etc.

Standard form :

The standard form of biquadratic polynomials is ax⁴+ bx³  + cx² + dx + e, where a 0 and a, b, c, d, e are constants.

For examples,

x²  + x³ + 2x² + 3x + 1; here a = 1, b = 1, c = 2, d = 3, e = 1.

y⁴+2y³+3y-y+√2, here a = 1, b = 2, c = 3, d = –1, e = √2

If a = 0 of any biquadratic polynomial, then it reduces to a cubic polynomial.

 

6. n-Polynomials:

Polynomials of degree n are called n-polynomials. Such as—

\(x^n+1, y^n-1, u^n+u^{n-1}+1\) + 1,……… etc.

 

Standard form :

The standard form of n-polynomials is p (x) =\(a_n x^n+a_{n-1} x^{n-1}+a_{n-2} x^{n-2}+\cdots \cdots \cdots+a_1 x+a_0\), where \(a_n \neq 0, a_0, a_1, a_2, \cdots \cdots \cdots \cdots \cdots, a_{n-2}, a_{n-1}, a_n\), are constants and n is a whole number.

 

Zero Polynomials:

If p(x) = \(a_n x^n+a_{n-1} x^{n-1}+a_{n-2} x^{n-2}+\cdots \cdots \cdots+a_1 x+a_0=0\)

\(a_0=a_1=a_2=\cdots \cdots \cdots \cdots \cdots=a_{n-1}=a_n=0\), then p (x) is called a zero polynomial.

It has already been noticed before that the degree of zero polynomials is undefined.

Properties of Polynomials :

1. The sum of two polynomials is a polynomial.

For example, let f (x) = x² + 1 and g (y) = y + √2 be any two polynomials.

Then f(x)+g (y) = x²+1+y+ √2 = x²+y+1+√2, which is also a polynomial.

 

2. The difference between two polynomials is a polynomial.

For examples, let f (x) = x² + 2x + 3 and g (x) = x² – x – 1.

∴ f(x) – g (x) = x²+2x+3-x²+x+1=3x+4, which is a polynomial.

g(x) − f(x) = x² – x – 1 – x² – 2x -3 = -3 x -4, which is also a polynomial.

 

3. The product of two polynomials is a polynomial.

For example, let f (x) = x + 1 and g (x) = x²+x+1.

∴ f(x) . g(x) = (x+1)(x² + x + 1) = x² + x² + x + x²+x+1

= x³ + 2x² + 2x +1, which is a polynomial.

 

4. The division between two polynomials may or may not be a polynomial. For example, let f(x) = x³ and g(x) = x.

Now, \(\frac{f(x)}{g(x)}=\frac{x^3}{x}=x^2\) = x² , which is a polynomial.

But, \(\frac{g(x)}{f(x)}=\frac{x}{x^3}=\frac{1}{x^2}=x^{-2}\) is not a polynomial, since here the index of x is (-2), which is not a whole number.

 

Algebra Chapter 1 Polynomials Select The Correct Answer (MCQ)

Examples 1.

1. Which one of the following is not a polynomial?

1. 0
2. \(\frac{x+\frac{1}{x}}{\frac{1}{x}}\)
3. – a (a = constant)
4. √t, t = variable

Answer:

4. is correct since √t = t^1/2 and \(\frac{1}{2}\)is not a whole number.

 

2. Which one of the following is a polynomial of degree 0?

1. 0
2. – k² (k = constant)
3. x (x = variable)
4. √2t,(t = variable)

Answer:

2. is correct, since – k²= – k.x⁰, i.e. the highest power of the variable is 0.

 

3. Which one of the following is a linear polynomial of one variable?

1. √5x
2. 2 – x – x²
3. x – y + xy
4. xⁿ⁺² (n = constant)

Answer:

1. is correct, since √5x is of one variable (x) and the highest power of x is 1 is a constant. 

∴ √5x is a linear polynomial of one variable.

 

4. The degree to which one of the following polynomials is undefined?

1. 0
2. – 1
3. + 1 (= variable)
4. kt (k = constant)

Answer:

1. is correct since 0 = 0. x⁰ = 0.x¹ = 0.x²……… etc, i..e., the highest power of the variable is not fixed or is not possible to determine.

∴ the degree of the polynomial 0 is undefined.

 

5. Which one of the following is a quadratic polynomial of one variable?

1. x + y – xy
2. x+1 (n= least natural number)
3. a² (a = constant)
4. 2t (variable)

Answer:

2. is correct, since n = 1 ( least natural number = 1), 

∴ \(x^{n+1}=x^{1+1}=x^2\) i.e., the degree of the polynomial x² is 2.

 

6. Which one of the following is a quadratic polynomial of two variables?

1. x + y + xy
2. x² + x + 1
3. 2-y-y²
4. ax² + bx + c (a + 0)

Answer:

1. is correct, since there are two variables x and y in this expression, and the power of

x + y =1+1 = 2.

 

7. The co-efficient of x2 of the polynomial &x 19 is

1. 0
2. 8
3. 19
4. None of these

Answer:

1. is correct, since there is no term containing.

∴ The co-efficient of x² is 0.

 

8. The co-efficient of x of the polynomial √11 – 3√11x + x² is

1. no co-efficient
2. 0
3. √11
4. None of these

Answer:

3. is correct, since, we know that x⁰ = 1.

∴ The term containing xo is a constant term, i..e, √11.

 

9. The binomials of one variable having degree 17 are

1. x¹⁷+ y
2. y¹⁷ + 1
3. x¹⁷ +y¹⁷
4. y – x¹⁷

Solution:

2. Is correct, since it is a polynomial of one variable (y) and the highest power of the variable is 17, i.e., the degree of the polynomial is 17.

 

10. x + \(\frac{5}{x}\) is

1. A polynomial of one variable
2. A linear polynomial of one variable
3. A quadratic polynomial of one variable
4. Not a polynomial

Solution:

2. Is correct, since \(x+\frac{5}{x}=x+5 x^{-1}\) and the power of x^-1 is (-1), which is not a whole number.

 

Algebra Chapter 1 Polynomials Short Answer Type Questions (SAQ)

Question 1.

1. Find the degrees of the polynomials

1. 5t-√7

Solution:

The variable of the polynomial 5t-√7 is t, the highest power of which is 1.

∴ The degree of the polynomial is 1.

 

2. 3

Solution: 

3 is a constant term, i.c., the power of the variable (whatever it may be) is 0. (3 = 3. X0 or 3.y0 or ……… etc.)

∴ The degree of the polynomial is 0.

 

2. What are the coefficients of x² of the two given polynomials?

1. \(\frac{\pi}{2} x^2+x\)

Solution:

The term of the given polynomial containing 2 is \(\frac{\pi}{2} x^2\)

Hence, the coefficient is \(\frac{π}{2}\)

 

2. √2x-1

Solution:

There is no term containing x² in the given polynomial (√2x-1).

Hence, the co-efficient of x² is 0.

 

3. Given one example of a binomial of degree 35 and a monomial of degree 100 each. 

Solution:

A binomial of degree 35 is x³⁵⁺¹ and a monomial of degree 100 is y¹⁰⁰

 

4. What do you mean by linear polynomials? Give an example.

Solution:

Linear Polynomials:-

The polynomials of degree 1, i.e., the polynomials in which the highest power of the variable (or variables) is 1, are called linear polynomials.

Example: (x + 1) is a linear polynomial.

 

Algebra Chapter 1 Polynomials Long Answer Type Questions

 

Question 1. Write which of the following algebraic expressions are polynomials and also state the degree of those which are polynomials :

1. 4x²-3x+√7

Solution:

4x²-3x+√7 is a polynomial since all the powers (2, 1, 0) of the variable x are whole numbers.

The degree of the polynomial is 2 since the highest power of the variable x is 2.

 

2. y²+ √2

Solution:

The algebraic expression (y²+√2) is a polynomial since all the indices (2, 0) of the variable y are whole numbers.

The degree of this polynomial is 2 since the highest power of the variable y is 2.

 

3. y³ – \(\frac{3}{4}\)y + √7

Solution:

(y³ – \(\frac{3}{4}\) y + √7) is a polynomial, since all the powers (3, 1, 0) of the variable y are whole numbers. The degree of this polynomial is 3 since the highest power of the variable y is 3.

 

4. \(\frac{1}{x}\) – x² + 2x + √3

Solution:

(\(\frac{1}{x}\) − x² +2x+ √3) is not a polynomial, since the power of the variable x of the term \(\frac{1}{x}\) = x (-)1 is (1), which is not a whole number.

5. 2x⁶– 4x⁵ +7×2 + 3

Solution:

(2x⁶ – 4x + 7x² +  3) is a polynomial, since all the powers (6, 5, 2, 0) of the variable x are whole numbers.

The degree of this polynomial is 6 since the highest power of the variable x is 6.

 

6. x² + 2x^-1 + 4

Solution:

(x^-2 + 2x^-1 + 4) is not a polynomial, since the powers (-2 and – 1) of the variable x are not whole numbers.

 

7. \(\frac{1}{x}\) – x + 2

Solution:

(\(\frac{1}{x}\) – x + 2) is not a polynomial, since the power of the variable x of its term \(\frac{1}{x}\) = x (-)1 is (-1) which is not a whole number.

 

8. x¹⁵ – 1

Solution:

The algebraic expression (x¹⁵ – 1) is a polynomial since all the powers (15 and 0) of its variable x are whole numbers.

The degree of this polynomial is 15 since the highest of its variable x is 15.

 

9. 3√t + \(\frac{t}{27}\)

Solution:

(3√t + \(\frac{t}{27}\)) is not a polynomial, since the index of its variable r in the term \(\sqrt[3]{t}=t^{\frac{1}{3}} \text { is } \frac{1}{3}\) which

is not a whole number.

 

10. \(\frac{1}{√2}\)x² – √2x+2

Solution:

The algebraic expression \(\left(\frac{1}{\sqrt{2}} x^2-\sqrt{2} x+2\right)\) is a polynomial, since all the powers (2, 1, 0) of its

variable x are whole numbers.

The degree of this polynomial is 2 since the highest power of its variable x is 2.

 

11. 0

Solution:

0 is a polynomial, since 0= 0. x⁰ = 0. x¹ = 0.x², i.e., O can be expressed as a co-efficient of any one of x⁰, x¹, x¹, …….etc, where the indices (0, 1, 2, ……. ) of the variable x all of which are whole numbers.

The degree of this polynomial is undefined since the highest power of the variable x is impossible to determine.

 

12. 15

Solution:

15 is a polynomial since 15 can be expressed as 15x⁰ (..x⁰ = 1), i.e., the index (0) of the variable x is 0, which is a whole number.

The degree of this polynomial is 0 since the highest power of its variable x is 0.

 

13. y³ + 4

Solution:

The algebraic expression (y³ + 4) is a polynomial since all the indices (3, 0) of its variable y are whole numbers.

The degree of this polynomial is 3 since the highest power of its variable y is 3.

 

14. z+ \(\frac{3}{z}\) +2

Solution:

\(\left(z+\frac{3}{z}+2\right)\) is not a polynomial, since the index of the variable z in the term is not a whole number.

\(\frac{3}{z}=3 z^{-1}\) is (- 1),

 

Question 4. Find the coefficients as per the directions given in the following polynomials:

1. The co-efficient of x² in the polynomial 2 + x + x². 

Solution:

The coefficient of x in the polynomial 2 + x + x² is 1, since x² = 1x². 

 

2. The co-efficient of x² in the polynomial 2 − x² + x3.

Solution:

The co-efficient of x² in the polynomial (2-x+x³) is (-1), − x² = (− 1). x².

 

3. The co-efficient of x in the polynomial x² −x + 2.

Solution:

The co-efficient of x in the polynomial x – x + 2 is (− 1), since – x = (− 1) · x. 

 

4. The co-efficient of x³ in the polynomial 5x³ – 13x² + 2.

Solution:

The co-efficient of x³ in the polynomial 5x³ – 13x²+ 2 is 5 since the term containing x³ is 5x³.

 

Question 5. 

1. Give two different examples of monomials of degree 4, having only one variable.

Solution:

Two different monomials of degree 4, having only one variable are 2x⁴ and y⁴.

 

2. Give two different examples of monomials of degree 3 having only one variable. 

Solution:

Two different monomials of degree 3, having only one variable are x³ and 2y³.

 

Question 6. 

1. Give an example of a binomial of degree 0.

Solution: 

A binomial of degree 0 is a + b, where both a and b are constant.

 

2. Give an example of a trinomial of degree 0.

Solution:

A trinomial of degree 0 is a + b + c, where a, b, and c are constants.

 

Question 7. Write which of the following polynomials are linear, quadratic, and cubic polynomials:

1. x² + x

Solution:

(x² + x) is a quadratic polynomial, since the highest power of its variable x is 2.

 

2. x – x³

Solution:

(x-x³) is a cubic polynomial, since the highest power of its variable is 3.

 

3. 2

Solution:

is a quadratic polynomial, since its highest index is 2.

 

4. y + y²+ 4 

Solution:

(y+ y²+ 4) is a quadratic polynomial, since the highest index of its variable is 2.

 

5. 3t

Solution:

3t is a linear polynomial since the highest power of its variable t is 1.

 

6. 7x³

Solution:

7x³ is a cubic polynomial since the highest power of its variable x is 3.

 

7. 1 + x

Solution:

(1 + x) is a linear polynomial, since the highest index of its variable x is 1.

 

8. x² + y² + a²

Solution:

(x²+ y²+ a²) is a quadratic polynomial, since the highest index of both the variables x and y of it is 2.

 

9. x + y + z

Solution:

(x+y+2) is a linear polynomial, since the highest power of both the variables x and y of it is 1.

 

10. x + y = xy

Solution:

(x + y = xy) is a quadratic polynomial, since the power of the term xy in it is (1 + 1) = 2, which is the highest among all others.

 

Question 8. Find the number of terms of each of the following binomials:

1. (1 + x)²

Solution:

The required number of terms = 2 + 1 = 3.

 

2. (2 + y)³

Solution:

The required number of terms = 3 + 1 = 4.

 

3. (1 + z)¹⁰

Solution:

The required number of terms = 10 + 1 = 11.

 

4. (a + x)¹⁰⁰ 

Solution:

The required number of terms = 100 + 1 = 101. 

 

5. \(\left(1+x^2\right)^q\)

Solution:

The required number of terms = q + 1.

 

6. (1+ y³)⁷

Solution:

The required number of terms = 7+ 1 = 8.

 

7. {(a – x) (a + x)}²⁰

Solution:

Here, the given expression = {(ax) (a + x)}²⁰ = (a² – x²)²⁰

∴ The required number of terms = 20 + 1 = 21.

 

8. \(\left(\frac{x+2 x^2+x^3}{x}\right)^n\)

Solution:

Given expression \(\left(\frac{x+2 x^2+x^3}{x}\right)^n\)

= (1 + 2x + x²)ⁿ  = {(1 + x)²}ⁿ  = (1 + x)²ⁿ 

∴ The required number of terms = 2n + 1.

 

Algebra Chapter 1 Polynomials Zero Polynomials

 

Let p (x) be a polynomial and p(x) = a_nxⁿ+a_n-1 x^n-1 + …………………. + a₁x+a₀,a_n ≠ 0 where n is a whole number and a₀, a₁, . . . . ,a_n are constants.

Now, on putting x = 0 in p (x), we get,

p(0) = a_n.1ⁿ +a_0-10^n-1 + ………………………..… +a_1.0 + a_0 = a₀

 Again, on putting x = 1 in p (x), we get,

p (1) =a_n.1ⁿ +a_n__l.1^{n –1} + ……………………… +a_1.1 + a₀

= \(a_n+a_{n-1}+\cdots \cdots \cdots+a_1+a_0\)

= \(a_0+a_1+a_2+\cdots \cdots \cdots+a_{n-1}+a_n\)

In this way, by putting a real number instead of x in p (x), we get different values of p (x).

These values are called the value of the polynomial for that certain value of x.

For example, let p (x) = x + 1 be a polynomial.

Now, At x = 0, p (x) = p (0) = 0 + 1 = 1.

At x = 1, p (x) = p (1) = 1 + 1 = 2.

\(At x=2, p(x)=p(2)=2+1=3
At x=a, p(x)=p(a)=a+1
At x=n, p(x)=p(n)=n+1\)

Again, let f (x) = 2x² – 3x + 4.

f(0) = 2 – 0² – 3 – 0 + 4 = 4,

f(1) = 2-1² – 3 – 1 + 4 = 3,

f(2) = 2 – 2² – 3 – 2 + 4 = 6  etc.

Similarly, if g (t) = 4f⁴ – 3t³ + 2t² + 4, then

g (0) = 4.0⁴ – 3.0³ + 2.0² + 4 = 4

g (1) = 4.1⁴ – 3.1³ + 2.1² + 4 = 7

g (2) = 4.2⁴ – 3.2³ + 2.2² + 4 = 52.……….etc.

 

Zero of linear Polynomials:

The value of the variable in a linear polynomial for which the value of the polynomial is zero is called the zero of that linear polynomial.

For example, let p (x) = x + 8 be a linear polynomial, then p (- 8) = – 8 + 8 = 0,

∴ (- 8) is a zero of the polynomial.

Also, let a be another zero of p (x) = x + 8

P (a) = 0 => a + 8 = 0

=> a = – 8.

∴ There is one and only one zero of a linear polynomial.

 

Zero of constant Polynomials: 

Let p (x)= 10 be a constant polynomial.

We can write, p (x) = 10. xº, x ≠ 0, (∵ xº = 1),

∴ p (1) = 10. 1o = 10.1 = 10 ( ∵ 1º = 1),  p (2) = 0. 2º = 10.1 = 10 ( ∵ 2º = 1), ………………. etc.

So, for no real value of x, the value of p (x) = 10 is zero.

Similarly, it can be proved that the value of any constant polynomial is never zero for any real value of its variable (or variables).

∴ There are no zeros of the constant polynomials.

 

Zero of zero polynomials: 

Let p (x) = 0 be a zero polynomial.

We can write, p (x) = 0x°, x ≠ 0 ( ∵ x° = 1)

Clearly, p (0) = 0.

Also, p (1) = 0.1º = 0; p (2) = 0.2º = 0; p (3) = 0.3º = 0,……… etc.

∴ 0, 1, 2, 3 …….. etc are the zeros of the zero polynomial p (x) = 0.

i.e., for every real value of x, p (x) = 0.

∴ Each and every real number is a zero of the zero polynomials.

 

Determination of the zero of the polynomials: 

Let p (x) = x + 3 be a polynomial.

We want to determine the zero (or zeroes) of it.

So that p (x) = 0 ⇒ x + 3 = 0 ⇒ x = -3

∴ (-3) is a zero of the polynomial p (x) = x + 3.

Again, let p (x) = x² – 3x + 2

Now, p (x) = x² – 3x + 2 = 0 ⇒ x² – 2x – x + 2=0

⇒ x (x-2) -1( x-2) = 0

⇒ x – 2 = 0 or, x = 2 and x (x – 2) (x – 1)= 0

1 = 0 or, x = 1.

∴ 1 and 2 are two zeroes of p (x) = x² – 3x + 2. 

Therefore, to determine the zeroes of any polynomial, we first assume the given algebraic expression equal to zero obtaining an algebraic equation thereby. 

Later on, solving that equation, we find the value (or values) of the variable of that polynomial given. 

The values thus obtained are the required zero (or zeroes).

 

WORKING RULE:

STEP-I: Put the given algebraic expression equal to zero.

STEP-II: Find the value (or values) of the variable (or variables) consisting of the polynomial. 

From the above discussions we can conclude that:

1. There may or may not have a zero of a given polynomial.
2. The zero of a zero polynomial is any real number.
3. The zero of a zero polynomial is any real number.
4. It is not necessarily true that the zero of a zero polynomial is always 0.
5. 0 may be the zero of a polynomial.
6. There may exist more than one zero of a polynomial.
7. There is one and only one zero of a linear polynomial.

 

Algebra Chapter 1 Polynomials Select The Correct Answer (MCQ):

Examples 1. 

1. If p(x) \(\frac{x^2-64}{x-8}\) then p (8) =

1. 1
2. 0
3. 16
4. Undefined

Solution:

 

 

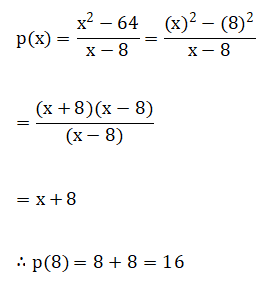
2. If p (x) = x²+9x – 6, then p (0) =

1. – 6
2. 0
3. 1
4. 4

Solution:

p (0) = 0² + 9.0 – 6

= 0 + 0 – 6

= -6.

p (0) = -6.

 

3. If f(x+1)= 2x² – 3x -1, then f(0) = 4.

1. – 1
2. – 2
3. 0
4. 4

Solution:

f (x + 1) = 2x² -3x-1

f(-1 + 1) = 2-(-1)² – 3.(-1) – 1

(putting x = – 1) 

or, f (0) = 2 + 31

or, f (0) = 4.

f (0) = 4

 

4. If f(x) = x when 0 < x < 1

= 2 – x when 1 ≤ x ≤2

= \(x-\frac{x^2}{2}\) when x², then f(1.5) =

1. 1.5
2. 0.5
3. 0.375
4. 1.

Solution:

When x = 15, 1 ≤ x ≤2

⇒ 1≤ 1.5 ≤ 2.

∴ if x = 1.5, then f(x) = 2 – x

⇒ f (1.5) = 2 – 1.5

= 0.5

 

5. If f (x) = \(\frac{1-x}{1+x}\) then f (\(\frac{1}{x}\))

1. \(\frac{1-x}{1+x}\)

2. \(\frac{x-1}{x+1}\)

3. \(\frac{1+x}{1-x}\)

4. \(\frac{x+1}{x-1}\)

Solution:

f(x) = \(\frac{1-x}{1+x}\)

∴ f(x) = \(f\left(\frac{1}{x}\right)=\frac{1-\frac{1}{x}}{1+\frac{1}{x}}=\frac{x-1}{x+1}\)

 

6. If f(x) = -6 + 10x – 7x², then ƒ(− 1) =

1. -3
2. -6
3. -23
4. -9

 

Solution:

When f(-1) = -6 + 10 x (-1) – 7 x (-1)²

= – 6 – 10 – 7

= – 23.

ƒ(− 1) = – 23.

 

7. The zero of the polynomial p (x) = x² – 2x – 8 is

1. – 2
2. 1
3. 0
4. 1

 

Solution:

As per question, x² – 2x – 8 = 0

or, x² – 4x + 2x – 8 = 0

or, x (x-4) + 2(x-4) = 0 

or, (x-4) (x + 2) = 0.

∴ either x – 40 ⇒ x = 4

or, x + 2 = 0 ⇒ x = -2. 

∴ – 2 is a zero of p (x).

 

8. What is the zero of p (x) = 2x-3?

1. \(– \frac{2}{3}\)
2. \(– \frac{3}{2}\)
3. \(\frac{2}{3}\)
4. \(\frac{3}{2}\)

Solution:

As per question, 2x – 3 = 0 ⇒ 2x =3 ⇒ x = \(\frac{3}{2}\)

 

9. If p (x) =x+4, then the value of [p (x) + p (-x)] is

1. – 8
2. 8
3. 2x
4. -2x

Solution: 

Given p (x) = x + 4 = p(x) = x + 4

∴ [p(x) + p (-x)] = x + 4x + 4 = 8.

 

10. The zero of the polynomial f (x) = ax + b (a + 0) is

1. \(\frac{a}{b}\)
2. \(\frac{b}{a}\)
3. \(-\frac{a}{b}\)
5. \(-\frac{b}{a}\)

Solution: 

As per question, ax + b = 0

⇒ ax = -b

x = \(– \frac{b}{a}\)

 

Algebra Chapter 1 Polynomials Short Answer Type Questions

Question 1.

1. If (3x – 1)⁷ = \(a_7 x^7+a_6 x^6+a_5 x^5+\ldots+a_1 x+a_0\), then find the value of \(a_7+a_6+a_5+\ldots+a_0\)

Solution:

Putting x = 1. in the given equation,

We get, (3.1 – 1)⁷ = \(a_7 \cdot 1^7+a_6 \cdot 1^6+a_5 \cdot 1^5+\ldots+a_1 \cdot 1+a_0\)

(3 – 1)⁷ = \(a_7+a_6+a_5+\ldots \ldots+a_1+a_0 or, 2^7=a_7+a_6+a_5+\ldots . . . .+a_1+a_0\)

or, 128 = \(a_7+a_6+a_5+\ldots \ldots+a_1+a_0\)

\(a_7+a_6+a_5+\ldots \ldots \ldots+a_0=128\)

 

2. If p (x) = 4, then find p (x) + p (-x).

Solution:

p (x) = 4,

∴ p (x) = 4, p (x) + p (x) = 4 + 4

= 8.

p (x) + p (-x) = 8.

 

3. If f(y)= \(\frac{y+1}{y+2}\), then find ƒ(0) + f(-1).

Solution:

f(y) = \(\frac{y+1}{y+2}\)

∴ f(0) = \(\frac{0+1}{0+2}=\frac{1}{2}\)

and f(-1) = \(\frac{-1+1}{-1+2}=\frac{0}{1}\)

= 0.

ƒ(0) + f(-1) = 0.

 

4. If f(x) = \(\frac{2-x}{2+x}\) then determine f (x−1).

Solution: 

Given that f(x) = \(\frac{2-x}{2+x}\)

\(f\left(x^{-1}\right)=\frac{2-x^{-1}}{2+x^{-1}}=\frac{2-\frac{1}{x}}{2+\frac{1}{x}}=\frac{2 x-1}{2 x+1}\)

 

5. If f(x+2)=x² + 2x + 3, then find f (x + 4)

Solution:

Given that f(x + 2)= x² + 2x + 3.

Putting (x+2) instead of x in (1) we get,

f(x + 2 + 2) = (x + 2)² + 2 (x + 2) 3.

or, f(x+4)= x² + 4x + 4 + 2x + 4 + 3

= x² + 6x + 11

f(x+4) = x² + 6x + 11.

 

6. If f(\(\frac{1}{y}\) = \(\frac{2}{y}\) – \(\frac{1}{y^2}\) then find f(y).

solution:

Given that f(\(\frac{1}{y}\) = \(\frac{2}{y}\) – \(\frac{1}{y^2}\))………………………….(1)

Putting \(\frac{1}{y}\) instead of y in (1) we get,

 

 

7. Find the zero of the polynomial f (x) = x² – 3x + 2.

Solution:

f(x) = x² – 3x + 2 = 0

or, x²-x- x- 2x + 2 = 0.

or, x (x-1)-2(x-1)= 0

or, (x-1) (x-2)=0.

∴ Either x 10 x = 1;

or, x-2=0x = 2.

∴ The required zeroes are 1 and 2.

 

8. Determine the zeroes of, the polynomial p (x) = x² – 2x – 8. 

Solution:

p (x)=0 ⇒ x²-2x-8= 0 

or, x²-4x+2x-8=0 

or, x (x-4)+2 (x-4)= 0 

or, (x-4) (x+2)= 0.

∴ Either x – 4 = 0 ⇒ x = 4

or, x + 2 = 0

x = -2.

∴ The required zeroes are -2 and 4.

 

9. If f (x) = 0 when x is an integer

 = 2 when x is not an integer,

then find

1.  f(0)

Solution :

(a) 0 is an integer, 

∴ f(x) = 0 f (0) = 0. 

 

2. f (√2)

Solution:

√2 is not an integer,

∴ f(x)=2

f(√2)=2.

 

10. If f(x) = 2x + 3, then x is rational.

= x2 + 1, when x is irrational.

then find

1. f (0)

Solution:

0 is a rational number,

∴ f (x) = 2x + 3

⇒f (0) 2.0 + 3 = 0 + 3 = 3.

 

2. f (π).

Solution:

f (π) is an irrational number, 

∴ f(x) = x² + 1

f(π) = π² + 1.

 

Algebra Chapter 1 Polynomials Long Answer Type Questions

 

Question 1. If f (x) = ax + b and f(0) = 3, f(2)= 5, then find the value of a and b. 

Solution: Given that f (x) = ax + b = f (0) = a0 + b = b.

Also, f (0) = 3. (Given) b= 3 [ b = f (0) = 3]

Again, f(2) = a.2 + b 

= 2a + b 

= 2a + 3 [ ∵ b = 3]

As per the question, f(2) = 5

2a +3 = 5

2a = 2 

⇒ a = 1.

∴ the required value: a = 1, b = 3.

 

Question 2. If f (x) = ax² + bx + c and ƒ (0) = 2, f(1) = 1, f(4) = 6, then find the values of a, b, and c. 

Solution:

Given that f(x) = + bx + c,

∴ f (0) = a.0²+ b.0 + c = c.

As per the question, f (0) = 2

c = 2  [ ƒ (0) = c]

Again, f (x) = ax² + bx + c, 

∴ f(1) = a.1² + b.1 + c = a+b+c.

As per question, f(1) = 1, a+b+c=1 

or, a+b+2= 1 ( c=2) or, a=-1-b…. (1) 

Also, f (x) = ax² + bx + c

f(4) = a4²+ b4 + c = 16a + 4b+c,

= 16 (-1 – b) + 4b+ 2 [a= -1 – b and c = 2]

= 16 – 16b + 4b+2= -12b – 14.

As per the question, f(4) = 6 

=> -12b – 14 = 6

or, -12b = 20

b = \(\frac{20}{-12}\)

= \(– \frac{5}{3}\)

Now, we get from (1), a = -1 -[lstex]\frac{5}{3}[/latex] = -1 + \(\frac{5}{3}\)

= 2/3.

∴ a = 2/3, b = \(– \frac{5}{3}\)

 

Question 3. If f(x) = \(\frac{a(x-b)}{a-b}+\frac{b(x-a)}{b-a}\), then prove that f(a) + f(b) = a+b.

Solution:

Given that \(\frac{a(x-b)}{a-b}+\frac{b(x-a)}{b-a}\)

 

 

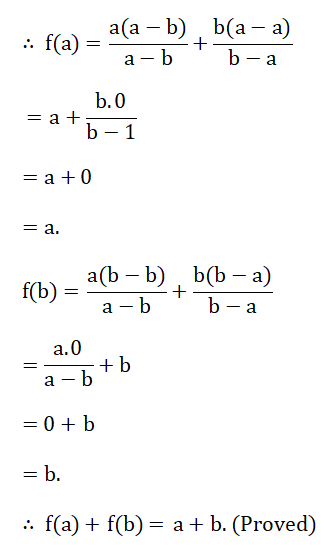
Question 4. If f(x) = \(\frac{a x-b}{b x-a}\). then find the value of f(x).f(\(\frac{1}{x}\))

Solution:

Given that f(x) = \(\frac{a x-b}{b x-a}\)

 

 

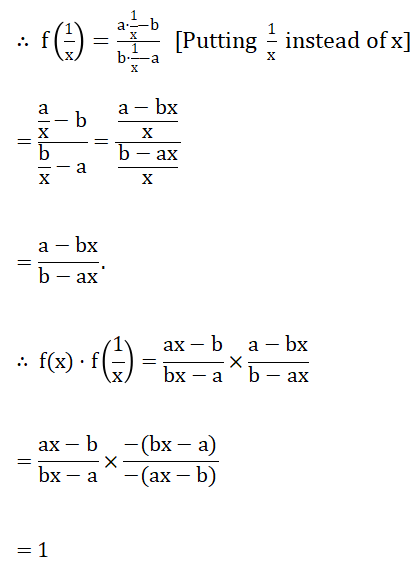
Question 5. If f (x) = x²- 5x + 6, then find f (x² + 2).

Solution:

Given that f(x) = x²- 5x + 6.

∴ f(x² + 2) = (x2 + 2)² – 5 (x²+2)+6 (Putting (x²+2) instead of x.) 

= (x²)² + 2x².2 + (2)² – 5x² – 10 +6

= x⁴ + 4x² + 4 – 5x²- 4 

= x⁴ – x²

:. f(x² + 2) = x⁴ – x².

 

Question 6. If f(x) =3x, then prove that f (x + 1) = 9f (x – 1).

Solution:

Given that f (x) = 3^x,

:. f (x + 1) = 3^x+1 = 3^x .3…… (1)

and f (x-1)=3^x-1

= 3^x.3^-1

= 3^x \(\frac{1}{3}\)

∴ 9ƒ (x-1)=9 × 3^x . \(\frac{1}{3}\) =3^x .3…………….(2)

∴ from (1) and (2) we get, f (x + 1) = 9 f (x – 1). (Proved)

 

Question 7. If f (x) = x⁹ – 6x⁸– 2x⁷ + 12x⁶ + x⁴ – 7x³ + 6×2 + x – 3, then find the value of f (6). 

Solution:

Given that f= x⁹ – 6x⁸ – 2x⁷ + 12x+x+ – 7x³ + 6x² + x-3.

∴ f (6) = 6⁹ – 6.6⁸ – 2.6⁷+ 12.6⁶  + 6⁴ – 7.6³ + 6.6² + 6-3

=6⁹ -6⁹ -2.6⁷ +2.6.6⁶ + 6.6³ -7.6³ +6³ +6³

=-2.6⁷ +2.6⁷ +7.6³ -7.6³ + 6-3

= 6 – 3 

= 3.

∴ the required value = 3.

 

Question 8. If f (x) = log₃x and g (x) = x2, then prove that f (g (3)) = 2.

Solution: 

Given that g (x) = x², . 8 (3) = 3² = 9.

Again, given that f(x) = log₃x, 

∴ f (9) = log₃9 = log₃3²

2 log₃3 (by the formula of log)

= 2 x 1 (log₃3 = 1) = 2.

Now, f (g (3)) = f (9) [ ∵ g (3) = 9]

= 2 [ ∵ f (9)=2]

∴ f (g (3)) = 2. (Proved)

 

Algebra Chapter 1 Polynomials Identity And Equation

 

Identity:

Definition: If two mathematical statements are related by the sign ‘equal’ in such a way that for any real value of the variable (or variables) existing in it, the left-hand side and the right-hand side of the relation are always equal, then the relation is called an identity.

For example, we know that (x + y)²=x+2xy + y². Then this relation is an identity; since for any real value of the two variables x and y, the left-hand side and the right-hand side are equal. Let x = 1 and y = 2 be any two real values of x and y. Now,

L.H.S.= (x + y)²

= (1 + 2)²

= 3²

= 9 and

R.H.S.= x²+2xy + y²

= 1² + 2(-1)(-2) +2²

= 1 + 4 +4 

= 9.

∴ L.H.S.  = R.H.S.

Similarly, it can be shown that for any other real values of x and y, the relation is true. 

∴ (x + y)² = x² + 2xy + y² is an identity.

Mathematically, for any real value of x if p (x) = 0, then the relation p (x) = 0 is called an identity.

 

Equation:

Definition:

If two mathematical statements are related by the sign ‘equal’ in such a way that for a certain number of real values of the variable (or variables) existing in it, the left-hand side and the right-hand side of the relation are always equal then the relation is called an equation.

For example, let p (x) = x²-1 and p (x) = 0, ie, 1 = 0, then it is an equation. Since x = 2 and x= 3 are only the two certain real values of the variable for which the left-hand side and the right-hand side of this relation are equal.( 2² – 5 – 2 + 6= 0 and 3² – 5.3 + 6 =0).

For any other real value of x, the relation is not satisfied.

Mathematically, if for a certain number of real values of x, the relation p (x) = 0 is true, then p (x) = 0 is called an equation.

 

Algebra Chapter 1 Polynomials Roots of Polynomial

 

Definition:

The real value (or values) of the variable (or variables) existing in the equation of a polynomial for which the equation is satisfied is called a root of the equation.

For example, let p (x) = x²-1 and p (x) = 0, i..e., x² – 10 be an equation of a polynomial. 

Then we get, p (1) =  1² – 1= 0

Also p (-1) = (-1)²-1 = 0.

∴ 1 and – 1 are two real values of x for which the equation p (x) = 0 is satisfied.

∴ 1 and – 1 are the two roots of the equation p (x) = 0.

 

Division algorithm of Polynomials:

In the previous classes, you have done a lot of divisions of constant numbers, especially in the arithmetic section. Such as-

 

 

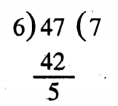
Here, Dividend = 47

Divisor = 6

Quotient = 7 and 

Remainder = 5.

The formula regarding this division you have learned in arithmetic is Dividend = Divisor x Quotient + Remainder,

 i.e. 47 = 6 x 7 + 5.

This very formula of arithmetic is generally used as a Division algorithm in algebra.

In algebra, Dividend = f (x), where f (x) is a polynomial

 Divisor = g(x) where g (x) is a non-zero polynomial

Quotient = q(x), where q (x) is a polynomial, and Remainder = r (x) where r (x) is also a polynomial of degree less than that of g (x).

∴ according to the Division algorithm,

 

 

Since f (x), g (x), q (x) and r (x) are all polynomials the followings are remembered seriously:

1. The degree of f (x) must be equal to or more than that of g (x), i.e., the degree of the dividend must be equal to or more than the degree of the divisor.

If it comes to any case that the degree of the dividend is less than the degree of the divisor, then the whole division algorithm changes to the form of indices and we shall discuss it in the next chapters.

In this chapter, we shall deal with those division algorithms, where the degree of the dividend is equal to or more than that of the divisor.

2. The degree of the divisor is always at least 1 degree higher than that of the remainder, i.e., if the degree of the remainder is 1, the degree of the divisor must be at least (1 + 1) = 2, if the degree of the remainder is 2, then that of the divisor must be at least (2 + 1) = 3, etc.

Therefore, the Degree of g (x) > Degree of q (x).

3. If (x) = 0, i.e., the remainder is a zero polynomial, then f (x) = g(x) × g (x).

In such cases, we say that the dividend (f(x)) is divisible by the divisor (g(x)) and g (x) and q (x) are both the factors of f (x).

Again, f(x) must be a multiple of both g (x) and q (x).

For example,

 

 

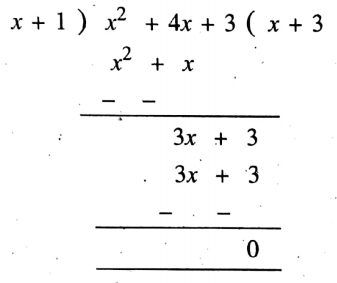
In this division algorithm,

Dividend = x²+ 4x + 3 = f (x),

Divisor = x + 1 = g(x)

Quotient = x +3 and q (x),

Remainder = 0 = r (x).

Since (x) = 0, both the polynomials

g(x) = x + 1 and q (x) = x + 3 are factors of the polynomial f (x) = x² + 4x + 3. 

 

Therefore, the theorem related to the factors of a polynomial is as follows:

Theorem :

If f (x) is a polynomial of the variable x and if for any x = a, f (x) = 0, then (x – a) is a factor of f (x).

For example, let f (x) = x²- 7x + 10 be a polynomial of x.

Putting x = 2 in f (x) we get, f(2) = 2² -7 x 2+10

= 4 -14 + 10

= 0.

∴ (x -2) is a factor of f (x) = x² – 7x + 10, i.e., the polynomial x² – 7x + 10 is divisible by x-2. 

∴ we can say that the polynomial f (x) is divisible by (x – a) if f (a) = 0.

Conversely, if f (x) = 0, then the polynomial f (x) is divisible by x-a, i.e., (x – a) is a factor of f(x) or f (x) is a multiple of x-a.

 

Algebra Chapter 1 Polynomials Remainder theorem

Remainder Theorem

If f (x) is a polynomial of degree n (n ≥ 1) and a be any real number, then the remainder, when f(x) is divided by (x-a), is f(a).

Proof:

Let f (x) be a polynomial of degree n (n ≥ 1) and let f (x) is divided by (x-a), the unique quotient is q(x) and the unique remainder is r (x),

i..e, f (x) = (x − a) q (x) + r (x).

According to the division algorithm, the degree of r (x) is less than the degree of the division (x − a). 

Now, the degree of (x – a) = 1.

∴ the degree of r (x) = 0.

∴ (x) is a constant. 

Let r (x) = r.

:. f(x) = (x − a) q (x) + r…… (1)

Since (1) is an identity,

∴ for any real value of x, (1) must be satisfied.

Thus, for any real value a of x, the identity (1) must also be satisfied.

∴ f (a) = (a – a) q (a) + r or, f (a) = 0 + r or, f (a) = r

∴ r = f (a), i.e., the remainder = f (a). (Proved)

 

Algebra Chapter 1 Polynomials Select The Correct Answer (MCQ)

 

Question 1.

1. If P (x) = x+ax² + 6x + a be divided by (x + a), the remainder is-

1. 5a
2. – 5a
3. – a
4. a

Solution:

If P (x) = x² + ax² + 6x + a be divided by (x + a), then the remainder is

 

 

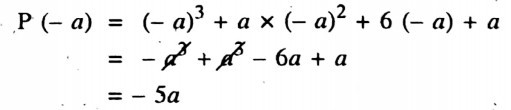
∴ The correct answer is 2. – 5a

 

2. If the polynomial P(x) = x² – 2x + a be divided by (x-3), the remainder is 0. Then a =

1. 0
2. – 1
3. – 3
4. 3

Solution:

If P(x) =  x² – 2x + a is divided by (x-3), the remainder is

P (3) = (3)² – 2 x 3 + a

=9-6+ a

= a + 3

According to question, a + 3 = 0 

a = -3

∴ The correct answer is 3. – 3

 

3. If f (x) = x² + ax – 2a² + 1, g(x) = x – a, q(x) = x + 2a and f (x) = g(x). q(x) + r (x) then

1. 1
2. 0
3. a
4. -2a

Solution:

f(x) = g(x) . q(x) + r(x)

 

 

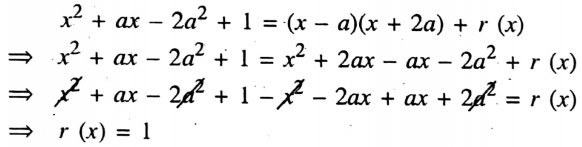
∴ The required answer is 1. 1

 

Algebra Chapter 1 Polynomials Short Answer Type Questions

 

Question 1. Using the remainder theorem, find the remainder when x³-3x² + 2x + 5 is divided by

1. x² and 

Solution:

x + 2 =0

⇒ x= -2.

∴ The required remainder = (-2)³ – 3 (-2)² + 2 (-2) + 5

= – 8 – 12 – 4 + 5

= -19.

The required remainder = -19.

 

2. 2x + 1.

Solution:

2x + 1 = 0

2x – 1

x = \(– \frac{1}{2}\)

∴ The required remainder

 

 

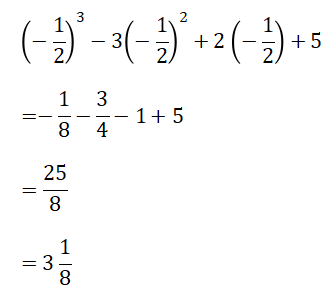
Question 2. Using the remainder theorem determines the remainders when the following polynomials are divided by (x-1)

1. x³ – 3x² + 4x + 50

Solution:

x-1 = 0 ⇒ x = 1.

The required remainder = 1³ – 3.1² + 4.1 + 50

= 1 – 3 + 4+ 50

= 52

The required remainder = 52

 

2. 11x³ – 12x² – x + 7

Solution:

The required remainder = 11.1³ – 12.1² – 1 + 7

= 11 – 12 + 6

= 5.

The required remainder = 5.

 

Question 3. Find the remainder using the remainder theorem, when 

1. (x³-6x² + 9x – 8) is divided by x-3.

Solution:

x – 3 = 0

x = 3.

∴ The required remainder = 3³ – 6.3² + 9.3 – 8

= 27 – 54 + 27 – 8

= -8

The required remainder = -8

 

2. (x³ – ax² + 2x – a) is divided by x-a.

Solution:

x – a = 0

⇒ x = a.

The required remainder = a³ – a.a² + 2a – a 

= a³ – a³ + 2a – a 

= a.

The required remainder = a.

 

Question 4. Using the remainder theorem, examine whether (2x + 1) is a multiple of the polynomial p (x) = 4x³ + 4×2 – x – 1 or not.

Solution:

2x + 1 = 0

2x  = -1

⇒ x = \(– \frac{1}{2}\)

Now,

 

 

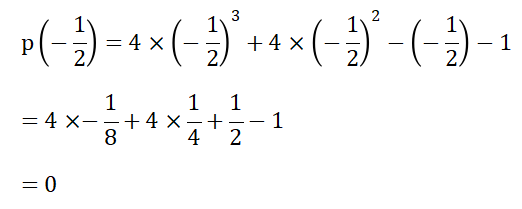
∴ p (x) is a multiple of (2x + 1).

 

Question 5. Find the value of an if the remainders, when both the polynomials (ax + 3x² – 3) and (2x³- 5x + a) are divided by (x-4), are the same.

Solution: 

x – 4 = 0

⇒ x= 4.

So, when (a³ + 3x² -3) is divided by (x-4),

the remainder = a.4³ + 3.4² – 3

= 64a + 48 – 3

= 64a + 45.

Again, when (2x³ – 5x + a) is divided by (x-4).

the remainder = 2.4³ – 5.4 + a

= 128 – 20 + a

= a + 108

As per question, 64a + 45 = a + 108 

or, 63a = 63 

or, a = 1.

The required value of a = 1.

 

Question 6. If the polynomials x³ + 2x²-px-7 and x³+ px² – 12x + 6 be divided by (x + 1) and (x-2) respectively, the remainders are r₁ and r₂ and if 2r₁ + r₂ = 6, then find the value of p.

Solution: 

x+1 = 0

⇒ x = -1.

 

 

The required value of p = 4\(\frac{2}{3}\)

 

Question 7. Find the remainder when

1. (x³ + 3x³ + 3x + 1) is divided by (x + π)

Solution:

Given (x³ + 3x³ + 3x + 1):-

x +π = 0

⇒ x = -π.

The required remainder = (-π)³ + 3 (- π)² + 3 (− π) + 1

= – π³ + зπ² – зπ + 1.

The required remainder = – π³ + зπ² – зπ + 1.

 

2. (x³ – ax² + 6x – a) is divided by (x-a)

Solution:

Given (x³ – ax² + 6x – a)

x – a = 0

x = a.

The required remainder = a³ – a.a² + 6·a – a 

= a³ – a³ + 6a – a

= 5a.

The required remainder = 5a.

 

Question 8. Prove that (3x³+ 7x) is not a multiple of (7 + 3x).

Solution: 

Given Polynomial (3x³+ 7x)

7+ 3x = 0

3x = – 7

⇒ x = \(– \frac{7}{3}\)

Now, 

 

 

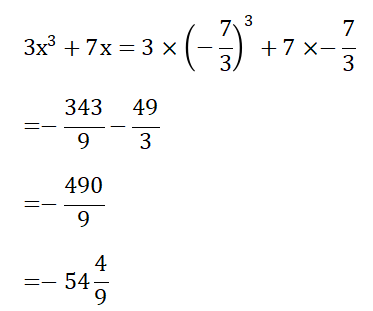
3x³ + 7 x ≠ 0,

∴ (3x³ + 7x) is not a multiple of (7 + 3x). (Proved).

 

Question 9. The polynomial (px² + qx + r) is divisible by (x² – 1) and if x = 0, the value of the polynomial is 2. Determine the values of p, q, and r.

Solutions:

Given Polynomial (px² + qx + r):-

x² – 1 = 0

or, x² = 1

or, x = ± 1.

By the first condition given,

p.1²+q.1+r=0 

or, p + q + r = 0……. (1)

and p.(-1)²+q.(-1) + r = 0 

or, p – q + r = 0……..(2)

 By the second condition given,

p.0²+q.0+ r = 2

or, r = 2.

∴ From (1) we get, p+q=2, and from (2) we get

p – q = -2

∴ p = -2, q = 0 and r = 2.

 

Question 10. If the polynomial (x+4x²+4x-3) is divided by x, what should be the remainder? 

Solution : 

Given Polynomial (x+4x²+4x-3)

x can be written as (x = 0).

∴ x = 0

∴ The required remainder = 0³ + 4.0² + 4.0 – 3

= -3.

∴ The Reminder Is -3

 

Algebra Chapter 1 Polynomials Long Answer Type Questions

 

Question 1. Identify the identities and the equations among the following statements

1. x² – (a + b) x + ab = 0

Solutions: 

x² – (a + b) x + ab = 0 

or, x² – ax – bx+ ab = 0.

or, x (x – a) b (x – a) = 0 

or, (x − a) (x – b) = 0.

∴ either x – a = 0⇒x= a 

or, x-b=0

⇒ x = b.

The given statement is satisfied for only two values of x = a and x = b of x and is not satisfied for any other real value of x.

. The given statement is an equation.

 

2. (x+y) (x – y) = x² – y²

Solution:

Given statement is (x + y) (x − y) = x² – y².

Let x = 2 and y= 1.

∴ L.H.S. = (2 + 1) (2 – 1) 

= 3.1 

= 3.

R.H.S. = (2)² – (1)²

= 4 – 1

= 3.

L.H.S. = R.H.S, i.e, the statement is true for x = 2 and y = 1.

Again, let x = 4, y = 2.

∴ L.H.S.. = (4 + 2) (4 – 2) 

= 6 x 2 

= 12.

R.H.S. = (4)² – (2)²

= 16 – 4

= 12.

∴ L.H..S. = R.H.S., i.e, the statement is true for x = 4 and y = 2.

Similarly, let x = m, y = n (m, n ∈ N);

∴ L.H.S. = (m + n) (m – n)

= m² + mn – mn – n²

= m² – n².

R.H.S.= m² – n²

L.H.S. = R.H.S., i.e. the statement is for x = m and y = n, where m, n ∈ N.

Thus, it can be shown that for any real value of x and y, the given statement is true.

∴ The given statement is an identity.

 

3. x² – (2a + \(\frac{1}{a}\)) x + 2 = 0

Solution:

x² – (2a + \(\frac{1}{a}\)) x + 2 = 0

or, x² – 2ax – \(\frac{1}{a}\) + 2 = 0.

∴ either x – 2a = 0 

x = 2a,

or, x – \(\frac{1}{a}\) = 0

x = \(\frac{1}{a}\)

the statement is true for only two values of x.

∴ The given statement is an equation.

 

4. ab = \(\left(\frac{a+b}{2}\right)^2-\left(\frac{a-b}{2}\right)^2\)

Solution:

The given statement is ab = \(\left(\frac{a+b}{2}\right)^2-\left(\frac{a-b}{2}\right)^2\)

Let a 1 and b = 1,

∴ L.H.S. ab 1 x 1 = 1 and R. H.S.= \(\left(\frac{1+1}{2}\right)^2-\left(\frac{1-1}{2}\right)^2\) = 12 – 0

= 1.

∴ L.H.S. R.H.S., i.e., the statement is true for a = 1, b = 1.

Again, Let a = m and b = n.

∴ L.H.S. m x n = mn and R.H.S.

 

 

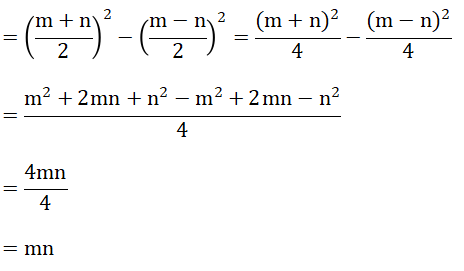
∴ L.H.S. R.H.S., i.e. the statement is true for a = m and b = n.

In a similar way, it can be shown that for any real value of a and b, the given statement is true. 

The given statement is an identity.

 

Question 2. Find the roots of each of the following equations of polynomials

1. p(x) = 2x + √11

Solutions: 

p(x) = 0 = 2x+√11 = 0

⇒ 2x = -√11

⇒ x = \(– \frac{√11}{2}\)

∴ The required root = \(– \frac{√11}{2}\)

 

2. p (x, y) = x² + y² – 2x + 1

Solution:

p (x, y) = x²+ y²-2x+1 = 0

x²-2x+1+ y2 = 0

(x – 1)² + y² = 0 both (x – 1² = 0 and y² = 0

⇒ x – 1 = 0

⇒ x = 1

∴ The required roots are x = 1 and y = 0.

 

3. p (x) = ax² + bx + c, a 0 and a, b, c are constants.

Solution:

p(x) = 0

ax² + bx + c = 0

x² + \(\frac{b}{a}\) x + \(\frac{c}{a}\) = 0 [Dividing by a]

 

 

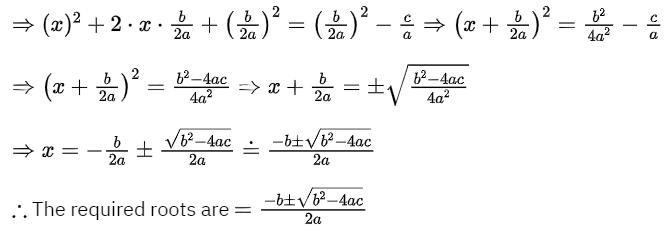
4. p(x) = x² – \(\frac{2}{p}\) (p²+1)x+4

Solution:

 

 

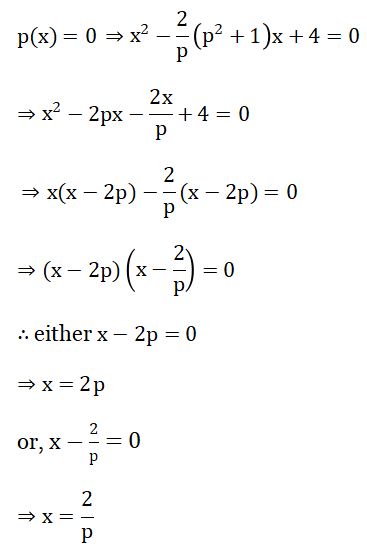
∴ The required roots are 2p and \(\frac{2}{p}\).

 

Question 3. 

1. Find the root of the equation of the linear polynomial f (x) = 2x + 3.

Solutions:

2x + 3 = 0

or, 2x = 3

or, x = \(– \frac{3}{2}\)

∴ The required root = \(– \frac{3}{2}\)

 

2. Show that if one of the roots of the equation of the quadratic polynomial x² – 6ax – 91 = 0 is 7; then the other root is (-13).

Solutions:

One root of x² – 6ax – 91 = 0 is 7

∴ 7² – 6a⁷ – 91 = 0 

or, 49 – 42a = 91 

or, 42a = – 42 

or, a = \( – \frac{42}{42}\)

∴ the given equation is x² – 6 (-1). x-91 = 0.

or, x² + 6x – 91 = 0.

Now, x²+ 6x-91 = 0

x² + 13x-7x-91 = 0

⇒ x(x + 13) -7(x + 13) = 0

(x+13) (x-7)=0

∴ Either x + 13 = 0 

x = -13, 

or, x-7 = 0

⇒ x = 7.

∴ The other root = – 13. (Proved)

 

Alternative Method:

Let the other root = β

We know that the product of the roots, where the equation is ax² + bx + c = 0.

Here, a = 1 and c = – 91.

∴ 7β = \(– \frac{91}{1}\)

β = -13

The other root = -13.

 

Question 4. If the polynomial x⁴ – 2x³ + 3x² – a – ax + b is divided by (x 1) and (x + 1), the respective remainders are 5 and 19. Determine the remainder when the polynomial is divided by (x + 2).

Solution:

x+1 = 0

⇒ x = 1.

By the first condition given, 1⁴ – 2.1³ + 3.1² – a.1 + b = 5

or, 1 – 2 + 3 – a+b= 5

or, b = a + 3……….(1).

Again, x + 1 = 0 or, x = -1.

By the second condition given,

(-1)⁴ – 2(-1)³ + 3 x (1)² – a x (-1) + b = 19

or, 1 + 2 + 3 + a + b = 19 

or, a + b = 13

or, a + a + 3 = 13 [from (1)]

or, 2a = 10

or, a = 5

∴ From (1) we get, b = 5 + 3 = 8 ( a = 5 )

Now, x + 2 = 0

or, x = -2.

By the third condition given,

∴ The required number = (-2)⁴ – 2(-2) + 3(-2)² – a (-2) + b

= 16+ 16 + 12 + 2a + b

= 44 + 2 x 5+ 8

= 52 + 10

= 62.

The required number = 62.

 

Question 5. The polynomial (x³+ px² -x+ q) is divisible by (x² – 1) and when it is divided by (x²), the remainder is 15. Find the values of p and q.

Solution:

x² – 1 = 0

or, x² = 1

or, x = ± 1.

Again, x – 2 = 0 

or, x = 2.

By the first condition given, 1³+ p.1² – 1 + q = 0

or, 1 + p – 1 + q = 0 

or, q = – p.

Again, (1)³ + p.(-1)² – (-1) + q=0

or, 1+p+ 1+ q = 0

or, q = -p

∴ q = -p……….. (1)

By the second condition given, 2³ + p.2² – 2 + q = 15

or, 8+ 4p²+q= 15

 or, 4p – p = 15 – 8 +2 [by (1)]

or, 3p = 9 

or, p = 3.

From (1) we get, q=3.

∴ p = 3 and q = -3.

 

Algebra Chapter 1 Polynomials Factor Theorem

Factor theorem :

If p (x) is a polynomial of degree n (n ≥ 1) and a be any real number, then

1. (x – a) is a factor of p (x) when p (a) = 0

2. p (a) = 0 when (x – a) is a factor of p (x)

Proof :

According to the remainder theorem, if p (x) is divided by (x-a), then a polynomial q (x) is obtained such that 

p (x) = (x – a) q (x) + p (a) …………………… (1)

1. When p (a) = 0, from (1) we get, p (x) = (x – a) q(x) + 0

or, p (x) = (x – a) q (x)

(x – a) is a factor of p (x). (Proved)

 

2. When (x – a) is a factor of p (x), p (x) = (x – a) q(x) ……. (2)

where q(x) is obtained on dividing the polynomial p (x) by (x-a).

Now putting x – a = 0 or, x = a in (2) we get,

p (a) = (a – a) q (a) 

or, p (a) = 0 x q (a) 

or, p (a) = 0.

p (a) 0. (Proved)

 

From the factor theorem, we get the following corollaries:

1. (x+a) is a factor of p (x) if p (-a) = 0.

2. (ax + b) are the factors of p (x) if p \(\left(\mp \frac{b}{a}\right)\) = 0.

In the following examples, you should know much more about the applications of the factor theorem.

 

Algebra Chapter 1 Polynomials Select The Correct Answer (MCQ):

Question 1. 

1. If for the polynomial f(x), f(1/2)=0, then one of the factors of f (x) is —

1. x – 1

2. x + 1

3. 2x-1

4. 2x + 1

Solution:

f(1/2) = 0

∴ x = 1/2

or, 2x = 1 

or, 2x – 1 = 0

i.e., (2x-1) is a factor of f (x).

 

2. If the polynomial p (x) = x+6x² + 4x + k is divisible by (x + 2), then k =

1. – 6

2. – 7

3. – 8

4. – 9

Solution: 

As per the question, (x+2) is a factor of p (x).

∴ p (-2) = 0 ( ∵ x+2 = 0 ⇒ x =-2) 

or, (-2)³ + 6 x (-2)² + 4 x (2) + k = 0 

or, -8 + 24 + 8 + k = 0 

or, k = − 8.

 

3. If (x – 1) is a factor of the polynomial f (x), but not a factor of the polynomial g (x), then (x 1) is a factor of which one of the following?

1. f (x) – g(x)
2. f (x) + g(x)
3. f (x) g (x)
4. (f(x)+ g(x)) g (x)

Solution:

 

 

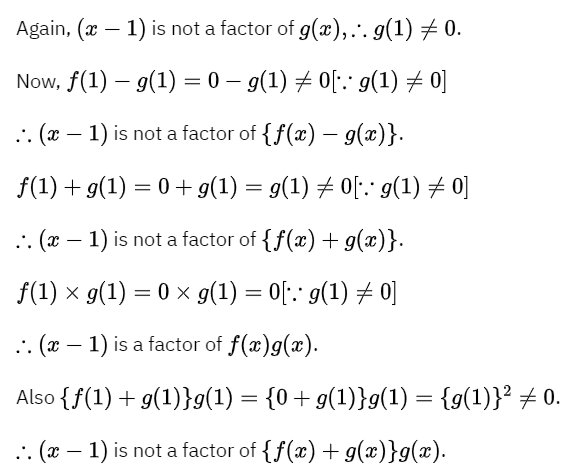
4. If (n² – 1) is one of the factors of the polynomial f(n) = an⁴ + bn³ + cn² + dn+e, then

1. a + c + e = b + d.
2. a + b + e = c + d
3. a + b + c = d + e
4. b + c + d = a + e

Solution:

n² – 1 = 0

⇒ n = ± 1.

As per the question, f (1) = 0 and f (-1) = 0.

Now, f(1) = 0 a.14 + b.13 + c.1² + d.1 + e = 0 

or, a + b + c + d + e = 0. 

Also, f (-1) = 0 a.(− 1)² + b.(− 1)³ + c.(− 1)² + d.(− 1) + e = 0.

⇒ a – b + c – d + e = 0

⇒ a + c + e = b + d.

∴ 1. a + c + e = b + d. is the correct answer.

 

5. (x + 1) will be a factor of the polynomial p (x) = x + 1, when

1. n is a positive integer
2. n is a negative integer
3. n is an even positive integer
4. n is an odd positive integer

Solution:

(x + 1) is a factor of p (x) = xⁿ + 1

∴ p(- 1) = 0 ( ∵ x + 1 = 0 ⇒ x = 1)

or, (- 1)ⁿ + 1 = 0 ……… (1)

Now, if n be an even positive integer, let n = 2m, where m is any natural number.

m

∴ from (1) we get, (- 1)^2m+ 1 = 0 

or, ((-1)2)^m + 1 = 0 

or, 1^m + 1 = 0

or, 1+1=0( m is any natural number) 

or, 2 = 0, which is clearly impossible.

∴ n is not an even positive number.

Now, if n is an odd positive integer, let n = 2^m+ 1, where m is any natural number.

∴ from (1) we get, (- 1)2m+1 + 1 = 0

or, (- 1)^2m x (-1) + 1 = 0         

or, (-1)^2m x (-1)+ 1 = 0 

or, 1 x (-1) + 10 [∵ m is any natural number]

or, 1 + 1 = 0 [∵ 1 x -1 = -1]

or, 0 = 0, which is possible.

∴ If n is an odd positive integer, then p (− 1) = 0.

4. n is an odd positive integer is a correct answer.

 

6. If x be a factor of the polynomial p (x) = (x -1) (x + 2) (x + 3), then p (0) =

1. 2

2. 3

3. 4

4. 6

Solution:

p (0) = (0 – 1) (0 + 2) (0 – 3) 6. 

∴ 4. 6 is correct.

 

7. If (x + 1) be a factor of the polynomial p (x) = x²+k, then k =

1. -1

2. 0

3. 1

4. 2

Solution: p(- 1) = 0 [ …. x + 1=0 or, x = – 1 ]

or, (- 1)³ + k = 0

or, – 1 + k = 0

or, k = 1.

∴ 3. 1 is correct.

 

8. If one of the factors of the polynomial p (a) = a⁴ + x² – 20 be (x² + 5), then the other factor is

1. x² + 4
2. x² – 4
3. x² – 1
4. x² – 5

Solution:

Let the other factor = x²+ a.

(x² + 5) (x² + a) = x⁴ + x² – 20

or, x⁴ + 5x² + ax² + 5a = x⁴ + x² – 20

or, x⁴ + (5 + a)x² + 5a = x⁴ + x² – 20, which is an identity.

5a = – 20      [Equating the constant terms]

or, a = – 4

∴ The required factor = x² – 4.

 

Alternative Method:

x⁴ + x² – 20 = x⁴ + 5x² – 4x² – 20

= x² (x² + 5) – 4 (x² + 5)

= (x² + 5) (x² – 4).

∴ The required factor = x² – 4.

 

9. If f(x) = kx²-3x + k and g (x) = x – 1 be two polynomials, then g (x) will be a factor of f(x) when k=

1. \(\frac{2}{3}\)
2. \(\frac{3}{2}\)
3. \(– \frac{3}{2}\)
4. \(– \frac{2}{3}\)

Solution:

g(x) will be a factor of f (x) when /(1) = 0 [ ∵ g (x) = 0 x – 1 = 0 or, x = 1]

or, k.1² – 3.1 + k = 0

or, k – 3 + k = 0

or, 2k = 3

or, k = \(\frac{3}{2}\)

 

10 . Which one of the following is a factor of the polynomial p(x) =4x³+ 4x²- x – 1?

1. 2x – 1
2. x
3. x + 1
4. 2x + 1.

Solution:

2x – 1 = 0

x = {latex]\frac{1}{2}[/latex]

∴ \(p\left(\frac{1}{2}\right)=4 \times\left(\frac{1}{2}\right)^3+4 \times\left(\frac{1}{2}\right)^2-\frac{1}{2}-1=\frac{1}{2}+1-\frac{1}{2}-1=0\)

∴ (2x – 1 )is a factor of p(x),

i,e., 1. 2x – 1 is the correct.

 

Algebra Chapter 1 Polynomials Short Answer Type Questions

 

Question 1.

1. If (x – 1) be a factor of (4x² – kx + 1), find the value of k.

Solution :

Given That (x – 1) Is The  Factor Of The Polynomial (4x² – kx + 1)

x – 1 = 0

=> x= 1.

As per question, 4.1² – k. 1 + 1 = 0 or, 4 – k + 1 = 0

or, k = 5

∴ The Value Of k Is 5.

2. Determine whether n is an odd or even positive integer, when (a + 1) is a factor of (xⁿ – 1).

Solution:

Given That (a + 1) Is A Factor Of (xⁿ – 1)

x+1=0=>x = -1.

Now, putting x = – 1 in xⁿ – 1 we get,

(-1)ⁿ – 1 = (- 1)^2m – 1 when n is an even positive integer, let n = 2m, m ∈ N.

{(-1)²}^m – 1 = 1m – l = 1 – I = 0,

∴ n is an even positive integer.

 

3. If (x + a) is one of the factors of the polynomial x³ + ax² – 2x + a – 12, then find the value of a.

Solution:

Given Polynomial x³ + ax² – 2x + a – 12

x + a = 0

x = -a

x³ + ax² – 2x + a – 12 = (- a)³ + a x (- a)² – 2 x (- a) + a – 12

= – a³ + a³ + 2a + a – 12 = 0

= 3a – 12 = 0

As per question, 3a – 12 = 0

or, 3a = 12

or, a = 4.

∴ The Value Of a is 4

 

4. If (x- 3) be a factor of the polynomial (k²x³ – kx² + 3kx – k), then find the value of k

Solution:

x-3 = 0=>x=3

Now, k²x³ – kx² + 3kx – k = k².3³ – k3² + 3k³ – k – k (27 k – 1).

As per the question, k (27 k – 1) = 0

∴ Either k = 0

or, 27k -1=0

or, k = \(\frac{1}{27}\)

k = 0 or \(\frac{1}{27}\)

 

Algebra Chapter 1 Polynomials Long Answer Type Questions

 

Question 1. Determine for which cases of the followings the polynomial g (x) will be a factor of f(x).

1. When f(x) = 3x³ + x² – 20x + 12 and g (x) = 3x – 2,

 Solution:

g (x) = 0 => 3x – 2 = 0 => 3x = 2 =>x = \(\frac{2}{3}\)

 

 

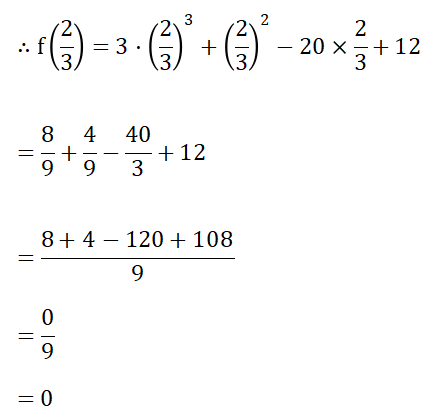
∴ g(x) is factor of f(x)

 

2. When f(x) = x⁴ – x² – 12 and g (x) = x + 2.

Solution:

g(x) = 0

=> x+2 = 0

=> x = -2.

f (- 2) = (- 2)⁴ – (- 2)² – 12

= 16 – 4 – 12

= 0.

∴ g (x) is a factor of f (x).

 

Question 2. Find the value of k if the polynomial p (x) = 2x⁴ + 3x³ + 2kx² + 3x + 6 is divisible by (x + 2).

Solution :

If p(x) is divisible by (x + 2), then p (- 2) = 0 [ ∵ x + 2 = 0 => x = – 2]

2.(-2)⁴ + 3.(- 2)³ + 2k.(- 2)² + 3.(- 2) + 6 = 0

or, 2 x 16 + 3 x (-8) + 2k x 4-6 + 6 = 0

or, 32 – 24 + 8k = 0

or, &k = – 8

or, k = -1

The value of k = -1

 

Question 3. Determine the values of a and b if x² – 4 is a factor of the polynomial ox⁴ + 2x³ – 3x² + bx – 4.

Solution:

x²-4 = 0

=>x² = 4

=>x = ±2.

As per question, a.(2)⁴ + 2.(2)³ – 3.(2)² + b.2-4 = 0

or, 16a + 16 – 12 + 2b – 4 = 0

or, 16a + 2b = 0 or, 8a + b = 0 or, b = – 8a ……..(1)

Again, a.(- 2)⁴ + 2.(- 2)³ – 3.(- 2)² + b.(- 2) – 4 = 0

or, 16a – 16 – 12 – 2b – 4 = 0

or, 16a – 2b = 32

or, 16a – 2 x (- 8a) = 32 [from (1)]

or, 16a + 16a = 32.

or, 32a = 32

or, a = 1.

From (1) we get. b = – 8 x 1 = – 8.

∴ The required values are a = 1, and b = – 8.

 

Question 4. If n is any positive integer (even or Odd), prove that (x – y) is a factor of the polynomial xⁿ – yⁿ.

Solution :

Let p (a) = xⁿ – yⁿ and g (x) = x – y

Then, if p (x) is divided by g (a), we get a polynomial q (x) as the quotient and the remainder R, which is independent of a such that

p {x) = g (x) q (x) + R or, xⁿ – yⁿ = (x – y) q (x) + R ……………………………..(1)

Since (1) is an identity, it is true for all real values of x ( …. R is independent of x).

On putting x – y = 0 or, x = y in (1), we get, yⁿ – yⁿ = (y – y) q (x) + R or, yⁿ – yⁿ = 0 x q (x) + R or, 0 = 0 + R

[ ∵ n is a positive integer (odd or even), ∴ yⁿ – yⁿ– = 0],

or, R = 0,

∴ f (x) = g (x) x q (x) or, xⁿ – yⁿ = (xⁿ  – yⁿ) q (x).

(x – y) is a factor of xⁿ – yⁿ (Proved).

 

Alternative Method:

x – y = 0

x = y

Now, xⁿ  – y ⁿ = yⁿ  – y ⁿ= 0 [∵ n is a positive integer (odd or even)]

∴ (x – y) is a factor of xⁿ  – y ⁿ.(proved)

 

Question 5. Prove that (x – y) can never be a factor of the polynomial xⁿ  + yⁿ , where n is any positive integer (odd or even).

Solution:

Let f (x) = xⁿ + yⁿ and g (x) = x – y. 

If f (x) is divided by g (x) we get a polynomial q(x) as the quotient and the remainder R, which is independent of x, such that xⁿ + yⁿ = (x – y)q (x) + R…….. (1)

Now, (1) is an identity.

So it is true for all values of x [∵ R is independent of x]

∴ On putting x – y = 0 or, x = y in (1) we get,

Yⁿ + yⁿ (y- y) q (x) + R 

or, 2yⁿ= 0x q(x) + R [∵ n is a positive integer (odd or even)] 

or, R = 2yⁿ ≠ 0

(x – y) is never a factor of xⁿ + yⁿ (Proved)

Alternative Method:

x – y = 0

⇒ x = y

∴ xⁿ + yⁿ = yⁿ + yⁿ = 2yⁿ [ ∵ n is a positive integer (odd or even)]

∴ x ⁿ+ yⁿ  ≠ 0

∴ (x-y) can never be a factor of xⁿ + yⁿ (Proved)

 

Question 6. If (x + 1) and (x + 2) be any two factors of the polynomial (x³ + 3x² + 2ax + b), then determine the value of a and b.

Solution: 

x + 1 = 0 or, x = = – 1.

∴ x³ + 3x² + 2ax + b = (-1)² + 3.(-1)² + 2a.(-1) + b

= – 1 + 3 – 2a + b = 2a + b + 2 …….(2)

As per question, 2a + b + 20 or, b = 2a – 2.

Again, x+20⇒x=-2.

x2 + 3x² + 2ax + b = (-2)+ 3 x (-2)²+ 2a x (-2)+b

= – 8 + 12 – 4a + b 

= – 4a + b + 4.

As per question, – 4a + b + 4 = 0

or, – 4a + 2a – 2 +4 = 0 [by (1)]

Or, – 2a + 2 = 0 

or, 2a = -2

or, a = 1

∴ From (1) we get, b = 2 x 12

= 2 – 2

= 0.

The required values are a = 1 and b = 0.

 

Question 7. Determine the values of a and b when the polynomial (ax + bx² + x 6) is divided by (x – 2), the remainder is 4, and (x+2) is a factor of the polynomial.

Solution:

x-2=0

⇒ x= 2.

As per question, a.2³ + b.2² +26= 4

or, 8a+ 4b = 8

or, 2a + b = 2

or, b = 2-2a …..(1)

Again, x+2 = 0 or, x = – 2

As per question, a.(-2)³ + b.(-2)² + (-2)-6 = 0 

or, 2a + b.2 = 0 [Dividing by 4] 

or, 2a + 2a = 0

or, 4a= 0 

or, a = 0.

∴ The required values are a = 0 and b = 2 – 2(0)

= 2.

 

Question 8. Find the relation between p and r if (x – \(\frac{1}{2}\) be two factors of the polynomial px² + 5x + r.

Solution:

To Find The Relation Between p And r

Given Polynomial px² + 5x + r

x – 2 = 0

⇒ x = 2.

 

 

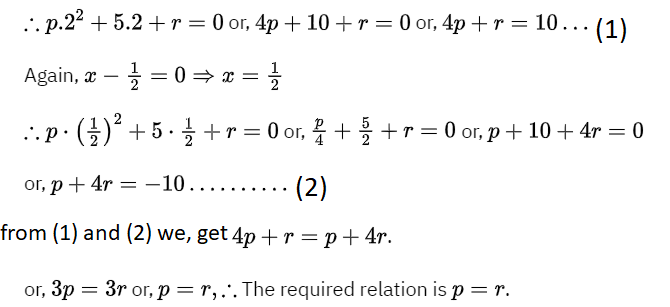
Question 9. If (x + b) is a common factor of both the polynomials (x² + px + q) and (x² + lx + m), then prove that b = \(\frac{q-m}{p-l}\)

Solution:

Given Two Polynomial (x² + px + q) and (x² + lx + m) 

And Given That The Common Factor x+b

x+b=0

⇒ x = – b.

∴ (-b)2 + p. (-b) + q = 0 

or, b2 – pb + q = 0…………(1)

Again, b² + 1 (-b) + m = 0 or, b² – 1b + m = 0……(2) 

Subtracting (2) from (1) we get, b (1 – p) + q – m=0

or, b = \(\frac{m-q}{l-p}\) = \(\frac{q-m}{p-l}\)(Proved)

 

Question 10. Find the condition for which the polynomial x³+(a + b) x + p is divisible by (x + a + b).

Solution: 

Given Polynomial x³+(a + b) x + p

x+a+b = 0

⇒x = – b.

As per question, (-a – b)3 + (a + b) (-a – b) + p = 0

or, – (a + b)³ – (a + b)² + p = 0 

or, p = (a + b)³ + (a + b)²

or, p = (a + b)² + (a + b + 1), which is the required condition.

 

Question 11. If both the polynomials x⁴¹ +a and x⁴¹+ b be divisible by (x + 1) prove that a + b = 2.

Solution:

Given Polynomial x⁴¹ +a and x⁴¹+ b

x+1 = 0

⇒ x = -1.

n = 0.

Now, (x + 1) is a factor of x⁴¹ + a

(-1)⁴¹ + a = 0

or, 1+ a = 0 

or, a = 1.

Again, (x + 1) is a factor of x⁴¹+ b.

(-1)⁴¹+ b = 01+b=0 

or, b = 1.

a + b= 1 + 1 

= 2. (Proved)

 

Question 12. If the polynomial (xⁿ + 1) is divisible by both (x + a) and (x + b), then prove that

Solution:

Given Polynomial  (xⁿ + 1):-

(xⁿ+ 1) is divisible by (x + a).

(a)ⁿ + 10          [∵ x+a = 0 ⇒ x = -a]

or, (-a)ⁿ = 1…….…. (1)

Again, (xⁿ+1) is divisible by (x + b).

∴ (b)ⁿ + 1 = 0     [∵ x+b=0 ⇒ x = b]

or, (-b)ⁿ= -1……….(2)

From (1) and (2) we get,

(-a)ⁿ = (-b)ⁿ or, \(\frac{(-a)^n}{(-b)^n}=1\)

\(\text { or, }\left(\frac{-a}{-b}\right)^n=1 \text { or, }\left(\frac{a}{b}\right)^n=\left(\frac{a}{b}\right)^0\left[∵\left(\frac{a}{b}\right)^0=1\right]\)

or, n = 0,

∴ n = 0. (Proved)