Surface Tension Viscosity Definition and Examples

Surface Tension Viscosity

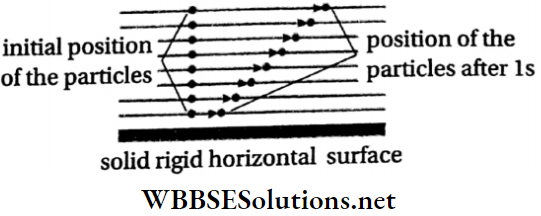
When a liquid flows slowly over a fixed horizontal surface, i.e., when the flow is laminar, the layer of the liquid in contact with the fixed surface remains at rest due to adhesion.

• The layer just above it moves slowly over the lower one, the third layer moves faster over the second one, and so on. The velocities of the layers of liquid increase with the increase in distance from the horizontal rigid surface.
• For two consecutive horizontal layers inside the liquid, the upper layer moves with a velocity greater than that of the lower one.
• The upper layer tends to accelerate the lower layer, while the lower layer tends to retard the upper one. In this way, the two adjacent layers tend to decrease their relative velocity—as if a tangential force acts on the upper layer and tries to oppose its motion.
• This tangential force is called viscous force. Therefore, to maintain a constant relative motion between the layers, an external force must act. If no external force acts, then the relative motion between the layers will cease and the flow of the liquid will stop.

Viscosity Definition: The property by virtue of which a liquid opposes the relative motion between its adjacent layers is called viscosity of the liquid.

Read and Learn More: Class 11 Physics Notes

Comparison of viscosity with friction: Viscosity is a general property of a fluid. The frictional force acting between two solid surfaces resembles in many ways the viscosity of a liquid.

• Hence, viscosity is called internal friction of a liquid. Like friction, the viscous force is absent if a liquid is at rest.
• The difference between the frictional force in solids and viscosity in liquids is that the viscous force depends on the area of liquid surface while the frictional force does not.

Viscosity and mobility of different liquids: Viscosities of different liquids are different. If alcohol and oil are poured separately into two identical vessels and stirred, then oil will come to rest earlier. This shows that the viscosity of oil is greater.

The greater the viscosity of a liquid, the lesser is its mobility. For example, the viscosity of honey is more than that of water and hence honey flows much slower than water. Coal tar has the least mobility.

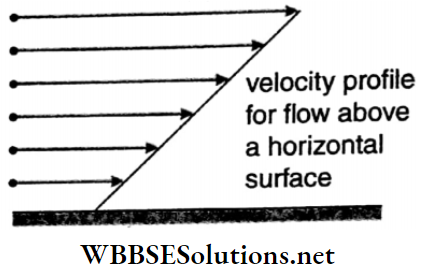
Velocity profile: The surface formed by joining the end points of the velocity vectors of different layers of any section of a flowing liquid is called its velocity profile. Velocity profile for flow above a horizontal surface is shown in Fig.

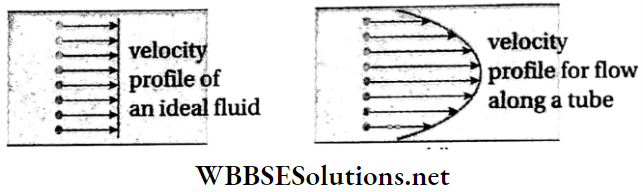
Velocity profile of a non-viscous liquid: An ideal liquid is non-viscous. For such a liquid, there is no resistance due to viscosity. The velocities of the different layers are the same.

Every particle in a given cross-section of the liquid moves forward with the same velocity. On joining the ends of these velocity vectors, we get a plane surface. Therefore, we can say that the velocity profile of a non-viscous liquid is linear (on 2D graph).

Velocity profile of a viscous liquid: When a viscous liquid flows through a horizontal tube, the layer of liquid in contact with the wall of the tube remains stationary due to adhesion. So the velocity of that layer is zero.

• The layer of the liquid which flows along the axis of the tube has the maximum velocity. As we progress from the centre towards the walls, the velocity decreases.
• Therefore, on joining the ends of the velocity vectors, we get a parabolic surface. The velocity profile of a viscous liquid is a parabola (on 2D graph).

Coefficient of Viscosity: Let PQ be a solid horizontal surface. A liquid is in streamline motion over the surface PQ. Two liquid surfaces CD and MN are at distances x and (x+dx) respectively from the fixed solid surface. The velocity of layer CD is ν and that of layer MN is ν+ dν.

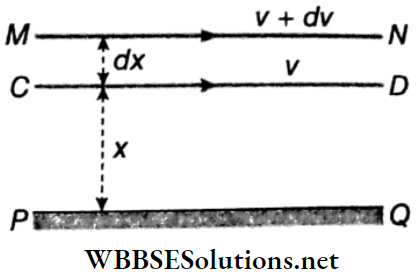
Due to the viscosity of the liquid, an opposing force acts between these two layers and tries to slow down the relative motion of the layers. If this opposing viscous force is F, then for streamline motion of the liquid, Newton proved that

1. F ∝ A; A = area of cross-section of the liquid surface, and
2. \(F \propto \frac{d v}{d x} ; \frac{d v}{d x}\) = velocity gradient = rate of change of velocity with distance perpendicular to the direction of flow.

∴ \(F \propto A \frac{d v}{d x} \text { or, } F=-\eta A \frac{d v}{d x}\) ….(1)

Here, η is a constant known as the coefficient of viscosity. Its value depends on the nature of the liquid.

Equation (1) is known as Newton’s formula for the streamline flow of a viscous liquid. Liquids that obey this law are called Newtonian liquids and liquids that do not obey this law are called non-Newtonian liquids.

From equation (1), we get, \(\eta=\frac{F}{A \frac{d v}{d x}}\)

If A = 1 and \(\frac{d v}{d x}=1\), then η = F; from this, we can define the coefficient of viscosity.

Coefficient of Viscosity Definition: The coefficient of viscosity of a liquid is defined as the required tangential force acting per unit area to maintain unit relative velocity between two liquid layers unit distance apart.

Units of coeffcient of viscocity: \(\eta=\frac{F}{A \frac{d v}{d x}}=\frac{F d x}{A d \nu}\)

So, unit of \(\eta=\frac{\mathrm{N} \cdot \mathrm{m}}{\mathrm{m}^2 \cdot\left(\mathrm{m} \cdot \mathrm{s}^{-1}\right)}=\mathrm{N} \cdot \mathrm{s} \cdot \mathrm{m}^{-2}=\mathrm{Pa} \cdot \mathrm{s}\)

Unit:

• dyn · s · cm^-2 CGS System or g · cm^-1 · s^-1
• N · s · m^-2 or Pa · s or kg · m^-1 · s^-1 SI

Relation: \(1 \mathrm{~kg} \cdot \mathrm{m}^{-1} \cdot \mathrm{s}^{-1}=\frac{1 \mathrm{~kg}}{1 \mathrm{~m} \times 1 \mathrm{~s}}=\frac{1000 \mathrm{~g}}{100 \mathrm{~cm} \times 1 \mathrm{~s}}\)

= 10 g · cm^-1 · s^-1

Poise and decompose: The coefficient of viscosity of a liquid is 1 poise, when a tangential force of 1 dyn is required to maintain a relative velocity of 1 cm · s^-1 between two parallel layers of the liquid 1 cm apart where each layer has an area of 1 cm².

So, 1 poise is the CGS unit of the coefficient of viscosity η.

1 poise = 1 dyn • s • cm^-2 = 1 g • cm^-1 • s^-1.

As, 1 kg · m^-1 · s^-1 = 10g · cm^-1 • s^-1 = 10 poise,

the SI unit of η is called 1 decapoise = 10 poise.

The coefficient of viscosity of a liquid is 1 decompose, when a tangential force of 1 newton is required to maintain a relative velocity of 1 m · s^-1 between two parallel layers separated by  distance of 1 m, where each layer has an area of 1 m².

Dimension of coefficient of viscosity: \([\eta]=\frac{[\mathrm{F}]}{[\mathrm{A}]\left[\frac{d \nu}{d x}\right]}=\frac{\mathrm{MLT}^{-2}}{\mathrm{~L}^2 \cdot \frac{\mathrm{LT}^{-1}}{\mathrm{~L}}}=\mathrm{ML}^{-1} \mathrm{~T}^{-1}\)

Effect of pressure and temperature on the coefficient of viscosity

Effect of pressure: Usually, viscosity increases with pressure. In less viscous liquids, the viscosity increases at a low rate with pressure.

• But for highly viscous liquids, an increase in pressure results in a rapid rise in its viscosity. However, water behaves differently and, with an increase in pressure, its viscosity decreases.
• From the kinetic theory of gases, it is known that a change in pressure does not affect the viscosity of a gas. But for a large increase (or decrease) in pressure, viscosity is affected.

Effect of temperature: Usually, the coefficient of viscosity of liquids decreases with a rise in temperature. The relation between temperature and coefficient of viscosity is rather complicated. One commonly used equation relating these two is

⇒ \(\eta_t=\frac{A}{(1+B t)^C}\)

where, η_t = coefficient of viscosity of a liquid at t°C and A, B, and C are constants for a particular fluid.

For gases, the coefficient of viscosity increases with an increase in temperature.

Critical Velocity and Reynolds Number

Critical velocity: The maximum velocity of a fluid, up to which the flow of the fluid is streamlined and beyond which the flow becomes turbulent, is regarded as the critical velocity for that fluid.

On gradually increasing the velocity of a fluid, the streamline flow does not become turbulent abruptly. Rather this change occurs gradually.

With the help of experimental demonstration and also by dimensional analysis, it can be proved that the critical velocity (ν_c) of a fluid is

1. Inversely proportional to the density (ρ) of the fluid,
2. Directly proportional to the coefficient of viscosity (η) of the fluid, and
3. Inversely proportional to the characteristic length (l) of the channel. So,

⇒ \(v_c \propto \frac{\eta}{\rho l} \text { or, } v_c=N_c \cdot \frac{\eta}{\rho l}\) …..(1)

In the case of a tube, the characteristic length is the diameter of the tube while, for a canal, the characteristic length is its breadth.

If, for a liquid, ρ and η are known and its critical velocity ν_c can be determined experimentally during its flow through a tube of diameter l, then from equation (1), the value of the constant N_c for that liquid can be determined. This value is nearly 2300.

For any velocity ν of the fluid flow, equation (1) can also be written in an equivalent form as

⇒ \(v=N \cdot \frac{\eta}{\rho l} \text { or, } N=\frac{\rho l v}{\eta}\)…….(1)

N is called the Reynolds number.

Special cases:

1. If ν<ν_c, i.e., the velocity of fluid flow is less than the critical velocity, then comparing equations (1) and (2), we can say that N<N_c. It means that the value of Reynolds number is less than 2300. So, if the value of Reynolds number is less than 2300, then the flow will be streamlined.

2. On the other hand, if ν>ν_c, i.e., the velocity of the fluid is greater than the critical velocity, then N >N_c, and hence the value of Reynolds number will be greater than 2300. If Reynolds number is greater than 2300, then the flow will be turbulent.

Dimension of Reynolds number: From equation (2) we get, dimension of N

= \(\frac{\text { dimension of } \rho \times \text { dimension of } l \times \text { dimension of } \nu}{\text { dimension of } \eta}\)

= \(\frac{M L^{-3} \cdot L \cdot L T^{-1}}{M L^{-1} T^{-1}}=1\)

So, N is a dimensionless quantity; it is a pure number.

Reynolds number: A dimensionless number N= \(\frac{\rho l v}{\eta}\) can be formed by combining the characteristic length (l) of a fluid channel and the velocity (v), density (ρ) and coefficient of viscosity (η) of the fluid the magnitude of N determines whether the fluid flow is streamlined or turbulent. This number N is called the Reynolds number.

• In the above discussion, 2300 is an approximate value of Nc. Usually, for N < 2000, the fluid flow is streamlined, and for N> 3000 the fluid flow is turbulent. If N lies between 2000 and 3000, the streamline flow of a fluid gradually changes into turbulent flow.
• In the above discussion, 2300 is an approximate value of N_c. Usually, for N < 2000, the fluid flow is streamlined, and for N > 3000 the fluid flow is turbulent. If N lies between 2000 and 3000, the streamline flow of fluid gradually changes into turbulent flow.
• As N is a pure number, its value does not depend on the system of units chosen. For a particular flow, the value of N remains the same.

If the radius of a tube of flow is considered, instead of its diameter, then the effective value is, N_c ≈ 1150.

Viscosity Numerical Example

Example: A plate of area 100 cm2 is floating on an oil of depth 2 mm. The coefficient of viscosity of oil is 15.5 poise. What horizontal force is required to move the plate horizontally with a velocity of 3 cm · s^-1?
Solution:

A plate of area 100 cm2 is floating on an oil of depth 2 mm. The coefficient of viscosity of oil is 15.5 poise.

The viscous force, F = \(\eta A \frac{d v}{d x}\)

Here, A = 100 cm², η = 15.5 poise,

dν = 3 cm · s^-1 and dx = 2 mm = 0.2 cm.

∴ F = 15.5 x 100 x 3/0.2 = 23250 dyn

So the required horizontal force is 23250 dyn.

Terminal Velocity of a Body in a Viscous Medium and Stokes’ Law: When a body falls through a viscous medium (liquid or gas), it drags a layer of the fluid adjacent to it due to adhesion. But fluid layers at a large distance from the body are at rest.

• As a result, there is relative motion between different layers of the fluid at different distances from the body. But the viscosity of the fluid opposes this relative motion.
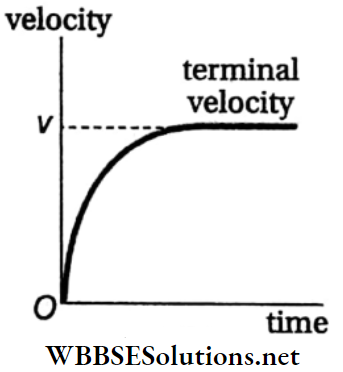
• The opposing force due to viscosity increases with increase in the velocity of the body due to the gravitational acceleration g. If the body is small in size, then after an interval of time the opposing upward force (i.e., viscous force and buoyant force) becomes equal to the downward force (weight of the body).
• Then the effective force acting on the body becomes zero and the body begins to fall through the medium with a uniform velocity, called the terminal velocity. A graph representing the change in velocity of a falling object with time is shown in Fig.

Stokes’ law: Stokes proved that, if a small sphere of radius r is falling with a terminal velocity ν through a medium of coefficient of viscosity η, then the opposing force acting on the sphere due to viscosity is

F = 6 πηrν ………..(1)

Equation (1) expresses Stokes.

1. To establish Stokes’ law, the following assumptions are
   made.
2. The fluid medium must be infinite and homogeneous. E3D The sphere must be rigid with a smooth surface.
3. The sphere must not slip when falling through the medium.
4. The fluid motion adjacent to the falling sphere must be streamlined.
5. The sphere must be small in size, but it must be greater than the intermolecular distance of the medium.

Equation for terminal velocity: if the density of the material of the sphere is ρ, then the weight of the sphere = \(\frac{4}{3} \pi r^3 \rho g .\)

If the density of the fluid medium is σ, then the upward buoyant force acting on the sphere = \(\frac{4}{3} \pi r^3 \sigma g\)

∴ The resultant downward force acting on the sphere =

= \(\frac{4}{3} \pi r^3 \rho g-\frac{4}{3} \pi r^3 \sigma g=\frac{4}{3} \pi r^3(\rho-\sigma) g\)….(2)

If the sphere attains terminal velocity, then

⇒ \(6 \pi \eta r \nu=\frac{4}{3} \pi r^3(\rho-\sigma) g \text { or, } \nu=\frac{2}{9} \frac{r^2(\rho-\sigma) g}{\eta}\) ….(3)

So, from equation (3), we see that the terminal velocity obeys the following rules.

1. Terminal velocity is directly proportional to the square of the radius of the sphere.
2. It is directly proportional to the difference of densities of the material of the sphere and that of the medium.
3. It is inversely proportional to the coefficient of viscosity of the medium.

If the density of the body is less than the density of the medium, i.e., ρ < σ, then it is clear that the terminal velocity becomes negative. Hence, the velocity of the body will be in the upward direction. For this reason, air or other gas bubbles move upwards through water.

Applications of Stokes’ law:

1. Falling of rain drops through air: Water vapour condenses on the particles suspended in air far above the ground to form tiny water droplets. The average radius of these tiny water droplets is 0.001 cm (approx.)

• Assuming the coefficient of viscosity of air as 1.8 x 10^-4  poise (approx.) the terminal velocity of these droplets is calculated as 1.2 cm · s^-1 (approx.) which is negligible. So, these water droplets float in the sky. Collectively these droplets form clouds.
• But as they coalesce to form larger drops, their terminal velocities increase. For example, the terminal velocity of a water droplet of radius 0.01cm becomes 120 cm · s^-1 (approx.). As a result, they cannot float any longer and so they come down as rain.

2. Coming down with the help of a parachute: When a soldier jumps from a flying airplane, he falls with acceleration due to gravity but due to viscous drag in air, the acceleration goes on decreasing till he acquires terminal velocity.

The soldier then descends with constant velocity and opens his parachute close to the ground at a pre-calculated moment, so that he may land safely near his destination.

Terminal Velocity Numerical Examples

Example 1. An oil drop of density 950 kg · m^-3 and radius 10^-6 m is falling through air. The density of air is 1.3 kg · m^-3 and its coefficient of viscosity is 181 x 10^-7 SI unit. Determine the terminal velocity of the oil drop, [g = 9.8 m · s^-2]
Solution:

An oil drop of density 950 kg · m^-3 and radius 10^-6 m is falling through air. The density of air is 1.3 kg · m^-3 and its coefficient of viscosity is 181 x 10^-7 SI unit.

Terminal velocity, \(\nu=\frac{2}{9} \cdot \frac{r^2(\rho-\sigma) g}{\eta}\)

[Here, ρ = 950 kg · m^-3 ; r = 10^-6 m; σ = 1.3 kg · m^-3; η = 181 x 10^-7 SI]

= \(\frac{2}{9} \cdot \frac{\left(10^{-6}\right)^2(950-1.3) \times 9.8}{181 \times 10^{-7}}\)

= \(1.14 \times 10^{-4} \mathrm{~m} \cdot \mathrm{s}^{-1}\)

Example 2. An air bubble of radius 1 cm is rising from the bottom of a long liquid column. If its terminal velocity is 0.21 cm · s^-1, calculate the coefficient of viscosity of the liquid. Given that the density of the liquid is 1.47 g · cm^-3. Ignore the density of air.
Solution:

An air bubble of radius 1 cm is rising from the bottom of a long liquid column. If its terminal velocity is 0.21 cm · s^-1

Coefficient of viscosity of the liquid, \(\eta=\frac{2}{9} \cdot \frac{r^2(\rho-\sigma) g}{v}\)

[Here, r = 1cm; v = -0.21 cm · s^-1; ρ = 0; cσ = 1.41 g · cm^-3]

= \(\frac{2}{9} \times \frac{(1)^2(0-1.47) \times 980}{-0.21}=1524.4 \text { poise. }\)