Simple Harmonic Motion Multiple Choice Questions And Answers

Simple Harmonic Motion

Question 1. A particle executing an SHM has a maximum acceleration of a and a maximum velocity of β. Then, its time period of oscillations will be

1. \(\frac{\beta^2}{\alpha^2}\)
2. \(\frac{\beta^2}{\alpha}\)
3. \(\frac{\alpha}{\beta}\)
4. \(\frac{2 \pi \beta}{\alpha}\)

Answer: 4. \(\frac{2 \pi \beta}{\alpha}\)

In an SHM, maximum acceleration = ω²A = α

and maximum velocity = ωA = β, where A = amplitude.

∴ \(\frac{\alpha}{\beta}=\omega=\frac{2 \pi}{T}\)

⇒ \(T=\frac{2 \pi \beta}{\alpha}\)

Question 2. Out of the following four functions, each representing the motion of a particle, which represents an SHM?

1. y = sin ω -cos ωt

2. y = 1 + ωt+ ω²t²

3. y = sin³ωt

4. \(y=A \cos \left(\frac{3 \pi}{4}-3 \omega t\right)\)

1. All except (2)
2. Both (1) and (4)
3. Both (1) and (3)
4. Only (1).

Answer: 2. Both (1) and (4)

For an SHM, \(\frac{d^2 y}{d t^2}=-k y\)

This is satisfied only by the given functions (1) and (4).

In anSHM, displacement = y = Asin(ω ± Φ).

(1) can also be expressed as

⇒ \(y=\sqrt{2} \sin \left(\omega t-\frac{\pi}{4}\right)\)

which is an SHM, and so is (4).

Question 3. A simple pendulum performs an SHM about x = 0 with an amplitude of a and a time period of T. The speed of its bob at x = \(\frac{a}{2}\) will be

1. \(\frac{\sqrt{3} \pi a}{2 T}\)
2. \(\frac{\pi a}{T}\)
3. \(\frac{3 \pi^2 a}{T}\)
4. \(\frac{\sqrt{3} \pi a}{T}\)

Answer: 4. \(\frac{\sqrt{3} \pi a}{T}\)

The speed at a distance x = \(\frac{a}{2}\) from the mean position (x = 0) is

⇒ \(v=\omega \sqrt{a^2-x^2}=\omega \sqrt{a^2-\frac{a^2}{4}}\)

=\(\frac{\sqrt{3}}{2} a \omega\)

=\(\frac{\sqrt{3}}{2}\left(\frac{2 \pi}{T}\right) a\)

=\(\frac{\sqrt{3} \pi a}{T}\)

Question 4. A particle executes an SHM with a time period of T. Its motion is represented by \(x=a \sin \left(\omega t+\frac{\pi}{6}\right)\) After the elapse of what fraction of time period, the velocity of the particle will be equal to half its maximum velocity?

1. \(\frac{T}{8}\)
2. \(\frac{T}{6}\)
3. \(\frac{T}{3}\)
4. \(\frac{T}{12}\)

Answer: 2. \(\frac{T}{6}\)

The instantaneous velocity is given by

⇒ \(v=\frac{d x}{d t}=a \omega \cos \left(\omega t+\frac{\pi}{6}\right)\)

Now, \(v=\frac{1}{2} v_{\max }=\frac{a \omega}{2}\)

⇒ \(\frac{a \omega}{2}=a \omega \cos \left(\omega t+\frac{\pi}{6}\right)\)

⇒ \(\frac{1}{2}=\cos \left(\omega t+\frac{\pi}{6}\right)=\cos \frac{\pi}{3}\)

⇒ \(\omega t=\frac{\pi}{3}-\frac{\pi}{6}=\frac{\pi}{6} .\)

∴ \(t=\frac{\pi}{6 \omega}\)

=\(\frac{\pi}{6\left(\frac{2 \pi}{T}\right)}\)

=\(\frac{T}{12}\)

Question 5. A particle executes an SHM with an amplitude of A and a time period of T. The minimum time taken by the particle to travel half its amplitude from the equilibrium position is

1. \(\frac{T}{4}\)
2. \(\frac{T}{8}\)
3. \(\frac{T}{12}\)
4. \(\frac{T}{2}\)

Answer: 3. \(\frac{T}{12}\)

In an SHM, x = \(A \sin \frac{2 \pi t}{T}\)

For \(x=\frac{A}{2}, \frac{A}{2}\)

=\(A \sin \frac{2 \pi t}{T}\)

⇒ \(\frac{1}{2}=\sin \frac{\pi}{6}\)

=\(\sin \frac{2 \pi t}{T}\)

∴ t = \(\frac{T}{12}\)

Question 6. Which of the following statements is true for the velocity (v) and the acceleration of a particle executing an SHM?

1. When v is maximum, a is maximum.
2. The value of a is zero for all values of u.
3. When v is zero, a is zero.
4. When v is maximum, a is zero.

Answer: 4. When v is maximum, a is zero.

In an SHM,| acceleration | = ω²x

and velocity = \(\omega \sqrt{A^2-x^2}\)

At the extreme position, x = ±A.

Now, \(|a|_{\max }=\omega^2 A \text { at } x= \pm A\)

⇒ \(|a|_{\min }=0 \quad \text { at } x=0 \text {, }\)

⇒ \(|v|_{\max }=\omega A \text { at } x=0\)

and \(|v|_{\min }=0 \quad \text { at } x= \pm A\)

Thus, v is maximum with acceleration = 0 at the equilibrium position
(x = 0).

Question 7. A simple harmonic oscillator has a displacement amplitude of a and a time period of T. The time required to travel from x = a to x = \(\frac{a}{2}\) is

1. \(\frac{T}{6}\)
2. \(\frac{T}{2}\)
3. \(\frac{T}{3}\)
4. \(\frac{T}{4}\)

Answer: 1. \(\frac{T}{6}\)

In an SHM, when we start counting time from the extreme position, the displacement is y = a cos at.

For \(y=\frac{a}{2}=a \cos \frac{2 \pi t}{T}\)

⇒ \(\frac{2 \pi t}{T}=\frac{\pi}{3}\)

⇒ \(t=\frac{T}{6}\)

Question 8. The angular velocity and the amplitude of a particle executing an SHM are co and A respectively. At a displacement of x from the mean position, the KE is K and the PE is U. Then, the ratio of K to U is equal to

1. \(\frac{A^2-\omega^2 x^2}{\omega^2 x^2}\)
2. \(\frac{x^2 \omega^2}{A^2-\omega^2 x^2}\)
3. \(\frac{A^2-x^2}{x^2}\)
4. \(\frac{x^2}{A^2-x^2}\)

Answer: 3. \(\frac{A^2-x^2}{x^2}\)

KE = \(K=\frac{1}{2} m v^2=\frac{1}{2} m \omega^2\left(A^2-x^2\right)\)

and \(\mathrm{PE}=U=\frac{1}{2} m \omega^2 x^2\)

∴ \(\frac{K}{U}=\frac{A^2-x^2}{x^2}\)

Question 9. A particle is executing an SHM along a straight line. Its velocities at the distances x₁ and x₂ from the mean position are v₁ and v₂ respectively. Its time period is

1. \(2 \pi \sqrt{\frac{x_1^2+x_2^2}{v_1^2+v_2^2}}\)
2. \(2 \pi \sqrt{\frac{x_2^2-x_1^2}{v_1^2-v_2^2}}\)
3. \(2 \pi \sqrt{\frac{v_1{ }^2+v_2{ }^2}{x_1{ }^2+x_2{ }^2}}\)
4. \(2 \pi \sqrt{\frac{v_1{ }^2-v_2{ }^2}{x_1^2-x_2{ }^2}}\)

Answer: 2. \(2 \pi \sqrt{\frac{x_2^2-x_1^2}{v_1^2-v_2^2}}\)

⇒ \(\text { At } x=x_1, v_1^2=\omega^2\left(A^2-x_1^2\right)\)…(1)

⇒ \(\text { At } x=x_2, v_2^2=\omega^2\left(A^2-x_2{ }^2\right)\)…(2)

Subtracting (2) from (1), we get

⇒ \(v_1^2-v_2^2=\omega^2\left(x_2^2-x_1^2\right)\)

⇒ \(\omega^2=\left(\frac{2 \pi}{T}\right)^2=\frac{v_1^2-v_2^2}{x_2^2-x_1^2}\)

∴ time period = \(T =2 \pi \sqrt{\frac{x_2^2-x_1^2}{v_1^2-v_2^2}}\)

Question 10. A particle executing an SHM with an amplitude of 5 m has a maximum speed of 31.4 cm s^-1. The frequency of its oscillations is

1. 3 Hz
2. 2 Hz
3. 4 Hz
4. 1 Hz

Answer: 4. 1 Hz

Maximum velocity = \(v_{\max }=A \omega=A\left(\frac{2 \pi}{T}\right)=A(2 \pi f)\)

∴ frequency = \(f=\frac{v_{\max }}{A \cdot 2 \pi}\)

=\(\frac{31.4 \mathrm{~cm} \mathrm{~s}^{-1}}{(5 \mathrm{~cm}) \cdot 2(3.14)}\)

=\(\frac{10 \pi}{10 \pi} \mathrm{s}^{-1}\)

= 1Hz.

Question 11. A particle executes a linear SHM with an amplitude of 3 cm. When the particle is at 2 cm from the mean position, the numerical magnitude of its velocity is equal to that of its acceleration. Then, the time period is

1. \(\frac{\sqrt{5}}{\pi} \mathrm{s}\)
2. \(\frac{\sqrt{5}}{2 \pi} \mathrm{s}\)
3. \(\frac{4 \pi}{\sqrt{5}} \mathrm{~s}\)
4. \(\frac{2 \pi}{\sqrt{3}} \mathrm{~s}\)

Answer: 3. \(\frac{4 \pi}{\sqrt{5}} \mathrm{~s}\)

Given that amplitude = A = 3 cm.

At x = 2 cm, v = a

⇒ \(\omega \sqrt{A^2-x^2}=\omega^2 x .\)

Substituting the values,

⇒ \(\omega \sqrt{(3 \mathrm{~cm})^2-(2 \mathrm{~cm})^2}=\omega^2(2 \mathrm{~cm})\)

⇒ \(\sqrt{5} \mathrm{~cm}=\frac{2 \pi}{T}(2 \mathrm{~cm})\)

∴ time period = \(T=\frac{4 \pi}{\sqrt{5}} \mathrm{~s}\)

Question 12. When two displacements represented by the equations y₁ = asinωt and y₂ = bcos ωt are superposed, the motion is

1. Not simple harmonic
2. Simple harmonic with an amplitude of \(\frac{a}{b}\)
3. Simple harmonic with an amplitude of \(\sqrt{a^2+b^2}\)
4. Simple harmonic with an amplitude of \(\frac{(a+b)}{2}\)

Answer: 3. Simple harmonic with an amplitude of \(\sqrt{a^2+b^2}\)

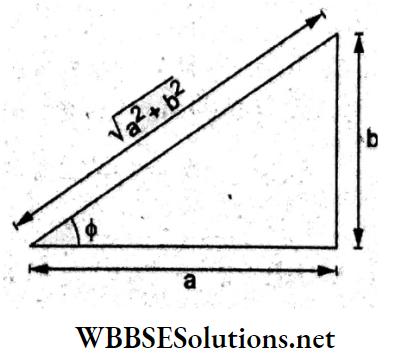
When the two SHMs are superposed, the resultant displacement is

y = y₁ + y₂ = a sin ωt + b cos ωt

\(\sqrt{a^2+b^2}\left(\frac{a}{\sqrt{a^2+b^2}} \sin \omega t+\frac{b}{\sqrt{a^2+b^2}} \cos \omega t\right)\)

⇒ \(\sqrt{a^2+b^2}(\sin \omega t \cos \phi+\cos \omega t \sin \phi)\)

As shown in the adjoining figure,

⇒ \(y=\sqrt{a^2+b^2} \sin (\omega t+\phi)\)

This represents an SHM with an amplitude of

A = \(A=\sqrt{a^2+b^2}\)

Question 13. Two simple harmonic motions of angular frequencies 100 rad s^-1 and 1000 rad s^-1 have the same displacement amplitude. The ratio of their maximum accelerations is

1. 1:10
2. 1: 10²
3. 1: 10³
4. 1: 10⁴

Answer: 2. 1: 10²

The maximum acceleration is given by \(|a|_{\max }=\omega^2 A.\)

⇒ \(\frac{\left|a_1\right|_{\max }}{\left|a_2\right|_{\max }}=\left(\frac{\omega_1}{\omega_2}\right)^2\)

=\(\left(\frac{100 \mathrm{rad} \mathrm{s}^{-1}}{1000 \mathrm{rad} \mathrm{s}^{-1}}\right)^2=\frac{1}{100}\)

Hence, the required ratio is 1: 10²

Question 14. Which of the following equations of motion represents an SHM, where c, D, and a are constants?

1. Acceleration = -cx + Dx²
2. Acceleration = -c(x + a)
3. Acceleration = c(x + a)
4. Acceleration = cx

Answer: 2. Acceleration = -c(x + a)

In an SHM, the restoring force (F) is directly proportional to the negative of the displacement from the mean position.

Thus, F = -kx.

Then, acceleration = \(\frac{F}{m}=-\frac{k x}{m}=-\omega^2 x\)

This condition for an SHM is also satisfied for acceleration = -c(x + a), whereflisa constant.

Question 15. A particle executes an SHM. When the displacements from the mean position are 4 cm and 5 cm, foe corresponding velocities of the foe particle are 10 cm s^-1 and 8 cm s^-1 respectively. The period of oscillation of the particle is

1. 2πs
2. \(\frac{\pi}{2} \mathrm{~s}\)
3. πs
4. \(\frac{3 \pi}{2} s\)

Answer: 3. πs

Given that x₁ = 4 cm, v₁ = 10 m s^-1, x₂ = 5 cm and v₂ = 8 cm s^-1.

∴ time period = \(T=2 \pi \sqrt{\frac{x_2^2-x_1^2}{v_1^2-v_2^2}}\)

Substituting the values, we have

⇒ \(T=2 \pi \sqrt{\frac{5^2-4^2}{10^2-8^2}} \mathrm{~s}\)

=\(2 \pi \sqrt{\frac{9}{36}} \mathrm{~s}=\pi \mathrm{s}\)

Question 16. A simple harmonic oscillator at a given time has a displacement of 0.02 m and an acceleration equal to 2.0 m s^-2. The angular frequency of the oscillator is

1. 100 rad s^-1
2. 1 rad s^-1
3. 10 rads^-1
4. 2 rad s^-1

Answer: 3. 10 rad s^-1

⇒ \(|a|=\omega^2 x\)

⇒ \(\omega=\sqrt{\frac{|a|}{x}}\)

=\(\sqrt{\frac{2.0 \mathrm{~m} \mathrm{~s}^{-2}}{0.02 \mathrm{~m}}}\)

= 10 rad s^-1

Question 17. If the maximum speed of a particle in an SHM (x = Asin ωt) is given by V₀, what is its average speed from t = 0 to t = \(\frac{T}{4}\)?

1. \(\frac{\pi v_0}{2}\)
2. \(\frac{2 v_0}{\pi}\)
3. \(\frac{\pi v_0}{4}\)
4. \(\frac{v_0}{\sqrt{2}}\)

Answer: 2. \(\frac{2 v_0}{\pi}\)

The instantaneous speed is given by v = Aω cos ωt = v₀ cos ωt.

∴ the average speed in the interval from t = 0 to t = \(\frac{T}{4}\) is

⇒ \(v_{\mathrm{av}}=\frac{1}{T / 4} \int v_0 \cos \omega t d t=\frac{4 v_0}{T}\left[\frac{\sin \omega t}{\omega}\right]_0^{T / 4}\)

=\(\frac{4 v_0}{T \omega}=\frac{2 v_0}{\pi}\)

Question 18. The time period of a simple pendulum kept in an artificial satellite orbiting the Earth is

1. πs
2. \(\frac{1}{\pi} \mathrm{s}\)s
3. Zero
4. Infinity

Answer: 4. Infinity

Any object inside an artificial satellite orbiting the earth is in a state of weightlessness, so g_eff = 0.

time period = T = \(2 \pi \sqrt{\frac{l}{g_{\text {eff }}}}\)

=\(2 \pi \sqrt{\frac{l}{0}}=\infty\)

Question 19. The period of oscillations of a spring-block system in an orbiting satellite is (take m = mass and k = spring constant)

1. Zero
2. Infinity
3. \(2 \pi \sqrt{\frac{m}{k}}\)
4. \(\sqrt{\frac{m g}{k}}\)

Answer: 3. \(2 \pi \sqrt{\frac{m}{k}}\)

The time period of oscillations of a spring-block system is given by \(T=2 \pi \sqrt{\frac{m}{k}}\) which is independent of the acceleration due to gravity (g).

In an orbiting satellite, even if the effective value of g is zero (condition of weightlessness), T will have the same value.

Question 20. The time period of a simple pendulum in a stationary lift is T. If the lift starts accelerating upwards with an acceleration of \(\frac{g}{3}\) the time period of the pendulum will be

1. √3T
2. \(\frac{T}{3}\)
3. \(\frac{\sqrt{3} T}{2}\)
4. \(\frac{T}{\sqrt{3}}\)

Answer: 3. \(\frac{\sqrt{3} T}{2}\)

The effective value of g in a lift accelerating upwards at a is g’ = g + a.

Here, a = \({g}{3}\) Hence,

⇒ \(T=2 \pi \sqrt{\frac{l}{g}}\)

and \(T^{\prime}=2 \pi \sqrt{\frac{l}{g+\frac{g}{3}}}\)

= \(\frac{\sqrt{3}}{2}\left(2 \pi \sqrt{\frac{l}{g}}\right)\)

= \(\frac{\sqrt{3} T}{2}\)

Question 21. Let an SHM be represented by the equation y = Asin 4πt + Bcos 4πt, where y, A, and B are in meters. The displacement amplitude of oscillations is

1. A + B
2. A-B
3. \(\sqrt{A^2+B^2}\)
4. \(\sqrt{A^2-B^2}\)

Answer: 3. \(\sqrt{A^2+B^2}\)

For an SHM, the displacement at a time t may be given by

x = a sin ωt + b cos ωt,

for which the displacement amplitude is \(\sqrt{a^2+b^2}\)

Here, y = A sin ωt + B cos ωt, where ω = 4π

∴ amplitude = \(\sqrt{A^2+B^2}\)

Question 22. A horizontal platform is executing an SHM in the vertical direction ’ with a frequency of f. A block of mass m is placed on the platform. What is the maximum amplitude of the platform so that the block is not detached from it?

1. \(\frac{g}{4 \pi^2 f^2}\)
2. \(\frac{g}{2 \pi^2 f^2}\)
3. \(\frac{m g}{2 \pi^2 f^2}\)
4. \(\frac{m g}{4 \pi^2 f^2}\)

Answer: 1. \(\frac{g}{4 \pi^2 f^2}\)

During the upward motion of the platform, the acceleration (a = -ω² x) acts downwards. At the uppermost extreme point, when the platform starts descending, acceleration changes its sign, so the weight (mg) and the force ma_max = mω²A act in opposite directions. For the block not to detach from the platform, mω²A ≤ mg.

∴ maximum amplitude = \(A_{\max }=\frac{g}{\omega^2}=\frac{g}{4 \pi^2 f^2}\)

Question 23. A block is placed on a rough horizontal platform. The platform is executing an SHM of time period T in the horizontal plane. The coefficient of static friction between the block and the platform is mmm The maximum amplitude of the platform for which the block does not slip on the surface of the platform is

1. \(\frac{\mu g T}{2 \pi}\)
2. \(\frac{\mu g T^2}{2 \pi^2}\)
3. \(\frac{\mu g T^2}{4 \pi^2}\)
4. μgT²

Answer: 3. \(\frac{\mu g T^2}{2 \pi^2}\)

The force of limiting friction is f_max = μN = mμg.

For the block not to slip at the extreme position, f_max > mω²A.

For the maximum amplitude,

⇒ \(m \omega^2 A_{\max }=f_{\max }=\mu m g\)

⇒ \(A_{\max }=\frac{\mu g}{\omega^2}=\frac{\mu g T^2}{4 \pi^2}\)

Question 24. A tunnel is drilled along the diameter of the earth and a stone is dropped into the tunnel. Then,

1. The stone will reach the center of the earth and stop there
2. The stone will reach the other end of the earth and stop there
3. The stone will execute an SHM about the center of the earth
4. The stone will reach the other side of the tunnel and escape the gravitational pull of the earth

Answer: 3. The stone will execute an SHM about the center of the earth

The speed of the stone goes on increasing and gets its maximum value at the center of the earth. Due to inertia, it moves further away from the centre, and the speed goes on decreasing to become zero at the other end. Thus, the stone undergoes an SHM with the centre as the origin, the radius R as the amplitude, and 2R as the path length.

Question 25. In the preceding problem, if R is the radius of the earth and g is the acceleration due to gravity at the earth’s surface, the period of oscillations of the stone will be

1. \(\frac{R}{g}\)
2. \(2 \sqrt{R g}\)
3. \(2 \pi \sqrt{\frac{R}{g}}\)
4. \(\frac{1}{2 \pi} \sqrt{\frac{R}{g}}\)

Answer: 3. \(2 \pi \sqrt{\frac{R}{g}}\)

Let M = mass of the earth and R = radius of the earth. When the stone during its fall be at P, where OP = x, the force due to gravity is

⇒ \(F=\frac{G(\text { mass of the inner core }) \cdot m}{O P^2}\)

⇒ \(\frac{G m}{x^2} \cdot \frac{4}{3} \pi x^3 \cdot \frac{M}{\frac{4}{3} \pi R^3}\) [∵ \(\text { density }=\frac{M}{\frac{4}{3} \pi R^3}\)]

⇒ \(\frac{G M m x}{R^3}\)

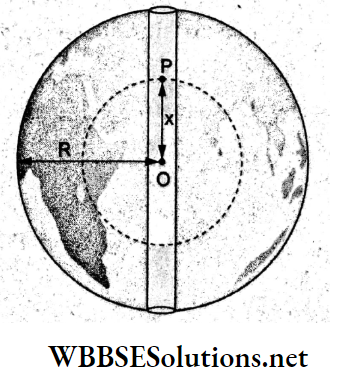
∴ acceleration = \(a=\frac{F}{m}=-\frac{G M x}{R^3}\) [∵ f and x are in opposite directions]

⇒ \(-\frac{g x}{R}\)

Thus, a ∝ -x.

Hence, the motion is an SHM for which

⇒ \(\omega^2=\frac{g}{R}\)

⇒ \(\omega=\frac{2 \pi}{T}=\sqrt{\frac{g}{R}}\)

⇒ \(T=2 \pi \sqrt{\frac{R}{g}}\)

Question 26. The time period of a simple pendulum of infinite length is

1. Infinity
2. \(2 \pi \sqrt{\frac{R}{g}}\)
3. \(2 \pi \sqrt{\frac{2 R}{g}}\)
4. \(2 \pi \sqrt{\frac{R}{2 g}}\)

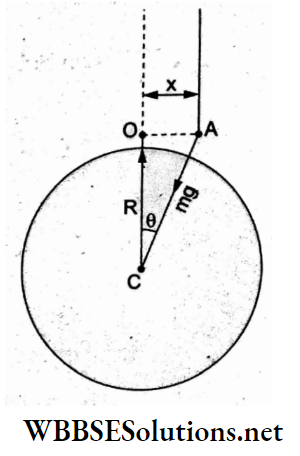
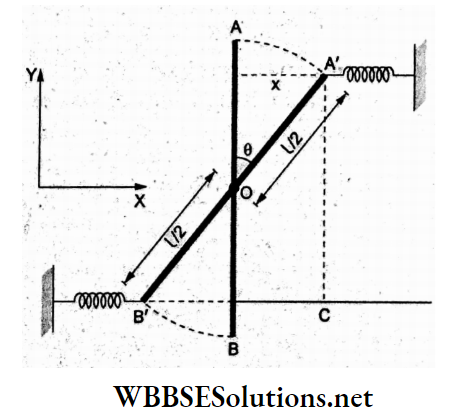
Answer: 2. \(2 \pi \sqrt{\frac{R}{g}}\) Let the thread of infinite length be attached to the pendulum bob of mass m. When the bob is displaced from O to A, the weight (mg) acts radially along AC, whose component along AO \(\left(m g \sin \theta=m g \frac{O A}{A C}=\frac{m g}{R} \cdot x\right)\) provides the restoring force for the SHM.

Thus, the acceleration is

⇒ \(a=\frac{F}{m}=-\frac{g}{R} \cdot x\)….(1)

Note that the minus sign is due to the force along AO and the displacement along OA. Further, threads have been shown parallelly as die threads are of infinite lengths.

Comparing (1) with the acceleration in an SHM (a = -ω²x), we have

⇒ \(\omega^2=\frac{g}{R} \Rightarrow T=2 \pi \sqrt{\frac{R}{g}}\)

Question 27. A cubical block of wood (density = p_w) of length I and mass M floats in a liquid,(density = p_liq). It is slightly pressed vertically and then released. It will execute an SHM. The period of oscillation is

1. \(2 \pi \sqrt{\frac{L \rho_{\text {liq }}}{\rho_w g}}\)
2. \(2 \pi \sqrt{\frac{M}{\rho_{\mathrm{w}} g}}\)
3. \(2 \pi \sqrt{\frac{L \rho_{\mathrm{w}}}{\rho_{\mathrm{liq}} g}}\)
4. \(2 \pi \sqrt{\frac{L}{g}}\)

Answer: 3. \(2 \pi \sqrt{\frac{L \rho_{\mathrm{w}}}{\rho_{\mathrm{liq}} g}}\)

Let the wooden block be displaced vertically down by x so that the force of buoyancy, or upthrust, \(\left(L^2 x\right) \rho_{\mathrm{liq}} g\) acts upwards.

∴ \(F=M a=-\left(L^2 \rho_{\text {liq }} g\right) x\)

⇒ \(a=-\left(\frac{L^2 \rho_{\mathrm{liq}} g}{M}\right) x=-\left(\frac{\rho_{\mathrm{liq}} g}{L \rho_{\mathrm{w}}}\right) x\)

[∵ M = L³p_w]

Since a ∝ -X, the motion is an SHM, whose time period is

⇒ \(T=2 \pi \sqrt{\frac{L \rho_{\mathrm{w}}}{\rho_{\mathrm{liq} g} g}}\)

Question 28. A particle executing an SHM has its KE = K₀ COS²ωt. The maximum values of the potential energy and the total energy are respectively

1. 0 and 2K₀
2. \(\frac{K_0}{2} \text { and } K_0\)
3. K₀ and K₀
4. K₀ and 2K₀

Answer: 3. K₀ and K₀

KE = K₀ COS² ωt, so the maximum KE is K₀.

Since the total energy is conserved,

KE_max = PE_max = total energy = K₀.

∴ PE_max = K₀ and total energy = K₀.

Question 29. The potential energy of a simple harmonic oscillator when the particle is halfway to its end point is (where E is the total energy)

1. \(\frac{E}{2}\)
2. \(\frac{E}{4}\)
3. \(\frac{2E}{3}\)
4. \(\frac{E}{8}\)

Answer: 2. \(\frac{E}{4}\)

At a distance x from the mean position, PE = \(\frac{1}{2} m \omega^2 x^2\)

For \(x=\frac{A}{2}, \mathrm{PE}=\frac{1}{2} m \omega^2 \frac{A^2}{4}\)

=\(\frac{1}{4}\left(\frac{1}{2} m \omega^2 A^2\right)\)

=\(\frac{\text { total energy }}{4}\)

=\(\frac{E}{4}\)

Question 30. In an SHM, when the displacement is half the displacement amplitude, what fraction of the total energy is kinetic?

1. \(\frac{1}{2}\)
2. \(\frac{1}{4}\)
3. \(\frac{3}{4}\)
4. \(\frac{2}{3}\)

Answer: 3. \(\frac{3}{4}\)

The KE in an SHM is \(\frac{1}{2} m \omega^2\left(A^2-x^2\right)\)

AT \(x=\frac{A}{2}, \mathrm{KE}=\frac{1}{2} m \omega^2\left(A^2-\frac{A^2}{4}\right)\)

=\(\frac{3}{4}\left(\frac{1}{2} m \omega^2 A^2\right)\)

⇒ \(\frac{3}{4}(\text { total energy })\)

=\(\frac{3 E}{4}\)

∴ \(\frac{\mathrm{KE}}{\text { total energy }}=\frac{\frac{3 E}{4}}{E}\)

=\(\frac{3}{4}\)

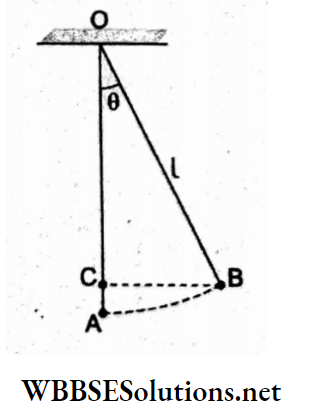
Question 31. A simple pendulum is displaced to an angle from its equilibrium position and then released. While passing through the equilibrium position, its velocity v will be

1. \(\sqrt{2 g l}\)
2. \(\sqrt{2 g l \cos \theta}\)
3. \(\sqrt{2 g l \sin \theta}\)
4. \(\sqrt{2 g l(1-\cos \theta)}\)

Answer: 4. \(\sqrt{2 g l(1-\cos \theta)}\)

When released from the angular position 0, the loss in PE is

mg(CA) = mg(OA-OC)

= mg(l-l cosθ)

= mgl(1-cosθ).

This loss in PE appears as KE = \(\frac{1}{2}\) mv2.

∴ \(\frac{1}{2}\)mv²= mgl(1-cos θ)

⇒ \(v=\sqrt{2 g l(1-\cos \theta)}\)

Question 32. The displacement of a particle (executing an SHM) between the positions of the maximum PE and the maximum KE are (A being the displacement amplitude)

1. \(\pm \frac{A}{2}\)
2. ±A
3. ±2A
4. ±1

Answer: 2. ±2A

In an SHM, the maximum KE is obtained at x = 0, and the position for the maximum PE is x = ±A.

Hence, the required displacement is ±A.

Question 33. In an SHM, the restoring force is F = -kx, where k is the force constant, x is the displacement from the mean position and a is the amplitude. Then, the total mechanical energy depends upon

1. k, a and m
2. k, x and m
3. k and a
4. k and x

Answer: 3. k and a

Since F = -kx, the potential energy is

⇒ \(U=-W\)

⇒ \(=-\int d W\)

⇒ \(=-\int F d x\)

⇒ \(=-\int_0^x(-k x) d x\)

⇒ \(=\frac{1}{2} k x^2\)

∴ total mechanical energy = E = maximum PE

⇒ \(\frac{1}{2} k x^2\)

=\(\frac{1}{2} k a^2\) [∵ x = a]

Thus, the total energy depends on k and a.

Question 34. A force of 6.4 N stretches a vertical spring by 0.1 m. The mass that must be suspended from the spring s6 that it oscillates with a period of (π/4) s is

1. \(\frac{\pi}{4} \mathrm{~kg}\)
2. 1 kg
3. \(\frac{1}{\pi} \mathrm{kg}\)
4. 10 kg

Answer: 2. 1 kg

Since F = kx, the force constant is

⇒ \(k=\frac{F}{x}=\frac{6.4 \mathrm{~N}}{0.1 \mathrm{~m}}=64 \mathrm{~N} \mathrm{~m}^{-1}\)

The time period of the spring-block system is given by

⇒ \(T=2 \pi \sqrt{\frac{m}{k}} \Rightarrow \frac{\pi}{4} s=2 \pi \sqrt{\frac{m}{64}}=\frac{2 \pi \sqrt{m}}{8}\)

m = 1 kg.

Question 35. The periodic time of oscillations of a spring-block system of mass M is T. If the mass is increased to 2M, the time of oscillations T will be equal to

1. 2T
2. √2T
3. \(\frac{T}{\sqrt{2}}\)
4. T

Answer: 2. √2T

For a spring-block system,

⇒ \(T=2 \pi \sqrt{\frac{M}{k}}\)

and \(T^{\prime}=2 \pi \sqrt{\frac{2 M}{k}}\)

= \(\sqrt{2}\left(2 \pi \sqrt{\frac{M}{k}}\right)\)

= \(\sqrt{2} T\)

Question 36. A block of mass m is attached to the lower end of a spring whose upper end is fixed to a rigid support. The spring has a negligible mass. When the block is slightly pulled down and released it oscillates with a time period of 3 s. When the mass is increased by 1 kg, the time period of oscillations increases to 5 s. The value of mis

1. \(\frac{3}{4}\) kg
2. \(\frac{4}{3}\) kg
3. \(\frac{16}{9}\) kg
4. \(\frac{9}{16}\) kg

Answer: 4. \(\frac{9}{16}\) kg

With mass = m, T = \(2 \pi \sqrt{\frac{m}{k}}\)

= 3s.

With mass = m + 1 kg,

⇒ \(T^{\prime}=2 \pi \sqrt{\frac{m+1 \mathrm{~kg}}{k}}\)

= 5s.

∴ \(\frac{T^{\prime}}{T}=\frac{5 \mathrm{~s}}{3 \mathrm{~s}}\)

=\(\sqrt{\frac{m+1 \mathrm{~kg}}{m}}\)

⇒ \(m=\frac{9}{16} \mathrm{~kg}\)

Question 37. A block of mass fn is suspended vertically from a light spring which oscillates with a frequency f. With what frequency will the system oscillate if another block of mass 4m is suspended from the same spring?

1. \(\frac{f}{4}\)
2. 4f
3. \(\frac{f}{2}\)
4. 2f

Answer: 3.

Time period = \(T=2 \pi \sqrt{\frac{m}{k}}, \text { so frequency }=f=\frac{1}{T}\)

=\(\frac{1}{2 \pi} \sqrt{\frac{k}{m}}\)

When the mass is changed from m to 4m, the frequency becomes

⇒ \(f^{\prime}=\frac{1}{2 \pi} \sqrt{\frac{k}{4 m}}\)

=\(\frac{1}{2}\left(\frac{1}{2 \pi} \sqrt{\frac{k}{m}}\right)\)

=\(\frac{f}{2}\)

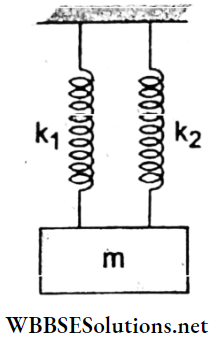
Question 38. A mass m is suspended successively by two springs of force constants and separately. The time periods of oscillations in die two cases are T₁ and T₂ respectively. If the same mass is suspended by connecting the two springs in parallel (as shown in the figure), the time period of oscillations is T. The correct relation is

1. T₂ = T₁² + T₂²
2. \(\frac{1}{T}=\frac{1}{T_1}+\frac{1}{T_2}\)
3. T = T₁ + T₂
4. \(T^{-2}=T_1^{-2}+T_2^{-2}\)

Answer: 4. \(T^{-2}=T_1^{-2}+T_2^{-2}\)

With the first spring, \(T_1=2 \pi \sqrt{\frac{m}{k_1}}\)

With the second spring, \(T_2=2 \pi \sqrt{\frac{m}{k_2}}\)

With the two springs in parallel, the equivalent spring constant is k = k₁ + k₂, so the time period is

⇒ \(T=2 \pi \sqrt{\frac{m}{k_1+k_2}}\)

∴ \(\frac{1}{T_1^2}=\frac{1}{4 \pi^2} \cdot \frac{k_1}{m} \text { and } \frac{1}{T_2^2}=\frac{1}{4 \pi^2} \cdot \frac{k_2}{m}\)

∴ \(\frac{1}{T^2}=\frac{1}{4 \pi^2} \cdot \frac{k_1+k_2}{m}=\frac{1}{T_1^2}+\frac{1}{T_2^2}\)

⇒ \(T^{-2}=T_1^{-2}+T_2^{-2}\)

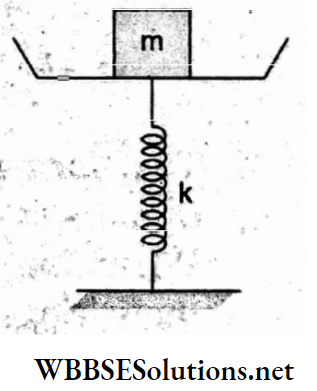
Question 39. A block having mass m = 2 kg is placed on a flat pan attached to a vertical spring fixed on the ground, as shown in the figure. The combined mass of the pan and the spring is negligible. When pressed slightly and released, the bloc executes an SHM. The spring constant is k = 200 N m^-1. What should be the minimum amplitude of the motion so that the block gets detached from the pan? (Take g = 10 m s^-2.)

1. 8.0 cm
2. 10.0 cm
3. 4.0 cm
4. Any value less than 12 cm

Answer: 2. 10.0 cm

The pan (as a frame) while moving upwards gets decelerated, i.e., its acceleration (a = -ω²x) is directed downwards. So, the pseudo force is

⇒ \(\vec{F}_{\mathrm{ps}}=(\text { mass of the block })\left(-\vec{a}_{\mathrm{fr}}\right)\)

which is vertically upwards.

Maximum pseudoforce = ma_max = mω²A,

where A is the displacement amplitude.

For no detachment, m ω²A < mg. So, the minimum amplitude is

⇒ \(A=\frac{g}{\omega^2}=\frac{g}{\frac{k}{m}}=\frac{m g}{k}\)

Substituting the values,

⇒ \(A=\frac{(2 \mathrm{~kg})\left(10 \mathrm{~m} \mathrm{~s}^{-2}\right)}{200 \mathrm{~N} \mathrm{~m}^{-1}}\)

= 0.1 m

= 10.0 cm.

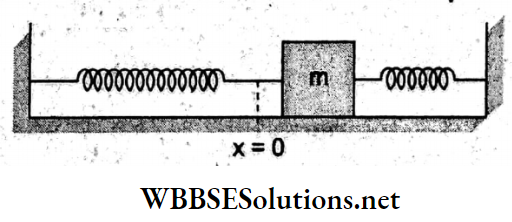
Question 40. What is the expression for the time period (T) of the block-spring system executing an SHM smooth horizontal surface?

1. \(T=2 \pi \sqrt{\frac{m}{k_1+k_2}}\)
2. \(T=2 \pi \sqrt{\frac{k_1+k_2}{m}}\)
3. \(T=2 \pi \sqrt{\frac{k_1 k_2 m}{k_1+k_2}}\)
4. \(T=2 \pi \sqrt{\frac{\left(k_1+k_2\right) m}{k_1 k_2}}\)

Answer: 1. \(T=2 \pi \sqrt{\frac{m}{k_1+k_2}}\)

Let the block be displaced on either side by x so that the first spring gets extended by x and the second one gets compressed by x as shown in the adjoining figure.

The net force towards the left is (k₁ + k₂)x.

⇒ \(|a|=\frac{F}{m}=\left(\frac{k_1+k_2}{m}\right) x\)

=\(\omega^2 x\)

⇒ \(T=\frac{2 \pi}{\omega}\)

=\(2 \pi \sqrt{\frac{m}{k_1+k_2}}\)

Question 41. In the adjoining figure, two blocks, each of mass m, are connected to the two ends of a light spring of force constant K. Thereisnofriction anywhere. If the blocks are displaced slightly in opposite directions and then released, they will execute an SHM with a frequency f, which is equal to

1. \(\frac{1}{2 \pi} \sqrt{\frac{k}{2 m}}\)
2. \(\frac{1}{2 \pi} \sqrt{\frac{2 k}{m}}\)
3. \(\frac{1}{2 \pi} \sqrt{\frac{m}{k}}\)
4. \(\frac{1}{2 \pi} \sqrt{\frac{k}{m}}\)

Answer: 2. \(\frac{1}{2 \pi} \sqrt{\frac{2 k}{m}}\)

In the absence of external forces, the center of mass of the system (lying at the center of the spring) will remain fixed. For the force constant k of a half spring,

⇒ \(k^{\prime}\left(\frac{l}{2}\right)=k l \Rightarrow k^{\prime}=2 k\)

With the mass m and the spring constant k – 2k, the time period is,

⇒ \(T=2 \pi \sqrt{\frac{m}{2 k}}\)

and the frequency is,

⇒ \(f=\frac{1}{2 \pi} \sqrt{\frac{2 k}{m}}\)

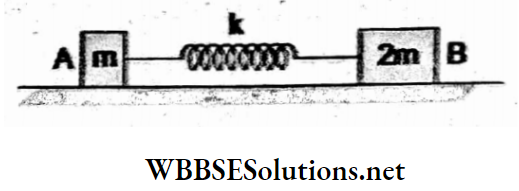
Question 42. Two blocks A and B of respective masses m and 2m are attached to the two ends of a light spring of force constant k. If the blocks are pulled apart in opposite directions and released, the system of the blocks oscillates with a time period T. Find the expression for T. (Assume the friction to be negligible.)

1. \(2 \pi \sqrt{\frac{2 m}{k}}\)
2. \(2 \pi \sqrt{\frac{m}{k}}\)
3. \(2 \pi \sqrt{\frac{2 m}{3 k}}\)
4. \(2 \pi \sqrt{\frac{m}{2 k}}\)

Answer: 3. \(2 \pi \sqrt{\frac{2 m}{3 k}}\)

Taking block A as the origin, the position of the centre of mass of the system is

⇒ \(x_{\mathrm{CM}}=\frac{m \cdot 0+2 m \cdot l}{m+2 m}=\frac{2}{3} l\)

This position remains fixed in the absence of external forces.

The equivalent force constant is

⇒ \(\left(\frac{2}{3} l\right) k^{\prime}=l \cdot k\)

⇒ \(k^{\prime}=\frac{3}{2} k\)

∴ time period = \(T=2 \pi \sqrt{\frac{m}{k^{\prime}}}\)

=\(2 \pi \sqrt{\frac{2 m}{3 k}}\)

Question 43. Two springs of force constants k₁ are connected to a block of mass m placed on a frictionless surface, as shown in the figure. When the force constants of both the springs have the same value k, the frequency of oscillations of the block is

1. \(\frac{1}{2 \pi} \sqrt{\frac{k}{m}}\)
2. \(\frac{1}{2 \pi} \sqrt{\frac{k}{2 m}}\)
3. \(\frac{1}{2 \pi} \sqrt{\frac{2 k}{m}}\)
4. \(\frac{1}{2 \pi} \sqrt{\frac{m}{k}}\)

Answer: 2. \(\frac{1}{2 \pi} \sqrt{\frac{k}{2 m}}\)

For die given system of springs connected in series, the equivalent spring constant V is given by

⇒ \(\frac{1}{k^{\prime}}=\frac{1}{k_1}+\frac{1}{k_2}=\frac{k_1+k_2}{k_1 k_2}\)

⇒ \(k^{\prime}=\frac{k_1 k_2}{k_1+k_2}\)

∴ frequency = \(f=\frac{1}{2 \pi} \sqrt{\frac{k^{\prime}}{m}}=\frac{1}{2 \pi} \sqrt{\frac{k_1 k_2}{\left(k_1+k_2\right) m}}\)

When \(k_1=k_2=k, f=\frac{1}{2 \pi} \sqrt{\frac{k}{2 m}}\)

Question 44. In the preceding question, the frequency of oscillations of the block of mass m connected to a single spring horizontally is 4 Hz. When the spring is replaced by two identical springs, as shown in the figure, die effective frequency will be

1. 4√2 Hz
2. 1.5 Hz
3. 1.31 Hz
4. 2√2 Hz

Answer: 4. 2√2 Hz

The frequency of the spring-block system is \(f=\frac{1}{2 \pi} \sqrt{\frac{k}{m}}, \text { so } f \propto \sqrt{k}\)

With one single spring, f ∝ Vk.

With two identical springs in series,

⇒ \(f^{\prime} \propto \sqrt{\frac{k}{2}}\)

∴ \(\frac{f^{\prime}}{f}=\frac{\sqrt{\frac{k}{2}}}{\sqrt{k}}\)

⇒ \(\frac{1}{\sqrt{2}}\)

⇒ \(f^{\prime}=\frac{1}{\sqrt{2}} f\)

=\(\frac{1}{\sqrt{2}}(4 \mathrm{~Hz})\)

=\(2 \sqrt{2} \mathrm{~Hz}\)

Question 45. Four massless springs, whose force constants are 2k, 2k, k, and 2k, are attached to a block of mass M as shown in the figure. If the block is displaced in the horizontal direction to either 2k side released, the frequency of oscillations of the block is

1. \(\frac{1}{2 \pi} \sqrt{\frac{k}{7 M}}\)
2. \(\frac{1}{2 \pi} \sqrt{\frac{k}{4 M}}\)
3. \(\frac{1}{2 \pi} \sqrt{\frac{4 k}{M}}\)
4. \(\frac{1}{2 \pi} \sqrt{\frac{7 k}{M}}\)

Answer: 3. \(\frac{1}{2 \pi} \sqrt{\frac{4 k}{M}}\)

The equivalent force constant to the left of the block is k (for the series combination) and that to the right is 2k + k = 3k. These taken together constitute a parallel combination for which the force constant is

k_eq = k + 3k = 4k and thus frequency = \(f=\frac{1}{2 \pi} \sqrt{\frac{k_{\text {eq }}}{M}}=\frac{1}{2 \pi} \sqrt{\frac{4 k}{M}}\)

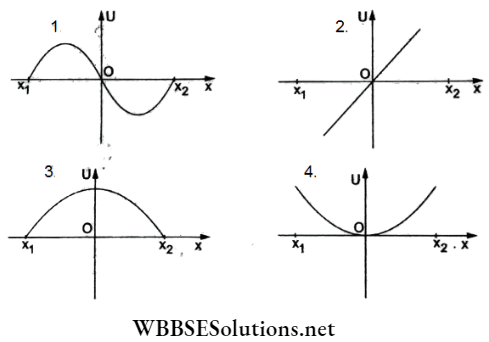
Question 46. A particle executes an SHM between the extreme positions x = x₁ and x = x₂, the equilibrium position being O. Which of the following graphs correctly represents the variation of potential energy with a position?

Answer: 4.

In an SHM, potential energy \(U=\frac{1}{2} k x^2=\frac{1}{2} m \omega^2 x^2\)

This is positive for both negative and positive values of x, and zero at the mean position (x = 0). This is given in the option (d).

Question 47. Which of the following graphs correctly represents the variation of acceleration with time (t) for a particle executing a simple harmonic motion for which the displacement is x = Acos ωt?

Answer: 3.

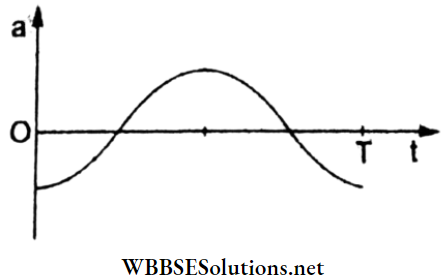
The given expression for displacement in the SHM is x = Acos ωt, represented in the adjoining graph 1. On differentiation, we get acceleration

⇒ \(a=\frac{d^2 x}{d t^2}=-\omega^2 A \cos \omega t\)

= -a₀ cos ωt.

This is just the mirror image of Graph 1 about the central line (x = 0), as shown in Graph 2.

Question 48. The variation of the acceleration of a particle in an SHM with its displacement (x) is shown by the graph

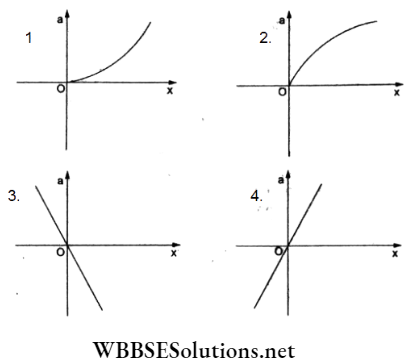
Answer: 3.

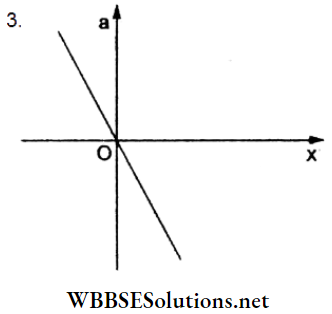
The acceleration in an SHM is given by a ∝ -x

=> a = -kx.

The a-x graph is a straight line with a negative slope and passing through the origin, as shown in the option (3)

Question 49. The displacement of a particle from the mean position is given by y = asin 4π(2t + 0.4). The time period of oscillations is

1. 0.25 s
2. 0.2s
3. 1.0 s
4. 2.0 s

Answer: 1. 0.25 s

In the given equation,

y = asin4 π(2t + 0.4)

= a sin(8πt +1.6π) = a sin(ωt + 0).

Thus, to = \(\frac{2 \pi}{T}\) = 8πt

T = \(\frac{1}{4}\) s

= 0.25 s.

Question 50. A particle executes an SHM such that its acceleration is given by a = -bx, where x is its displacement from the mean position. The time period of oscillations is

1. 2π√b
2. \(\frac{2 \pi}{\sqrt{b}}\)
3. \(\frac{2 \pi}{b}\)
4. \(\sqrt{\frac{2 \pi}{b}}\)

Answer: 2. \(\frac{2 \pi}{\sqrt{b}}\)

For an SHM, acceleration-a = -ω²x.

Comparing this with the given equation a = -bx, we get

⇒ \(\omega^2=b \Rightarrow \omega=\frac{2 \pi}{T}=\sqrt{b}\)

⇒ \(T=\frac{2 \pi}{\sqrt{b}}\)

Question 51. A particle executes an SHM with a frequency f. The frequency with which its KE oscillates is

1. \(\frac{f}{2}\)
2. f
3. 2f
4. 4f

Answer: 3. 2f

For an SHM, x = asin ωt

v = aω cos ωt

and KE = E = \(\frac{1}{2}\) mv²

From (1), the frequency of oscillations is to, while. the frequency of variation of KE is 2

This is also clear in the given graph. The x-t graph completes one cycle in the time period T, whereas KE completes two complete oscillations (one from O to \(\frac{T}{2}\) and the other from \({T}{2}\) to T.

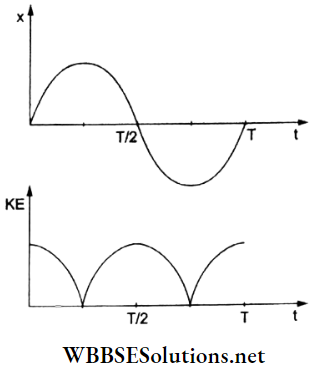
Question 52. The displacement of a particle executing an SHM is expressed by the equation y = A₀ + A sin ωt + B cos ωt. Then, the amplitude of its oscillations is

1. \(A_0+\sqrt{A^2+B^2}\)
2. \(\sqrt{A^2+B^2}\)
3. \(\sqrt{A_0^2+(A+B)^2}\)
4. A + B

Answer: 2. \(\sqrt{A^2+B^2}\)

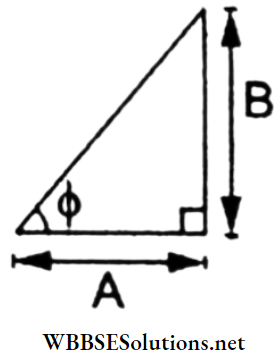
The given equation represents an SHM with a displaced origin of displacement amplitude \(\), as shown below.

y= A₀+ A sin ωt + Bcos ωt

⇒ \(A_0+\sqrt{A^2+B^2}\left(\frac{A}{\sqrt{A^2+B^2}} \sin \omega t+\frac{B}{\sqrt{A^2+B^2}} \cos \omega t\right)\)

⇒ \(A_0+\sqrt{A^2+B^2}(\sin \omega t \cos \phi+\cos \omega t \sin \phi)\)

⇒ \(A_0+\sqrt{A^2+B^2} \sin (\omega t+\phi)\)

where \(\phi=\tan ^{-1}\left(\frac{B}{A}\right)\) from the triangle.

The amplitude \(\sqrt{A^2+B^2}\) is always associated with the sinusoidal function.

Question 53. The average velocity of a particle executing an SHM in one r complete oscillation is

1. \(\frac{A \omega}{2}\)
2. Aω
3. \(\frac{A \omega^2}{2}\)
4. Zero

Answer: 4. Zero

An SHM is expressed by y = Asin ωt and its velocity is given by

⇒ \(v=\frac{d y}{d t}=A \omega \cos \omega t\)

We know that the average value of any sinusoidal function (sine, cosine or any of their combinations) in one complete cycle (t = 0 to t = T) is always zero.

So, v = Aω cos ωt being sinusoidal has a zero average velocity

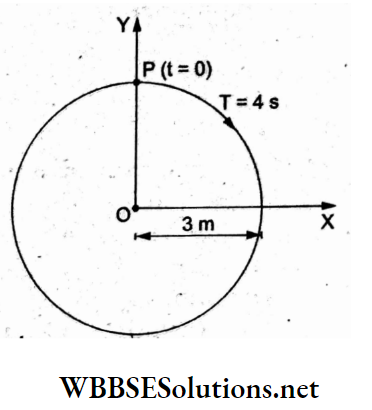
Question 54. The radius of a circle, the period of revolutions, the initial position, and the sense of revolutions are indicated in the adjoining figure. The projection of the rotating radius vector \(\overrightarrow{\mathrm{OP}}\) on the y-axis is given by

1. y(t) = -3cos 2nt, where y is in metres
2. \(y(t)=4 \sin \frac{\pi t}{2}\), where y is in metres
3. \(y(t)=3 \cos \frac{3 \pi t}{2}\), where y is in metres
4. \(y(t)=3 \cos \frac{\pi t}{2}\), where y is in metres

Answer: 4. \(y(t)=3 \cos \frac{\pi t}{2}\), where y is in metres

The projection of a uniform circular motion on its diameter executes an SHM with an amplitude of A = radius of the circle. Here, at t = 0, a particle is at the extreme position. So, the instantaneous position (y) will be a cosine function.

Further, T = 4 s.

So,

⇒ \(\omega=\frac{2 \pi}{T}=\frac{\pi}{2} \mathrm{rad} \mathrm{s}^{-1}\)

Hence, \(y=A \cos \omega t=3 \cos \frac{\pi t}{2}\)

Question 55. In a damped oscillation, the graph between velocity (v) and position (x)will be

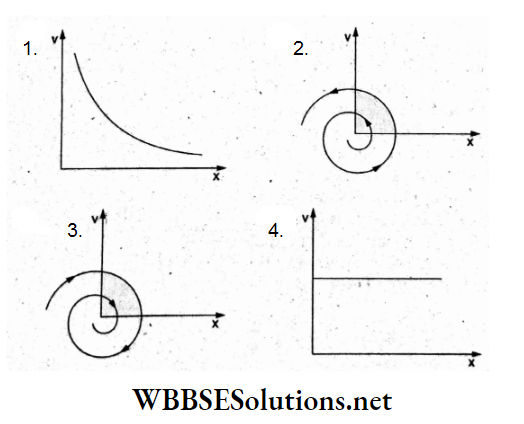
Answer: 3.

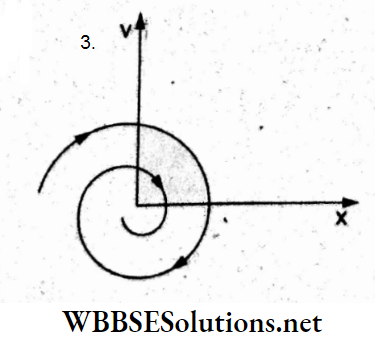
In a damped SHM, the velocity changes periodically between the extreme positive and negative values at the origin with the zero values at the two extremes. Due to damping, the velocity amplitude goes on decreasing. This variation corresponds to the option (3).

Question 56. For the system given in the adjoining figure, M = 1 kg, R = 2 cm, m = 250 g, and k = 100 N m^-1. The angular frequency of the spring-block system will be

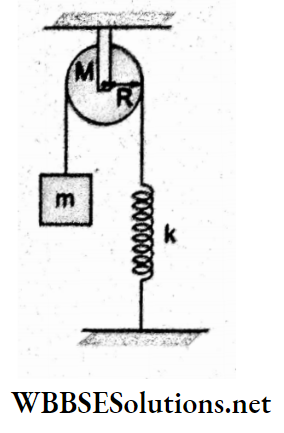
1. \(\frac{20}{\sqrt{3}} \mathrm{rad} \mathrm{s}^{-1}\)
2. 10√3 rad s^-1
3. \(\frac{10}{\sqrt{3}} \mathrm{rad} \mathrm{s}^{-1}\)
4. 20√3 rad s^-1

Answer: 1. \(\frac{20}{\sqrt{3}} \mathrm{rad} \mathrm{s}^{-1}\)

From the law of conservation of energy,

⇒ \(\frac{1}{2} m v^2+\frac{1}{2} I \omega^2+\frac{1}{2} k x^2=\text { constant }\)

Differentiating the above equation with respect to time,

⇒ \(\frac{1}{2} m \cdot 2 v \cdot \frac{d v}{d t}+\frac{1}{2} I \cdot 2 \omega \frac{d \omega}{d t}+\frac{1}{2} k \cdot 2 x \frac{d x}{d t}=0\)

⇒ \(m v a+I \omega \alpha+k x v=0 \Rightarrow m v a+\frac{1}{2} M R^2 \cdot \frac{v}{R} \cdot \frac{a}{R}+k x v=0\)

⇒ \(a\left(m+\frac{M}{2}\right)=-k x \Rightarrow a=-\left(\frac{k}{m+\frac{M}{2}}\right) x \Rightarrow a=-\omega^2 x\)

This represents an SHM with an angular frequency of

⇒ \(\omega=\sqrt{\frac{k}{m+\frac{M}{2}}}=\sqrt{\frac{100 \mathrm{Nm}^{-1}}{\frac{1}{4} \mathrm{~kg}+\frac{1}{2} \mathrm{~kg}}}\)

⇒ \(\sqrt{\frac{400}{3}} \mathrm{~s}^{-1}=\frac{20}{\sqrt{3}} \mathrm{rad} \mathrm{s}^{-1}\)

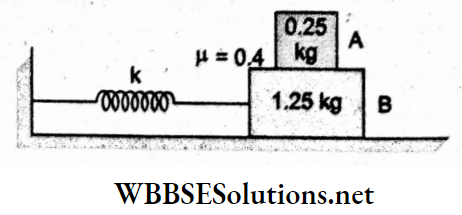
Question 57. The spring constant is given by k = 100 N m^-1. The system executes an SHM with an amplitude of A. Find the maximum amplitude A_max so, that block A does not slip over B.

1. 2 cm
2. 6 cm
3. 8 cm
4. 4 cm

Answer: 2. 6 cm

For no slipping between the blocks A and B, the limiting static friction is

⇒ \(f_{\max }=\mu \delta V \geq F_{\mathrm{ps}} \Rightarrow \mu m_{\mathrm{A}} g \geq m_{\mathrm{A}} a_{\mathrm{fr}}\)….(1)

For the combined system, (mA +mB)a = -kx.

∴ maximum acceleration = \(\frac{k A}{m_A+m_B}, \text { where } A=\text { amplitude }\)

∴ \(A \leq \frac{\mu g\left(m_{\mathrm{A}}+m_{\mathrm{B}}\right)}{k}\)

maximum amplitude = \(\frac{0.4 \times 10 \times 1.5}{100} \mathrm{~m}=6 \mathrm{~cm}\)

Question 58. A particle executes an SHM with an amplitude of 5 cm. When the particle is at 4 cm from the mean position, the numerical value of the magnitude of its velocity in SI units is equal to that of its acceleration. Its periodic time is

1. \(\frac{8 \pi}{3} \mathrm{~s}\)
2. \(\frac{3 \pi}{8} \mathrm{~s}\)
3. \(\frac{7 \pi}{4} \mathrm{~s}\)
4. \(\frac{4 \pi}{3} \mathrm{~s}\)

Answer: 1. \(\frac{8 \pi}{3} \mathrm{~s}\)

Given that amplitude = A = 5 cm, displacement-x-4 cm.

Hence, velocity = \(v=\omega \sqrt{A^2-x^2}\) and the magnitude of die acceleration is |a| = ω²x,

∵ \(\omega^2 x=\omega \sqrt{A^2-x^2}\)

∴ \(\frac{2 \pi}{T}=\frac{\sqrt{(5 \mathrm{~cm})^2-(4 \mathrm{~cm})^2}}{4 \mathrm{~cm}}\)

⇒ \(T=2 \pi \cdot \frac{4}{3} s=\frac{8 \pi}{3} s\)

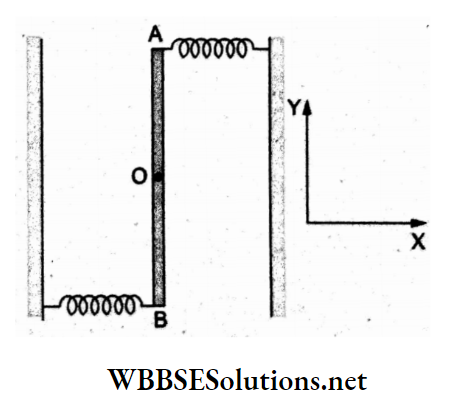
Question 59. Two light identical springs, each of spring constant k, are attached horizontally at the two ends of a uniform horizontal rod AB of length l and mass m. The rod is pivoted at its centre O and can rotate freely in the horizontal plane. The other ends of the two springs are fixed to two rigid supports, as shown in the figure. The rod is now turned through a small angle and then released. The frequency of the resulting oscillations will be

1. \(\frac{1}{2 \pi} \sqrt{\frac{k}{2 m}}\)
2. \(\frac{1}{2 \pi} \sqrt{\frac{3 k}{2 m}}\)
3. \(\frac{1}{2 \pi} \sqrt{\frac{6 k}{m}}\)
4. \(\frac{1}{2 \pi} \sqrt{\frac{k}{m}}\)

Answer: 3. \(\frac{1}{2 \pi} \sqrt{\frac{6 k}{m}}\)

The compression in each spring is \(x=\frac{l}{2} \sin \theta \approx l\left(\frac{\theta}{2}\right) \text { for } \theta \rightarrow 0\)

The force on each end of the rod is

⇒ \(F=k x=\frac{k l \theta}{2}\)

The controlling torque Y during the rotational motion is

⇒ \(\tau=-k x\left(A^{\prime} C\right)=-\frac{k l \theta}{2}(l \cos \theta)\)

⇒ \(-\frac{k l^2 \theta}{2} \text { for } \cos \theta \rightarrow\)

But torque \(I \alpha=\left(\frac{m l^2}{12}\right)\)

⇒ \(\left(\frac{m l^2}{12}\right) \alpha=-\left(\frac{k l^2}{2}\right) \theta \Rightarrow \alpha=-\left(\frac{6 k}{m}\right) \theta\)

This represents an angular SHM with an angular frequency of

⇒ \(\omega=2 \pi f=\sqrt{\frac{6 k}{m}}\)

∴ \(f=\frac{1}{2 \pi} \sqrt{\frac{6 k}{m}}\)

Question 60. An SHM is represented by y = 5(sin 3πt + √3 cos 3πt) cm. The amplitude and the time period of oscillations are respectively

1. 5 cm and \(\frac{3}{2}\) s
2. 5 cm and \(\frac{2}{3}\) s
3. 10 cm and \(\frac{2}{3}\) s
4. 10 cm and \(\frac{3}{2}\) s

Answer: 3. 10 cm and \(\frac{2}{3}\) s

The given equation is \(y=5 \sin (3 \pi t)+5 \sqrt{3} \cos (3 \pi t)\)

Comparing this equation with y = Asin

⇒\(a=\sqrt{A^2+B^2}=\sqrt{5^2+(5 \sqrt{3})^2} \mathrm{~cm}\)

= 10 cm.

∴ angular frequency = \(\omega=\frac{2 \pi}{T}=3 \pi \Rightarrow T=\frac{2}{3}\)

Question 61. A pendulum executes an SHM with its maximum kinetic energy Kv If the length of the pendulum is doubled and it performs an SHM with the same amplitude as in the first case, the maximum kinetic energy is K₂. Then,

1. K₂ = 2K₁
2. K₂ = K₁
3. \(K_2=\frac{K_1}{2}\)
4. \(K_2=\frac{K_1}{4}\)

Answer: 3. \(K_2=\frac{K_1}{2}\)

The maximum KE in the given SHM is

⇒ \(K_1=\frac{1}{2} m \omega_1^2 A^2\)

When the length of the pendulum is doubled keeping its amplitude (A) unchanged, the maximum KE becomes

⇒ \(K_2=\frac{1}{2} m \omega_2^2 A^2\)

∴ \(\frac{K_1}{K_2}=\left(\frac{\omega_1}{\omega_2}\right)^2=\left(\frac{\frac{1}{2 \pi} \sqrt{\frac{g}{l}}}{\frac{1}{2 \pi} \sqrt{\frac{g}{2 l}}}\right)^2=2\)

⇒ \(K_2=\frac{K_1}{2}\)

Question 62. A spring-block system having a block of mass m executes an SHM with an amplitude of A and a frequency of l. While crossing the mean position, the mass is reduced to m/2. The resulting amplitude changes to kA, where k is equal to

1. \(\frac{1}{2}\)
2. \(\frac{1}{\sqrt{2}}\)
3. √2
4. 1

Answer: 3. √2

At the mean position, the velocity is maximum (= Aw) but the acceleration is zero, the net force is zero, and hence the momentum is conserved.

∴ \(m A \omega=\frac{m}{2} \cdot A^{\prime} \omega^{\prime}\)

⇒ \(A \sqrt{\frac{k}{m}}=\frac{A^{\prime}}{2} \sqrt{\frac{\frac{k}{m}}{2}}\)

⇒ \(A=\frac{A^{\prime}}{\sqrt{2}}\)

⇒ \(A^{\prime}=\sqrt{2} A\)

∴ k = √2.

Question 63. An object of mass m is suspended at the end of a light wire of length L and of the area of cross-section A. The Young modulus of the material of the wire is Y. If the mass is pulled down slightly and then released, its frequency of oscillations in the vertical direction is

1. \(f=\frac{1}{2 \pi} \sqrt{\frac{Y L}{m A}}\)
2. \(f=\frac{1}{2 \pi} \sqrt{\frac{m L}{Y A}}\)
3. \(f=\frac{1}{2 \pi} \sqrt{\frac{Y A}{m L}}\)
4. \(f=\frac{1}{2 \pi} \sqrt{\frac{m A}{Y L}}\)

Answer: 3. \(f=\frac{1}{2 \pi} \sqrt{\frac{Y A}{m L}}\)

For the extension x, let the restoring force be F.

∴ \(Y=\frac{\frac{F}{A}}{\frac{x}{L}}\)

⇒ \(F=-\left(\frac{Y A}{L}\right) x\)

∴ acceleration = \(a=\frac{F}{m}\)

=\(-\left(\frac{Y A}{m L}\right) x\)

=\(-\omega^2 x\)

This represents an SHM with

⇒ \(\omega=\sqrt{\frac{Y A}{m L}}\)

or, \(f=\frac{\omega}{2 \pi}=\frac{1}{2 \pi} \sqrt{\frac{Y A}{m L}}\)

Question 64. A spring of force constant is suspended from a support, and a block of mass m is attached to its lower end. The block is released when the spring is relaxed. The motion is described by y(t)= y₀sin²ωf, where y is measured from the free end of the released spring. Then, die angular frequency (ω) is

1. \(\frac{1}{2} \sqrt{\frac{g}{y_0}}\)
2. \(\sqrt{\frac{g}{y_0}}\)
3. \(\sqrt{\frac{g}{2 y_0}}\)
4. \(\sqrt{\frac{2 g}{y_0}}\)

Answer: 3. \(\sqrt{\frac{g}{2 y_0}}\)

Given that \(y(t)=y_0 \sin ^2 \omega t=\frac{y_0}{2}(1-\cos 2 \omega t)\)

Hence, the elongation in the spring when in equilibrium (before the block is released) is \(\frac{y_0}{2}\), which is produced by the weight mg of the object.

Now, \(m g=k\left(\frac{y_0}{2}\right) \text { and } 2 \omega=\sqrt{\frac{k}{m}}=\sqrt{\frac{2 g}{y_0}}\) [∵ \(\text { in an SHM, } \omega^2=\frac{k}{m}\)]

∴ \(\omega=\frac{1}{2} \sqrt{\frac{2 g}{y_0}}=\sqrt{\frac{g}{2 y_0}}\)