Important Questions for Class 11 Physics Rotation Of Rigid Bodies

 Rotation Of Rigid Bodies Long Answer Type Questions

Question 1. Is there any change in the angular velocity of the earth when a body strikes the earth’s surface from outside?
Answer:

We know that, the angular momentum of a body = moment of inertia of that body x angular velocity of the body.

• The velocity of anybody coming from outside and striking the surface of the earth is usually directed towards the centre of the earth. For this reason, the body possesses no angular momentum with respect to the axis of rotation of the earth.
• As a result, the angular momentum of the earth remains conserved. However due to the inclusion of the body, the mass of the earth increases, and consequently, the moment of inertia of the earth also increases.
• For this reason, the angular velocity of the earth decreases slightly. But actually, the mass of such a foreign body is so small that there is no appreciable change in either the moment of inertia or the angular velocity of the earth.

Question 2. Why is It easier to rotate an object tied to the end of a short string than that of a long string?
Answer:

We know that the moment of inertia of an object about the axis of rotation is, I = mr², where m is the mass of the object and r is the perpendicular distance of the object from the axis of rotation.

So, with the increase in the length of the string, the moment of inertia of the object also increases. The object is rotated along a circular path by applying a torque against the resistive force of the air.

Now, torque = moment of inertia x angular acceleration.

Hence, with the increase in moment of inertia, the magnitude of required torque also increases.

Moreover, the centrifugal reaction generated at the centre of rotation is mω²r, where ω is the angular velocity of the stone. So, keeping the value of ω constant, if the value of r is increased, centrifugal reaction also increases.

Hence, if the string is short, then less torque is required to rotate the stone tied to the string. Also, it has to withstand a smaller centrifugal reaction and as a result, it is easier to rotate the object.

Question 3. A solid and a hollow sphere of the same mass have the same outer radius. Which one has a larger radius of gyration?
Answer:

The mass of the solid sphere is distributed uniformly from its centre. On the other hand, the mass of the hollow sphere is distributed over a comparatively further distance.

The more the distance of the mass distribution from the axis of rotation of two bodies of identical mass having the same outer radius, the more the radius of gyration.

In this sense, the mass of the hollow sphere is distributed over a comparatively larger distance from the axis of rotation than that of the solid sphere, and hence the radius of gyration of the hollow sphere will be greater.

Question 4. If the ice at the poles of the earth melts, how would this affect the length of the day?
Answer:

If the polar ice melts, a part of the water thus produced will shift from the poles towards the equatorial region, and hence, this water will shift away from the axis of rotation of the earth. Consequently, the moment of inertia of the earth will increase.

Now, according to the principle of conservation of angular momentum, with the increase in moment of inertia of the earth, its angular velocity will decrease, and hence, the length of the day will increase.

Question 5. A cricket ball sometimes rebounds from the cricket pitch with a velocity greater than which it was bowled with by a bowler. How can it be possible?
Answer:

If the cricket ball spins just before it hits the ground, then this spinning kinetic energy is added to the kinetic energy of the translation of the ball. As a result, the ball rebounds from the cricket pitch with a greater velocity by virtue of this spuming or rotational kinetic energy.

Question 6. Can the moment of Inertia of a body be different about different axes?
Answer:

The moment of inertia of a body depends on the mass of the body, the position of the axis of its rotation and the distribution of mass of the body about its axis of rotation. So, the moment of inertia of a particular body may be different in different axes.

Question 7. A man is standing on a rotating table and he drops a heavy mass from his hand outside the table. How will the angular speed of the table change?
Answer:

When the mem drops the heavy mass from his hand outside the table, the moment of inertia of the system about the axis of rotation decreases. We know that angular momentum = moment of inertia x angular velocity. Since no torque is applied from outside, according to the principle of conservation of angular momentum, the angular velocity of the system will increase due to a decrease of its moment of inertia.

Question 8. When a body of mass m slides down from the top of an inclined plane and reaches the bottom, its velocity becomes v. When a circular disc of the same mass is rolled down the inclined plane, it acquires a velocity v₁. Show that, \(v_1=\sqrt{\frac{2}{3}} v\).
Answer:

Let the vertical height of the inclined plane be h. In case of the first body, \(\frac{1}{2} m v^2=m g h \quad \text { or, } \quad v=\sqrt{2 g h}\)…(1)

If the body is a circular disc, then it possesses both translational kinetic energy and rotational kinetic energy while rolling down the inclined plane.

If I am a moment of inertia of the circular disc and ω be angular velocity of the disc at the bottom of the inclined plane,

⇒ \(\frac{1}{2} m v_1^2+\frac{1}{2} I \omega^2=m g h\)

or, \(\frac{1}{2} m v_1^2+\frac{1}{2} \cdot \frac{1}{2} m r^2 \cdot \frac{v_1^2}{r^2}=m g h \)

r = (radius of the disc, \(I=\frac{1}{2} m r^2, \omega=\frac{v_1}{r}\))

or, \(\left(\frac{1}{2}+\frac{1}{4}\right) v_1^2=g h \text { or, } \frac{3}{4} v_1^2=g h \text { or, } v_1=\sqrt{\frac{4}{3} g h}\)

∴ \(\frac{v_1}{v}=\sqrt{\frac{4}{2} g h}=\sqrt{\frac{2}{3}} \text { or, } v_1=\sqrt{\frac{2}{3}} v .\)

Question 9. Prove that the length of a day becomes T’ = 6h instead of T = 24 h if the earth suddenly contracts to half its present radius (consider the earth as a spherical body), without having any change in its mass.
Answer:

Since the earth is a solid sphere, its moment of inertia, I ∝ R²(R = radius of the earth)

(moment of inertia of solid sphere = \(\frac{2}{5}\)MR²)

So, if the present radius is R and the changed radius is \(\frac{R}{2}\) then,

⇒ \(\frac{I}{I^{\prime}}=\frac{R^2}{\left(\frac{R}{2}\right)^2}=4\)

Again, if the present angular velocity is ω and the changed angular velocity is ω’, then according to the principle of conservation of angular momentum,

⇒ \(I \omega=I^{\prime} \omega^{\prime} \text { or, } \omega^{\prime}=\frac{I}{I^{\prime}} \omega=4 \omega\)

∴ \( \frac{2 \pi}{T^{\prime}}=4 \cdot \frac{2 \pi}{T} \quad \text { or, } T^{\prime}=\frac{T}{4}=\frac{24}{4}=6 \mathrm{~h} \text {. } \)

Question 10. Show that the torque acting on a body is equal to the rate of change of angular momentum of the body.
Answer:

We know that angular momentum, \(\vec{L}=\vec{r} \times \vec{p} \quad \text { or, } \vec{L}=\vec{r} \times m \vec{v}\)

⇒\(\frac{d \vec{L}}{d t}=\frac{d \vec{r}}{d t} \times m \vec{v}+\vec{r} \times \frac{d}{d t}(m \vec{v})\)

= \(\vec{v} \times m \vec{v}+\vec{r} \times \vec{F}=\vec{r} \times \vec{F}\) (because \(\vec{v} \times \vec{v}=0\))

= \(\vec{r}\)

So, the rate of change of angular momentum of a body is equal to the torque acting on the body

Question 11. What is the relation between torque and angular acceleration?
Answer:

Torque, \(\vec{\tau}= \frac{d \vec{L}}{d t}=\frac{d}{d t}(I \vec{\omega})=I \frac{d \vec{\omega}}{d t}\)

(\(\vec{L}\)= angular momentum = \(I \vec{\omega}\))

= \(I \vec{\alpha}[\vec{\alpha}\) = angular acceleration

Question 12. Keeping the radius of the earth unchanged, If the mass of the earth is doubled, then what will be the length of a day?
Answer:

If the present angular velocity is ω and the changed angular velocity is ω’, then according to the principle of conservation of angular momentum, Iω = I’ω’

[here, I and I’ are the present and the changed moments of inertia of the earth respectively]

∴ \(\frac{2}{5} M R^2 \times \frac{2 \pi}{24}=\frac{2}{5} \times 2 M \times R^2 \times \frac{2 \pi}{T^{\prime}}\)

[here, the present mass of the earth is M, its radius is R and the changed length of a day is T’]

or, \(\frac{1}{24}=\frac{2}{T^{\prime}} \quad \text { or, } \quad T^{\prime}=48 \text { hours }\)

So, the length of a day will be 48 h.

Question 13. A circular disc of mass m and radius r is rolling over a horizontal table top with angular velocity ω. Prove that the total energy of the disc, K = \(\frac{3}{4}\)mω²r²
Answer:

The total kinetic energy of the disc,

K = translational kinetic energy+rotational kinetic energy

= \(\frac{1}{2} m v^2+\frac{1}{2} I \omega^2\)

Here, v = linear velocity of the disc = ωr

I = moment of inertia of the disc about the perpendicular axis passing through its centre

= \(\frac{1}{2} m r^2\)

K = \(\frac{1}{2} m(\omega r)^2+\frac{1}{2} \cdot \frac{1}{2} m r^2 \cdot \omega^2\)

= \(\frac{1}{2} m \omega^2 r^2+\frac{1}{4} m \omega^2 r^2=\frac{3}{4} m \omega^2 r^2\)

Question 14. Between two circular discs of equal mass and equal thickness but of different densities, which one would have a greater moment of Inertia about its central perpendicular axis?
Answer:

Suppose the mass of each disc is M, thickness d, densities of their materials ρ₁ and ρ₂ (ρ₁ > ρ₂), radii r₁ and r₂ respectively.

∴ \(M=\pi r_1^2 d \rho_1=\pi r_2^2 d \rho_2 \quad \text { or, } r_1^2 \rho_1=r_2^2 \rho_2\)

or, \(\frac{r_1^2}{r_2^2}=\frac{\rho_2}{\rho_1}\)

∴ \(\rho_1>\rho_2, \quad \frac{\rho_2}{\rho_1}<1\)

∴ \(\frac{r_1^2}{r_2^2}<1\)

The moment of inertia of the two discs about their central perpendicular axes are \(I_1=\frac{1}{2} M r_1^2 \text { and } I_2=\frac{1}{2} M r_2^2\)

∴ \(\frac{I_1}{I_2}=\frac{r_1^2}{r_2^2}<1\)

∴ \(I_1<I_2\)

So, the disc having a lower density will have a greater moment of inertia about its centred perpendicular axis.

Question 15. Given the moment of inertia of a disc of mass M and radius R about any of its diameters to be \(\frac{M R^2}{4}\). Find its moment of inertia about an axis normal to the disc and passing through a point on its edge.
Answer:

Given,

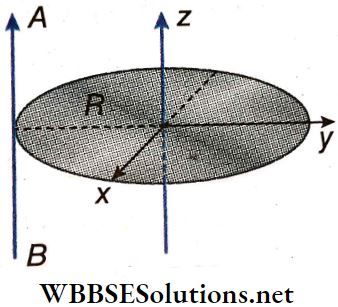
According to the parallel-axes theorem, the moment of inertia of the disc about the axis AB, normal to the disc and passing through a point on its edge,

Question 16. ‘Moment of inertia plays the same role in rotational motion as mass plays in translational motion’explain the statement.
Answer:

When a force acts on a body, some linear acceleration is produced in that body. Similarly, angular acceleration is generated in a body due to the application of a torque on it. So the rotational analogues of force and linear acceleration are torque and angular acceleration, respectively.

Again, in the case of linear motion, force = mass x acceleration; and in the case of circular motion, torque = moment of inertia x angular acceleration. Comparing these two relations it can be inferred that the rotational analogue of mass is the moment of inertia.

So, moment of inertia in rotational motion plays the same role as mass plays in linear motion.

Question 17. Find the moment of inertia of a sphere about a tangent to the sphere. Given the moment of inertia of the sphere about any of its diameters to be \(\frac{2 M R^2}{5}\), where M is the mass of the sphere and R is the radius of the sphere.
Answer:

The centre of mass (cm) of C the sphere is on its diameter AB.

So, I_cm = \(\frac{2}{5}\)MR²

According to the parallel-axes theorem, the moment of inertia of the sphere about the tangent CD.

I = \(I_{\mathrm{cm}}+M R^2=\frac{2}{5} M R^2+M R^2=\frac{7}{5} M R^2\)

Question 18. A solid sphere of mass m and radius R rolls down from the top of a table. With how much angular speed will it touch the ground?
Answer:

At position 2, the resultant of mgcosθ and n supplies the necessary centripetal force to roll on, i.e., mω²R = mgcosθ – n

[ω = angular speed of the spehere]

When n = 0, the sphere will not be in contact more with the table.

Then, \(m \omega^2 R=m g \cos \theta \quad \text { or, } \cos \theta=\frac{\omega^2 R}{g}\)

The centre of mass of the sphere is lowered down by R(1-cosθ).

Let I be the moment of inertia of the sphere about the tangent at the contact point between the table and the sphere. From the conservation law of mechanical energy,

⇒ \(m g \cdot R(1-\cos \theta)=\frac{1}{2} \times I \omega^2=\frac{7}{10} m R^2 \omega^2\) (because \(I=\frac{7}{5} m R^2\))

∴ \(m g R\left(1-\frac{\omega^2 R}{g}\right)=\frac{7}{10} m R^2 \omega^2\)

or, \(m g R-m \omega^2 R^2=\frac{7}{10} m R^2 \omega^2\)

∴ \( \omega=\sqrt{\frac{10 g}{17 R}}\)

Considering a free fall of the sphere, as no torque acts on it, this angular speed remains unaltered. It means that the sphere touches the ground with an angular speed \(\sqrt{\frac{10 g}{17 R}}\)

Question 19. A uniform rod AB of mass M and length L is hung from a ceiling in such a way that the rod can rotate freely in the vertical plane around point A. An object of mass m coming horizontally with velocity v₀ hits the rod at point B and sticks to the rod. What will be the maximum angle with the vertical that the rod makes in this type of collision? (Here, M = 6m)

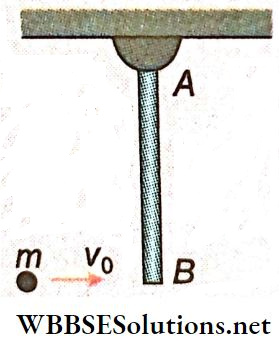
Answer:

In the case of the composite system of the rod and the object of mass m, applying the law of conservation of angular momentum about A,

⇒ \(m v_0 L=I \omega=\left[\frac{M L^2}{3}+m L^2\right] \omega=\left(\frac{M}{3}+m\right) L^2 \omega\)

∴ \(\omega=\frac{m v_0}{\left(\frac{M}{3}+m\right) L}=\frac{m v_0}{(2 m+m) L}=\frac{v_0}{3 L}\)

The distance of the centre of mass of the composite system from point A when the object sticks to the rod

= \(\frac{6 m \times \frac{1}{2}+m \times L}{6 m+m}=\frac{4}{7} L\)

The rod makes the maximum angle θ with the vertical, and at that position, the centre of mass of the composite system raises at a height h from its initial position.

h = \(\frac{4}{7} L(1-\cos \theta)\)

According to the law of conservation of mechanical energy, \(\frac{1}{2} I \omega^2=(m+6 m) g h\)

or, \(\frac{1}{2}\left(\frac{1}{3} M L^2+m L^2\right) \times \frac{\nu_0^2}{9 L^2}=4 m g L(1-\cos \theta)\)

or, \(1-\cos \theta=\frac{v_0^2}{24 g L} therefore \theta=\cos ^{-1}\left[1-\frac{\nu_0^2}{24 g L}\right]\)

Question 20. A spherical object of mass m is released on a smooth inclined plane which is inclined at an angle θ with the horizontal. State whether it will roll or slip. Give reasons in support of your answer.
Answer:

No frictional force acts on a smooth plane. The only downward force acting on the centre of mass of the object along the inclined plane is mgsinθ. But there is no torque about the centre of mass due to the absence of the frictional force. So, the object will slip down without rolling with acceleration gsinθ.

Unit 5 Motion Of System Of Particles And Rigid Body Chapter 2 Rotation Of Rigid Bodies Synopsis

Two equal, parallel but oppositely directed forces acting simultaneously along two different lines of action constitute a couple.

• The vector quantity formed by the combination of the couple applied on a body and the arm of the couple, which produces a rotational tendency in that body is called the moment of the couple or torque.
• The moment of the couple or torque is expressed by the product of the magnitude of any of the forces of the couple and the arm of the couple.
• The torque about a point = the algebraic sum of the moments of the two forces of the couple with respect to that point. The torque acting on a body with respect to any axis of rotation divided by the corresponding angular acceleration generated is called the moment of inertia of that body with respect to that axis of rotation.

If the whole mass of a body is assumed to be concentrated at a point such that the moment of inertia of the whole body equals the moment of inertia of that point, then the radial distance of the point from the axis of rotation is called the radius of gyration.

Parallel-axes Theorem: The moment of inertia (I) of a rigid body about any axis is equal to the sum of its moment of inertia about a parallel axis through its centre of mass (I_cm) and the product of the mass of the body (M) with the square of the perpendicular distance between the two axes (r²).

Mathematical expression: I = I_cm + Mr²

Perpendicular-axes Theorem: The moment of inertia of a plane lamina about an axis perpendicular to its plane (I_z) is equal to the sum of the moments of inertia of the lamina about two mutually perpendicular axes (I_x +I_y) lying on the plane of the lamina and intersecting each other at the point through where the perpendicular axis passes. Mathematical expression: I_x+I_y= I_z

The dynamical property generated in a rotating body by the moment of inertia of the body about an axis and its angular velocity together is called the angular momentum of the body about that axis.

Principle Of Conservation Of Angular Momentum: If the net external torque on a body is zero, the angular momentum of the body rotating about an axis always remains conserved.