Formula, Graph, Examples, Experiment Hooke’s Law Of Elasticity

Elasticity Force Constant Of A Spring

One end of a spring is fixed to a rigid support and a stretching force F is applied at its other end. As a result, the spring elongates by a length x.

According to Hooke’s law, F ∝ x or, F = kx ……..(1)

[where k is a proportionality constant]

According to Newton’s third law of motion, the spring also exerts an equal but opposite reaction F_e.

F_e is called the elastic force of the spring.

Therefore, F_e = -F.

So, from equation (1), we get, F_e = -kx ….(2)

Here, the negative sign indicates that x and F_e are oppositely directed.

The constant k in the above equation is called the force con¬stant of the spring. Now, for x = 1, F = k.

So, the force constant of a spring (or, simply, the spring constant) is defined as the external force required to produce a unit elongation.

Read and Learn More: Class 11 Physics Notes

Units of force constant:

• dyn.cm^-1
• N.m^-1

The force constant of a spring measures the stiffness of the spring. The higher the value of k, the stiffer is the spring.

⇒ \(1 \mathrm{~N} \cdot \mathrm{m}^{-1}=\frac{1 \mathrm{~N}}{1 \mathrm{~m}}=\frac{10^5 \mathrm{dyn}}{10^2 \mathrm{~cm}}=10^3 \mathrm{dyn} \cdot \mathrm{cm}^{-1}\)

Force Constant of Spring Combinations

Series combination: Let the spring constants of two massless springs A and B be k₁ and k₂ respectively. These two springs are joined in series and hung from a rigid support. A downward force F is applied at the free end of spring B. Let the elongations in springs A and B
be x₁ and x₂ respectively. Hence, from equation (1), we get,

F = \(k_1 x_1=k_2 x_2\)

or, \(F=\frac{x_1}{\frac{1}{k_1}}=\frac{x_2}{\frac{1}{k_2}}=\frac{x_1+x_2}{\frac{1}{k_1}+\frac{1}{k_2}}\)…..(3)

Now, the total elongation in this combination of springs is (x₁ + x₂) . If k is the equivalent spring constant of this combination, then,

F = \(k\left(x_1+x_2\right)=\frac{x_1+x_2}{\frac{1}{k}}\)…….(4)

From (3) and (4), we get, \(\frac{1}{k}=\frac{1}{k_1}+\frac{1}{k_2} \text { or, } k=\frac{k_1 k_2}{k_1+k_2}\)

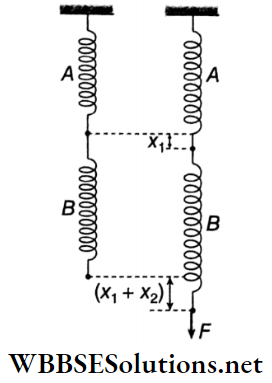
The relation shows k<k_{1 •} k₂. This means that series combinations reduce the effective spring constant.

Parallel combination: Let the spring constants of two massless springs A and B be k₁ and k₂ respectively. These two springs are placed in parallel and hung from a rigid support. Next, a downward force F is applied to the combination so that the springs elongate.

Here, the forces on A and B are F₁ and F₂ respectively (where, F = F₁ + F₂) and the elongation of each is x.

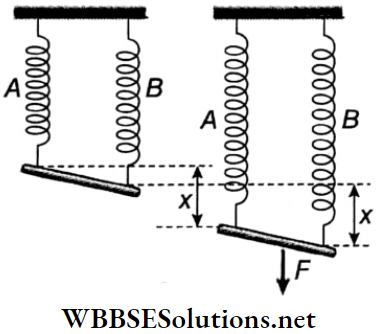
So, from equation (1), we get, \(F_1=k_1 x \text { and } F_2=k_2 x\)

∴ \(F_1+F_2=\left(k_1+k_2\right) x \text { or, } F=\left(k_1+k_2\right) x\)……(5)

Now, the total elongation of this combination of springs is x.
If k is the equivalent spring constant of this combination, then F = kx  …..(6)

From (5) and (6), we get, k = k₁ + k₂.

This shows that parallel combinations increase the effective spring constant.

Force constant and length of a spring: Let, a spring of length l has a force constant k. If a force F produces an elongation x, then F = kx, or, k = \(\frac{F}{x}\).

Now, let us consider half the length, i.e., \(\frac{l}{2}\) of the same spring. As the length is half, the elongation will also be half, i.e., \(\frac{x}{2}\), due to the same force F. The new force constant of this half-spring is \(k^{\prime}=\frac{F}{\frac{x}{2}}=\frac{2 F}{x}=2 k\), i.e., the spring constant is doubled.

Therefore, the spring constant is inversely proportional to its length, i.e., K = \(\frac{l}{L}\) or, kl = constant

Energy stored in a stretched spring: if a force F = kx stretches a spring from x to x+dx, the work done, dW = Fdx = kxdx. So, the total work done in stretching the spring from elongation 0 to l is W = \(\int d W=k \int_0^l x d x=\frac{1}{2} k l^2\). This is stored in the stretched spring as its potential energy. Therefore, the energy stored = \(\frac{1}{2} k l^2\)

Elasticity Force Constant Of A Spring Numerical examples

Example 1. When a mass of 4 kg is hung from the lower end of a spring, it elongates by 1 cm.

1. What is the force constant of the spring?
2. If a load of 2 kg is hung from the lower end of the spring, then find its elongation.

Solution:

When a mass of 4 kg is hung from the lower end of a spring, it elongates by 1 cm.

1. We know that, F = kx or, k = \(\frac{F}{x}\)

Here, F = 4 x 9.8 N ; x = 1 cm = 0.01 m

∴ k = \(\frac{4 \times 9.8}{0.01}=3920 \mathrm{~N} \cdot \mathrm{m}^{-1}\)

2. We know that, F = kx or, x = \(\frac{F}{k}\)

Here, F = 2 x 9.8 N; k = 3920 N • m^-1

∴ x = \(\frac{2 \times 9.8}{3920}=0.005 \mathrm{~m}\)

Example 2. The force constant of a spring is k. The spring is cut into three equal parts. Find the force constant of each part.
Solution:

The force constant of a spring is k. The spring is cut into three equal parts.

Let us consider that the spring elongates by x when a force F is applied on it. So, the force constant of the spring, k = \(\frac{F}{x}\)

Now, if the spring is cut into three equal parts, then on the application of the same force F, each part of the spring will elongate by \(\frac{x}{3}\).

Therefore, the force constant of each part, \(k^{\prime}=\frac{F}{\frac{x}{3}}=\frac{3 F}{x}=3 k\)

Example 3. The force constant of a spring of length l is k. The spring is cut into two parts of lengths l₁ and l₂. If I₁ = nI₂, then find the spring constants k₁ and k₂ of the two parts, n is an integer.
Solution:

The force constant of a spring of length l is k. The spring is cut into two parts of lengths l₁ and l₂. If I₁ = nI₂,

According to the problem,

l = \(l_1+l_2=l_1+\frac{l_1}{n}=l_1\left(\frac{n+1}{n}\right)\)

Again, l = \(l_1+l_2=n l_2+l_2=l_2(n+1)\)

We know that for a particular spring, the force constant is inversely proportional to the length.

∴ \(k \propto \frac{1}{l}\)  or, kl = constant

∴ \(k_1 l_1=k l=k l_1 \frac{(n+1)}{n}\)

or, \(k_1=\frac{k}{n}(n+1)\)

Similarly, \(k_2 l_2=k l=k l_2(n+1) or, k_2=k(n+1)\)