Kinetic Theory Of Gases – Pressure Of A Perfect Gas According To The Kinetic Theory
Gas molecules, due to their random motion, collide continuously with the inner walls of its container. As a result, an outward force or thrust acts on the walls. The pressure of a gas is defined as the force exerted normally by the gas molecules on unit area of the walls of its container.
There are a large number of molecules in the container and they have random velocities in all possible directions. Statistically, on unit surface of any wall of the container, equal number of molecules collide with equal average velocity. As a result, the gas exerts equal pressure on the walls in all directions.
It is evident that the pressure of a gas is high, if
- The gas molecules are heavy so that a large force acts on the walls,
- The molecules move fast, so that a big impact is exerted on the walls and
- The molecules are densely situated so that the number of collisions with the walls is high.
This means that the pressure of a gas on the walls of its container depends on
- The mass of each molecule,
- Average velocity of the molecules and
- The number of molecules in a unit volume inside the container.
Derivation of the Expression for Pressure of an Ideal Gas: Let us take a cubical container.
It is filled with a gas. The inside surfaces of the container are such that the molecules suffer elastic collisions with them.
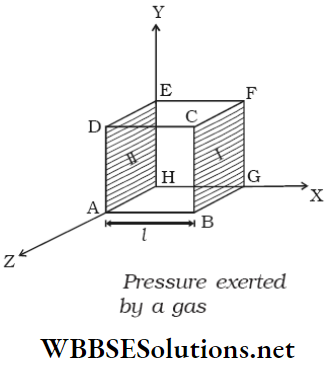
Let l be the length of each side of the container, m be the mass of each gas molecule and N be the total number of molecules in the container.ρ
Sp, A = \(l^2=\text { area of each of the six inner surfaces, }\)
V = \(l^3=\text { volume of the gas of the container, }\)
M = \(m N=\text { mass of the gas, }\)
ρ = \(\frac{M}{V}=\frac{m N}{V}=\frac{m N}{l^3}=\text { density of the gas, }\)
n = \(\frac{N}{V}=\frac{N}{\beta}\)= number of molecules in unit volume
= number density of the gas
= molecular concentration
Then, ρ = mn
Now, one corner O of the cubical container is taken as the origin, and the x, y, and z axes are chosen along the three mutually perpendicular sides at O.
Let c be the velocity of a gas molecule and u, v, w be the velocity components along the three axes, respectively.
So, c² = u² + v² + w²
Now, let us consider only the parallel surfaces R and S, which are perpendicular to the x-axis. As the collisions are elastic, the molecule hits the surface R at velocity u and rebounds from this surface In the opposite direction, i.e., the velocity of the molecule will he -u.
But v and w do not change In this case. So, for surfaces R and s, only the velocity component u or -u should be considered.
Thus, mu = momentum of the molecule before collision,
mu = momentum of the molecule after the collision,
So, a change in the momentum of the molecule
= final momentum- initial momentum
= – mu -(mu) = -2mu
After a collision at R, the molecule moves towards S, Again the collision is S brings the molecule back to R, The effective distance traveled by the molecule between two collisions with the same surface = 2l.
The time taken between these two collisions, t = \(\frac{2 l}{u}\)
So, the rate of change in momentum of the molecule \(\frac{-2 m u}{\frac{2 m}{u}}=-\frac{m u^2}{1}\)
From Newton’s second law of motion, die force exerted by the surface R on the molecule = –\(\frac{m u^2}{l}\);
Again, horn Newton’s third law, the equal and opposite force exerted by the molecule on the die surface R = +\(\frac{m u^2}{l}\).
Then, pressure on surface R due to the molecule
= \(\frac{\text { force }}{\text { surface area }}=\frac{\frac{m u^2}{1}}{p^2}=\frac{m u^2}{p^3}=\frac{m u^2}{V}\)
Now, we consider all the N molecules and die components u1, u2…., uN respectively of velocities along the x-axis.
So, the net pressure on surface R, due to all the N molecules in the container, Is \(p_x=\frac{m_1}{v}\left(u_1^2+u_2^2+\cdots+u_N^2\right)\)
Similarly, the pressure on surfaces perpendicular to die y and z axes, respectively, are \(p_y =\frac{m}{v}\left(v_1^2+v_2^2+\cdots+v_N^2\right)\)
and \(p_2 =\frac{m}{v}\left(w_1^2+w_2^2+\cdots+w_N^2\right)\)
As the gas exerts equal pressure in all directions, \(p_{\mathrm{x}}=p_y=p_z=p=\) pressure of the gas.
So, p= \(\frac{1}{3}\left(p_x+p_y+p_z\right)\)
= \(\frac{1}{3}\frac{m}{V}[\left(u_1^2+u_2^2+\cdots+u_N^2\right)+\left(v_1^2+v_2^2+\cdots+v_N^2\right)\)
…. + \(\left.\left(w_1^2+w_2^2+\cdots+w_N^2\right)\right]\)
= \(\frac{1}{3} \frac{m}{V}\left[\left(u_1^2+v_1^2+w_1^2\right)+\left(u_2^2+v_2^2+w_2^2\right)+\right.\)
…… + \(\left(u_N^2+v_N^2+w_N^2)\right]\)
= \(\frac{1}{3} \frac{m}{V}\left(c_1^2+c_2^2+\cdots+c_N^2\right)\)
= \(\frac{1}{3} \cdot \frac{m N}{V} \cdot \frac{\left.\left.c_1^2+c_2^2+\cdots+v_N^2+w_N^2\right)\right]}{N}\)
= \(\frac{1}{3} m \frac{N c^2}{V}\left[c^2=\frac{c_1^2+c_2^2+\cdots c_N^2}{N}(c=\text { ms speed })\right]\)
= \(\frac{1}{3} \frac{M_0}{V} c^2 \text { [total mass of the gas, } M_0=m N]\)
= \(\frac{1}{3} \rho c^2\left[\text { density of the gas, } \rho=\frac{M_0}{V}\right]\)…(1)
This expression shows that pressure depends on the total volume V, but not on the length l of the cubical container. This means that the expression is true for containers of all shapes.
This is the expression for the pressure of an ideal gas, according to kinetic theory.
From equation (1), \(c=\sqrt{\frac{3 p}{\rho}}\)….(2)
In this relation, pressure p and density ρ are bulk thermodynamic properties that are measured by experiments. So, from these measured values, we get an estimate of the rms speed c of the molecules.
Molecular number density: The number of molecules in unit volume N/V = n, this ‘n’ is called the molecular number density of gas.
Now, density, \(\rho=\frac{M_0}{V}=\frac{m N}{V}=m n\)
∴ From equation (1) we get, p = \(\frac{1}{3} m n c^2\)….(3)
This equation is mainly used in chemistry. It can be understood that the pressure of gas depends on three quantities
- Mass of gas molecule (m),
- Number of gas molecules in unit volume (n) and
- rms Speed of the molecules.
Kinetic Theory Of Gases – Pressure Of A Perfect Gas According To The Kinetic Theory Numerical Examples
Example 1. The velocity of 10 gas molecules in a container, are 2, 3, 3, 4, 4, 4, 5, 5,7, and 10 km • s-1, respectively. Find out the mean velocity and rms speed.
Solution:
Mean velocity, \(\bar{c}=\frac{2+3+3+4+4+4+5+5+7+10}{10}=\frac{47}{10}\)
= \(4.7 \mathrm{~km} \cdot \mathrm{s}^{-1} ;\)
rms speed \(\tilde{c}=\sqrt{\frac{2^2+3^2+3^2+4^2+4^2+4^2+5^2+5^2+7^2+10^2}{10}}\)
= \(\sqrt{\frac{269}{10}}=\sqrt{26.9}=5.1865 \mathrm{~km} \cdot \mathrm{s}^{-1} .\)
Example 2. Find out the rms speed of a gas of density 2 g · L-1 at 76 cm Hg pressure. Given, density of mercury = 13.6 g · cm-3 and 980 cm · s-2
Solution:
p = \(76 \times 13.6 \times 980 \mathrm{dyn} \cdot \mathrm{cm}^{-2},\)
ρ = \(2 \mathrm{~g} \cdot \mathrm{L}^{-1}=\frac{2}{1000} \mathrm{~g} \cdot \mathrm{cm}^{-3}\)
So, rms speed,
c = \(\sqrt{\frac{3 p}{\rho}}\)
= \(\sqrt{\frac{3 \times 76 \times 13.6 \times 980 \times 1000}{2}}\)
= \(3.9 \times 10^4 \mathrm{~cm} \cdot \mathrm{s}^{-1}=0.39 \mathrm{~km} \cdot \mathrm{s}^{-1} .\)
Example 3. Determine the rms speed of air molecules at STP. Given, the density of mercury = 13.6 g · cm-3; the density of air = 0.00129 g · cm-3
Solution:
p = \(76 \times 13.6 \times 980 \mathrm{dyn} \cdot \mathrm{cm}^{-2},\)
ρ = \(0.00129 \mathrm{~g} \cdot \mathrm{cm}^{-3}\)
∴ c = \(\sqrt{\frac{3 p}{\rho}}=\sqrt{\frac{3 \times 76 \times 13.6 \times 980}{0.00129}}\)
= \(4.85 \times 10^4 \mathrm{~cm} \cdot \mathrm{s}^{-1}=0.485 \mathrm{~km} \cdot \mathrm{s}^{-1} .\)
Example 4. The rms speed of hydrogen molecules at STP 1.85 km s-1. What is the density of hydrogen gas?
Solution:
Here, c = 1.85 km · s-1 = 1.85 x 105 cm · s-1
p = 76 x 13.0 x 980 dyn · cm-2
From the equation p = \(\frac{1}{3} \rho c^2\) we get,
ρ = \(\frac{3 p}{c^2}=\frac{3 \times(76 \times 13.6 \times 980)}{\left(1.85 \times 10^5\right)^2}\)
= \(0.000089 \mathrm{~g} \cdot \mathrm{cm}^{-3} .\)
Example 5. Find out the rms speed of nitrogen gas molecules at 0°C. The density of nitrogen gas at STP = 1.25 g · L-1 and the density of mercury = 13.6 g · cm-3.
Solution:
p = 76 x 13.6 x 980 dyn cm-2,
ρ = 1.25 g · L-1 = 1.25 x 10-3 g · cm-3
rms speed, = \(\sqrt{\frac{3 p}{\rho}}\)
= \(\sqrt{\frac{3 \times(76 \times 13.6 \times 980}{1.25 \times 10^{-3}}}\)
= \(4.93 \times 10^4 \mathrm{~cm} \cdot \mathrm{s}^{-1} .\)
Example 6. Find out the ratio of the rms speeds of hydrogen and nitrogen molecules at STP.
Solution:
According to Avogadro’s law, at STP the volume of 1 mol gas is 22.4L.
∴ Density of hydrogen \(\rho_{\mathrm{H}}=\frac{2}{22.4} \mathrm{~g} \cdot \mathrm{L}^{-1}\)
and density of nitrogen, \(\rho_{\mathrm{N}}=\frac{28}{22.4} \mathrm{~g} \cdot \mathrm{L}^{-1}\)
∴ The ratio of the rms speeds of hydrogen and nitrogen is,
⇒ \(\frac{c_{\mathrm{H}_2}}{c_{\mathrm{N}_2}}=\frac{\sqrt{\frac{3 p}{\rho_{\mathrm{H}}}}}{\sqrt{\frac{3 p}{\rho_{\mathrm{N}}}}}=\sqrt{\frac{\rho_{\mathrm{N}}}{\rho_{\mathrm{H}}}}=\sqrt{\frac{28}{2}}=\sqrt{\frac{14}{1}}=3.74: 1\)

